Open Journal of Soil Science
Vol.07 No.07(2017), Article ID:77472,7 pages
10.4236/ojss.2017.77009
Fractal Kinetics Parameters Regulating Carbon Decomposition Rate under Contrasting Soil Management Systems
Léon E. Parent
Department of Soils and Agrifood Engineering, Université Laval, Québec, Canada

Copyright © 2017 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: May 23, 2017; Accepted: July 4, 2017; Published: July 7, 2017
ABSTRACT
Agricultural soils can sequester and release large amounts of carbon. Accessibility of soil carbon to microbial attacks depends on biological, chemical, and physical protection mechanisms such as organic matter composition and particle size, soil aggregation, and chemical protection through the silt-clay- organic matter complex. While soil and organic matter are fractal objects controlling exposure of reactive surfaces to the environment, soil aggregation and biomass production and quality are regulated by agricultural practices. Organic matter decomposition in soil is generally described by the classical first-order kinetics equations fitted to define distinct carbon pools. By comparison, fractal kinetics assigns a coefficient to adjust time-dependent decomposition rate of total soil carbon to protection mechanisms. Our objective was to relate fractal parameters of organic matter decomposition to soil management systems. Retrieving published data, the decomposition of organic matter was modeled in a silt loam soil maintained under pasture, annual cropping or bare fallow during 11 years. The classical first-order kinetics model returned quadratic relationships indicating that reactive carbon decreased with time. Fractal kinetics rectified the relationships successfully. Initial decomposition rate (k1 at t = 1) was 7 × 10−4 for pasture, 1 × 10−4 for annual cropping, and 0.5 × 10−4 for bare-soil fallow. Fractal coefficients h were 0.71, 0.45, and 0.25 for pasture, annual cropping and fallow, respectively. Due to aggregation, physical protection against microbial attacks was highest under pasture management, leading to higher carbon sequestration despite higher biomass production and “priming” effects. Parameters k1 and h proved to be useful indicators for soil quality classification integrating the opposite effects of labile carbon decomposition and carbon protection mechanisms that regulate the decomposition rate of organic matter with time as driven by soil management practices.
Keywords:
Aggregation, Annual Cropping, Carbon Pools, Carbon Sequestration, Fallow, Fractal Coefficient, Pasture, Soil Quality

1. Introduction
The soil is a huge reservoir of organic carbon approximately three times as large as the vegetation of terrestrial ecosystems and twice that of the atmosphere [1] . Properly managed soils can mitigate climate change through carbon sequestration and enhanced soil quality. Organic matter decomposition in soils is generally modeled by first-order kinetics that assigns rate coefficients to carbon pools defined by the model as labile to recalcitrant to decomposition [2] [3] [4] [5] [6] . The classical first-order kinetics equation assumes that each reaction rate is constant and that the mixture is homogeneous and fully dispersed [7] .
However, most reactions in nature are fractal because they occur on low-di- mensional heterogeneous surfaces where substrate accessibility, hence reaction rate, decreases with time [7] [8] [9] . Soil and organic matter are fractal objects [10] [11] that interact with each other [12] . Organic matter is a mixture of objects of various sizes and biochemical compositions [6] [13] . In batch reactions, surface area of particles and substrate reactivity per unit surface are enhanced by shredding and grinding, and by agitating the mixture [7] . Fractal kinetics [7] provides a means to regulate organic matter decomposition rate because carbon accessibility to microbial attacks changes with time due to “priming effect” of labile carbon [14] [15] [16] and to biological, chemical and physical protection mechanisms in the soil in situ [12] [17] . Indeed, biochemical composition of organic particles, organic particle size, soil aggregation and the silt-clay-organic matter complex limit surface areas of labile and recalcitrant organic matter materials. Biomass production and quality and soil aggregation are regulated by agricultural practices.
A hierarchical soil aggregation model for physical protection of organic matter against microbial attacks in a fractal soil system has been conceptualized by [18] , described numerically by fractal [10] and Euclidean [19] geometry, and illustrated by [20] . Plant residues and fungi decompose into fragments and various substances, providing a nucleus for the formation of micro-aggregates less than 250 µm in diameter within macro-aggregates [21] . The micro-aggregates are mechanically strong while macro-aggregates may be destroyed by agricultural practices. By assigning a power coefficient to time to regulate the carbon decomposition rate in soils [22] [23] [24] [25] , fractal kinetics can quantify the effect of tillage and crop rotation practices on enhancing or decreasing protection mechanisms against organic matter decomposition.
The aim of this paper was to relate fractal parameters of organic matter decomposition to agricultural practices regulating carbon sequestration in soils.
2. Material and Methods
2.1. Fractal Kinetics
First-order kinetics describes reactant disappearance as follows:
 (1)
(1)
where A is concentration of the reactant remaining at time t and k is the first- order rate constant. The analytical solution of Equation (1) is as follows:
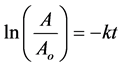 (2)
(2)
where A concentration at time t is expressed as the proportion of initial reactant concentration Ao.
The rate “constant” k for reactions in diffusion-limited heterogeneous systems such as fractal objects has been shown both phenomenologically and theoretically to decrease with time as follows [7] :
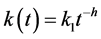 (3)
(3)
where h is a fractal coefficient (0 ≤ h ≤ 1, t ≥ 1) and k1 is rate coefficient at t = 1. If , reaction rate is maximum and kinetics gets closer to classical first-or- der Equation (2). Otherwise, the reaction follows fractal kinetics.
, reaction rate is maximum and kinetics gets closer to classical first-or- der Equation (2). Otherwise, the reaction follows fractal kinetics.
In organic matter decomposition studies, the fractal power coefficient p reduces reaction rate over time as follows [11] [22] [23] [24] [25] :
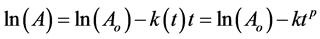 (4)
(4)
where p = 1 − h. The value of h is a measure of protection mechanisms against organic matter decomposition, and k1 is maximum reaction rate at t = 1.
2.2. Computational Example
A computational example was retrieved from literature [26] . Briefly, a silt loam soil maintained under pasture, annual cropping or bare fallow during 11 consecutive years was sieved to less than 4 mm, incubated in the laboratory for 98 days, and monitored for CO2 production. The 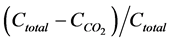 ratio, where
ratio, where  is total carbon concentration and
is total carbon concentration and 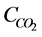 is cumulative CO2 released during the incubation period, was log-transformed, then related to t for classical fractal kinetics or to
is cumulative CO2 released during the incubation period, was log-transformed, then related to t for classical fractal kinetics or to 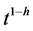 for fractal first-order kinetics. The value of h was iterated between 0 and 1 using Microsoft Excel until maximum r2 value.
for fractal first-order kinetics. The value of h was iterated between 0 and 1 using Microsoft Excel until maximum r2 value.
3. Results and discussion
3.1. Classical First-Order Kinetics
The soil under pasture released the largest amount of CO2. The classical first- order kinetics showed significantly quadratic trends (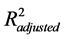 = 0.997 - 0.999) across treatments (Figure 1). Therefore, the reaction rates decreased with time, indicating that reactive surfaces became less accessible [7] . Classical first-order kinetics addresses this problem by splitting the curve into carbon pools (Thuriès
= 0.997 - 0.999) across treatments (Figure 1). Therefore, the reaction rates decreased with time, indicating that reactive surfaces became less accessible [7] . Classical first-order kinetics addresses this problem by splitting the curve into carbon pools (Thuriès
Figure 1. First-order kinetics of organic matter decomposition in soil under different soil management practices (bold colored lines) showing quadratic relationships (thin black lines). Data retrieved from [26].
et al., 2001). Because the clay-silt complex was similar across soils, microbial access to carbon pools was regulated by organic matter composition and encapsulation within aggregates. The soil under pasture that favored aggregation contained the largest amount of easily decomposable polysaccharides from recent plant residues while that under fallow showed smaller carbon content with higher concentration of recalcitrant polyphenols [26] . The sand fraction contained more easily biodegradable carbon forms compared to silt or clay.
3.2. Fractal kinetics
There were highly significant linear relationships between soil organic matter decomposition and  (Table 1). Initial decomposition rate k1 was 14 times larger in the soil under pasture compared to bare-soil fallow, indicating large differences in labile carbon content due to higher biomass production under pasture. However, the higher was the h value, the smaller was
(Table 1). Initial decomposition rate k1 was 14 times larger in the soil under pasture compared to bare-soil fallow, indicating large differences in labile carbon content due to higher biomass production under pasture. However, the higher was the h value, the smaller was . Soil carbon decomposing at reduced rate needs not to be classified into carbon pools because fractal coefficient h not only accounts for chemical and physical protection but also for the recalcitrance of residual carbon that increases with time. A single total C pool decomposing at rate
. Soil carbon decomposing at reduced rate needs not to be classified into carbon pools because fractal coefficient h not only accounts for chemical and physical protection but also for the recalcitrance of residual carbon that increases with time. A single total C pool decomposing at rate 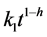 sufficed to describe organic matter decomposition in a fractal soil. Biochemical composition of organic matter materials provided an explanation for reduced reaction rate.
sufficed to describe organic matter decomposition in a fractal soil. Biochemical composition of organic matter materials provided an explanation for reduced reaction rate.
Despite “priming” effects by labile polysaccharides [14] [15] [16] , carbon sequestration was highest under pasture management due to high biomass production by the sod and physical protection against microbial attacks through soil aggregation. In contrast, the h coefficient was lowest in the degraded bare-soil fallow where aggregation was low and biochemical carbon protection as polyphenols was high. The final result was carbon accumulation under pasture management compared to carbon depletion in the bare-soil fallow. As expected from the theory on carbon sequestration [18] [20] and in conformity with the fractal hypothesis [7] , CO2 release during organic matter decomposition is accelerated
Table 1. Fractal first-order parameters of organic matter decomposition in soil (data retrieved from [26] .
under conventional tillage that destroys soil aggregates and increases the exposure to microbial attacks of the formerly aggregate-protected organic matter [27] . As fractal coefficient decreased and soil particles were dispersed under fallow, the course of organic matter decomposition approached that of classical first- order kinetics. As fractal coefficient h increased due to soil aggregation, more carbon could be sequestered in the soil. The effect of conservation practices [28] and crop rotation [4] [29] on carbon sequestration can thus be compared using the h parameter.
Fractal parameters k1 and h could also be used as soil quality indicators linked to soil functions like water regulation and partitioning, soil filtering and buffering, and nutrient storing and cycling [30] . High k1 values reflected high respiration rate and microbial biomass, hence high biological activity. High h values reflected high organic carbon accumulation rate, content of particulate organic carbon, cation exchange capacity, and aggregation. As shown by k1 and h, biological activity and aggregation, hence soil quality, were highest in the soil under pasture management and lowest in the soil under fallow.
4. Conclusion
The classical first-order kinetics that describes the decomposition of carbon pools at specific rate constants assumes that the medium is homogeneous and agitated. However, the soil is heterogeneous and structured, often showing fractal geometry. Fractal kinetics described successfully the course of total carbon decomposition in a fractal soil. Initial decomposition rate was highest in the pasture soil, which was well supplied with polysaccharides, and lowest in the fallow soil enriched in polyphenols. The pasture soil showed the highest h value due to higher aggregation that protects organic matter against microbial attacks. The h value regulated reaction rate as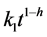 , allowing organic matter to accumulate in the pasture soil despite higher initial decomposition rate compared with annual cropping and bare-soil fallow. Fractal parameters reflected soil quality and the effect of agricultural practices on soil carbon sequestration and release rates.
, allowing organic matter to accumulate in the pasture soil despite higher initial decomposition rate compared with annual cropping and bare-soil fallow. Fractal parameters reflected soil quality and the effect of agricultural practices on soil carbon sequestration and release rates.
Acknowledgements
The author thanks the Natural Sciences and Engineering Research Council of Canada (NSERC-DG 2254) for financial support.
Cite this paper
Parent, L.E. (2017) Fractal Kinetics Parameters Regulating Carbon Decomposition Rate under Contrasting Soil Management Systems. Open Journal of Soil Science, 7, 111-117. https://doi.org/10.4236/ojss.2017.77009
References
- 1. Zhi, J., Jing, C., Lin, S., Zhang, C., Liu, Q., et al. (2014) Estimating Soil Organic Carbon Stocks and Spatial Patterns with Statistical and GIS-based Methods. PLoS ONE, 9, e97757. https://doi.org/10.1371/journal.pone.0097757
- 2. Andrén, O. and Katterer, T. (1997) ICBM: The Introductory Carbon Balance Model for Exploration of Soil Carbon Balances. Ecological Modelling, 7, 1226-1236.https://doi.org/10.1890/1051-0761(1997)007[1226:iticbm]2.0.co;2
- 3. Coleman, K. and Jenkinson, D.S. (2014) RothC—A Model for the Turnover of Carbon in Soils. Model Description and User Guide. Rothamsted Research, Harpenden, UK. https://www.rothamsted.ac.uk/sites/default/files/RothC_guide_WIN.pdf
- 4. La Scala Jr., N., Lopes, A., Spokas, K., Archer, D.W. and Reicosky, D. (2009) First-Order Decay Models to Describe Soil C-CO2 Loss after Rotary Tillage. Scientia Agricola, 66, 650-657. https://doi.org/10.1590/S0103-90162009000500010
- 5. Parton, W.J., Schimel, D.S., Cole, C.V. and Ojima, D.S. (1987) Analysis of Factors Controlling Soil Organic Matter Levels in Great Plains Grasslands. Soil Science Society of America Journal, 51, 1173-1179. https://doi.org/10.2136/sssaj1987.03615995005100050015x
- 6. Thuriès, L., Pansu, M., Feller, C., Herrmann, P. and Rémy, J.C. (2001) Kinetics of Added Organic Matter Decomposition in a Mediterranean Sandy Soil. Soil Biology and Biochemistry, 33, 997-1010. https://doi.org/10.1016/S0038-0717(01)00003-7
- 7. Kopelman, R. (1988) Fractal Reaction Kinetics. Science, 241, 1620-1625.https://doi.org/10.1126/science.241.4873.1620
- 8. Kopelman, R. (1986) Rate Process on Fractals: Theory, Simulations, Experiments. Journal of Statistical Physics, 42, 185-200. https://doi.org/10.1007/BF01010846
- 9. Savageau, M.A. (1995) Michaelis-Menten Mechanism Reconsidered: Implication of Fractal Kinetics. Journal of theoretical Biology, 176, 115-124.https://doi.org/10.1006/jtbi.1995.0181
- 10. Anderson, A.N., McBratney, A.B. and Crawford, J.W. (1998) Applications of Fractals to Soil Studies. Advances in Agronomy, 63, 1-76.
- 11. Dolgonosov, B.M. and Gubernatorova, T.N. (2007) Kinetics of the Enzymatic Decomposition of Macromolecules with a Fractal Structure. Theoretical Foundations of Chemical Engineering, 41, 868-877. https://doi.org/10.1134/S0040579507060127
- 12. Stewart, C.E., Plante, A.F., Paustian, K., Conant, R.T. and Six, J. (2008) Soil Carbon Saturation: Linking Concept and Measurable Carbon Pools. Soil Science Society of America Journal, 72, 379-392. https://doi.org/10.2136/sssaj2007.0104
- 13. Parent, S.-é. and Parent, L.E. (2015) Biochemical Fractionation of Soil Organic after Incorporation of Organic Residues. Open Journal of Soil Science, 5, 135-143. https://doi.org/10.4236/ojss.2015.56013
- 14. Chen, R., Senbayram, M., Blagodatsky, S., Myachina, O., Dittert, K., Lin, X., Blagodatskaya, E. and Kuzyakov, Y. (2014) Soil C and N Availability Determine the Priming Effect: Microbial N Mining and Stoichiometric Decomposition Theories. Global Change Biology, 20, 2356-2367. https://doi.org/10.1111/gcb.12475
- 15. Kuzyakov, Y. and Blagodatskaya (2015) Microbial Hotspots and Hot Moments in Soil: Concept & review. Soil Biology and Biochemistry, 83, 184-199.
- 16. Kuzyakov, Y., Friedel, J.K. and Stahr, K. (2000) Review of Mechanisms and Quantification of Priming Effects. Soil Biology and Biochemistry, 32, 1485-1498.
- 17. Plante, A.F. and McGill, W.B. (2002) Soil Aggregate Dynamics and the Retention of Organic Matter in Laboratory-Incubated Soil with Differing Simulated Tillage Frequencies. Soil and Tillage Research, 66, 79-92.
- 18. Oades, J.M. (1984) Soil Organic Matter and Structural Stability: Mechanisms and Implications for Management. Plant and Soil, 76, 319-337. https://doi.org/10.1007/BF02205590
- 19. Parent, L.E., de Almeida, C.X., Hernandes, A., Egozcue, J.J., Gülser, C., Bolinder, M.A., et al. (2012) Compositional Analysis for an Unbiased Measure of Soil Aggregation. Geoderma, 179-180, 123-131.
- 20. Six, J., Bossuyt, H., Degryze, S. and Denef, K. (2004) A History of Research on the Link between (Micro) Aggregates, Soil Biota, and Soil Organic Matter Dynamics. Soil and Tillage Research, 79, 7-31.
- 21. Six, J., Elliott, E.T. and Paustian, K. (2000) Soil Macroaggregate Turnover and Microaggregate Formation: a Mechanism for C Sequestration under No-Tillage Agriculture. Soil Biology and Biochemistry, 32, 2099-2103.
- 22. Bosatta, E. and Agren, G.I. (1991) Dynamics of Carbon and Nitrogen in the Organic Matter of the Soil: a Generic Theory. The American Naturalist, 138, 227-245. https://doi.org/10.1086/285213
- 23. Kelly, J.M. and Beauchamp, J.J. (1987) Mass Loss and Nutrient Changes in Decomposing Upland oak and Mesic Mixed-Hardwood Leaf Litter. Soil Science Society of America Journal, 51, 1616-1622. https://doi.org/10.2136/sssaj1987.03615995005100060038x
- 24. Middleburg, J.J. (1989) A Simple Rate Model for Organic Matter Decomposition in Marine Sediments. Geochimicaet Cosmochimica Acta, 53, 1577-1581.
- 25. Wider, R.K. and Lang, G.E. (1982) A Critique of the Analytical Methods Used in Examining Decomposition Data Obtained from Litterbags. Ecology, 63, 1636-1642. https://doi.org/10.2307/1940104
- 26. Gregorich, E.G., Gillepsie, A.W., Beare, M.H., Curtin, D., Sanei, H. and Yanni, S.F. (2015) Evaluating Biodegradability of Soil Organic Matter by Its Thermal Stability and Chemical Composition. Soil Biology and Biochemistry, 91, 182-191.
- 27. Beare, M.H., Cabrera, M.L., Hendrix, P.F. and Coleman, D.C. (1994) Aggregate-Protected and Unprotected Organic Matter Pools in Conventional- and No-Tillage Soils. Soil Science Society of America Journal, 58, 787-795. https://doi.org/10.2136/sssaj1994.03615995005800030021x
- 28. Curtin, D., Wang, H., Selles, F., McConkey, B.G. and Campbell, C.A. (2000) Tillage Effects on Carbon Fluxes in Continuous Wheat and Fallow-Wheat Rotations. Soil Science Society of America Journal, 64, 2080-2086. https://doi.org/10.2136/sssaj2000.6462080x
- 29. Wright, A.L. and Hons, F.M. (2004) Soil Aggregation and Carbon and Nitrogen Storage under Soybean Cropping Sequences. Soil Science Society of America Journal, 68, 507-513. https://doi.org/10.2136/sssaj2004.5070
- 30. Karlen, D.L., Andrews, S.S. and Doran, J.W. (2001) Soil Quality: Current Concepts and Applications. Advances in Agronomy, 74, 1-40.



