Open Journal of Acoustics
Vol.06 No.04(2016), Article ID:72679,10 pages
10.4236/oja.2016.64004
On Propagation of Rayleigh Type Surface Wave in a Micropolar Piezoelectric Medium
Baljeet Singh1, Ritu Sindhu2
1Department of Mathematics, Post Graduate Government College, Chandigarh, India
2Department of Mathematics, Maharshi Dayanand University, Rohtak, India

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: September 12, 2016; Accepted: December 9, 2016; Published: December 12, 2016
ABSTRACT
In the present paper, the governing equations of a linear transversely isotropic micropolar piezoelectric medium are specialized for x-z plane after using symmetry relations in constitutive coefficients. These equations are solved for the general surface wave solutions in the medium. Following radiation conditions in the half-space, the particular solutions are obtained, which satisfy the appropriate boundary conditions at the stress-free surface of the half-space. A secular equation for Rayleigh type surface wave is obtained. An iteration method is applied to compute the non-dimensional wave speed of the Rayleigh surface wave for specific material parameters. The effects of piezoelectricity, non-dimensional frequency and non-dimensional material constant, charge free surface and electrically shorted surface are shown graphically on the wave speed of Rayleigh wave.
Keywords:
Piezoelectric Medium, Micro-Rotation, Transverse Isotropy, Rayleigh Wave, Wave Speed

1. Introduction
The materials possessing linear coupling between mechanical and electric fields are termed as piezoelectric materials. Wave propagation in piezoelectric media has numerous applications in various fields of engineering. Some problems about propagation of plane waves in piezoelectric medium are studied by Kyame [1] , Pailloux [2] and Hruska [3] . Various other problems related to the phenomena of reflection and refraction of plane waves in piezoelectric materials are studied by Auld [4] , Parton and Kudryavtsev [5] , Galassi, et al. [6] , Singh [7] and Sharma [8] . Recently Salah et al. [9] studied the propagation of Rayleigh waves in a functionally graded piezoelectric material half-space.
Eringen [10] [11] [12] introduced the micro-continuum field theories of solids with electro-magnetic and thermal interactions. Craciun [13] formulated the basic equations of the linear theory of piezoelectric micropolar thermoelasticity with quasi-static electric fields. Ciumasu and Vieru [14] presented the variational formulation for the free vibration of a micropolar piezoelectric body. Zhilin [15] developed a theory of the micropolar piezoelectric materials. Iesan [16] established a uniqueness result and a reciprocal theorem in the linear theory of microstretch piezoelectricity. Aouadi [17] considered the linear dynamic theory of micropolar piezoelectricity and established a reciprocity relation with two processes at different instants. Gales [18] considered the linear theory of micromorphic piezoelectricity and formulated the initial boundary value problem and presented some uniqueness results. Chen [19] derived the linear constitutive equations for micropolar electromagnetic elastic solids.
The propagation of surface waves in a transversely isotropic micropolar piezoelectric medium is not attempted so far. Following Aouadi [17] , the governing equations for a transversely isotropic micropolar piezoelectric medium are formulated in x-z plane and are solved for possible surface waves. After considering the required radiation conditions in half-space and boundary conditions at free surface, a secular equation for non-dimensional wave speed of Rayleigh surface wave is obtained. The dependence of non-dimensional wave speed on frequency, material constants and electric field is shown graphically.
2. Governing Equations and Solution
We consider a homogeneous and transversely isotropic micropolar piezoelectric half space. We take the origin of the coordinate system on the free surface and the positive z axis along the normal into the half-space . We assume the components of the displacement and microrotation vectors of the form
. We assume the components of the displacement and microrotation vectors of the form 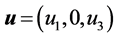 and
and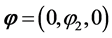 . Using symmetry relations in the coefficients, the governing equations given in Aouadi [17] are specialized for x-z plane in the following from after a lengthy calculation
. Using symmetry relations in the coefficients, the governing equations given in Aouadi [17] are specialized for x-z plane in the following from after a lengthy calculation
 (1)
(1)
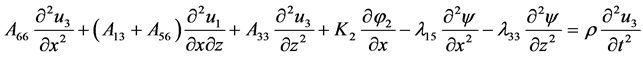 (2)
(2)
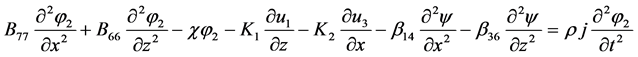 (3)
(3)
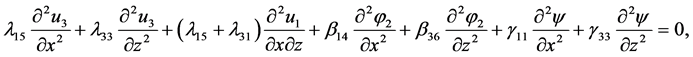 (4)
(4)
where 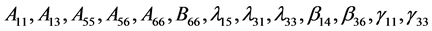 are constitutive coefficients.
are constitutive coefficients. .
.
We seek the surface wave solution of Equations (1) to (4) in the following form
 (5)
(5)
Making use of Equation (5) in Equations (1) to (4) and applying the radiation conditions ,
, 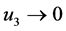 ,
, 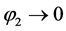 ,
, 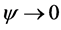 as
as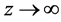 , we obtain the following particular solutions in half-space
, we obtain the following particular solutions in half-space
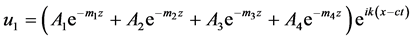 (6)
(6)
 (7)
(7)
 (8)
(8)

where the expressions for coupling coefficients


3. Boundary Conditions
The appropriate boundary conditions at 

And vanishing of electric displacement component or electric potential


where

4. Secular Equations
The particular solutions (6) to (9) satisfy the boundary conditions (10) and (11) at the free surface 

where

Or 
5. Particular Cases
a) The secular Equation (12) reduces for a transversely isotropic micropolar elastic case when
b) The secular Equation (12) reduces for a transversely isotropic piezoelectric case when
6. Numerical Results and Discussion
For numerical computation of non-dimensional wave speed of Rayleigh wave, the following relevant physical constants of a transversely isotropic micropolar piezoelectric material are considered
For above physical constants and by using a Fortran program of Iteration method, the secular Equation (12) is solved numerically to obtain the non-dimensional speed

constant.
The variation of non-dimensional speed 

electrically shorted (ES) cases. For CF case, the value of speed at 



Figure 1. Variation of non-dimensional speed 

shorted surface on non-dimensional speed of the Rayleigh wave in a transversely isotropic micropolar piezoelectric solid half-space.
The variation of non-dimensional speed 

observe the piezoelectric effects. The variation non-dimensional speed as shown by solid line (transversely isotropic micropolar piezoelectric case) in Figure 2 is same as shown in Figure 1. For transversely isotropic micropolar case, the variation of non-dimensional speed is shown by dotted line in Figure 2. It has value 2.2224 at 

The variation of non-dimensional speed 

Figure 2. Piezoelectric effect on non-dimensional speed 

Figure 3. Variation of non-dimensional speed 


and 15. For













(
7. Conclusion
Using symmetry relations in constitutive coefficients and assuming the components of the displacement and microrotation vectors in the form 

Cite this paper
Singh, B. and Sindhu, R. (2016) On Propagation of Rayleigh Type Surface Wave in a Micropolar Piezoelectric Medium. Open Journal of Acoustics, 6, 35- 44. http://dx.doi.org/10.4236/oja.2016.64004
References
- 1. Kyame, J.J. (1949) Wave Propagation in Piezoelectric Crystals. Journal of Acoustical Society of America, 21, 159-167.
https://doi.org/10.1121/1.1906490 - 2. Pailloux, P.M.H. (1958) Piezoelectric itecalcul des vitesses de propagation. Le Journal de Physique etle Radium, 19, 523-526.
https://doi.org/10.1051/jphysrad:01958001905052300 - 3. Hruska, K. (1966) The Rate of Propagation of Ultrasonic Waves in ADP and in Voigt’s Theory. Czechoslovak Journal of Physics, B16, 446-453.
https://doi.org/10.1007/BF01696256 - 4. Auld, B.A. (1973) Acoustic Field and Waves in Solids. Wiley Interscience Publication, New York.
- 5. Parton, V.Z. and Kudryavtsev B.A. (1988) Electromagnetoelasticity: Piezoelectrics and Electrically Conductive Solids. Gordan and Breach Science Publishers, New York.
- 6. Galassi, C., Dinescu, M., Uchino, K. and Sayer, M. (2000) Piezoelectric Materials: Advances in Science, Technology and Applications (Nato Science Partnership Subseries 3). Springer, Berlin.
- 7. Singh, B. (2010) Wave Propagation in a Prestressed Piezoelectric Half-Space. Acta Meccanica, 211, 337-344.
https://doi.org/10.1007/s00707-009-0234-8 - 8. Sharma, M.D. (2010) Piezoelectric Effect on the Velocities of Waves in an Anisotropic Piezo-Poroelastic Medium. Proceedings of the Royal Society A (Mathematical, Physical Engineering Sciences), 466, 1977-1992.
https://doi.org/10.1098/rspa.2009.0534 - 9. Salah, B., Njeh, A. and Ghozlen, M.H.B. (2012) A Theoretical Study of Propagation of Rayleigh Waves in Functionally Graded Piezoelectric Material (FGPM). Ultrasonics, 52, 306-314.
https://doi.org/10.1016/j.ultras.2011.08.016 - 10. Eringen, A.C. (1999) Microcontinuum Field Theories. I. Foundations and Solids. Springer, New York.
https://doi.org/10.1007/978-1-4612-0555-5 - 11. Eringen, A.C. (2003) Contiinuum Theory of Micromorphic Electromagnetic Thermoelastic Solids. International Journal of Engineering Science, 41, 653-665.
https://doi.org/10.1016/S0020-7225(02)00274-4 - 12. Eringen, A.C. (2004) Electromagnetic Theory of Microstretch Elasticity and Bone Modelling. International Journal of Engineering Science, 42, 231-242.
https://doi.org/10.1016/S0020-7225(03)00288-X - 13. Craciun, I.A. (1995) Uniqueness Theorem in the Linear Theory of Piezoelectric Micropolar Thermoelasticity. International Journal of Engineering Science, 33, 1027-1036.
https://doi.org/10.1016/0020-7225(94)00106-T - 14. Ciumasu, S.G. and Vieru, D. (1999) Variational Formulations for the Vibration of a Micropolar Piezoelectric Body. Journal of Acoustical Society of America, 105, 1240-1245.
https://doi.org/10.1121/1.425960 - 15. Zhilin, P.A. and Kolpakov, Y.E. (2005) A Micro-Polar Theory for Piezoelectric Materials, Lecture at XXXIII Summer School—Conference “Advanced Problems in Mechanics”, St. Petersburg.
- 16. Iesan, D. (2006) On the Microstretch Piezoelectricity. International Journal of Engineering Science, 44, 819-829.
https://doi.org/10.1016/j.ijengsci.2006.05.007 - 17. Aouadi, M. (2008) Aspects of Uniqueness in Micropolar Piezoelectric Bodies. Mathematics and Mechanics of Solids, 13, 499-512.
https://doi.org/10.1177/1081286507077106 - 18. Gales, C. (2012) Some Results in Micromorphic Piezoelectricity. European Journal of Mechanics—A/Solids, 31, 37-46.
https://doi.org/10.1016/j.euromechsol.2011.06.014 - 19. Chen, J. (2013) Micropolar Theory of Flexoelectricity. Journal of Advanced Mathematics and Applications, 1, 1-6.
Nomenclature











Appendix
The relations between 

and

Submit or recommend next manuscript to SCIRP and we will provide best service for you:
Accepting pre-submission inquiries through Email, Facebook, LinkedIn, Twitter, etc.
A wide selection of journals (inclusive of 9 subjects, more than 200 journals)
Providing 24-hour high-quality service
User-friendly online submission system
Fair and swift peer-review system
Efficient typesetting and proofreading procedure
Display of the result of downloads and visits, as well as the number of cited articles
Maximum dissemination of your research work
Submit your manuscript at: http://papersubmission.scirp.org/
Or contact oja@scirp.org
















































