World Journal of Engineering and Technology
Vol.03 No.03(2015), Article ID:57799,7 pages
10.4236/wjet.2015.33009
Rain Attenuation Effects on 2.6 GHz WiMAX Networks Deployment in Ghana
Patrick Fiati
Department of Electrical, Electronic Engineering, Kwame Nkrumah University of Science and Technology, Kumasi, Ghana
Email: patrickfiati@yahoo.com
Copyright © 2015 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 7 May 2015; accepted 5 July 2015; published 8 July 2015
ABSTRACT
WiMAX communication systems operating at 2.6 G frequencies are used for broadband multimedia and internet based services. At these frequencies, the signal will be affected by various propagation impairments such as rain attenuation, cloud attenuation, tropospheric scintillation, ionospheric scintillation, water vapour attenuation, and rain and ice depolarization. Among all the pro- pagation impairments, rain attenuation is the most important and critical parameter. In this research, rain attenuation is calculated at KNUST, Kumasi using ITU-R rain attenuation model. The preliminary results of the work will be used to calculate the attenuation experimentally and comparison can be made, which helps to develop a new rain attenuation model at 2.6 G bands. Rain attenuation is an important aspect of signal propagation above 2.6 GHz frequency. The attenuation time series generation from point rain rate measurement is crucial due to unavailability of actual signal measurements. In this research, a simple and realistic approach has been demonstrated for better estimation of rain attenuation using WiMAX-band signal propagation data and ground rain rate measurements in Ghana. The ITU-R model of rain attenuation has been modified by incorporating an effective slant path model. The effective slant path has been estimated and modeled in terms of a power-law relationship of rain rate data of 2007-2008. The methodology has been validated with the measured data of 2014. Comparison with ITU-R and GMET clearly demonstrates the improved predictability of the proposed model at the present tropical location.
Keywords:
Rain Attenuation, ITU-R Model, Rain Fall Rate, WiMAX, Alamouti

1. Introduction
Atmospheric effects play a major role in the design of satellite-to-earth links operating at frequencies above 2.6 GHz. Raindrops absorb and scatter radio waves, leading to signal attenuation and reduction of the system availa- bility and reliability. The severity of rain impairment increases with frequency and varies with regional locations [1] . Hence the incidence of rainfall on radio links becomes even more important for frequencies as low as about 7 GHz particularly in the tropical and equatorial climates, where intense rainfall events are common [2] . It is therefore very important when planning both microwave and terrestrial line-of-sight system links; to make an accurate prediction of rain induced attenuation on propagation paths [3] .
Initially, attenuation prediction attempts involved extrapolation of measurements to other locations, frequen- cies, and elevation angles; however, the complex nature and regional variability of rain make this approach highly inaccurate [4] . The method for the prediction of rain attenuation on microwave paths has been grouped into two classes: the empirical method which is based on measurement databases from stations in different climatic zones within a given region and the physical method which make an attempt to reproduce the physical behaviour involved in the attenuation process.
However, when a physical approach is used not all the input parameters needed for the analysis is available. Empirical method is therefore the most used methodologies [5] [6] . For the empirical methodology, an appro- priate distribution of rainfall rate at 1-minute integration time is needed for the site under studied in order to predict accurate rain attenuation for the location. This input is sometime provided by meteorological and envi- ronmental agencies, universities, and independent researchers.
Study has revealed that daily rainfall accumulations are universally recorded and hourly data are fairly available by national weather bureaus/environmental agencies [7] . There is still dearth of rainfall rate of 1-minute integration time necessary for the study of rain induced impairment to telecommunication especially in the tro- pical region (Ghana). This is because global national weather services are established to satisfy more traditional requirements such as those for agriculture, hydrology and forest management. A method for converting the available rain rate data to the equivalent 1-minute rain rate cumulative distribution is therefore necessary.
The critical role of the propagation impairment on communication systems cum lack of rain-measurement data from tropical regions for verification for modeling purposes has been the concern of many organizations like, the International Telecommunication Union (ITU), European Space Agency (ESA), and European co- operative program (COST) among others. This has become necessary because of the peculiarity of the tropical regions, which are characterized by high intensity rainfall, enhanced frequency of rain occurrence and the increased presence of large raindrops when compared with temperate climates [8] . Another very important effort towards gathering more information is through Tropical Rain Measurement Mission (TRMM) jointly developed by the United States and Japan, and the Global Precipitation Climatology Project (GPCP) of the World Climatic Research Programme (WCRP). As earlier stated, the data available from this mission can not directly be employed in system design, due to its long integration time. The aim of this research is to give additional tools to the system designers, in the form of contour maps of rain intensity and rain attenuation, for the design of WiMAX systems in the tropical countries particularly in Ghana.
Rain-rate and rain attenuation maps for the country of Ghana were developed using the models purposely designed for tropical zones by Moupfouma and Martins [9] (which is a mix between a log-normal distribution for low rain rates and a gamma distribution for high rain rates) and that of J. Chebils [10] model for the estimation of point rain rate, and the ITU model for rain attenuation prediction method prediction method [11] . The climatic mapping of rain-rate and rain-attenuation has naturally attracted a great deal of attention for instance this kind of work has earlier been carried out for USA [12] , Europe, Malaysia, Colombia and on global scale by ITUR [13] , Salonen and Baptisa, Crane, 1996.
Efforts has also been made by Fiati et al. to obtain 1 minute rain rate map for Ghana using Rice-Holmberg model however the model overestimates rain rates in the high-availability range (0.01%), and underestimates in the range between 0.1% to 1%. These percentages unavailability of time are crucial for communication purposes, hence the need for this work.
2. Analysis
Recent analysis suggests that the rain rate distribution is better described by a model which approximates a log- normal distribution at the low rates, and a gamma distribution at high rain rate. This kind of model was deve- loped by Moupfouma and Martins [14] . Thus, the Moupfouma model requires three parameters; λ, γ and R0.01. The first two parameters have been provided. To estimate R0.01, the use of J. Chebils model [15] appears suitable, it allows the usage of long-time mean annual accumulation, M, at the location of interest. The power law relationship of the model is given by R0.01 = αMβ (4) where α and β are regression coefficients. Chebil has made a comparison between some models based on measured values of R0.01 and M in Malaysia, Indonesia, Brazil, Singapore and Vietnam. He showed that his model is the best estimate of the measured data [16] .
The regression coefficient α and β are defined as α = 12.2903 and β = 0.2973 (5). Thus, using the refined Moupfouma model and Chebil model, the 1 min rain-rate cumulative distribution is fully determined from the longterm mean annual rainfall data.The input parameters needed for the model are: point rainfall rate for the location for 0.01% of an average year (mm/h), height above sea level of the Earth station (km), elevation angle, latitude of the Earth station (degree), frequency (GHz) and effective radius of the Earth (8500 km) [17] .
2.1. Formulations
Two Tx Antenna Schemes
One example of the Alamouti code encodes the Q = 2 complex symbols x1, x2 to be transmitted during T = 2 symbol periods, in the form
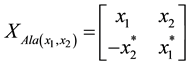
Tx Antennas: STTD
For Nr = 1 receive antenna, the optimal space time block code for two transmit antennas is STTD, with symbol rate .
.
Optimality is seen in many different ways. In flat fading, the received signal is
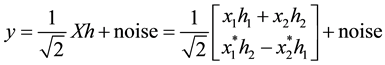
Here, the X is normalized by  so that the transmit power is
so that the transmit power is .
.
Conjugating the received signal
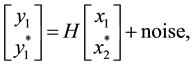
where the equivalent channel matrix is
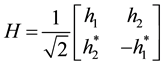
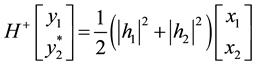
+ noise
where
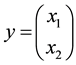

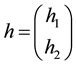
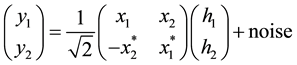

Conjugating the received signal,
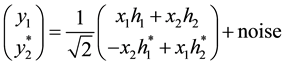
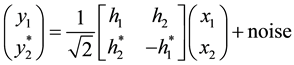
From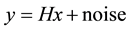 ,
,
where,
But,
From this,the complexity of the ML problem is in general exponential in the model dimension.
Here, the problem dimension is essentially dictated by the dimensions of the modulation matrix. With orthogonal designs, the off-diagonal elements of the equivalent correlation matrix vanish, and optimal ML detection is performed for each symbol independently of each other.
However, the equivalent correlation matrix for non-orthogonal schemes entertains non-zero off-diagonal elements, and this leads to a combinatorial optimization problem.
In space time coding problems, the ML solution is feasible if the dimensionality of R is sufficiently low, or it has some special structure that can be exploited. Unfortunately, a low model dimension tends to require either a low symbol rate or a small number of antennas.
The rain drop size distribution is exponentially expressed mathematically as

where 


The rainfall R is related to 


Step 1.
Calculate the rain height 

where 
Step 2.
Determine the slant-path length

Slant path through rain source: ITU-R 618-8 [18]
where θ is Elation angle in degrees, 
Step 3.
Obtain the horizontal projection, 

Step 4.
Determine the rainfall rate, 
Step 5.
Calculate the specific attenuation, 


where K and α depend on frequency, polarization, raidrop size distribution and temperature and obtained using,


where t is the polarization tilt angle relative to horizontal.
Step 6.
Determine the horizontal path adjustment factor, 

Step 7.
Calculate the adjusted rainy path length, 


where

Step 8.
Obtain the vertical redution factor

where


Step 9.
Determine the effective path length through rain, 

Step 10.
Calculate the predicted attenuation exceeded for 0.01% of an average year by using

Step 11.
The estimated attenuation to be exceeded for the other percentages of an average year, in the range 0.001% to 10% may then be estimated using 

where p is the percentage probability of interest and β is given by




2.2. Effects of Rain
The most well known effect of rain is that it attenuates the signal. The attenuation is caused by the scattering and absorption of electromagnetic waves by drops of liquid water. The scattering diffuses the signal, while absorption involves the resonance of the waves with individual molecules of water. Absorption increases the molecular energy, corresponding to a slight increase in temperature and results in an equivalent loss of signal energy. Attenuation is negligible for snow or ice crystals in which the molecules are tightly bound and do not interact with the waves.
3. Conclusions
Rain rate and rain attenuation contour maps have been developed for 0.1% and 0.01% of the time using the refined Moupfouma model for rain rate maps and ITU-R 618 for the rain attenuation maps over Ghana. The 0.1% of time of rain attenuation is needed for WiMAX network service-availability.
The information from these maps will be useful in the preliminary design for both terrestrial and earth- satellite microwave links, and to provide a broad idea of rain attenuation to microwave engineers for the proposed launching of 2.6 GHz WiMAX Network. Majority of the studies on Earth-space propagation have been conducted in Europe, the United States and Asia. But it will be more crucial for studies to be conducted in a tropical location like Kumasi, Accra,Tema-Ghana because of its high rainfall intensity. In order to compute reliable rain attenuation for a given location, an appropriate distribution of rainfall rate for the site is required. Rainfall rate statistics specified on a percent of time basis, that is the percent of time in a year or a month that the rain rate equals or exceeds a specific value is used in the rain attenuation prediction model. The ITU rain attenuation prediction method is based on 0.01% of a year rain rate parameter.
Data for the distribution must be based on long-term (typically more than 10 yrs) measured data with 1- minute integration time. But a large amount of rainfall data, typically collected by meteorological agencies in many countries is available for longer integration time such as 30 min., 60 min., etc. This is the case with Ghana, hence the need to rely on the data provided by ITU.
In this research, ITU-R model is used to predict the rainfall rate and attenuation due to rain, at KNUST, Ghana. The attenuation is calculated, for different rainfall rates and exceedence percentages of an average year. The preliminary results indicate that the attenuation increases with frequency and rainfall rate. These predicted values can be compared with the measured experimental data after installation of the setup in the location.
As for future works, we will consider (1) constructing a test bed to measure rain attenuation experimentally from measured data per 1min of integration time and comparing results with simulated equations of rain attenuation and (2) looking at other forms of atmospheric conditions that affect the signal strength of WiMAX networks such as dust particles and developing equations to calculate attenuation.
Cite this paper
PatrickFiati, (2015) Rain Attenuation Effects on 2.6 GHz WiMAX Networks Deployment in Ghana. World Journal of Engineering and Technology,03,83-90. doi: 10.4236/wjet.2015.33009
References
- 1. Choi, Y.S., Lee, J.H. and Kim, J.M. (1997) Rain Attenuation Measurements of the Koreasat Beacon Signal on 12 GHz. CLIMPARA98, Ottawa, Vol. 208, No. 211.
- 2. Characteristics of Precipitation for Propagation Modelling, Recommendation ITU-R P.837-4, ITU-R P Sers., ITU-R, Int. Telecomm. Union, Geneva, 2003.
- 3. Salonen, E.T. and Poiares-Baptista, J.P.V. (1997) A New Global Rainfall Rate Model. Proceedings of the 10th International Conference on Antennas and Propagation (Pub N 14-176-436), 2, 182-185.
- 4. Segal, B. (1986) The Influence of Raingauge Integration Time on Measured Rainfall-Intensity Distribution Functions. Journal of Atmospheric and Oceanic Technology, 3, 662-671.
- 5. Crane, R. and Dissanayake, A.W. (1997) ACTS Propagation Experiment: Attenuation Distribution Observations and Prediction Model Comparison. Proceedings of the IEEE, 85, 879-892.
- 6. Dutton, E.J. (1984) Microwave Terrestrial Link Rain Attenuation Prediction Parameter Analysis. US Department of Communications, National Telecommunications and Information Administration (STIA) Tech. Rep., Volumes 84-148.
- 7. Emiliani, L.D., Agudelo, J., Gutierrez, E., Restrepo, J. and Fradique-Mendez, C. (2004) Development of Rain-Attenuation and Rain Rate Maps for Satellite System Design in the Ku and Ka bands in Colombia. IEEE Antennas and Propagation Magazine, 46, 54-68.
- 8. Ajayi, G.O., Feng, S., Radicella, S.M. and Reddy, B.M. (1996) Handbook on Radio Propagation Related to Satellite Communications in Tropical and Subtropical Countries. ICTP, Trieste, 7-14.
- 9. Moupfouma, F. (1985) Model of Rainfall-Rate Distribution for Radio System Design. IEEE Proceedings, 132, 39-43.
- 10. Chebil, J. and Rahman, T.A. (1999) Development of 1 min Rain Rate Contour Maps for Microwave Applications in Malaysia Peninsula. Electronics Letters, 35, 1772-1774.
- 11. Gunes, M., Gunes, F. and Dimiller, K. (1994) Development of a Climatic Map of Attenuation by Rainfall for Turkey. Proceedings of the 7th Mediterranean Electrotechnology Conference, 2, 383-386.
http://dx.doi.org/10.1109/MELCON.1994.381078 - 12. Dutton, E.J. and Dougherty, H.T. (1979) Year-to-Year Variability of Rainfall for Microwave Applications in the USA. IEEE Transactions on Communications, 27, 829-832.
- 13. Specific Attenuation Model for Rain for Use in Prediction Methods. Recommendation P.838-1, 2, ITU-R P Sers., ITU-R, Int. Telecomm. Union, Geneva, 1999.
- 14. Crane, R.K. (1996) Electromagnetic Wave Propagation through Rain. Wiley Interscience, New York.
- 15. Gallois, A.P., Hartigan, P.P. and Bock, A.M. (1989) A Comparison of Slant Path Attenuation Models Applied to the Selection of Satellite Beacon Receiver Sites. Proceedings of the 6th International Conference on Antennas and Propagation, 2, 271-275.
- 16. Propagation Data and Prediction Methods Required for the Design of Earth-Space Telecommunications Systems. Recommendation P.618-8, ITU-R P Sers., ITU-R, Int. Telecomm. Union, Geneva, 1999.
- 17. Watson, P.A., Sathiaseelan, V. and Potter, B. (1981) Development of a Climatic Map of Rainfall Attenuation for Europe, No. 300, 134. Post Graduate School of Electrical and Electronic Engineering, University of Bradford, West Yorkshire, Rep.
- 18. ITU-R Rec. P.618-8, Propagation Data and Prediction Methods Required for the Design of Earth-Space Telecommunication Systems. International Telecommunications Union, Geneva, April 2003.

















