Optics and Photonics Journal
Vol.04 No.06(2014), Article ID:46707,15 pages
10.4236/opj.2014.46012
Designs of All-Optical Higher-Order Signed-Digit Adders Using Polarization- Encoded Based Terahertz- Optical-Asymmetric-Demultiplexer (TOAD)
Ali Hajjiah*, Abdullah Alqallaf, Abdallah Cherri
Department of Electrical Engineering, College of Engineering & Petroleum, Kuwait University, Safat, Kuwait
Email: *ali.hajjiah@ku.edu.kw
Copyright © 2014 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 1 February 2014; revised 2 March 2014; accepted 1 April 2014
ABSTRACT
Various designed circuits for multiple-valued all-optical arithmetic are demonstrated. The ter- ahertz-optical-asymmetric-demultiplexer (TOAD) switch is used as the basic structure unit in the proposed circuits due to its compact size, thermal stability, and low power operation. The designs of trinary and quaternary signed-digit numbers based adders are presented using different polarized states of light. These proposed polarization-encoded based adders use much less switches and their speeds are higher than the intensity-encoded counterparts. Further, it will be shown that one of the proposed trinary signed-digit adders is twice as fast as a recently reported modified signed-digit adder.
Keywords:
Multiple-Valued Signed-Digit, All-Optical Gates, Polarization-Encoding, Terahertz-Optical-Asymmetric-Demultiplexer (TOAD)

1. Introduction
Multiple-valued logic and arithmetic is being extensively investigated as being a promising choice for future digital and optical computing [1] -[10] . Redundant signed-digit is among the various multiple-valued number systems [11] . Attractions features of this choice are higher information transmission, higher data storage i.e. more logic density and higher processing speed due to the opportunity of having parallel computing algorithms [12] . The addition/subtraction of two signed-digit numbers restricts the carry propagation to one or two adjoining digits. Many researchers proposed optoelectronics implementations to achieve fast computing arithmetic [13] - [18] . On the other hand, the recent developments in all-optical switches that are capable of demultiplexing Tb/s pulse trains push the researchers to shift towards all-optical implementations to design ultra-fast logical and arithmetic units using binary and nonbinary algorithms [19] - [24] .
Recently, all-optical arithmetic operations based on terahertz-optical-asymmetric-demultiplexer (TOAD) switch were demonstrated [20] [22] . All-optical multiple logic gates (XOR, NOR, NAND) as well as binary half- and full-adders were reported using parallel TOAD structures. Further, with the help of TOAD switches, fast nonbinary adders were demonstrated [23] where the input and the control signals are all-optical in nature. The high speed is achieved due to the use of the nonlinear optical materials and the nonbinary modified signed- digit (MSD) number representation. In contrast to binary adder, the proposed circuits can be extended to n digits by employing additional stages without affecting the total addition time. Since the multiple-valued digits cannot be directly implemented using the two binary states of the TOAD switches, intensity-encoding schemes are used to transform the signed-digits into binary bits. More recently, the intensity-encoded MSD adder was improved in terms of its TOAD switches count and its speed by utilization of polarization-encoded scheme [24] .
Now since multiple-valued logic/arithmetic is being viewed as an interesting approach in optical information processing, we present, in this paper, design methods to implement trinary signed-digit (TSD) and quaternary signed-digit (QSD) arithmetic. TSD and QSD number systems are represented by five and seven literals, respectively. Consequently, the two-bit intensity-encoding (light, no light) scheme, as reported in [24] , cannot be used in designing the higher-order signed-digit (SD) adders. A straightforward solution is to use a three-bit intensity- encoding scheme for each SD literal. However, in this case the all-optical circuit implementation will be very complicated. Alternatively, this limitation is solved by proposing the polarized state of light (vertical and horizontal) and a two-bit encoding scheme. The polarization-encoding method is used to design fast all-optical TSD and QSD number based adders. The proposed adders are compared in terms of numbers of gate delays, TOAD switches, beam combiners, and polarization converters. Further, these circuits are compared to the previously reported MSD adder [24] . It will be shown that the TSD adder is two times faster than the MSD one; while the QSD adder speed is the same. Sections 2 and 3 will briefly review the operation of the TOAD switch and the SD number representation, respectively. Section 4 and 5 provide five adder designs: three for the TSD and two for the QSD adders. In section 6, we summarize the results and make some conclusions.
2. Operation of TOAD Based Optical Switch
In the last few years, the TOAD switch [19] was extensively used in realizing various ultrafast logical and arithmetic operations. Its speed is due to its capability of demultiplexing tera bits per second pulse trains where a light signal controls another light beam. In addition to its speed, the relatively low power consumption makes it attractive for large-optical integration. TOAD switch consists of a loop mirror with an intra loop 2 × 2 coupler and a semiconductor optical amplifier (SOA) which is offset from the loop’s midpoint by a distance Δx as shown in Figure 1(a). The SOA constitutes the nonlinear element within the switch where when exposed to a strong light it saturates and its index of refraction is changed. Full details of the operation of the TOAD switch is explained in [19] [22] . The TOAD switch operates with two lights signal through nonlinear interaction in a material based on interferometer setup: a strong optical signal, called the control pulse—CP, and another optical signal, called the incoming pulse—IP.
Briefly, the TOAD switch works as follows. When there is no CP signal, the IP signal enters the loop shown in Figure 1(a) and splits into two counter propagating ones. These two signals now recombine and interfere at the input coupler and emerge as a single light at the input port. However, if a strong CP light is injected into the loop causing the SOA to saturate and its index of refraction is changed. Consequently, the two IP counter propagating signals will face differential phase shift such that they will interfere and re-emerge or transmitted from the output port. At the two ports (reflected and transmitted), a polarization or wavelength filter is used to block the optical CP signal and only pass the optical IP signal. The energy of the optical CP must be sufficient to modify the optical property of the SOA element whereas the two counter propagating IP signals do not. Further, the IP and the CP signals are to be entered the SOA at about the same time. Further, in the absence of the CP signal, the input light exits from the lower port in the figure (no light is present in the upper port). However, when both CP and IP signals are present simultaneously, all light is directed towards the upper port because of the refractive-index change induced by the CP signal (no light is present in the lower port). When there is no IP

Figure 1. (a) TOAD-based optical switch; (b) a schematic diagram for the switch.
signal, both channels receive no light as the filter blocks the CP signal. A schematic block diagram for the TOAD switch is shown in Figure 1(b).
TOAD switches can successfully be used for designing logical circuits. For instance, cascaded TOAD switches as shown in Figure 2 are used to generate the sixteen minterms for four input logical variables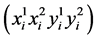 . This structure can be viewed as a 1 × 16 decoder where only one output is activated that corresponds to a specific minterm. Note that the output ports are numbered according to the corresponding generated minterms. ORing of these minterms (sum-of-products) is performed with flexible outputs interconnectivities (beam splitters/combiners) and ANDing operations are achieved by cascading stages (switches) where any output port signal can be used as an input to another switch. Note that in this case, the output optical signal of the first switch may need to be amplified and/or wavelength converted before it is fed to the next switch. As a result, this switch structure can be used as a preliminary non minimized basic building block to realize any logical Boolean function.
. This structure can be viewed as a 1 × 16 decoder where only one output is activated that corresponds to a specific minterm. Note that the output ports are numbered according to the corresponding generated minterms. ORing of these minterms (sum-of-products) is performed with flexible outputs interconnectivities (beam splitters/combiners) and ANDing operations are achieved by cascading stages (switches) where any output port signal can be used as an input to another switch. Note that in this case, the output optical signal of the first switch may need to be amplified and/or wavelength converted before it is fed to the next switch. As a result, this switch structure can be used as a preliminary non minimized basic building block to realize any logical Boolean function.
3. Signed-Digit Numbers
Signed-digit (SD) numbers are formally defined as follows [12] : given a radix r, each digit of SD number assumes (2α + 1) values of the digit set {−α,  , −1, 0, 1,
, −1, 0, 1,  , α} where α ≤ r − 1 and r ≥ 3. In general, a decimal number D may be represented in terms of an n-digits SD number as
, α} where α ≤ r − 1 and r ≥ 3. In general, a decimal number D may be represented in terms of an n-digits SD number as
 (1)
(1)
where bi digit is selected from the set {−α, 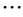 , −1, 0, 1,
, −1, 0, 1,  , α} to produce the appropriate decimal representation. For trinary signed-digit (TSD) r = 3, the digit set is {
, α} to produce the appropriate decimal representation. For trinary signed-digit (TSD) r = 3, the digit set is { ,
,  , 0, 1, 2}; whereas for quaternary signed-digit (QSD) r = 4 and the digit set is {
, 0, 1, 2}; whereas for quaternary signed-digit (QSD) r = 4 and the digit set is { ,
, 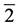 ,
,  , 0, 1, 2, 3}. Here the digits
, 0, 1, 2, 3}. Here the digits ,
,  , and
, and  denote −3, −2, and −1, respectively.
denote −3, −2, and −1, respectively.
For radix ≥ 3, the addition operation of two SD numbers is performed in two successive steps because some digit combinations cause carry propagation to the next higher-order digit. The generation of a carry may be avoided by mapping the two SD digits in question into an intermediate sum and an intermediate carry (also known as weight and transfer digits) such that the i-th intermediate sum and the (i−1)-th intermediate carry when added generate no carry. The two-step addition is governed by the following equations:


where 


Figure 2. 1 × 16 TOAD based decoder.
The totally-parallel adder for SD number representation is shown in Figure 3 where the functional blocks A and B represent the computing rules generated from Equations (2) and (3), respectively.
For TSD and QSD numbers, all possible two SD numbers that satisfy Equations (2) and (3) above are listed in Table 1 and Table 2, respectively. Note that the SD numbers in these tables are grouped according to the value of the output digit. The pairs of the to-be-added trinary digits are first divided into nine groups (for the first step) as shown in the second column of Table 1a whereas the third column of the table shows the necessary intermediate carry and intermediate sum digits for carry-free addition. Table 1(b) lists the rules for the second step of the addition. Thus, Table 1(a) and Table 1(b) represent the 25 and the 9 computational rules that are used in blocks A and B of Figure 3 for the TSD addition. Notice that these tables include polarization encoding of the SD digits that will be explained in the next section.
Similarly, the pairs of the to-be-added quaternary digits are arranged into thirteen groups for the first step and seven groups for the second step as shown in Table 2.
4. Polarization-Encoded TOAD-Based TSD Adder Circuits
It was mentioned in the previous section that the TOAD-based decoder method can be used to implement any logical function expressed as sum-of-products. The variables of the minterms are the control signals of the decoder. Now, since the TSD numbers {




Table 1. (a) Truth table and polarization-encoding for the first step TSD addition; (b) Truth table and polarization-encoding for the second TSD addition.


Table 2. (a) Truth table and polarization-encoding for the first step QSD addition; (b) Truth table and polarization-encoding for the second step QSD addition.
Figure 3. The two-step multiple-valued SD addition scheme.
Figure 4. Two-bit polarization-encoding of the multiple-valued SD digits.
4.1. TSD: DESIGN1
The TSD addition is a two-step process where the first step generates an intermediate transfer 








Figure 5. Designed circuits of the TSD adder: DESIGN1: (a) Step 1 V-channel circuit; (b) Step 1 H-channel; (c) Step 1 HV-channel circuit; and (d) Step 2 circuit.
representing the polarization state. Figure 5(d) represents the designed circuit for the second step (Table 1(b)) of the adder that generates the sum digits. In this figure, a simplified 1 × 4 decoder is used. This adder design requires only 36 switches and it takes seven gate delay units for the addition. Note that this is a non-minimized TSD adder design, which can be improved by reducing both the numbers of TOAD switches and the delay units. Further, polarization converters (PC) are used before the control signals and continuous light sources are needed for the circuit to work properly.
4.2. TSD DESIGN2
This subsection demonstrates a second design method for the TSD adder which is not based on the 1 × 16 TOAD decoder. When a closer look at the polarization-encoded TSD digits of Table 1(a) is carried out, it is observed that when the signals (





Figure 6. Designed circuits of the TSD adder: DESIGN2: (a) Step 1 V-channel circuit; (b) Step 1 H-channel; (c) Step 1 HV-channel circuit; and (d) Step 2 circuit.
On the other hand, the second step of the TSD adder is redesigned in order to eliminate the continuous H- and V-light sources in the circuits and at the same time maintain the same numbers of TOAD switches and the delay units as demonstrated in Figure 6(d). Furthermore, to clarify the generated outputs of each TOAD switch in the circuit, we posted the corresponding minterms at the output ports. This design method resulted in a better and a faster adder than DESIGN1. Thus, DESIGN2 requires only 24 gates and it takes only four gate delay units for the addition. Note that this design needs more beam combiners.
4.3. TSD DESIGN3
This proposed design method avoids using the 1 × 16 TOAD decoder, the polarization beam splitters that generate the H- and the V-channels, and the continuous H- and V-light sources. The TSD numbers {






5. Polarization-Encoded TOAD-Based QSD Adder Circuits
The quaternary literals {













Figure 7. Designed circuits of the TSD adder: DESIGN3: (a) Step 1 circuit; (b) Step 2 circuit.
5.1. QSD DESIGN1
This design uses the same approach as for DESIGN1 of the TSD adder where the 1 × 16 TOAD decoder constitutes the core part of the design. In this regard, polarization beam splitters are needed to generate the appropriate polarized CP. Table 2 lists the
5.2. QSD DESIGN2
It can be shown that by following a similar procedure to that of DESIGN2 of the TSD adder of the previous section, a better and reduced number of gate count for the QSD adder can be obtained. However, in this subsection we will provide a much better QSD adder, which follows the design method used in DESIGN3 of the TSD adder. Figure 9 presents this design where the nonzero outputs of the minterms (the entries in Table 2) are realized individually and they are shown at the output ports of the TOAD switches. To illustrate the working mechanisms of this design, let us consider generating the output for the minterms





6. Conclusions
In this paper, designs of ultra-fast all-optical TOAD-based adders for multiple-valued SD numbers are reported.




Figure 8. Designed circuits of the QSD adder: DESIGN1: (a) Step 1 V-channel circuit; (b) Step 1 H-channel circuit; (c) Step 1 HV-channel circuit; and (d) Step 2 circuit.


Figure 9. Designed circuits of the QSD adder: DESIGN2: (a) Step 1 circuit and (b) Step 2 circuit.
Table 3. Comparison between the multiple valued SD circuit designs.
These adders can be used as subtracters units once the augends are complemented and used as input digits to the designed adders. Three TSD (two QSD) circuits are designed and demonstrated. Table 3 summarizes the design elements of the proposed TSD and QSD adders as well as the most recently proposed MSD adder [24] . From this table, we can see that the QSD adder (DESIGN2) has the same speed (unit delay) as for the MSD adder while the gates count is higher as expected. Thus, this makes the proposed QSD adder an attractive circuit candidate knowing that the QSD number system (7 literals) provides more transmission information and more storage density than the MSD number system (3 literals).
On the other hand, the TSD adder (DESIGN3) is two times faster than both the QSD and the MSD counterparts. While the TSD gates count is almost the same as for the MSD adder and about 60% less than the QSD adder. Consequently, these results make the TSD adder very attractive and a good candidate for future considerations in digital optical computing.
It is worth mentioning that the previous proposed designs are theoretical. However, many theoretical simulations of similar TOAD-based circuits that prove their feasibilities were reported [25] - [28] . In addition, many reported works using TOAD switches demonstrated results with simulations using practical parameters or results from laboratories experimental data. Furthermore, note that when implementing multistage interconnection circuits using TOADs as the basic switch, one has to be aware of many practical issues such as synchronization and cascadability in addition to light intensity losses due to splitters/combiners, polarization, pulse duration, noise and others related issues [29] - [33] .
References
- Lakshmi, B. and Dhar, A.S. (2013) VLSI Architecture for Parallel Radix-4 CORDIC. Microprocessors and Microsystems, 37, 79-86. http://dx.doi.org/10.1016/j.micpro.2012.12.001
- Nikmehr, H., Phillips, B. and Lim, C.C. (2010) A Novel Implementation of Radix-4 Floating-Point Division/Square- Root Using Comparison Multiples. Computers & Electrical Engineering, 36, 850-863. http://dx.doi.org/10.1016/j.compeleceng.2008.04.013
- Ruiz, G.A. and Granda, M. (2011) Efficient Canonic Signed Digit Recoding. Microelectronics Journal, 42, 1090-1097. http://dx.doi.org/10.1016/j.mejo.2011.06.006
- Jaberipur, G. and Gorgin, S. (2010) An Improved Maximally Redundant Signed Digit Adder. Computers & Electrical Engineering, 36, 491-502. http://dx.doi.org/10.1016/j.compeleceng.2009.12.002
- Cherri, A.K. and Alam, M.S. (2000) Parallel Computation of Complex Elementary Functions Using Quaternary Signed-Digit Arithmetic. Optics & Laser Technology, 32, 391-399. http://dx.doi.org/10.1016/S0030-3992(00)00103-1
- Alam, M.S. (1994) Parallel Optical Computing Using Recoded Trinary Signed-Digit Numbers. Applied Optics, 33, 4392-4397. http://dx.doi.org/10.1364/AO.33.004392
- Chattopadhyay, T. (2010) All-Optical Quaternary Circuits Using Quaternary T-Gate. Optik, 121, 1784-1788. http://dx.doi.org/10.1364/AO.33.004392
- Chattopadhyay, T. (2010) All-Optical Symmetric Ternary Logic Gate. Optics & Laser Technology, 42, 1014-1021. http://dx.doi.org/10.1016/j.optlastec.2010.01.023
- Cherri, A.K. and Al-Zayed, A. (2010) Circuits Designs of Ultra-Fast All-Optical Modified Signed-Digit Adders Using Semiconductor Optical Amplifier and Mach-Zehnder Interferometer. Optik, 121, 1577-1585. http://dx.doi.org/10.1016/j.ijleo.2009.02.029
- Ghosh, A.K., Bhattacharya, A., Raul, M. and Basuray, A. (2012) Trinary Arithmetic and Logic Unit (TALU) Using Savart Plate and Spatial Light Modulator (SLM) Suitable for Optical Computation in Multivalued Logic. Optics & Laser Technology, 44, 1583-1592. http://dx.doi.org/10.1016/j.optlastec.2011.11.044
- Avizienis, A. (1961) Signed-Digit Number Representations for Fast Parallel Arithmetic. IRE Trans Electronic Computers, EC-10, 89-400.
- Hwang, K. (1979) Computer Arithmetic Principles: Architecture and Design. WILEY Publishing Co., New York.
- Cherri, A.K. and Khachab, N.I. (1996) Canonical Quaternary Signed-Digit Arithmetic Using Optoelectronics Symbolic Substitution. Optics & Laser Technology, 28, 397-403. http://dx.doi.org/10.1016/0030-3992(95)00109-3
- Awwal, A.A.S. (1993) Recoded Signed-Digit Binary Addition-Subtraction Using Optoelectronic Symbolic Substitution. Applied Optics, 31, 3205-3208. http://dx.doi.org/10.1364/AO.31.003205
- Ha, B. and Li, Y. (1994) Parallel Modified Signed-Digit Arithmetic Using an Optoelectronic Shared Content-Ad- dressable-Memory Processor. Applied Optics, 33, 3647-3662. http://dx.doi.org/10.1364/AO.33.003647
- Huang, H.X., Itoh, M., Yatagai, T. and Liu, L.R. (1996) Classified One-Step Modified Signed-Digit Arithmetic and Its Optical Implementation. Optics Engineering, 35, 1134-1140. http://dx.doi.org/10.1117/1.600602
- Qian, F., Li, G., Ruan, H. and Liu, L. (1999) Modified Signed-Digit Addition by Using Binary Logic Operations and Its Optoelectronic Implementation. Optics & Laser Technology, 31, 403-410. http://dx.doi.org/10.1016/S0030-3992(99)00078-X
- Nishimura, N., Awatsuji, Y. and Kubota, T. (2004) Two-Dimensional Arrangement of Spatial Patterns Representing Numerical Data in Input Images for Effective Use of Hardware Resources in Digital Optical Computing System Based on Optical Array Logic. Journal of Parallel and Distributed Computing, 64, 1027-1040. http://dx.doi.org/10.1016/j.jpdc.2004.06.011
- Sokoloff, J.P., Prucnal, P.R., Glesk, I. and Kane, M. (1993) A Terahertz Optical Asymmetric Demultiplexer (TOAD). IEEE Photonics Technology Letters, 5, 787-789. http://dx.doi.org/10.1109/68.229807
- Wang, B.C., Baby, V., Tong, W., Xu, L., Friedman, M., Runser, R.J., Glesk, I. and Pruncnal, P.R. (2002) A Novel Fast Optical Switch Based on Two Cascaded Terahertz Asymmetric Demultiplexers (TOAD). Optics Express, 10, 15-23. http://dx.doi.org/10.1364/OE.10.000015
- Gayen, D. and Roy, J.N. (2008) All-Optical Arithmetic Unit with the Help of Terahertz-Optical-Asymmetric-Demulti- plexer-Based Tree Architecture. Applied Optics, 47, 933-943. http://dx.doi.org/10.1364/AO.47.000933
- Roy, J.N. and Gayen, D.K. (2007) Integrated All-Optical Logic and Arithmetic Operations with the Help of a TOAD Based Interferometer Device-Alternative Approach. Applied Optics, 46, 5304-5310. http://dx.doi.org/10.1364/AO.46.005304
- Cherri, A.K. (2010) Terahertz-Optical-Asymmetric-Demultiplexer (TOAD)-Based Arithmetic Units for Ultra-Fast Op- tical Information Processing. SPIE Proceedings, 7671.
- Alqallaf, A.K., Hajjiah, A.T. and Cherri, A.K. (2013) Ultra-Fast All-Optical Polarization-Encoded Modified Signed- Digit Addition Using Terahertz-Optical-Asymmetric-Demultiplexer (TOAD) Switches. Optik, 124, 4887-4891. http://dx.doi.org/10.1016/j.ijleo.2013.02.028
- Minh, H.L., Ghassemlooy, Z. and Ng, W.P. (2008) Characterization and Performance Analysis of a TOAD Switch Employing a Dual Control Pulse Scheme in High-Speed OTDM Demultiplexer. IEEE Communications Letters, 12, 316-318. http://dx.doi.org/10.1109/LCOMM.2008.061299
- Zoiros, K.E., Das, M.K., Gayen, D.K., Maity, H.K., Chattopadhyay, T. and Roy, J.N. (2011) All-Optical Pseudoran- dom Binary Sequence Generator with Toad-Based D Flip-Flops. Optics Communications, 284, 4297-4306. http://dx.doi.org/10.1016/j.optcom.2011.05.007
- Gayen, D.K., Roy, J.N. and Pal, R.K. (2012) All-Optical Carry Lookahead Adder with the Help of Terahertz-Optical- Asymmetric-Demultiplexer. Optik, 123, 40-45. http://dx.doi.org/10.1016/j.ijleo.2010.11.029
- Chattopadhyay, T. (2012) Terahertz Optical Asymmetric Demultiplexer (TOAD) Based Half-Adder and Using It to Design All-Optical Flip-Flop. Optik, 123, 1961-1964. http://dx.doi.org/10.1016/j.ijleo.2011.11.012
- Wang, B.C., Baby, V., Tong, W., Xu, L., Friedman, M., Runser, R.J., Glesk, I. and Prucnal, P.R. (2002) A Novel Fast Optical Switch Based on Two Cascaded Terahertz Optical Asymmetric Demultiplexers (TOAD). Optics Express, 10, 15-23. http://dx.doi.org/10.1364/OE.10.000015
- Minh, H.L., Ghassemlooy, Z., Ng, W.P. and Ngah, R. (2004) Terahertz Optical Asymmetric Demultiplexer Switch with a Symmetrical Switching Window. Proceedings of the London Communication Symposium, University College, London, 89-92.
- Barman, A.D., Fresi, F., Sengupta, I.I., Potì, L. and Bogoni, A. (2009) Theoretical and Experimental Investigation of Inter-Channel Crosstalk Mitigation by Assist Light in a TOAD De-Multiplexer. Proceedings of the International Con- ference on Computers and Devices for Communication, (IEEE CODEC’09), Kolkata, 14-16 December 2009, 1-4.
- Lu, D., Jia, N., Zhong, K.P., Chen, M., Li, T.J. and Jian, S.S. (2009) Experimental and Theory Study the System Per- formance of TOAD Using for Demultiplexing in 160gb/S OTDM Transmission System. Proceedings of the Commu- nications and Photonics Conference and Exhibition (ACP), Asia, Shanghai, 2-6 November 2009, 1-7.
- Zoiros, K.E., Kalaitzi, A. and Koukourlis, C.S. (2010) Study on the Cascadability of a SOA-Assisted Sagnac Switch Pair. Optik, 121, 1180-1193. http://dx.doi.org/10.1016/j.ijleo.2009.01.001
NOTES
*Corresponding author.























