Journal of Crystallization Process and Technology
Vol.06 No.04(2016), Article ID:71477,27 pages
10.4236/jcpt.2016.64004
Crystal Growth and Nucleation in Glasses in the Lithium Silicate System
Galina A. Sycheva
Grebenshchikov Institute of Silicate Chemistry, Russian Academy of Sciences, St. Petersburg, Russia

Copyright © 2016 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: May 26, 2016; Accepted: October 22, 2016; Published: October 25, 2016
ABSTRACT
The crystal growth and nucleation in glasses in the lithium silicate system have been investigated. Phase separation in ultimately homogenized glasses of the lithium silicate system xLi2O×(100 − x)SiO2 (where x = 23.4, 26.0, 29.1, and 33.5 mol% Li2O) has been studied. The glasses of these compositions have been homogenized using the previously established special temperature-time conditions, which make it possible to provide a maximum dehydration and removal of bubbles from the glass melt. The parameters of nucleation and growth of phase separated in homogeneities and homogeneous crystal nucleation have been determined. The absolute values of the stationary nucleation rates Ist of lithium disilicate crystals in the 23.4Li2O×76.6SiO2, 26Li2O×74SiO2 and 29.1Li2O×70.9SiO2 glasses with the compositions lying in the metastable phase separation region have been compared with the corresponding rates Ist for the glass of the stoichiometric lithium disilicate composition 33.51Li2O×66.5SiO2. It has been found that the crystal growth rate has a tendency toward a monotonic increase with an increase in the temperature, whereas the dependences of the crystal growth rate on the time of low temperature heat treatment exhibit an oscillatory behavior with a monotonic decrease in the absolute value of oscillations. The character of crystallization in glasses with the compositions lying in the phase separation region of the Li2O-SiO2 system is compared with that in the glass of the stoichiometric lithium disilicate composition. The conclusion has been made that the phase separation weakly affects the nucleation parameters of the lithium disilicate and has a strong effect on the crystal growth.
Keywords:
Phase Separation, Crystal Nucleation, Stationary Nucleation, Crystal Growth in Phase Separated and Non-Phase Separated Glasses

1. Introduction
The glass forming systems may be regarded as model systems for technical glass ceramics. Many papers on investigation of nucleation in oxide glasses are known. Table 1 shows the compound glass-forming systems, for which the temperature dependence of the steady and unsteady crystal nucleation in the bulk nucleation were studied.
As can be seen from Table 1, the greatest attention was paid by researchers to the system Li2O-SiO2 [1] - [68] because of its practical importance. A specific feature of the system Li2O-SiO2 is that, in the region of compositions from 0 to 32 mol% Li2O, the glasses have a tendency towards the phase separation. Many authors [91] - [94] have believed that the process of phase separation of glasses into two vitreous phases favors a homogeneous crystallization of a glass with the formation of small crystals. As follows from [95] [96] , the role of the phase separation is reduced to the appearance of phase boundaries that serve as a particular catalyst of the crystallization. According to [93] ,
Table 1. Compound of glass-forming systems, for which the temperature dependences of the steady and unsteady crystal nucleation in the bulk nucleation were studied.
the crystallization of phase separated glasses cannot be referred to as catalytic. It is necessary to speak about the influence of the phase separation on a further crystallization process rather than about catalysis. Actually, a review of a number of experimental works [81] [97] - [101] demonstrates that the liquid-liquid phase separation before crystallization affects the crystal structure of glass-ceramic materials through at least three different ways.
1) The boundary between phases plays a role of an initiating agent.
2) Since, after the phase separation, the composition of a matrix or dispersed phase becomes closer to that of a crystallizing phase, the formation and growth of crystals progress more easily.
3) In one of the phases, the metastable phase precipitates and plays a role of a catalyst for the main crystalline phase.
The purpose of this work is to investigate the crystal growth and nucleation in glasses in the lithium silicate and sodium silicate systems that serve as a basis for the preparation of glass ceramic materials.
2. Sample Preparation and Experimental Technique
The rates of nucleation and growth of crystals and phase separated in homogeneities were studied in glasses of the analyzed compositions xLi2O×(100 − x)SiO2 (where x = 23.4, 26.0, 29.1 and 33.5 mol% Li2O). Since it was established earlier that the crystal nucleation parameters are strongly affected by water [35] and bubbles formed in the course of glass synthesis [102] , the glasses were homogenized to a maximum extent under specially established temperature-time conditions of synthesis. As was previously shown, bubbles can play a role of initiators of nucleation of lithium disilicate crystals and increase a number of crystals to 20% [103] . As the water content increases, the stationary nucleation rate increases and the position of the maximum shifts toward lower temperatures [35] . In this respect, all cares were taken in order to prepare glasses homogenized to a maximum extent. Water was removed by bubbling glass melts with argon for 20 min [49] . Bubbles were removed by performing the synthesis at a high temperature. The glasses were synthesized according to the technique described in [103] . A batch was prepared from lithium carbonate (analytical grade) and dehydrated silicon dioxide (analytical grade) by mixing in a drum. The glasses were synthesized in a platinum crucible (volume, 200 ml) in a Globar-heater furnace with silicon carbide heaters (synthesis temperature, 1550˚C; synthesis time, 5 h). Heat treatments were performed in SShOL electric shaft furnaces and a gradient furnace designed at a laboratory. The temperature was maintained accurate to within ±1˚C. The results of the chemical analysis of the synthesized glasses are presented in Table 2.
X-ray powder diffraction analysis was performed on a DRON-2 diffractometer (CuKα radiation; operating voltage 30 kV; current 20 mA; detector rotation rate, 2 deg/min). Differential thermal analysis (DTA) was carried out on a MOM derivatograph (heating rate, 10 K/min; sample weight, 1 g ; galvanometer sensitivity, 1/5; reference sample, Al2O3; platinum crucible). Optical microscopy investigations in transmitted and reflected light
Table 2. Chemical compositions under investigation (mol%).
were performed on Carl Zeiss Jenaval and Neophot 32 microscopes (
3. Temperature Dependence of the Nucleation and Growth Rate of Phase Separated Inhomogeneities in Lithium Silicate Glasses of the Compositions 23.4Li2O×76.6SiO2 (1), 26Li2O×74SiO2 (2), and 29.1Li2O×70.9SiO2 (3)
In the 33.5Li2O×66.5SiO2 glass with the composition closest to the stoichiometric composition of the lithium disilicate, excess SiO2 is absent and, therefore, no phase separation is observed. The compositions of the glasses containing 23.4 mol% Li2O (no. 1), 26Li2O (no. 2), and 29.1Li2O (no. 3) lie in the metastable phase separation region of the lithium-silicate system. The samples of glasses no. 1, no. 2, no. 3 and no. 4 were subjected to preliminary heat treatment at temperatures in the range 370˚C - 560˚C for different times. Then, they were held at a development temperature of 600˚C for 10 min. If glass no. 1, no. 2 or 3 is held at the temperature T = 600˚C for a time in the range 0 - 10 h, it remains transparent without visible opalescence in visual examination. If the glass is heat treated for t = 2 h 40 min at temperatures of 400˚C - 560˚C, it remains visually transparent and does not become opalescent. After additional heat treatment at 600˚C for 10 min, the glass acquires bright blue (yellow in transmission) going to milky opalescence. The electron microscopic images of the glasses preliminarily heat treated at temperatures in the range 400˚C - 560˚C and developed at 600˚C for 10 min are displayed in Figures 1(a)-1(c).
We counted the number of traces of particles per unit area in the electron microscopic image NS/S0 and determined the sizes of the maximum traces of particles R. The corresponding results for glass no. 1 are listed in Table 3.



Figure 1. Electron microscopic images of glasses (a) no. 1, (b) no. 2 and (c) no. 3 preliminarily heat treated at temperature 460˚C for 2 h 40 min and developed at 600˚C for 10 min (magnification, 28,800).
Table 3. Numbers of traces of particles per unit area in electron microscopic images NS/S0 and their sizes in glass no. 3 after heat treatment at T for 160 min and development at 600˚C for 10 min.
Figure 2 presents the quantitative characteristics of the phase separation structure in glasses no. 1 and no. 2 as a function of the temperature, namely, the ratio of the number of traces to the maximum number of traces NS/NSmax and the ratio of the radius of the particle trace to the maximum radius R/Rmax.



Figure 2. Quantitative characteristics of the phase separation structure in glasses (a) no. 1, (b) no. 2 and (c) no. 3 as a function of the temperature: (1) ratio of the number of traces to the maximum number of traces NS/NSmax and (2) ratio of the radius of the particle trace to the maximum radius R/Rmax.
Here, we assume that the dependence of the number of traces of phase separated droplets per unit area of the sample surface on the heat treatment temperature (at a constant heat treatment time) obeys the same function law as the temperature dependences of the stationary nucleation rate of droplets. Strictly speaking, in order to determine the stationary nucleation rate I(T), initially, it is necessary to determine the number of droplets per unit volume of the glass by the number of traces per unit area with the use of stereological formulas. Then, it is required to determine a variation in the number of droplets per unit volume as a function of the heat treatment time. Thereafter, it is necessary to check that the nucleation rate is stationary and to determine its value. This is not a simple problem, which is complicated by the fact that the droplets in the volume of the glass can have different sizes. The obtained dependence of the number of traces on the temperature for a fixed heat treatment time can differ substantially from the dependence of the stationary nucleation rate of droplets. However, we used a simpler method to approximately evaluate the temperature dependence of the nucleation rate of droplets. Instead of the dependence I(T), we constructed the dependence NS(T) of the number of traces of particles developed for the same specified time of development tdev at Tdev after preliminary heat treatment for the same specified time t at all temperatures T. Since the quantities I and NS are approximately proportional to each other, the curves I(T)/Imax and NS(T)/NSmax are very close to each other and, in order to evaluate the temperature of the maximum nucleation rate, it is sufficient to measure the dependence of the number traces of crystals NS without regarding for their shape and sizes [77] [80] . It can be seen from Figure 2 that these dependences exhibit maxima. The maximum values of the number of traces and sizes correspond to temperatures of 460 and 600˚C, respectively. On the whole, the results obtained can be explained as follows. The nucleation rate of a new phase (phase separated or crystalline) I has the form [68]
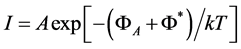 , (1)
, (1)
where Φ* is the increment of the thermodynamic potential of the glass due to the appearance of a critical (in composition and size) inhomogeneity nucleus, ΦA is the thermodynamic potential of diffusion jumps and transformations during the phase separation, and A is the factor that, like the quantities ΦA and Φ*, depends on the glass composition and temperature more weakly than an exponential function. If the glass composition c0 belongs to the bimodal region at a given temperature, the thermodynamic potential Φ* is relatively high because the inhomogeneities that do not reach some limiting concentration c1 appear to be energetically unfavorable irrespectively of their sizes. When the glass composition belongs to the spinodal region, inhomogeneities with an arbitrarily small difference  and, correspondingly, with negligible Φ* can be energetically favorable if only their size exceeds the critical size. As a consequence, there is an increase in the nucleation rate of inhomogeneities I (expression (1)). By using the notion of the concentration wave length λ introduced by Cahn, in the spinodal region, the value
and, correspondingly, with negligible Φ* can be energetically favorable if only their size exceeds the critical size. As a consequence, there is an increase in the nucleation rate of inhomogeneities I (expression (1)). By using the notion of the concentration wave length λ introduced by Cahn, in the spinodal region, the value 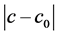 most rapidly increases in amplitude and, hence, the inhomogeneities with the size λm that is larger than the critical size λcr by a factor of
most rapidly increases in amplitude and, hence, the inhomogeneities with the size λm that is larger than the critical size λcr by a factor of  nucleate most rapidly. The wave amplitude λm increases as exp(t/τm); in this case, the magnitude 1/τm can be considered not only as the measure of the nucleation rate of the amplitude but also as the measure of the nucleation rate of inhomogeneities by taking I = B/τm. As a result, we have (2)
nucleate most rapidly. The wave amplitude λm increases as exp(t/τm); in this case, the magnitude 1/τm can be considered not only as the measure of the nucleation rate of the amplitude but also as the measure of the nucleation rate of inhomogeneities by taking I = B/τm. As a result, we have (2)
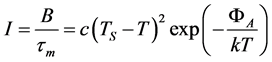 , (2)
, (2)
where B and c are temperature independent constants and TS is the spinodal temperature. From the condition dI/dT = 0, we find that the maximum of the rate is located at the temperature Tmax satisfying the Equation (3).
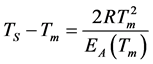 ,
,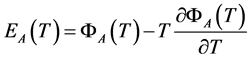 (3)
(3)
By assuming that the thermodynamic potential ΦA does not depend on the temperature T, i.e., ΦA = EA, and setting ΦA = 83 kcal/mol (activation energy of oxygen diffusion), at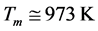 , Equation (3) takes the form
, Equation (3) takes the form
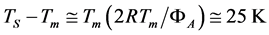 (4)
(4)
Under the assumption that the thermodynamic potential ΦA is equal to the activation energy of lithium self diffusion, we obtain

Therefore, the maximum of the ratio NS/NSmax in Figure 2 reflects the maximum of the nucleation rate of droplets with a diameter λ = 200 - 400 Å. This reflection is rather
indirect because the number of inhomogeneities n' nucleated for the time t'
can differ significantly (and differs) from the number n of large particles growing in the course of the development. The reason is that, after the increase in the temperature to 600˚C, the inhomogeneities with the composition and size that reach critical values at 600˚C are predominantly retained and will develop. The inhomogeneities with the composition that approaches the binodal composition at the corresponding temperature during low temperature heat treatment go beyond the binodal with the increase in temperature to 600˚C and should dissolve in the glass located between inhomogeneities. A number of inhomogeneities can merge together when reaching the critical sizes for a temperature of 600˚C. This dissolution and merging of small inhomogeneities can lead to the formation of larger and stable inhomogeneities with a composition close to the binodal composition at the temperature of 600˚C.
Although the relation between the quantities n and n' is complex, the maximum of the ratio NS/NSmax in Figure 2 reflects the rate of nucleation and development of phase separated inhomogeneities. Then, the temperature of its left boundary 

Haller et al. [105] calculated the spinodal for glasses in the lithium silicate system. A comparison of our data with those obtained in [105] leads to the following results. According to [105] , the spinodal temperature TS amounts to approximately 600˚C for glass no. 1 (23.4 mol% Li2O) and is in the vicinity of 500˚C for glass no. 2 (26.0 mol% Li2O). Below 500˚C, data in [105] are absent. However, if the curve is extrapolated to 400˚C, the spinodal temperature TS for glass no. 2 appears to be 400˚C. In our work, the maximum of the ratio NS/NSmax in Figure 2 lies in the temperature range 350˚C - 570˚C for glass no. 1 and ≈ 400˚C - 650˚C for glass no. 2. Therefore, the experimental and calculated data for glass no. 2 (26.0 mol% Li2O) are in good agreement, whereas, for glass no. 1 (23.4 mol% Li2O), the calculated value is by 200˚C higher than the experimental. For glasses no. 1 and no. 2, the difference 
In order to compare more rigorously the theory with the experiment, it is necessary to know the spinodal temperature TS. This temperature was evaluated as follows. Quenched glasses no. 1 and no. 2 (without low temperature heat treatments) were held in the gradient furnace at temperatures in the range 300˚C - 800˚C for 2 h. This led to the appearance of the opalescent band along the sample length, which was stable with respect to a further increase in the holding time. This band had a higher intensity in the central part and weaker intensities toward higher and lower temperatures up to its complete visual disappearance. The temperature of the upper edge of the visible opalescence (770˚C for glass no. 1) coincided with the phase separation temperature Tl (770˚C for glass no. 1) determined as the temperature of the disappearance of the visible opalescence after the opalescent part of the rod was displaced in the range of temperatures a priori higher than Tl. The analysis of the electron microscopic images demonstrates that, in going from the high temperature edge of the band to the low temperature edge, the number of particle traces per unit area (or the relative area occupied by particle traces) increases linearly. Initially, this process goes slowly and then accelerates. It is clear because the nucleation rate is minimum in the vicinity of the phase separation temperature Tl and increases as the spinodal temperature TS is approached as a result of the decrease in the thermodynamic potential Φ* in expression (1). When the traces of inhomogeneities begin to cover a larger part of the replica area and to merge together, the increase in the relative area S/S0 occupied by particles at 
3.1. Effect of Displacement of the Glass Composition from the Stoichiometric Lithium Disilicate Composition toward an Increase in the SiO2 Content on the Nucleation of Lithium Disilicate Crystals
In order to accelerate the search for the temperature range of crystal nucleation, we used the DTA curves and the temperature dependences of the viscosity, because it was previously shown [68] that the maximum of the crystal nucleation rate is located in the range of the endothermic effect in the DTA curve at the temperature corresponding to a viscosity of 1013 P. The temperature dependences of the viscosity for glasses no. 1 - no. 3 are plotted in Figures 3(a)-3(c). The inset to Figure 3 presents the coefficients A, B, and T0 in the approximation according to the Vogel-Fulcher-Tammann equation [104] . It can be seen from Figure 3 that the glass transition temperature determined as the temperature corresponding to a viscosity of 1013 P is equal to 453˚C for glass no. 1,


Figure 3. Temperature dependences of the decimal logarithm of the viscosity for glasses (a) no. 1, (b) no. 2, and (c) no. 3 and (d) temperature dependence of the natural logarithm of the viscosity for glass no. 2 (used for determining the activation energy of viscous flow in the glass).
458˚C for glass no. 2, 457˚C for glass no. 3 and 450˚C for glass no. 4. The slope of the temperature dependence of the natural logarithm of the viscosity on the reciprocal of the temperature (Figure 3(d)) was used to determine the activation energies of the viscous flow Eη = Rdlnη/d(1/T), which were equal to 148, 149, 150 and 152 kcal/mol for glasses no. 1 - no. 4, respectively.
The X-ray powder diffraction data (Figure 4) demonstrate that the initial glasses are X-ray amorphous. The lithium disilicate crystals nucleated at temperatures of preliminary heat treatments in the range 420˚C - 520˚C for all times cannot be revealed using X-ray diffraction analysis. This is associated with the fact that the lithium disilicate nuclei are extremely small and their total weight is insignificant and, hence, insufficient for identifying by X-ray diffraction analysis, because its sensitivity is equal to a few weight percent of a compound. After heat treatments, lithium disilicate Li2O 2SiO2 (PCPDFWIN no. 80 - 1470; orthorhombic structure; unit cell parameters a = 5.687 Å, b = 4.784 Å, and c = 14.640 Å) crystallizes in glasses no. 1 - no. 3 at a development temperature of 600˚C for 10 min.
It can be seen from the images obtained using the optical microscope in reflected and transmitted light (Figure 5) that the lithium disilicate grows in the form of ellipsoids of revolution with an axial ratio of 1.0:1.6 in glass no. 4 (with the composition closest to the stoichiometric lithium disilicate composition), with an axial ratio of 1.0:2.0 in glass

Figure 4. X-ray diffraction patterns of initial and heat treated glasses no. 2: (a) initial glass, (b) glass heat treated at 450˚C for 5 h, and (c) glass preliminarily heat treated at 450˚C for 5 h and developed at 600˚C for 10 min.

Figure 5. Shape of lithium disilicate crystals in: (a) glass no. 4 after preliminary heat treatment at the temperature 420˚C for 4 h; (b) glass no. 2 after preliminary heat treatment at the temperature 400˚C for 4 h; (с) glass no. 3 after heat treatment at the temperature 440˚C for 8 h. All samples were developed at the temperature 600˚C for 10 min. Image (a) was obtained in reflected light with the use of the Neophot 32 optical microscope at a magnification of 150. Image (b) was obtained in transmitted light with the use of the Jenaval optical microscope at a magnification of 200. Image (c) was obtained in reflected light with the use of an EM-125 transmission electron microscope at a magnification of 28000.
no. 3 and in the shape of regular spheres in glass no. 2. The number of crystals increases with an increase in the holding time during preliminary heat treatment.
In order to nucleate lithium disilicate crystals, the samples of initial glasses no. 1 - no. 4 were held at temperatures in the range 420˚C - 520˚C (range of the endothermic effect in the DTA curves). Then, the nucleated crystals were developed (to sizes visible in the optical microscope) at 600˚C for 10 min, i.e., at the temperature corresponding to the ascending branch of the exothermic peak in the DTA curve. The kinetics of homogeneous crystal nucleation was described using the stationary nucleation rate Ist, nonstationary nucleation time τ, and temperature dependences of these quantities.
3.2. Determination of the Crystal Nucleation Rate
The stationary nucleation rate Ist was determined from the experimental dependences of the number of crystals n(t) as the slope of the stationary portion of these dependences on the holding time of glasses at each holding temperature. In this work, the rate of stationary homogeneous crystal nucleation was determined by the cross section method (in reflected light) and by direct counting of the number of crystals (in transmitted light). The glass samples were subjected to preliminary heat treatment and rapidly cooled in air to room temperature. Then, the samples were repeatedly heat treated at the development temperature of 600˚C for 10 min. With the aim of examining the samples in reflected light, the surface layer was ground off and the prepared surface was polished. In order to increase the contrast of the boundaries between crystals and glass, the sample surface was etched in a 0.01 M HF solution for 10 s. The number and size of cross sections of crystals per unit surface area were determined from the micrographs with the use of the Neophot optical microscope or directly in the field of vision of the Jenaval microscope.
The number of crystals per unit volume n and the number of cross sections of crystals in the micro section NS are related by the expression n = NS/(SD), where S is the micro section area and D is the diameter of a maximum particle in the micro section. The nonstationary nucleation time τ was evaluated using the induction period tind, which differs from the time τ by the temperature independent factor.
The induction period tind was determined as the intersection point between the continuation of the linear portion of the dependence n(t) and the time axis. Then, we constructed the temperature dependences of all the aforementioned quantities.
4. Experimental Results on Crystal Nucleation and Their Discussion
The nucleation of the lithium disilicate was observed in all glasses. The dependences of the number of developed crystals n in the nucleation temperature range 400˚C - 520˚C (development at 600˚C for 10 min) were used to determine the stationary nucleation rate and the nonstationary nucleation time at each specific temperature. The induction period tind decreases monotonically with an increase in the temperature. The temperature dependence of the induction period tind(T) allows us to determine the activation energies for nucleation of lithium disilicate crystals Eτ from the formula Eτ = Rdlntind/d(1/T). The corresponding activation energies are equal to 129, 128, 127 and 126 kcal/mol for glasses no. 1 - no. 4, respectively. The temperature dependences of the stationary nucleation rate of lithium disilicate crystals Ist(T) for the glass with the stoichiometric lithium disilicate composition and glasses with displaced compositions are shown in Figure 6. It can be seen from this figure that the nucleation rate of lithium disilicate crystals Ist(Tmax) at the maximum decreases from 160 to 60 mm−3∙min−1; in this case, the position of the maximum Tmax is shifted toward higher temperatures by 12˚C. This relatively small change in the quantity Ist(Tmax) can be explained by the fact that the glasses with displaced compositions correspond to the phase separation region in the Li2O-SiO2 system, so that the nucleation of the lithium disilicate occurs in phase separated inhomogeneities with the composition that is displaced toward the lithium disilicate composition and depends weakly on the temperature. The shift in the maximum Tmax by 12˚C can be explained by the increases in the viscosity and the glass transition temperature of the melt in the phase separated (matrix) region [106] [107] enriched by SiO2 as compared to the lithium disilicate composition, which provides an increase in the temperature Tmax according to the relationship for the maximum of the nucleation rate



where Tmelt is the melting temperature and the enthalpy HA, activation entropy SA, and the nucleation barrier correspond to T = Tmax. It can be seen from Figure 6 that the temperature Tmax of the maximum nucleation rate of lithium disilicate crystals for all the compositions under investigation is close to the glass transition temperature Tg. The closeness of the temperatures Tmax and Tg for the glass of the stoichiometric compositions and the glasses with displaced compositions can be explained as follows. The
Figure 6. Temperature dependences of the stationary nucleation rate of lithium disilicate crystals Ist(T) for the glasses (1) no. 1, (2) no. 2 and (3) no. 3 with displaced compositions of the glasses and (4) with the stoichiometric lithium disilicate composition.
relative displacements of structural units that are necessary for crystal nucleation and occur through the breaking and switching of chemical bonds become sufficiently fast with an increase in the temperature beginning from the glass transition point Tg. The presence of the viscosity determined by the free activation energy Φη = ΦA leads to a shift in the maximum of the stationary nucleation rate Ist(T) according to relationship (6) toward higher temperatures (Tmax = Tg = 2/3Tmelt) as compared to its position Tmax = 1/3Tmelt that would be observed at H = 0.
5. Crystal Growth Rate
Before proceeding to the discussion of the results of the experiment on the determination of the growth rate of lithium disilicate crystals in glasses no. 1 - no. 3, let us consider the main notions used when determining the crystal growth. The crystal growth occurs as a result of accidental attachments and detachments of structural units from the surface of a supercritical nucleus (the supercritical nucleus is a nucleus with the size exceeding a critical size). The growth of the super-critical nucleus is thermodynamically favorable because this is accompanied by a decrease in the free energy of the system ΔΦ. The linear growth rate of the nucleus is determined by the equation

where l is the thickness of the monomolecular layer growing on the crystal for some time interval, equal to the linear size of the structural unit), and β+ and β− are the probabilities of attachment and detachment of one structural unit from the crystal surface per unit time:


Here, τ0 is the time of the order of the period of vibrations of atoms in a chemical bond, whose switching provides the transition of the structural unit to the ordered state of the crystal structure of the nucleus; ΔΦ is the change in the free energy of the system; and a is the linear size of the structural unit. Substitution of these expressions into formula (7) gives

where 


Now, we consider the temperature dependence of the stationary growth rate. Under the assumption that

According to expression (10), the growth rate is equal to zero at T → 0 and T → Tmelt. At some temperature (


From the extremum condition

The quantity kTmelt/HA in formula (12) usually does not exceed 1/10. Therefore, the temperature of the maximum growth rate is close to the melting temperature, and it is very difficult to experimentally determine this temperature. This inference is very important for experimenters.
6. Experimental Results on the Crystal Growth and Their Discussion
6.1. Crystal Growth Rate: Dependence on the Temperature
There is a large number of works on the rate of crystal nucleation (see Table 1) in lithium silicate glasses, whereas investigations of the concentration dependences of the growth rate are absent. There exist works in which the results are presented only for specific compositions of glasses in this system [99] [108] . The photographs of characteristic lithium disilicate crystals grown at a development temperature of 600˚C in glasses no. 1 - no. 3 are displayed in Figure 5. In order to determine the growth rate U, the dependences Rmax(t) were constructed for a series of samples held for different times t at a specified temperature. It was revealed that these dependences exhibit a linear behavior, which allows us to determine the crystal growth rate from their slope. In the case of spherical particles, their growth rate U is determined from the expression U = dRmax/dt', where t' is the time of holding of the glass at the development temperature. In the case of ellipsoids of revolution, the growth rate U is determined from the change in their average radius defined as the half sum of the major and minor radii of the ellipsoid. The crystal growth rates were studied at temperatures of 570˚C, 590˚C, 600˚C, 640˚C, 685˚C, 718˚C, 720˚C, and 730˚C. The growth rates U(T) of lithium disilicate crystals in glasses no. 1 - no. 4 as a function of the temperature are given in Table 4.
Figure 7 shows the temperature dependences of the growth rate U for glasses no. 1 - no. 3. The data presented in Figure 7(c) allow us to compare the growth rates U determined in our work with those obtained in [109] .
As can be seen from Figure 7(c), Burgner et al. [109] performed investigations at higher temperatures. We more thoroughly studied the growth rate at low temperatures. In the temperature range 575˚C - 600˚C, the results of both studies are in good agreement. It can be seen from Figure 7 that the growth rate U(T) increases with an increase in the temperature. In the temperature range 640˚C - 718˚C, the growth rate increases linearly. It is difficult to judge the accurate position of the maximum of the growth rate U(T), because the error in the measurement of the size at temperatures above 720˚C
Table 4. Growth rates U(T) (μm/min) of lithium disilicate crystals in glasses no. 1 - no. 4 as a function of the temperature.


Figure 7. Temperature dependences of the growth rate U for glasses (a) no. 1, (b) no. 2, (с) no. 3, and (d) no. 4 according to the data (1) obtained in this work and (2) taken from [109] .
can increase in an uncontrollable manner due to the fact that the time of heat treatment of the sample (1 - 2 min) is comparable to the time of its heating to a specified development temperature and we cannot state with assurance that crystals grew accurately at this temperature. Therefore, although the temperature dependence of the growth rate should have a maximum, we cannot argue that its true position is recorded. It is quite probable that the maximum of the growth rate is located at higher temperatures. The maxima of the nucleation rates are located at lower temperatures than the maxima of the growth rates. Theoretically, they can either be located at a large distance from each other or overlap. Since the accurate position of the maximum of the growth rate is not known, we can only to make the inference that, for the compositions under investigation, the positions of the temperature maxima of the nucleation and growth rates are spaced along the temperature scale by an uncertain value no less than 260˚C (720˚C - 460˚C).
6.2. Crystal Growth Rate: Dependence on the Time of Holding of the Glasses at the Temperature of 450˚C
The growth rates of lithium disilicate crystals as a function of the holding time glasses at a constant temperature were investigated at the temperature of 450˚C. The results of the measurements are presented in Figure 8.
It can be seen from this figure that the dependence of the growth rate of lithium disilicate crystals in glasses no. 1, no. 2 and no. 3 on the time of low-temperature heat treatment exhibit an oscillatory behavior with a gradual decay of the oscillation amplitude and a retardation of the growth process. In the framework of the existing theories of nucleation and growth, it was demonstrated for metal alloys [110] that the retardation of the growth process is caused simultaneously by nucleation, growth, and dissolution of particles. For glass no. 4 (with the stoichiometric lithium disilicate composition),


Figure 8. Dependences of the growth rate U on the time of heat treatment at 450˚C for glasses (a) no. 1, (b) no. 2, (c) no. 3 and (d) no. 4. Samples were developed at the temperature of 600˚C for 10 min.
the crystal growth rate does not depend on the time of low-temperature heat treatment (Figure 8(c)).
The gradual decrease in the crystal growth rate with an increase in the time of holding of the glass at a temperature of 450˚C for glasses no. 1, no. 2 and no. 3 (Figures 8(a)-8(c)) can be explained by the fact that the crystals grow in the glass with the composition lying in the metastable phase separation region. A part of the material is spent for forming the crystal layer, and the growing crystal appears to be surrounded by the region depleted in the building material (the so-called diffusion zone) (Figure 9). The formation of the next crystal layer requires the time it takes for phase separated droplets to come to the crystal growth region. In this case, the phase separated droplets consist predominantly of SiO2.
When the droplets come closer to the growing lithium disilicate crystal, they will block its growth, which manifests itself in the dependences (Figures 8(a)-8(c)) as a decrease in the crystal growth rate.
7. Conclusions
Thus, we have investigated the kinetics of phase separation in three lithium silicate glasses containing 23.4, 26.0 and 29.1 mol% Li2O with the compositions lying in the phase separation region in the Li2O-SiO2 system. It has been found that the temperature dependences of the ratio of the number of traces to the maximum number of traces NS/NSmax and the ratio of the radius of the particle trace to the maximum radius R/Rmax exhibit maxima. The crystal nucleation rate has been studied in glasses with the compositions displaced from the stoichiometric lithium disilicate composition toward an increase in the SiO2 content. The following features have been revealed.
1) The absolute values of the crystal nucleation rate in glasses with the lower content Li2O than that in the glass with the stoichiometric lithium disilicate composition vary insignificantly.
2) The position of the temperature maximum of the nucleation rate for the glass with the lower lithium oxide content is shifted on the temperature scale by 12˚C. This relatively small change in the quantities Ist(Tmax) and Tmax is explained by the fact that the glasses with the displaced composition correspond to the phase separation region in

Figure 9. Electron microscopic images of glasses (a) no. 1 and (b) no. 2 preliminarily heat treated at the temperature of 440˚C for 2 h and developed at 600˚C for 10 min.
the Li2O-SiO2 system, so that the lithium disilicate in glasses with displaced composition nucleates in phase separated inhomogeneities with compositions that are displaced toward the lithium disilicate composition and depend weakly on the temperature.
Variations in the crystal growth rate have been investigated as a function of the temperature and time of heat treatment. It has been shown that the temperature dependences of the crystal growth rate tend to an extremum. It has been found that the crystal growth rate depends substantially on the heat treatment time for phase separated glasses and that the growth rate is constant for the glass with the stoichiometric lithium disilicate composition. The dependence of the crystal growth rate on the heat treatment time in phase separated glasses exhibits a damped oscillatory behavior, which is explained by the depletion of the building material for crystals and the necessity of some time for its supply. Therefore, the performed investigations allow us to draw the following conclusion. Unlike the universally accepted opinion, the phase separation not only does not facilitate the conditions for the formation and growth of crystals but, in some cases (see, for example, the dependences of the crystal growth rate on the time of low-temperature heat treatment), even retards the crystal growth process. The data obtained on the nucleation and growth of crystals help to find optimum compositions and temperature-time conditions for the preparation of many glass-ceramic nanomaterials in lithium silicate system, including photostructured materials.
Cite this paper
Sycheva, G.A. (2016) Crystal Growth and Nucleation in Glasses in the Lithium Silicate System. Jour- nal of Crystallization Process and Tech- nology, 6, 29-55. http://dx.doi.org/10.4236/jcpt.2016.64004
References
- 1. Liebau, F. (1961) Untersuchungen an Schichtsilicaten des Formeltypes Am(Si2O5)n. I. Die Kristallstructur der Zimmertemperaturform des Li2Si2O5. Acta Crystallographica, 14, 389- 395.
http://dx.doi.org/10.1107/S0365110X61001303 - 2. Jaccodine, R.J. (1961) Study of Devitrification of Lithium Glass. Journal of the American Ceramic Society, 44, 8-11.
http://dx.doi.org/10.1111/j.1151-2916.1961.tb13708.x - 3. Kalinina, A.M., Filipovich, V.N., Kolesova, V.A. and Bondar I.A. (1963) On the Products of Crystallization of Lithium Silicate Glasses. In: Stekla, M.-L., Ed., The Vitreous State. Vyp.1. Katalizirovannaya kristallizatsiya Stekla (pp. 53-66). M.-L.: Izv. Akad. Nauk SSSR. (In Russian)
- 4. Glasser, F.P. (1967) Crystallisation of Lithium Disilicate from Li2O-SiO2 Glasses. Physics and Chemistry of Glasses, 8, 224-232.
- 5. Ito, M., Sacaino, T. and Moriya, T. (1968) Study on the Process of Crystallization of the Li2O2SiO2 Glass. I. Rates of Crystal Growth and Nucleation. Bulletin of the Tokyo Institute of Technology, 127-149.
- 6. Ito, M., Sacaino, T. and Moriya, T. (1968) Study on the Process of Crystallization of the Li2O2SiO2 glass I Variation of Electric Properties with Crystallization. Bulletin of the Tokyo Institute of Technology, 151-171.
- 7. Filipovich, V.N. and Kalinina, A.M. (1968) On the Nature of the Influence of Heat Treatment on the Crystallization Kinetics of Lithium Silicate Glasses. Izvesttiya Akademii Nauk SSSR Neorganicheskie Materialy, 4, 1532-1538. (In Russian)
- 8. Kinser, D.L. and Hench, L.L. (1968) Effect of a Metastable Precipitate on the Electrical Properties of an Li2O-SiO2 Glass. Journal of the American Ceramic Society, 51, 445-448.
http://dx.doi.org/10.1111/j.1151-2916.1968.tb11916.x - 9. Ogura, T., Hayami, R. and Kadota, M. (1968) Kinetics and Mechanism of Crystallization of Lithium Silicate Glasses. Journal of the Ceramic Association, 76, 277-284.
http://dx.doi.org/10.2109/jcersj1950.76.876_277 - 10. Freiman, S.W. and Hench, L.L. (1968) Kinetics of Crystallization in Li2O-SiO2 Glasses. Journal of the American Ceramic Society, 51, 382-387.
http://dx.doi.org/10.1111/j.1151-2916.1968.tb11898.x - 11. Kashchiev, D. (1969) Solution of the Non-Steady State Problem in Nucleation Kinetics. Surface Science, 14, 209-220.
http://dx.doi.org/10.1016/0039-6028(69)90055-7 - 12. Filipovich, V.N. and Kalinina, A.M. (1970) Crystallization Kinetics of Lithium Disilicate from Simple and Complex Glasses. Izvesttiya Akademii Nauk SSSR Neorganicheskie Materialy, 6, 351-356. (In Russian)
- 13. Kalinina, A.M. (1970) On the Polymorphism of Lithium Disilicate. Izvesttiya Akademii Nauk SSSR Neorganicheskie Materialy, 6, 907-913. (In Russian)
- 14. Hench, L.L., Frieman, S.W. and Kinser, D.L. (1971) The Early Stages of Crystallization in Li2O-2SiO2 Glass. Physics and Chemistry of Glasses, 12, 58-63.
- 15. Sycheva, G.A. (1972) Temperature Dependence of the Nucleation Rate of Crystals in Some Silicate Glasses/Brief Abstracts of the Conference of Young Specialists. Institute of Silicate Chemistry Behalf I.V. Grebenshchikova, Leningrad, 15-16. (In Russian)
- 16. Matusita, K. and Tashiro, M. (1973) Rate of Homogeneous Nucleation in Alkali Disilicate Glasses. Journal of Non-Crystalline Solids, 11, 471-484.
http://dx.doi.org/10.1016/0022-3093(73)90092-6 - 17. James, P.F. and Keown, S.R. (1974) Study of Crystallization in Lithium Silicate Glasses Using High-Voltage Microscopy. Philosophical Magazine, 30, 789-802.
http://dx.doi.org/10.1080/14786437408207235 - 18. James, P.F. (1974) Kinetics of Crystal Nucleation in Lithium Silicate Glasses. Physics and Chemistry of Glasses, 15, 95-105.
- 19. Kalinina, A.M., Fokin, V.M. and Filipovich, V.N. (1976) On the Method of Determining the Parameters Characterizing the Crystal Nucleation in Glasses. Fizika i Khimiya Stekla, 2, 298-305. (In Russian)
- 20. Kalinina, A.M., Fokin, V.M. and Filipovich, V.N. (1977) Induction Period of Crystal Nucleation in Li2O·2SiO2 Glass and Its Temperature Dependence. Fizika i Khimiya Stekla, 3, 122-129. (In Russian)
- 21. Fokin, V.M., Filipovich, V.N. and Kalinina, A.M. (1977) The Effect of Preliminary Heat Treatment on Crystal Nucleation in Li2O-2SiO2 Glass. Fizika i Khimiya Stekla, 3, 129-136. (In Russian)
- 22. James, P.F., Scott, B. and Armstrong, P. (1978) Kinetics of Crystal Nucleation in Lithium Disilicate Glass. A Comparison between Melts Prepared in Platinum and Silica Crucibles and between Melts Prepared from Ordinary and High Purity Starting Materials. Physics and Chemistry of Glasses, 19, 24-27.
- 23. Rowlands, E.G. and James, P.F. (1979) Analysis of Steady State Crystal Nucleation Rates in Glasses. Part 1. Methods of Analysis and Application to Lithium Disilicate Glass. Physics and Chemistry of Glasses, 20, 1-8.
- 24. Rowlands, E.G. and James, P.F. (1979) Analysis of Steady State Crystal Nucleation Rates in Glasses. Part 2. Further Comparison between Theory and Experiment for Lithium Disilicate Glass. Physics and Chemistry of Glasses, 20, 9-14.
- 25. Matusita, K. and Sakka, S. (1979) Kinetic Study of the Crystallisation of Glass by Differential Scanning Calorimetry. Physics and Chemistry of Glasses, 20, 81-84.
- 26. Ghoneim, N.A., El Batal, H.A., Ahmed, A.A. and Khalifa, F.A. (1979) Crystallization of Lithium Trisilicate Glasses. Transactions and journal of the British Ceramic Society, 78, 15- 22.
- 27. Gonzalez-Oliver, C.J.R., Johnson, P.S. and James, P.F. (1979) Influence of Water Content on the Rates of Crystal Nucleation and Growth in Lithia-Silicate and Soda-Lime-Silica Glasses. Journal of Materials Science, 14, 1159-1169.
http://dx.doi.org/10.1007/BF00561300 - 28. Fokin, V.M., Kalinina, A.M. and Filipovich, V.N. (1981) Nucleation in Silicate Glasses and Effect of Preliminary Heat Treatment on It. Journal of Crystal Growth, 52, 115-121.
http://dx.doi.org/10.1016/0022-0248(81)90178-0 - 29. Marotta, A., Buri, A., Branda, F. and Saiello, S. (1982) Nucleation and Crystallization of Li2O·2SiO2—A DTA Study. In: Simmons, J.H., Uhlmann, D.R. and Beall, G.H., Eds., Advances in Ceramics, Nucleation and Crystallization in Glasses, Vol. 4, American Ceramic Society, Columbus, 146-152.
- 30. Kalinina, A.M., Sycheva, G.A. and Filipovich, V.N. (1983) Homogeneous and Heterogeneous Crystal Nucleation in Some Silicate Glasses. Glastehnische Berichte, 2, 816-821.
- 31. Huang, Z.J., Yoko, T., Kamiya, K. and Sakka, S. (1983) Effect of Water on the Crystallization of Li2O-SiO2 Glasses and Gels. Yogyo-Kyokai-Shi, 91, 215-221. (In Japanese)
http://dx.doi.org/10.2109/jcersj1950.91.1053_215 - 32. Zanotto, E.D. and James, P.F. (1985) Experimental Test of the Classical Nucleation Theory for Glasses. Journal of Non-Crystalline Solids, 74, 373-394.
http://dx.doi.org/10.1016/0022-3093(85)90080-8 - 33. Sycheva, G.A., Kalinina, A.M. and Filipovich, V.N. (1986) Change of Nucleation Kinetic of Lithium Di-and Metasilicate Crystals in Photosensitive Glasses at Transition from Heterogeneous to Homogeneous Nucleation. Fizika i Khimiya Stekla, 11, 123-125. (In Russian)
- 34. Kalinina, A.M., Sycheva, G.A., Filipovich, V.N., et al. (1986) Auto-Catalytic Nucleation of Lithium Silicate Glasses. Fizika i Khimiya Stekla, 12, 260-264. (In Russian)
- 35. Kalinina, A.M., Fokin, V.M., Sycheva, G.A. and Filipovich, V.N. (1986) Three Types of Catalysis of Lithium Disilicate Crystal Nucleation in Lithium Silicate Glasses. Proceedings of the 14th International Congress on Glass, 1, 366-373.
- 36. Kalinina, A.M., Sycheva, G.A. and Filipovich, V.N. (1986) Catalyzed and Spontaneous Nucleation of Lithium Disilicate Crystals in Nonphotosensitive and Photosensitive Glasses, in Katalizirovannaya kristallizatsiya Stekla. In: Hodakovskaya, R.Ya., Ed., Trudy Gosudarstvennogo Instituta Stekla (Catalyzed Crystallization of a Glass: Transactions of the State Institute of Glass), Gos. Inst. Stecla, Moscow, 56-59. (In Russian)
- 37. Hishinuma, A. and Uhlman, D.R. (1987) Nucleation Kinetics in Some Silicate Glass- Forming Melts. Journal of Non-Crystalline Solids, 95-96, 449-456.
http://dx.doi.org/10.1016/S0022-3093(87)80143-6 - 38. Braetsh, V. and Frischat, G.H. (1987) Crystallization and Phase Separation in the System Li2O-SiO2. Journal of Non-Crystalline Solids, 95-96, 457-464.
http://dx.doi.org/10.1016/S0022-3093(87)80144-8 - 39. Market, M.F., Wang, T.H. and James, P.F. (1988) Nucleation and Growth Kinetics of Lithium Disilicate and Lithium Metasilicate in Lithia-Silica Glasses. Physics and Chemistry of Glasses, 29, 240-248.
- 40. Filipovich, V.N., Kalinina, A.M. and Sycheva, G.A. (1988) Glass Formation and Catalyzed Crystal Nucleation. Trudy 8th Vsesoyuznogo soveshchaniya po stekloobraznomu sostoyaniyu [Proceeding of 8th All-Union Conference on the Vitreous State], Leningrad, 28-31 October 1986, 87-96. (In Russian)
- 41. Schlesinger, M.E. and Lynch, D.C. (1989) Effect of VB and VIB Oxides on Nucleation Parameters in Lithium Disilicate Glass. Journal of Non-Crystalline Solids, 108, 237-248.
http://dx.doi.org/10.1016/0022-3093(89)90294-9 - 42. Sycheva, G.A., Kalinina, A.M. and Filipovich, V.N. (1989) Transition Kinetic from the Heterogeneous to the Homogeneous Nucleation in Some Silicate Glasses. In: Glass and Fine Ceramics, Varna, 18-20 September 1987: Proceedings of 9th National Scientific Conference with International Participation, Sophia, 239-245.
- 43. Ray, C.S. and Day, D.E. (1990) Determining the Nucleation Rate Curve for Lithium Disilicate Glass by Differential Thermal Analysis. Journal of the American Ceramic Society, 73. 439-442.
http://dx.doi.org/10.1111/j.1151-2916.1990.tb06532.x - 44. Boiko, G.G., Sycheva, G.A. and Valjuk, L.G. (1990) Influence of Synthesis Conditions of Photosensitive Glass on Its Crystallization Kinetics. In: Glass and Fine Ceramics, Varna, 18-20 October 1990: Proceedings of 10th National Scientific Conference with International Participation, 1, 282.
- 45. Kalinina, A.M., Filipovich, V.N. and Sycheva, G.A. (1991) The Mutual Influence of Crystal Nucleation in Complex Glasses. In: Hodakovskaya, R.Ya., Ed., Amorfno-kristallicheskie materially: Sintez, struktura, svoistva, primenenie. Sbornik trudov MKhTI im [Amorphous- Crystalline Materials: Synthesis, Structure, Properties, and Applications. A Collection of Articles of the Mendeleev University of Chemical Technology], Mendeleev University of Chemical Technology, Moscow, 77-85. (In Russian)
- 46. Deubener, J., Bruckner, R. and Sterenitzke, M. (1993) Induction Time Analysis of Nucleation and Crystal Growth in Di- and Metasilicate Glasses. Journal of Non-Crystalline Solids, 163, 1-12.
http://dx.doi.org/10.1016/0022-3093(93)90638-E - 47. Boiko, G.G., Sycheva, G.A. and Valjuk, L.G. (1995) Influence of Synthesis Conditions on the Kinetics of Crystallization of Photosensitive Lithium Silicate Glasses. Fizika i Khimiya Stekla, 21, 45-52. (In Russian)
- 48. Davis, M.J. (1996) Influence of Water and Thermal History on Viscosity and Nucleation Kinetics of Lithium Disilicate Melt. Geology and Geophysics, Yale University, New Haven, 159.
- 49. Sycheva, G.A. (1996) Transition from the Heterogeneous to the Homogeneous Nucleation in Some Silicate and Phosphate Glasses. Proceedings of the International Symposium on Glass Problems, 2, 373-379.
- 50. Mishima, N., Ota, R., Wakasugi, T. and Fukunaga, J. (1996) Analysis of Crystallization Behavior in Li2O 2SiO2 Glass by DTA Method Based on a Liquid Model. Journal of Non- Crystalline Solids, 197, 19-24.
http://dx.doi.org/10.1016/0022-3093(95)00570-6 - 51. Narayan, K.L., Kelton, K.F. and Ray, C.S. (1996) Effect of Pt Doping on Nucleation and Crystallization in Li2O 2SiO2 Glass: Experimental Measurements and Computer Modeling. Journal of Non-Crystalline Solids, 195, 148-157.
http://dx.doi.org/10.1016/0022-3093(95)00526-9 - 52. Zanotto, E.D. and Gomes Leite, M.L. (1996) The Nucleation Mechanism of Lithium Disilicate Glass Revisited. Journal of Non-Crystalline Solids, 202, 145-151.
http://dx.doi.org/10.1016/0022-3093(96)00187-1 - 53. Davis, M.J., Ihinger, P.D. and Lasaga, A.C. (1997) Influence of Water on Nucleation Kinetics in Silicate Melt. Journal of Non-Crystalline Solids, 219, 62-69.
http://dx.doi.org/10.1016/S0022-3093(97)00252-4 - 54. Kalinina, A.M., Filipovich, V.N. and Sycheva, G.A. (1997) Heterogeneous Nucleation in Photosensitive Glasses. Journal of Non-Crystalline Solids, 219, 80-83.
http://dx.doi.org/10.1016/S0022-3093(97)00255-X - 55. Holland, D., Iqbal, Y., James, P. and Lee, B. (1998) Early Stages of Crystallization of Lithium Disilicate Glasses Containing P2O5—An NMR Study. Journal of Non-Crystalline Solids, 232-234, 140-146.
http://dx.doi.org/10.1016/S0022-3093(98)00381-0 - 56. Sycheva G.A. (2015) Determination of the Size of the Critical Nucleus of Crystals in Lithium and Sodium Silicate Glass. Glass Physics and Chemistry, 41, 302-306.
http://dx.doi.org/10.1134/S1087659615030165 - 57. Iqbal, Y., Lee, B., Holland, D. and James, P. (1998) Metastable Phase formation in the Early Stage Crystallization of the Lithium Disilicate Glass. Journal of Non-Crystalline Solids, 224, 1-16.
http://dx.doi.org/10.1016/S0022-3093(97)00453-5 - 58. Hasdemir, I., Bruckner, R., Deubener, J. (1998) Crystallization of Lithium Di- and Metasilicate Solid Solutions from Li2O-SiO2 Glasses. Physics and Chemistry of Glasses, 39, 253- 257.
- 59. Davis, M.J. and Ihinger, P.D. (1998) Heterogeneous Crystal Nucleation on Bubbles in Silicate Melt. American Mineralogist, 83, 1008-1015.
http://dx.doi.org/10.2138/am-1998-9-1008 - 60. Davis M.J. and Ihinger P.D. (1999) Influence of Hydroxyl on Glass Transformation Kinetics in Lithium Disilicate Melt and a Re-Evaluation of Structural Relaxation in NBS 710 and 711. Journal of Non-Crystalline Solids, 244, 1-15.
http://dx.doi.org/10.1016/S0022-3093(98)00844-8 - 61. Burgner, L.L., Weinberg, M.C., Lucas, P., Soares Jr., P.C., Zanotto, E.D. (1999) XRD Investigation of Metastable Phase Formation in Li2O-SiO2 Glasses. Journal of Non-Crystalline Solids, 255, 264-268.
http://dx.doi.org/10.1016/S0022-3093(99)00376-2 - 62. Burgner, L.I., Lucas, P., Weinberg, M.C., Soares Jr., P.C. and Zanotto, E. (2000) D. Metastable Phase Formation in the Early Stage Crystallisation of Lithium Disilicate Glass. Journal of Non-Crystalline Solids, 274, 188-194.
http://dx.doi.org/10.1016/S0022-3093(00)00187-3 - 63. Kitamua, N., Fukumi, K., Mizoguchi, H., Makihara, M., Higuchi, A., Ohno, N. and Fukunaga, T. (2000) High Pressure Densification of Lithium Silicate Glasses. Journal of Non-Crystalline Solids, 274, 244-248.
http://dx.doi.org/10.1016/S0022-3093(00)00190-3 - 64. Kanert, O., Kuchler, R., Suter, D., Shannon, G.N. and Jain, H. (2000) Effect of Devitrification on the Ionic Diffusion of Li-Disilicate. Journal of Non-Crystalline Solids, 274, 202-207.
http://dx.doi.org/10.1016/S0022-3093(00)00189-7 - 65. Burgner, L.L. and Weinberg, M.C. (2001) Crystal Growth Mechanisms in Inorganic Glasses. Physics and Chemistry of Glasses, 42, 184-190.
- 66. Burgner, L.L. and Weinberg M.C. (2001) An Assessment of Crystal Growth Behavior in Lithium Disilicate Glass. Journal of Non-Crystalline Solids, 279, 28-43.
http://dx.doi.org/10.1016/S0022-3093(00)00325-2 - 67. Soares Jr., P.C., Zanotto, E.D., Fokin, V.M., Jain, H.J. and Weinberg, M.C. (2003) TEM and XRD Srudy of Early Crystallization of Lithium Disilicate Glasses. Journal of Non-Crystal- line Solids, 331, 217-227.
http://dx.doi.org/10.1016/j.jnoncrysol.2003.08.075 - 68. Filipovich, V.N. and Kalinina, A.M. (1971) On the Relation between the Maximum of the Crystal Nucleation Rate in Glasses and the Glass Transition Temperature, Izvesttiya Akademii Nauk SSSR Neorganicheskie Materialy, 7, 1844-1848.
- 69. Gonzalez-Oliver, C.J.R. (1979) Crystal Nucleation and Growth in Soda-Lime-Silica Glasses. PhD Thesis, the University of Sheffield, Sheffield, 175 p.
- 70. Gonzalez-Oliver, C.J.R. and James, P.F. (1980) Crystal Nucleation and Growth in a Na2O.2CaO.3SiO2 Glass. Journal of Non-Crystalline Solids, 38-39, 699-704.
http://dx.doi.org/10.1016/0022-3093(80)90518-9 - 71. Kalinina, A.M., Filipovich, V.N. and Fokin V.M. (1980) Stationary and Non-Stationary Crystal Nucleation Rates in the Na2O.2CaO.3SiO2 Glass of Stoichiometric Composition. Journal of Non-Crystalline Solids, 38-39, 723-728.
http://dx.doi.org/10.1016/0022-3093(80)90522-0 - 72. James, P.F. (1982) Nucleation in Glass-Forming Systems—A Review. In: Simmons, J.H. and Beau, G.H., Eds., Nucleation and Crystallization in Glasses Vol. 4: Advances in Ceramics, American Ceramic Society, Columbus, 1-48.
- 73. Ramsden, A.H. and James, P.F. (1984) The Effect of Amorphous Phase Separation on Crystal Nucleation Kinetics in BaO-SiO2 Glasses. Part 1. General Survey. Journal of Materials Science, 19, 1406-1419.
http://dx.doi.org/10.1007/BF00563035 - 74. Ramsden A.H. and James, P.F. (1984) The Effect of Amorphous Phase Separation on Crystal Nucleation Kinetics in BaO-SiO2 Glasses. Part 2. Isothermal Heat Treatments at 700°C. Journal of Materials Science, 19, 2894-2908.
http://dx.doi.org/10.1007/BF01026965 - 75. James, P.F. (1993) Experimental Studies of Crystal Nucleation in Glasses. In: Weinberg, M.C., Ed., Nucleation and Crystallization in Liquids and Glasses Vol. 30: Ceramic Transactions, American Ceramic Society, Westerville, 3-12.
- 76. Gránásy, L., Wang, T. and James, P.F. (1998) Kinetics of Wollastonite Nucleation in CaO·SiO2 Glass. Journal of Chemical Physics, 108, 7317-7326.
http://dx.doi.org/10.1063/1.476150 - 77. Filipovich, V.N., Kalinina, A.M. and Sycheva, G.A. (1975) Temperature Dependence of the Rate of Nucleation of Acicular Crystals in Sodium Silicate Glass. Izvesttiya Akademii Nauk SSSR Neorganicheskie Materialy, 11, 1305-1308. (In Russian)
- 78. Fokin, V.M. and Yuritsyn, N.S. (1997) The Nucleation and Growth Rates of Sodium Metasilicate Crystals in Sodium Silicate Glass 44Na2O-56SiO2. Fizika i Khimiya Stekla, 23, 236-239. (In Russian)
- 79. Burgner, L.L. and Weinberg M.C. (2000) Crystal Nucleation Rates in a Na2O-SiO2 Glass. Journal of Non-Crystalline Solids, 261, 163-168.
http://dx.doi.org/10.1016/S0022-3093(99)00597-9 - 80. Sycheva, G.A. (1998) Evalution of the Surface Energy at the Crystal-Glass Interface in Sodium Silicate Glass 4Na2O-54SiO2. Fizika i Khimiya Stekla, 24, 47-53.
- 81. Burnett, D.G. and Douglas, R.W. (1971) Nucleation and Crystallization in the Soda-Baria-Silica System. Physics and Chemistry of Glasses, 12, 117-124.
- 82. Strnad, Z. and Douglas, R.W. (1973) Nucleation and Crystallization in the Soda-Lime-Silica System. Physics and Chemistry of Glasses, 14, 33-36.
- 83. Lakshmi Narayan, K. and Kelton, K.F. (1997) First Measurements of Time-Dependent Nucleation as a Function of Composition in Na2O·2CaO·3SiO2 Glasses. Journal of Non- Crystalline Solids, 220, 222-230.
http://dx.doi.org/10.1016/S0022-3093(97)00276-7 - 84. Potapov, O.V., Fokin, V.M., Suslova, L.Y., Filipovich, V.N. and Ugolkov, V.L. (2000) Influence of Na2O Content on the Nucleation Kinetics in Glasses of Composition Close to the Na2O·2CaO·3SiO2 Stoichiometry. Fizika i Khimiya Stekla, 26, 39-47.
- 85. Sycheva, G.A. (1999) Nucleation Kinetics of Lithium Metasilicate in Photosensitive Lithium Aluminosilicate Glass. Fizika i Khimiya Stekla, 25, 501-511.
- 86. Filipovich, V.N., Kalinina, A.M. and Sycheva, G.A. (1981) Catalyzed Crystallization of Pyroxene Glasses. Fizika i Khimiya Stekla, 7, 55-60. (In Russian)
- 87. Sycheva, G.A. and Nasedkin, V.V. (1992) Some Physica-Chemical Parameters of Volcanic Glass Formation. In: Nasedkin, V.V., Ed., Perlite Genesis, Nauka, Moscow, 188 p, 129-125. (In Russian)
- 88. Sycheva, G.A. (1997) Influence of Tungsten and Molybdenum Oxides on the Crystallization Kinetics of Tin (II) Pyrophosphate in the SnO-SnO2-ZnO-P2O5 System. Fizika i Khimiya Stekla, 23, 368-375.
- 89. Sycheva, G.A. (1998) Surface Energy at the Crystal Nucleus-Glass Interface in Alkali Silicate Glasses. Fizika i Khimiya Stekla, 24, 342-347.
- 90. Sycheva, G.A. and Sigaev, V.N. (1997) Crystallization Kinetics in Ca, Sr and Ba Borophosphate Systems. Proceedings of the 2nd International Conference on Borate Glasses, Crystals and Melts, 554s, 254-260.
- 91. Hinz, W. and Kunth, P. (1960) Vitrokeramprodukte mit niedrigem Warmeausdehnungskoeffizienten. Silikattechnik, 11, 506-511.
- 92. Vogel, W. and Gerth, K. (1962) Catalyzed Crystallization in Glass. Proceedings of the Symposium on Nucleation and Crystallization in Glasses and Melts, Columbus, April 1961, 11-22.
- 93. Filipovich, V.N. (1963) Initial Stages of Crystallization of Glasses and Formation of Glass Ceramics. In: Stekloobraznoe sostoyanie, Vyp. 1: Katalizirovannaya kristallizatsiya stekla [Vitreous State, Vol. 1: Catalyzed Crystallization in Glass], Nauka, Moscow, 9-24 (In Russian)
- 94. Maurer, R. (1962) Crystal Nucleation in a Glass Containing Titanium. Journal of Applied Physics, 33, 2132-2139.
http://dx.doi.org/10.1063/1.1728909 - 95. Ohlberg, S.M., Golob H.R. and Strichler, D. (1962) Crystal Nucleation by Glass in Glass Separation. Proceedings of the Symposium on Nucleation and Crystallization in Glasses and Melts, Columbus, April 1961, 55-62.
- 96. Stookey, C.D. (1959) Catalyzed Crystallization of Glass in Theory and Practice. Industrial & Engineering Chemistry, 51, 805-808.
http://dx.doi.org/10.1021/ie50595a022 - 97. Nakagawa, K. and Izumitani, T. (1969) Relationship between Phase Separation and Crystallization in Li2O-2.5SiO2 Glass and a Lithium Silicate Containing a Large Amount of Titanium Oxide. Physics and Chemistry of Glasses, 10, 179-184.
- 98. Phillips, S.V. and McMillan, P.W. (1965) Phase Separation and Crystallization in Li2O-SiO2 Glass and Li2O-SiO2-P2O5 Glasses. Glass Technology, 6, 46-51.
- 99. Tomozawa, M. (1973) Liquid Phase Separation and Crystal Growth in Li2O-SiO2 Glass. Physics and Chemistry of Glasses, 14, 112-113.
- 100. Milyukov, E.M. (1965) On the Phase Separation and Micro Crystallization of Glasses in Li2O-SiO2 and Li2O-Al2O3-SiO2 Systems. Optiko-Mekhanicheskaya Promishlennost, 31-36. (In Russian)
- 101. Harper, H., James, P.F. and McMillan P.W. (1970) Crystal Nucleation in Lithium Silicate Glasses. Discussions of the Faraday Society, 50, 200-205.
http://dx.doi.org/10.1039/df9705000206 - 102. Sycheva, G.A. (2009) Formation of the Bubble Structure in the 26Li2O-74SiO2 Glass. Glass Physics and Chemistry, 35, 267-273.
http://dx.doi.org/10.1134/S1087659609030055 - 103. Sycheva, G.A. (2009) Influence of the Presence of Bubbles on the Parameters of Crystal Nucleation in the 26LI2O-74SiO2 Glass. Glass Physics and Chemistry, 35, 602-612.
http://dx.doi.org/10.1134/S1087659609060091 - 104. Mel’nichenko, T.D., Rizak, V.M., Mel’nichenko ,T.N. and Fedelesh, V.I. (2004) Parameters of the Fluctuation Free Volume Theory for Glasses in the Ge-As-Se System. Glass Physics and Chemistry, 30, 406-414.
http://dx.doi.org/10.1023/B:GPAC.0000045920.01447.ba - 105. Holler, W.S., Blackburn, D.H. and Simmons, J.H. (1974) Miscibility Gaps in Alkali-Silicate Binaries—Data and Thermodynamic Interpretation. American Ceramic Society Bulletin, 57, 120-126.
http://dx.doi.org/10.1111/j.1151-2916.1974.tb10832.x - 106. Andreev, N.S., Mazurin, O.V., Porai-Koshits, E.A., Roskova, G.P. and Filipovich, V.N. (1974) Yavleniya likvatsii v steklakh. Nauka, Leningrad. Translated under the Title Phase Separation in Glasses, 1984, North-Holland, Amsterdam.
- 107. Mazurin, O.V., Raskova, G.P., Aver’yanov, V.I. and Antropova, T.V. (1991) Dvukhfaznye stekla: Struktura, svoistva, primenenie [Two-Phases Glasses: Structure, Properties, and Applications]. Varshal, B.G., Ed., Nauka, Leningrad.
- 108. Braetsch, V. and Frischta, G.H. (1987) Crystallization and Phase Separation in the System Li2O-SiO2. Journal of Non-Crystalline Solids, 95-96, 457-462.
http://dx.doi.org/10.1016/S0022-3093(87)80144-8 - 109. Burgner, L.L., Weinberg, M.C. and Symmons, J.H. () Early Stage Crystallization Kinetics of Lithium Disilicate and Sodium Silicate Glasses. Final Report of US Department of Energy Grant DE-FG03ER45500, The University of Arizona, Tucson, 85721.
- 110. Ardell, A.S. (1972) The Effect of Volume Fraction on Particle Coarsening: The Theoretical Consideration. Acta Metallurgica, 20, 61-71.
http://dx.doi.org/10.1016/0001-6160(72)90114-9













