Applied Mathematics
Vol.3 No.9(2012), Article ID:23002,6 pages DOI:10.4236/am.2012.39149
Existence of Positive Solutions for a Third-Order Multi-Point Boundary Value Problem
1Laboratory of Advanced Materials, Faculty of Sciences, University Badji Mokhtar-Annaba, Annaba, Algerie
2Université 8 mai 1945 Guelma, Guelma, Algerie
Email: a_guezane@yahoo.fr, zenkoufi@yahoo.fr
Received June 25, 2012; revised July 25, 2012; accepted August 3, 2012
Keywords: Guo’s Fixed Point Theorem; Three Point Boundary Value Problem; Positive Solution; Leray Schauder Non-Linear Alternative; Contraction Principle
ABSTRACT
By using Leray-Schauder nonlinear alternative, Banach contraction theorem and Guo-Krasnosel’skii theorem, we discuss the existence, uniqueness and positivity of solution to the third-order multi-point nonhomogeneous boundary value problem (BVP1):

where 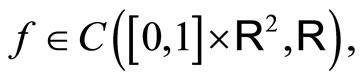

 for
for 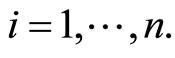 The interesting point lies in the fact that the nonlinear term is allowed to depend on the first order derivative
The interesting point lies in the fact that the nonlinear term is allowed to depend on the first order derivative .
.
1. Introduction
It shows that problems related to nonlocal conditions have many applications in many problems such as in the theory of heat conduction, thermoelasticity, plasma physics, control theory, etc. The current analysis of these problems has a great interest and many methods are used to solve such problems. Recently certain three point boundary value problems for nonlinear ordinary differential equations have been studied by many authors [1-9]. The literature concerning these problems is extensive and application of theorems of functional analysis has attracted more interest. Recently, the study of existence of positive solution to third-order boundary value problems has gained much attention and is a rapidly growing field see [1,2,6,8-11]. However the approaches used in the literature are usually topological degree theory and fixed-point theorems in cone. We are interested in the existence, uniqueness and positivity of solution to the third-order multi-point nonhomogeneous boundary value problem (BVP1):
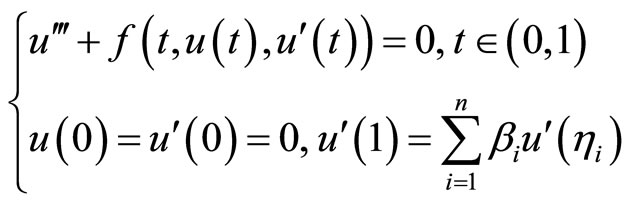
where 

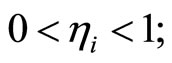 for
for 
The organization of this paper is as follows. In Section 2, we present some preliminaries that will be used to prove our results. In Section 3, we discuss the existence and uniqueness of solution for the BVP1 by using Leray-Schauder nonlinear alternative and Banach contraction theorem. Finally, in Section 4 we study the positivity of solution by applying the Guo-Krasnosel’skii fixed point theorem.
2. Preliminary Lemmas
We first introduce some useful spaces. we will use the classical Banach spaces,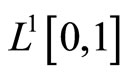

 . We also use the Banach space
. We also use the Banach space
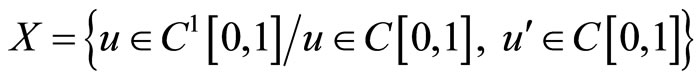 , equipped with the norm
, equipped with the norm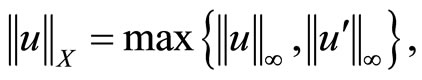 where
where 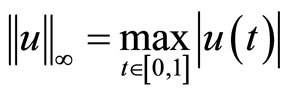 .
.
Firstly we state some preliminary results.
Lemma 1 Let  and
and 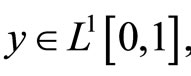 then the problem
then the problem
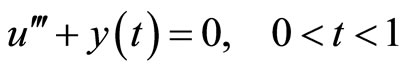 (2.1)
(2.1)
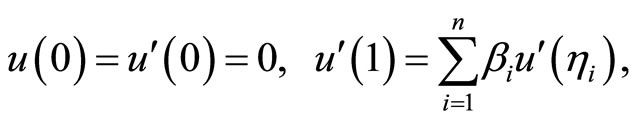 (2.2)
(2.2)
has a unique solution
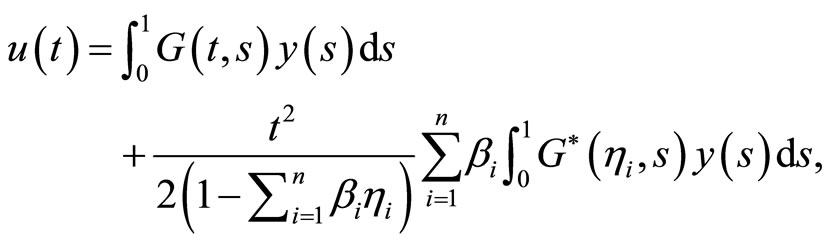 (2.3)
(2.3)
where
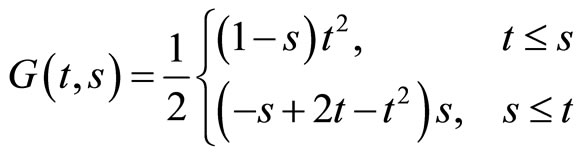 (2.4)
(2.4)
 (2.5)
(2.5)
Proof Integrating the Equation (2.1), it yields
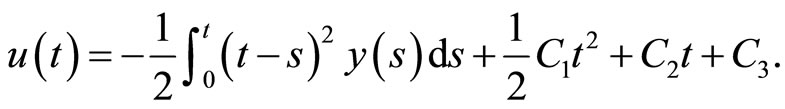
From the boundary condition  we deduce that
we deduce that  and
and .
.
And the boundary condition 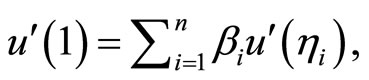 implies
implies
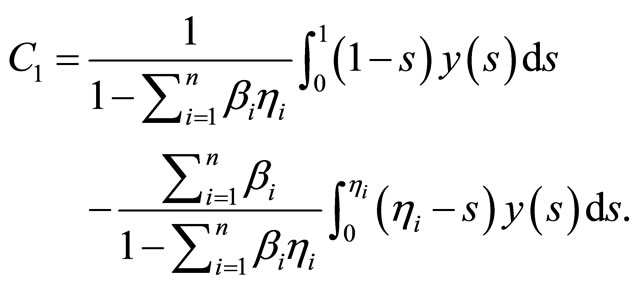
Therefore we have

Now it is easy to have

which achieves the proof of Lemma 1.
We need some properties of functions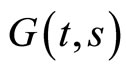 .
.
Lemma 2 For all t, s, such that 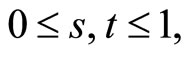 we have
we have
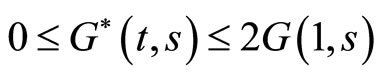
Proof It is easy to see that, if 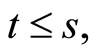
 If
If 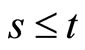 then
then

Lemma 3 For all t, s such that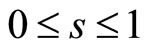 ,
, 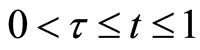 , we have
, we have
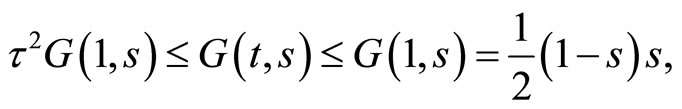
Proof For all 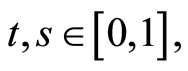 if
if  it follows from (2.4) that
it follows from (2.4) that
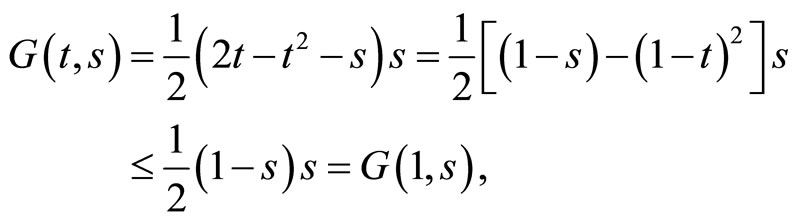
and
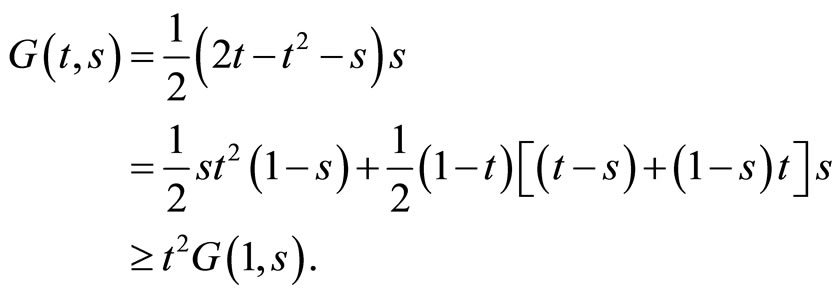
If  it follows from (2.4) that
it follows from (2.4) that
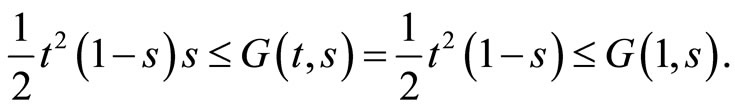
Therefore
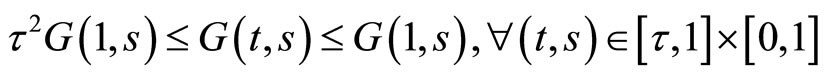
Lemma 4 (See [6]) We define an operator  by
by
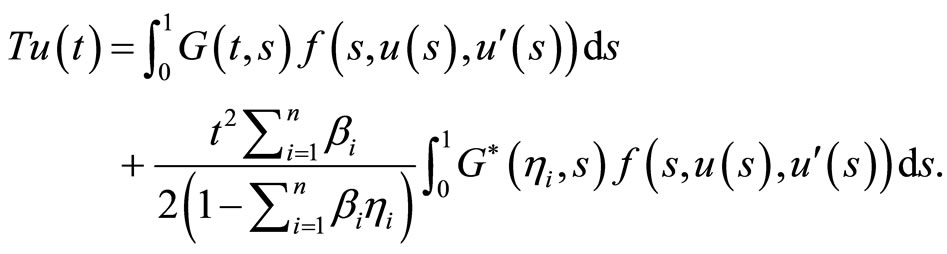
Lemma 5 (See [5]) The function 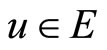 is a solution of the (BVP1) if and only if T has a fixed point in X, i.e.
is a solution of the (BVP1) if and only if T has a fixed point in X, i.e.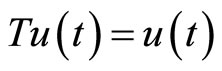 .
.
3. Existence Results
Now, we give some existence results for the BVP1 Theorem 6 Assume that  and there exist nonnegative functions
and there exist nonnegative functions  such that
such that 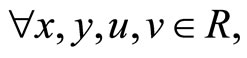
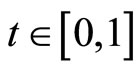 we have
we have

and

then, the (BVP1) has a unique solution in 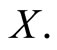
Proof We shall prove that T is a contraction. Let  then
then

So we can obtain

Similarly, we have
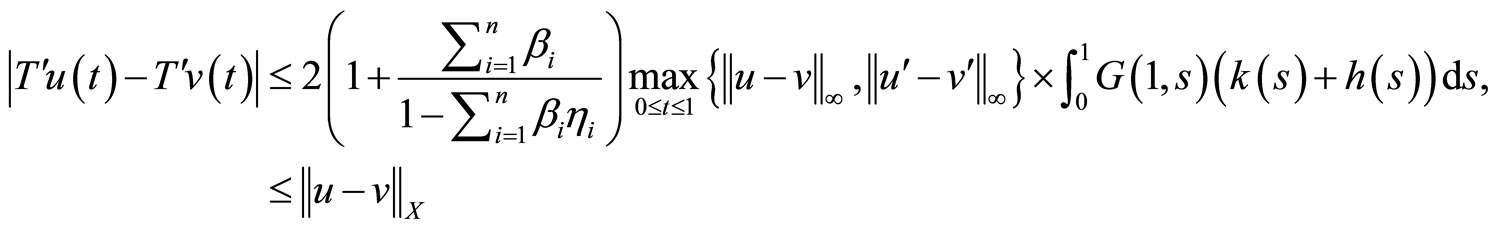
From this we deduce
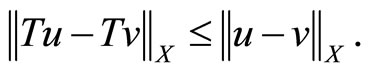
Then T is a contraction. From Banach contraction principe we deduce that T has a unique fixed point which is the unique solution of (BVP1).
We will employ the following Leray-Schauder nonlinear alternative [12].
Lemma 7 Let Fbe Banach space and  be a bounded open subset of F,
be a bounded open subset of F,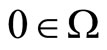 .
. 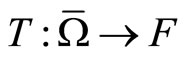 be a completely continuous operator. Then, either there exists
be a completely continuous operator. Then, either there exists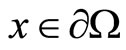 ,
, 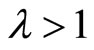 such that
such that , or there exists a fixed point
, or there exists a fixed point 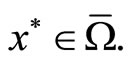
Theorem 8 We assume that 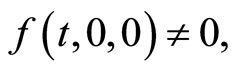
 and there exist nonnegative functions
and there exist nonnegative functions 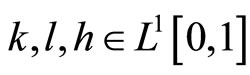 such that
such that

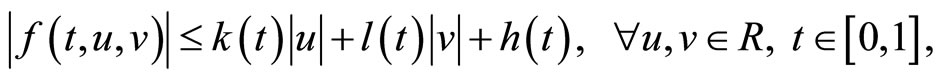
Then the (BVP1) has at least one nontrivial solution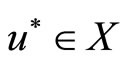 .
.
Proof Setting
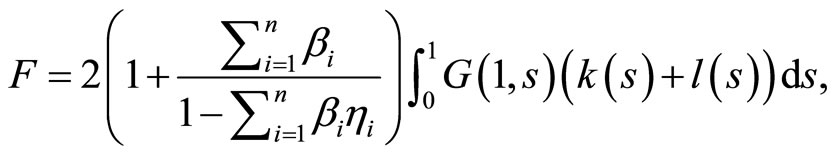

Remarking that 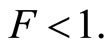
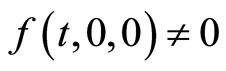 and
and 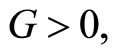 then there exists an interval
then there exists an interval 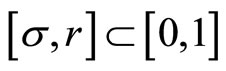 such that
such that
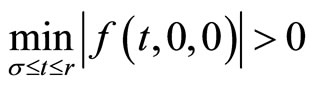 and
and 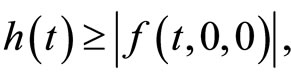 a.e.
a.e. 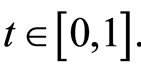
Le 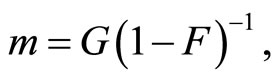
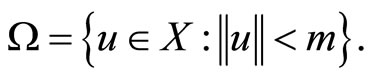 With the help of Ascoli-Arzela Theorem we show that
With the help of Ascoli-Arzela Theorem we show that 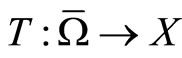 is a completely continuous mapping. We assume that
is a completely continuous mapping. We assume that 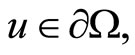
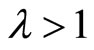 such that
such that  then
then 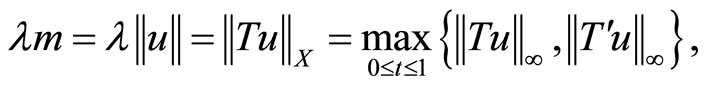 we have
we have

and
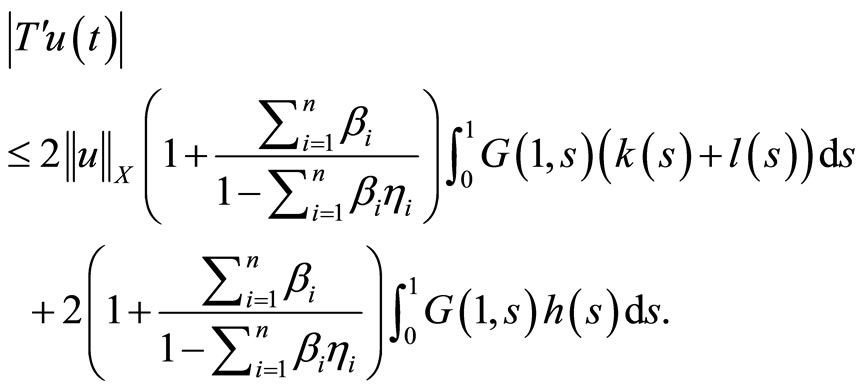
This shows that 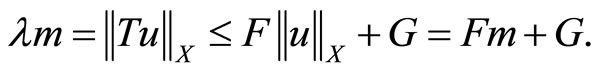 From this we get
From this we get
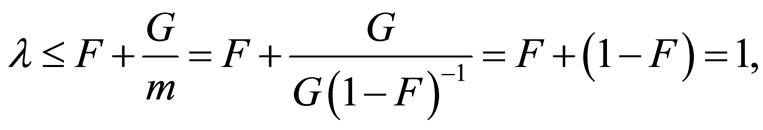
this contradicts 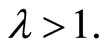 By applying Lemma 7, T has a fixed point
By applying Lemma 7, T has a fixed point 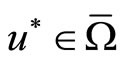 and then the BVP1 has a nontrivial solution
and then the BVP1 has a nontrivial solution 
4. Positive Results
In this section, we discuss the existence of positive solutions for (BVP1). We make the following additional assumptions.
(Q1) 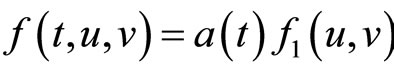 where
where 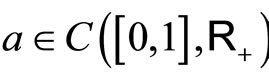 and
and 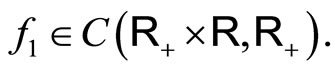
(Q2) 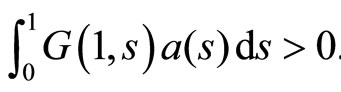
We need some properties of functions 
Lemma 9 For all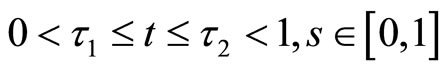 , we have
, we have
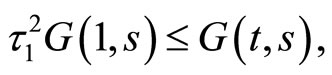
 where
where .
.
Proof It is easy to see that.
If 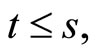
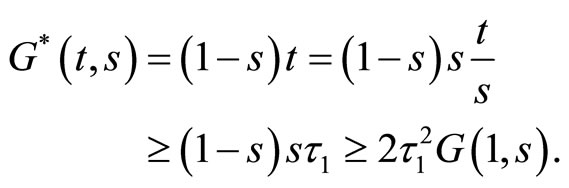
If 

Lemma 10 Let 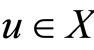 and assume that
and assume that 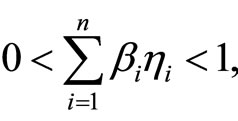
then the unique solution u of the (BVP1) is nonnegative and satisfies
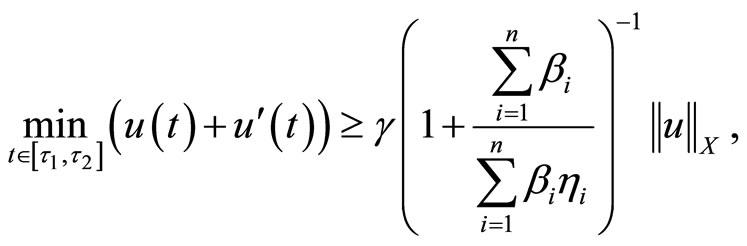
Proof Let 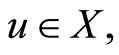 it is obvious that
it is obvious that 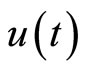 is nonnegative. For any
is nonnegative. For any  by (2.3) and Lemmas 2 and 3, it follows that
by (2.3) and Lemmas 2 and 3, it follows that
 (4.1)
(4.1)
On the other hand, (2.4) and Lemma 11 imply that, for any 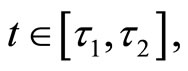 we have
we have

From (4.1) it yields
 (4.2)
(4.2)
Therefore, we have

Similarly, we get

On the other hand, for  and using Lemma 10 and (4.1) we obtain
and using Lemma 10 and (4.1) we obtain
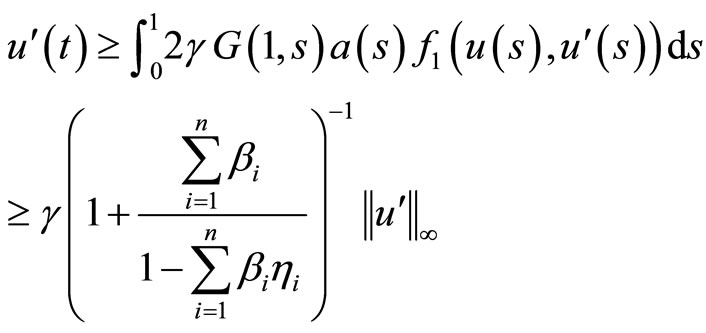 (4.3)
(4.3)
Therefore,
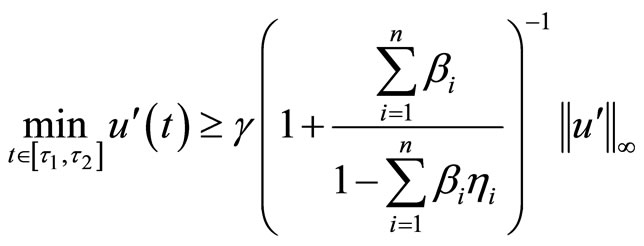
Finally, regrouping (4.2) and (4.3) we have
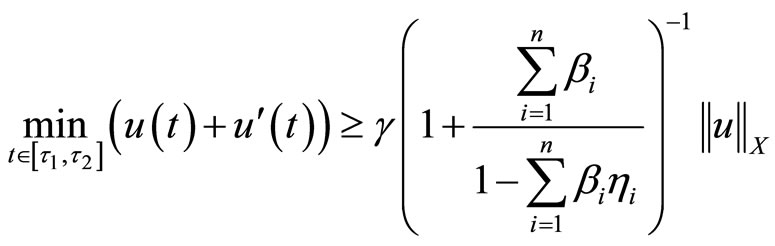
Definition 11 Let use introduce the following sets

K is a non-empty closed and convex subset of X.
Lemma 12 (See [5]) The operator T is completely continuous and satisfies 
To establish the existence of positive solutions of (BVP1), we will use the following Guo-Krasnosel’skii fixed point theorem [13].
Theorem 13 Let E be a Banach space and let  be a cone. Assume that
be a cone. Assume that ,
,  are open subsets of E with
are open subsets of E with 
 and let
and let

be a completely continuous operator. In addition suppose either 1) 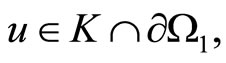
 and
and 
 or 2)
or 2) 
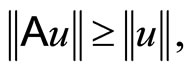 and
and 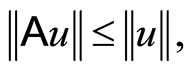

holds. Then  has a fixed point in
has a fixed point in 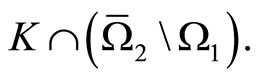
The main result of this section is the following Theorem 14 Let (Q1) and (Q2) hold, 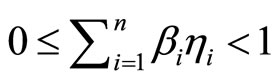 and assume that
and assume that
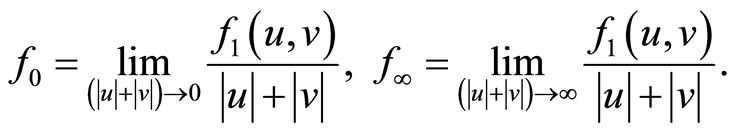
Then the problem (BVP1) has at least one positive solution in the case 1)  and
and 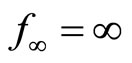
 or 2)
or 2) 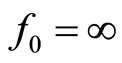 and
and 

Proof We prove the superlinear case. Since 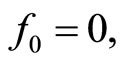 then for any
then for any 
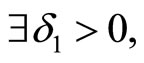 such that
such that  for
for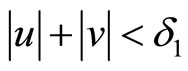 . Let
. Let 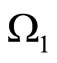 be an open set in X defined by
be an open set in X defined by

then, for any 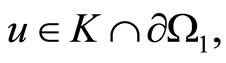 it yields
it yields
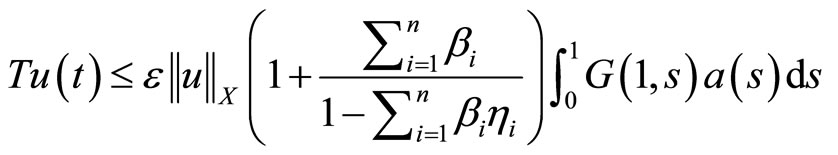
Therefore
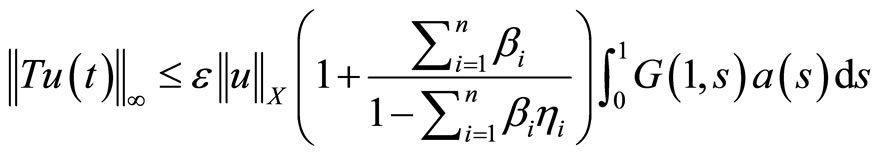
So
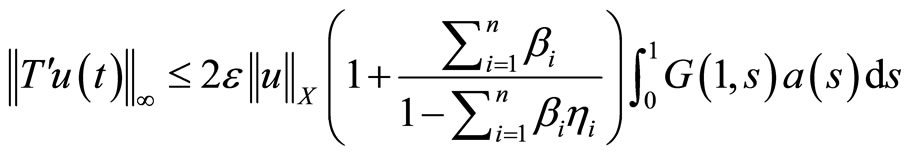
If we choose

then it yields
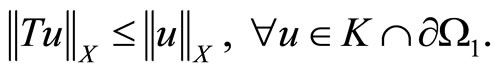
Now from  we have
we have 
 such that
such that  for
for . Let
. Let
 Denote by
Denote by  the open set
the open set 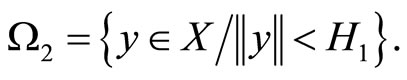
If 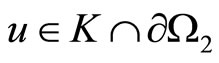 then
then

then 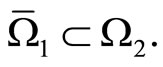 Let
Let  then
then
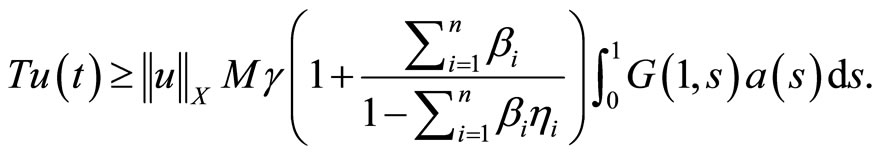
And
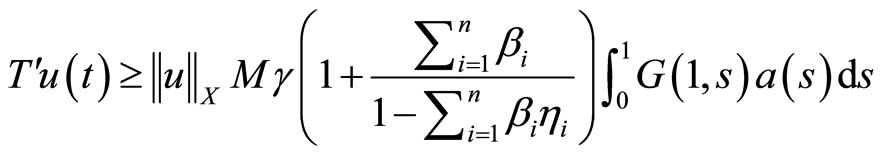
Choosing
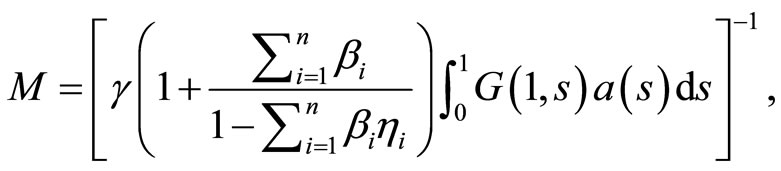
we get 
 By the first part of Theorem13, T has at least one fixed point in
By the first part of Theorem13, T has at least one fixed point in  such that
such that  This completes the superlinear case of the theorem 14. Proceeding as above we proof the sublinear case. This achieves the proof of Theorem 14.
This completes the superlinear case of the theorem 14. Proceeding as above we proof the sublinear case. This achieves the proof of Theorem 14.
Example 15 Consider the following boundary value problem
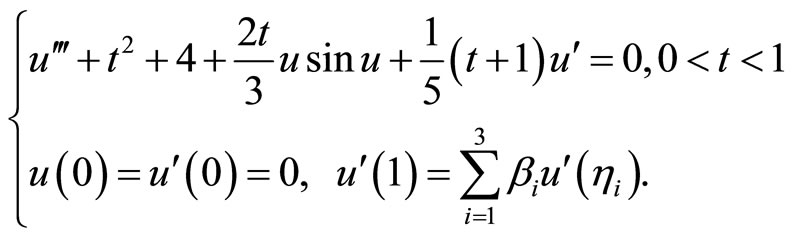 (E1)
(E1)
Set 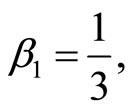
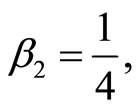
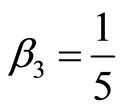 and
and 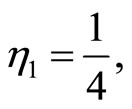
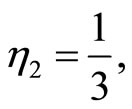
 where
where 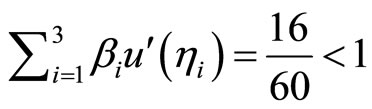 and and
and and  . One can choose
. One can choose
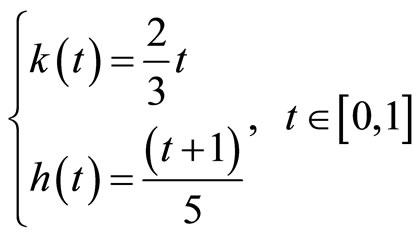
It is easy to prove that 
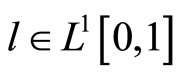 are nonnegative functions, and
are nonnegative functions, and

Hence, by Theorem 6, the boundary value problem (E1) has a unique solution in X.
2) Now if we estimate  as
as
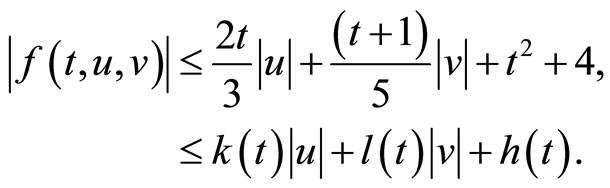
then one can choose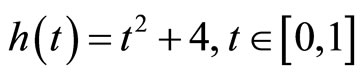 . So
. So 
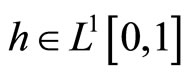 are nonnegative functions. Hence, by Theorem 8, the boundary value problem (E1) has at least one nontrivial solution,
are nonnegative functions. Hence, by Theorem 8, the boundary value problem (E1) has at least one nontrivial solution, 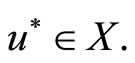
Example 16 Consider the following boundary value problem
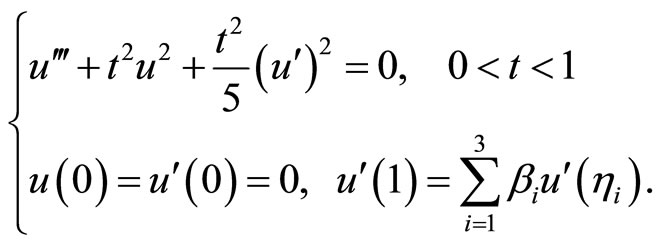 (E2)
(E2)
where, 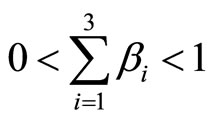
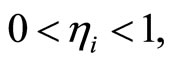 and
and

Then 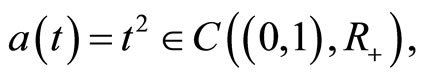
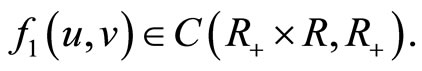 We put,
We put, 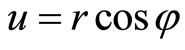 and
and 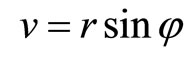 , when
, when 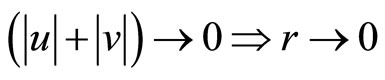 and when
and when 
Then
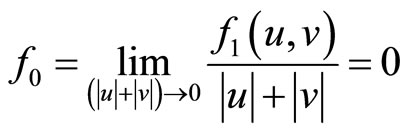
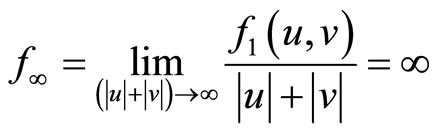
By theorem 13 1) the BVP (E2) has at least one positive solution.
REFERENCES
- D. Anderson and R. Avery, “Multiple Positive Solutions to Third-Order Discrete Focal Boundary Value Problem,” Acta Mathematicae Applicatae Sinica, Vol. 19, No. 1, 2003, pp. 117-122. doi:10.1007/s10255-003-0087-1
- D. R. Anderson, “Green’s Function for a Third-Order Generalized Right Focal Problem,” Journal of Mathematical Analysis and Applications, Vol. 288, No. 1, 2003, pp. 1-14. doi:10.1016/S0022-247X(03)00132-X
- A. Guezane-Lakoud and L. Zenkoufi, “Positive Solution of a Three-Point Nonlinear Boundary Value Problem for Second Order Differential Equations,” International Journal of Applied Mathematics and Statistics, Vol. 20, 2011, pp. 38-46.
- A. Guezane-Lakoud and S. Kelaiaia, “Solvability of a Three-Point Nonlinear Noundary-Value Problem,” Electronic Journal of Differential Equations, Vol. 2010, No. 139, 2010, pp. 1-9.
- A. Guezane-Lakoud, S. Kelaiaia and A. M. Eid, “A Positive Solution for a Non-Local Boundary Value Problem,” International Journal of Open Problems in Computer Science and Mathematics, Vol. 4, No. 1, 2011, pp. 36-43.
- J. R. Graef and B. Yang, “Existence and Nonexistence of Positive Solutions of a Nonlinear Third Order Boundary Value Problem,” Electronic Journal of Qualitative Theory of Differential Equations, No. 9, 2008, pp. 1-13.
- J. R. Graef and B. Yang, “Positive Solutions of a Nonlinear Third Order Eigenvalue Problem,” Dynamic Systems & Applications, Vol. 15, 2006, pp. 97-110.
- S. Li, “Positive Solutions of Nonlinear Singular ThirdOrder Two-Point Boundary Value Problem,” Journal of Mathematical Analysis and Applications, Vol. 323, No. 1, 2006, pp. 413-425. doi:10.1016/j.jmaa.2005.10.037
- B. Hopkins and N. Kosmatov, “Third-Order Boundary Value Problems with Sign-Changing Solutions,” Nonlinear Analysis, Vol. 67, No. 1, 2007, pp. 126-137. doi:10.1016/j.na.2006.05.003
- L. J. Guo, J. P. Sun and Y. H. Zhao, “Existence of Positive Solutions for Nonlinear Third-Order Three-Point Boundary Value Problem,” Nonlinear Analysis, Vol. 68, No. 10, 2008, pp. 3151-3158. doi:10.1016/j.na.2007.03.008
- Y. Sun, “Positive Solutions of Singular Third-Order ThreePoint Boundary Value Problem,” Journal of Mathematical Analysis and Applications, Vol. 306, No. 2, 2005, pp. 589-603. doi:10.1016/j.jmaa.2004.10.029
- K. Deimling, “Nonlinear Functional Analysis,” Springer, Berlin, 1985. doi:10.1007/978-3-662-00547-7
- D. Guo and V. Lakshmikantham, “Nonlinear Problems in Abstract Cones,” Academic Press, San Diego, 1988.

