ABSTRACT

A ring R is called right principally quasi-Baer (simply, right p.q.-Baer) if the right annihilator of every principal right ideal of R is generated by an idempotent. For a ring R, let G be a finite group of ring automorphisms of R. We denote the fixed ring of R under G by R^G. In this work, we investigated the right p.q.-Baer property of fixed rings under finite group action. Assume that R is a semiprime ring with a finite group G of X-outer ring automorphisms of R. Then we show that: 1) If R is G-p.q.-Baer, then R^G is p.q.-Baer; 2) If R is p.q.-Baer, then R^G are p.q.-Baer.

1. Introduction

Throughout this paper all rings are associative with identity. Recall from [1] that a ring R is called right principally quasi-Baer (simply, right p.q.-Baer) if the right annihilator of every principal right ideal of R is generated, as a right ideal, by an idempotent of R. A left principally quasi-Baer (simply, left p.q.-Baer) ring is defined similarly. Right p.q.-Baer rings have been initially studied in [1]. For more details on (right) p.q.-Baer rings, see [1-6].

Recall from [7] (see also [8]) that a ring R is called quasi-Baer if the right annihilator of every right ideal is generated, as a right ideal, by an idempotent of R. A ring R is called biregular if for each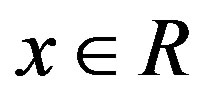, 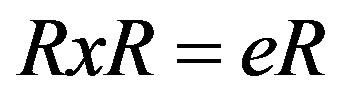for some central idempotent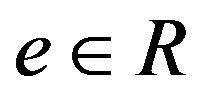. We note that the class of right p.q.-Baer rings is a generalization of the classes of quasi-Baer rings and biregular rings.

For a ring R, we use 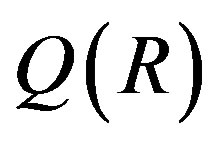to denote a fixed maximal right ring of quotients of R. According to [9] an idempotentof a ring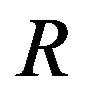is called left (resp., right) semicentral if 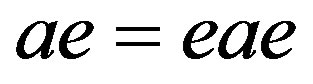(resp.,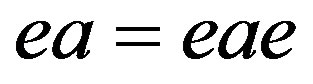) for all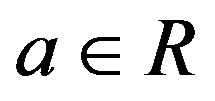. Equivalently, an idempotentis left (resp., right) semicentral if and only if eR (resp.,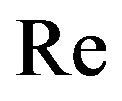) is a two-sided ideal of R. For a ring R, we let 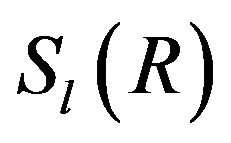(resp.,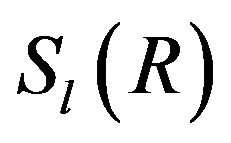) denote the set of all left (resp., right) semicentral idempotents. An idempotentof a ring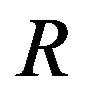is called semicentral reduced if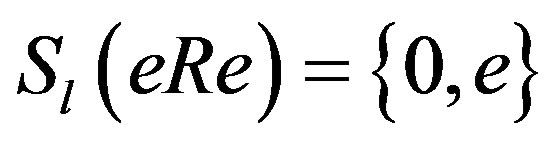. Recall from [2] that a ring R is called semicentral reduced if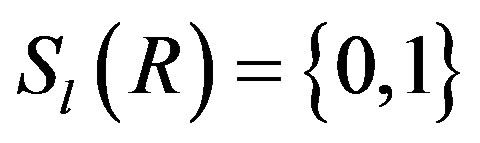, i.e., 1 is a semicentral reduced idempotent of R.

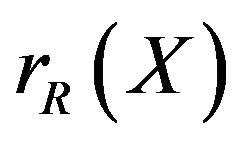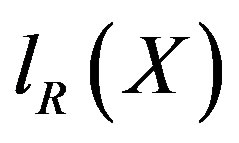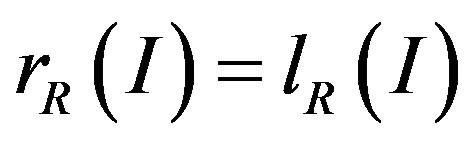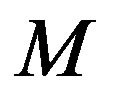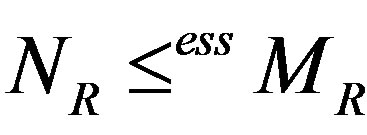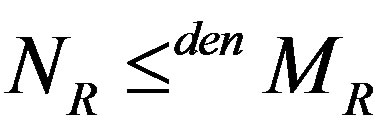For a nonempty subset X of a ring R, we use and to denote the right annihilator and the left annihilator of X in R, respectively. If R is a semiprime ring and I is a two-sided ideal of R, then. For a right R-moduleand a submodule N of M, we use and to denote that 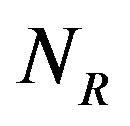is essential in 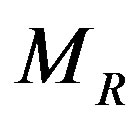and 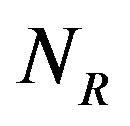is dense in, respectively.

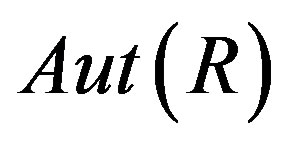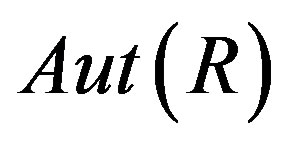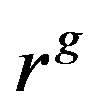For a ring R, we let denote the group of ring automorphisms of R. Let be a subgroup of. For and, we let denote the image of r under g. We use 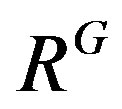to denote the fixed ring of R under G, that is.

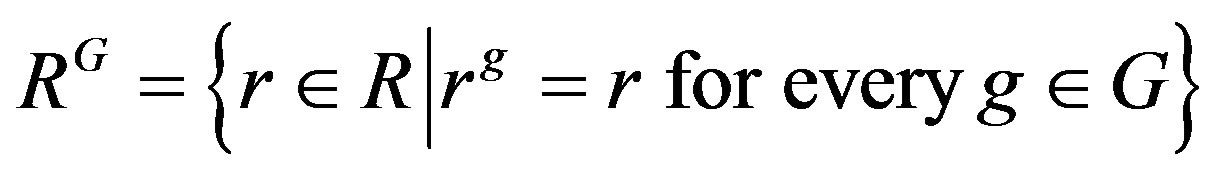We begin with the following example.

2. Preliminary

Example 2.1. There exist a ring R and a finite group G of ring automorphisms of R such that R is right p.q.-Baer but R^G is not right p.q.-Baer. Let 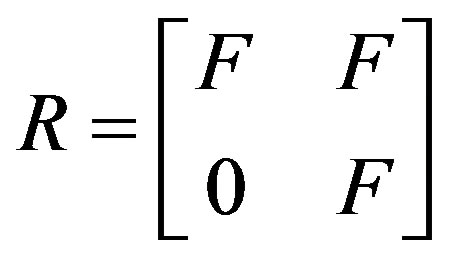with a field F of characteristic 2. Then R is right p.q.-Baer. Define 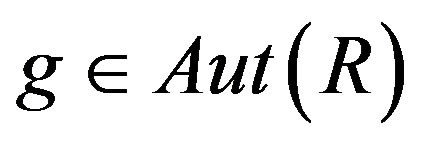by

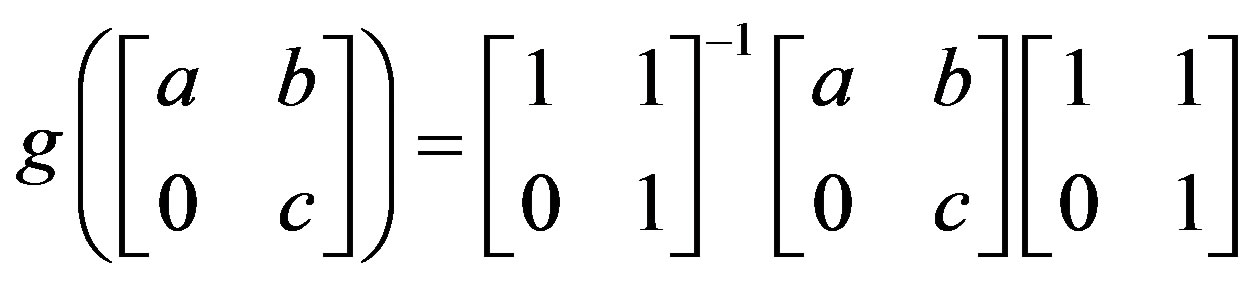
Then 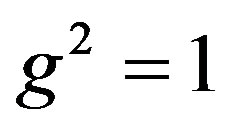since the characteristic of F is 2.

Now we show that R^G is not right p.q.-Baer. The fixed ring under G is

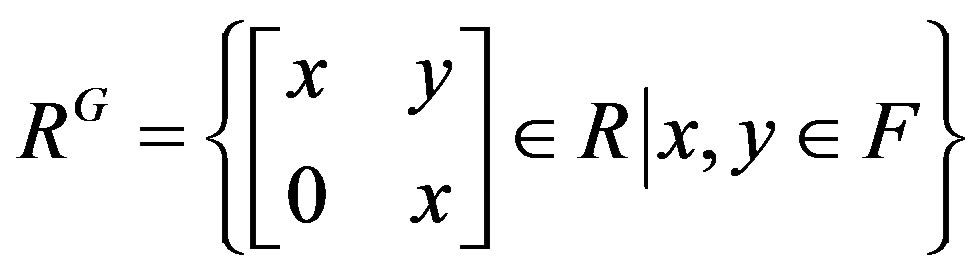
By computation we see that the idempotents of R^G are only 0 and 1, thus R^G is semicentral reduced. So if R^G is right p.q.-Baer, then R^G is a prime ring by [2, Lemma 4.2], a contradiction. Thus R^G is not right p.q.-Baer.Also we can see that R^G is not left p.q.-Baer.

Definition 2.2. Let R be a semiprime ring. For 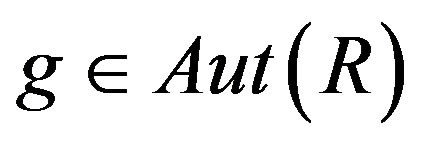, let

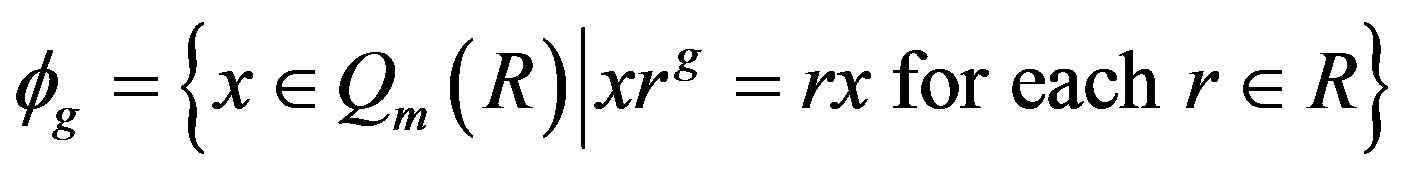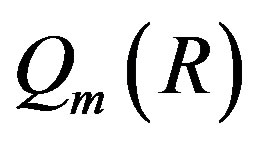where is the Martindale right ring of quotients of R (see [10] for more on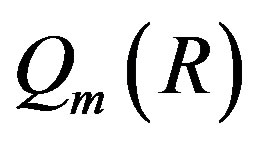). We say that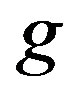is Xouter if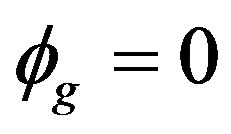. A subgroup G of 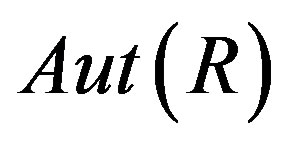is called Xouter on R if every 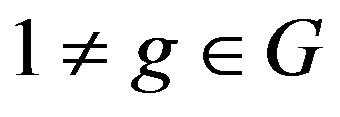is X-outer. Assume that R is a semiprime ring, then for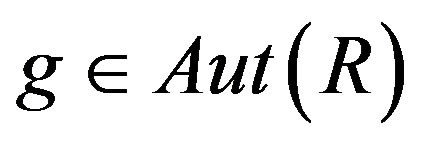, let

.

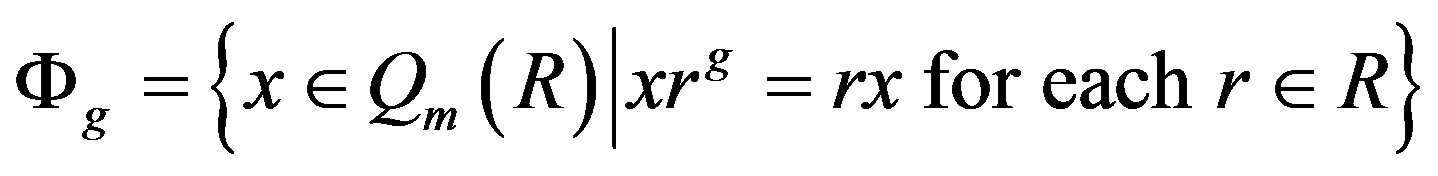For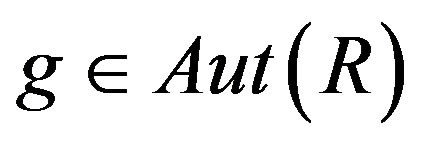, we claim that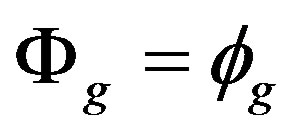. Obviously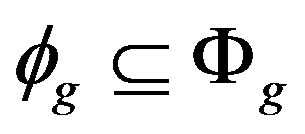. Conversely, if 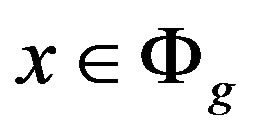then. The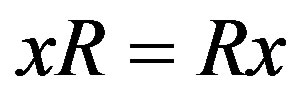re exists 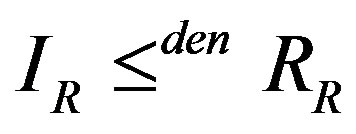such that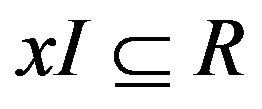. Therefore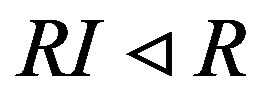, , 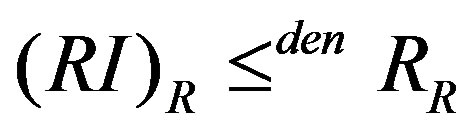and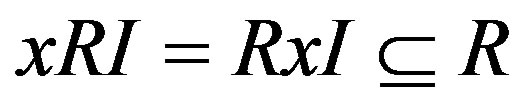. Thus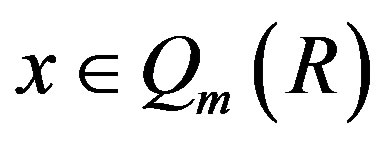, hence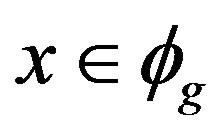. Therefore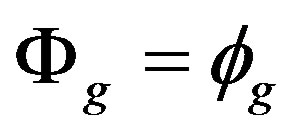. So if G is X-outer on R, then G can be considered as a group of ring automorph-ismms of 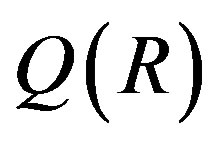and G is X-outer on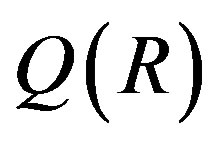. For more details for X-outer ring automorphisms of a ring, etc., see [10, p. 396] and [11].

We say that a ring R has no nonzero n-torsion (n is a positive integer) if with 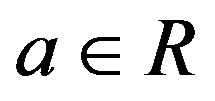implies.

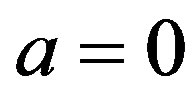Lemma 2.3. [12,13]

Let R be a semiprime ring and G a group of ring automorphisms of R. If 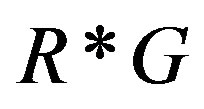is semiprime, then 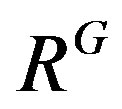is semiprime.

For a ring R, we use 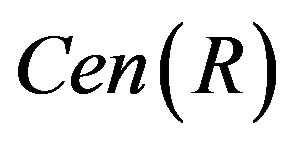to denote the center of R.

Lemma 2.4. For a semiprime ring R, let G be a group of X-outer ring automorphisms of R.

Then.

Proof.

Let 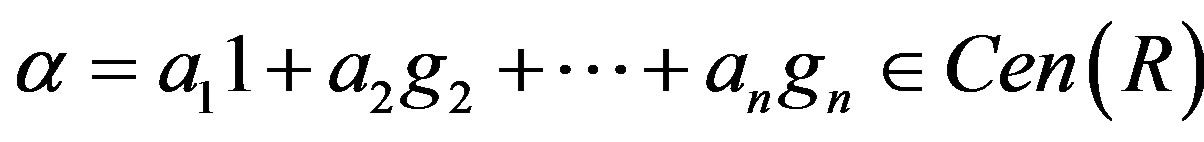with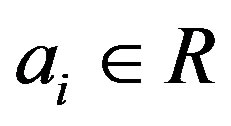, 1 the identity of G, and.

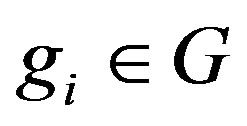The 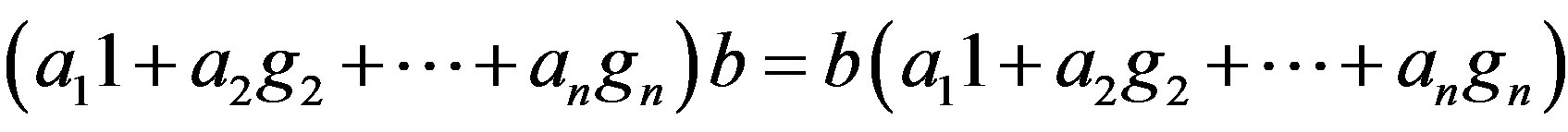for all. So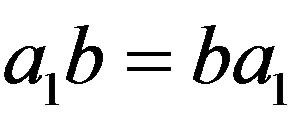, , 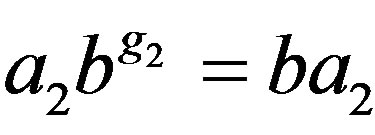···, 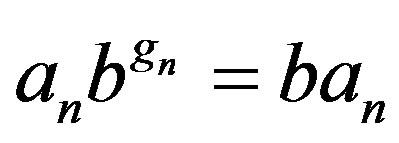for all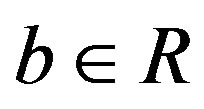. Since G is X-outer, it follows that

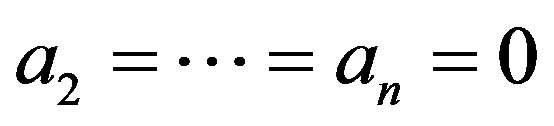. Hence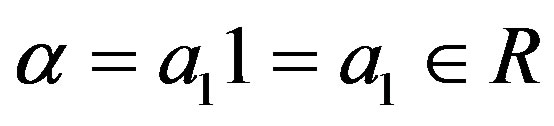. Also since 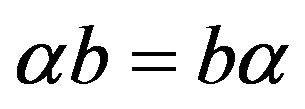for all, we have that.

Note that for all, 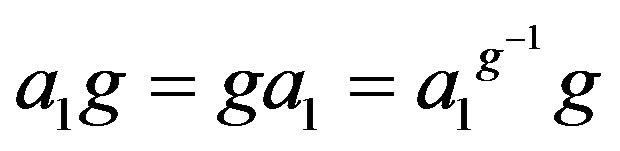implies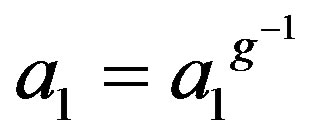. So. Thus

.

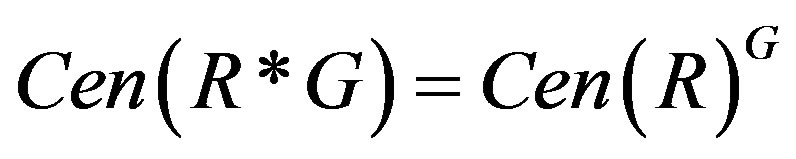Conversely, 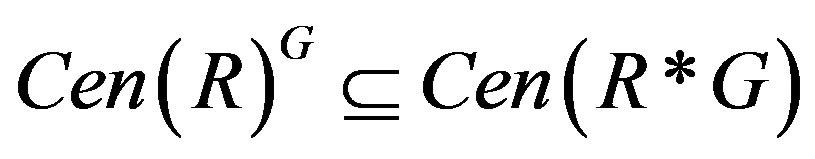is clear.

Therefore.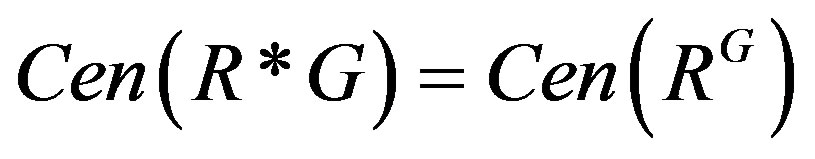

Lemma 2.5. [14] Assume that R is a semiprime ring and G is a finite group of X-outer ring automorphisms of R. Then.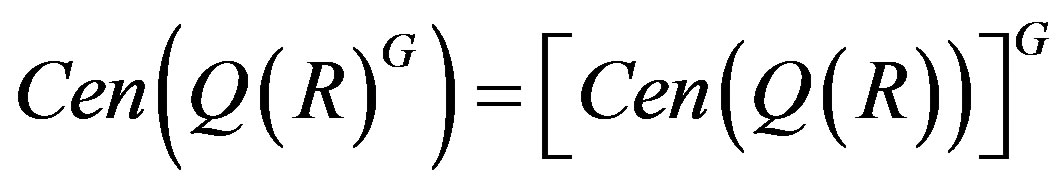

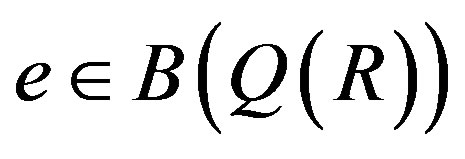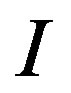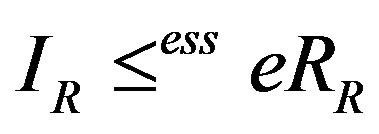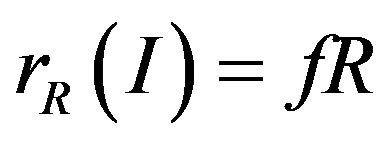Lemma 2.6. Assume that R is a semiprime ring and. Letbe a two-sided ideal of R such that and with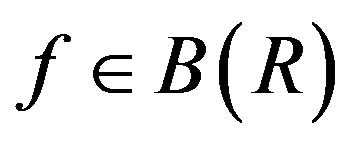. Then.

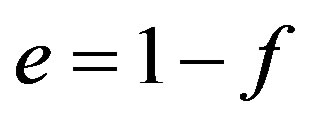Proof. Since R is semiprime,

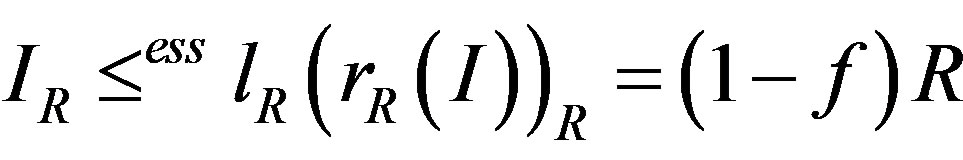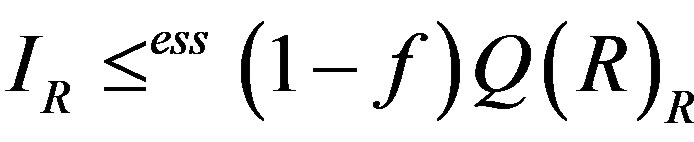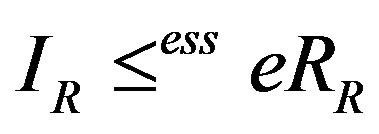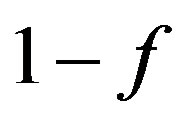. Thus. As,. We note that e and are in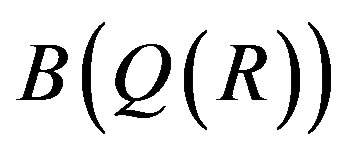. So we have that.

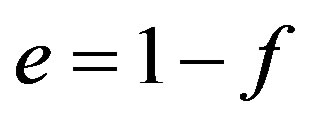Lemma 2.7. [15] Let R be a semiprime ring with a finite group G of X-outer ring automorphisms of R.

1) For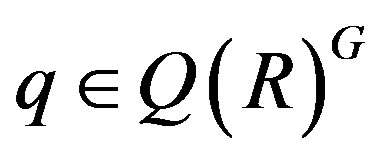, let I be a dense right ideal I of R^G such that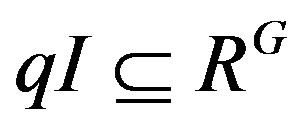. Then IR is a dense right ideal of R and the map defined by

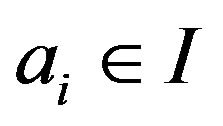, with and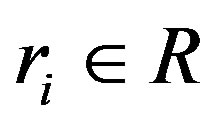, is a right R-homomorphism. Moreover.

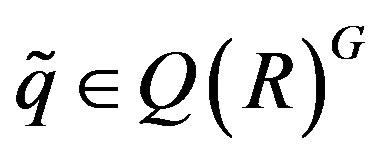2) The map 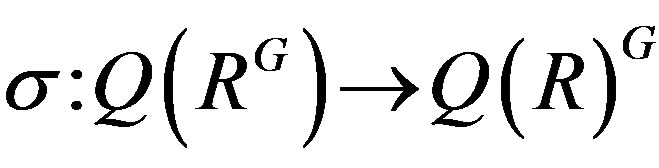defined by 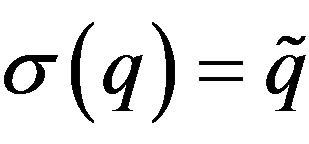is a ring isomorphism.

3) Let 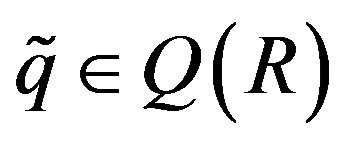and K a dense right ideal of R such that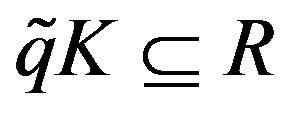. Then 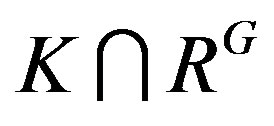is a dense right ideal of R^G

and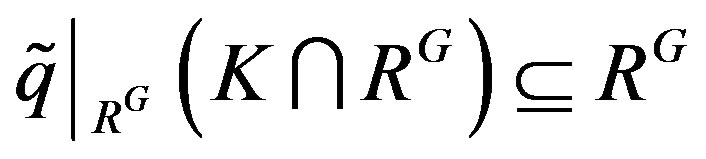, where 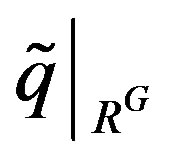is the restriction of 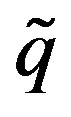to R^G. Thus.

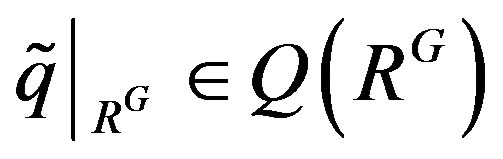For a ring R with a group G of ring automorphism of R, we say that a right ideal I of R is G-invariant if 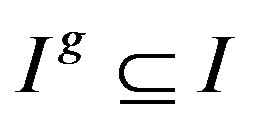for every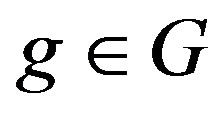, where.

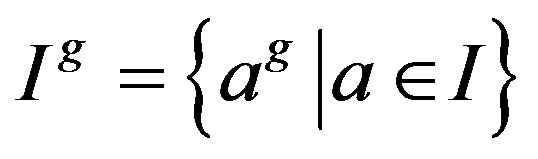Proposition 2.8. [1] Let R be a semiprime ring. Then the followings are equivalent.

1) R is right p.q.-Baer;

2) Every principal two-sided ideal of R is right essential in a ring direct summand of R;

3) Every finitely generated two-sided ideal of R is right essential in a ring direct summand of R;

4) Every principal two-sided ideal of R that is closed as a right ideal is a direct summand of R;

5) For every principal two-sided ideal I of R, 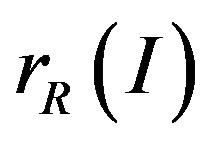is right essential in a direct summand of R;

6) R is left p.q.-Baer.

For a ring R with a group G of ring automorphisms of R, we say that a right ideal I of R is G-invariant if 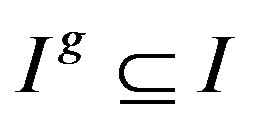for every, where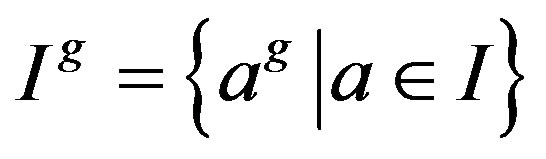. Assume that R is a semiprime ring with a group G of ring automorphisms of R. We say that R is G-p.q.-Baer if the right annihilator of every finitely generated G-invariant two-sided ideal is generated by an idempotent, as a right ideal. By Proposition 8, if a ring R is semiprime p.q.- Baer with a group G of ring automorphisms of R, then R is G-p.q.-Baer.

A ring R is called right Rickart if the right annihilator of each element is generated by an idempotent of R. A left Rickart ring is defined similarly. A ring R is called Rickart if R is both right and left Rickart. A ring R is said to be reduced if R has no nonzero nilpotent element. We note that reduced Rickart rings are p.q.-Baer rings.

We put

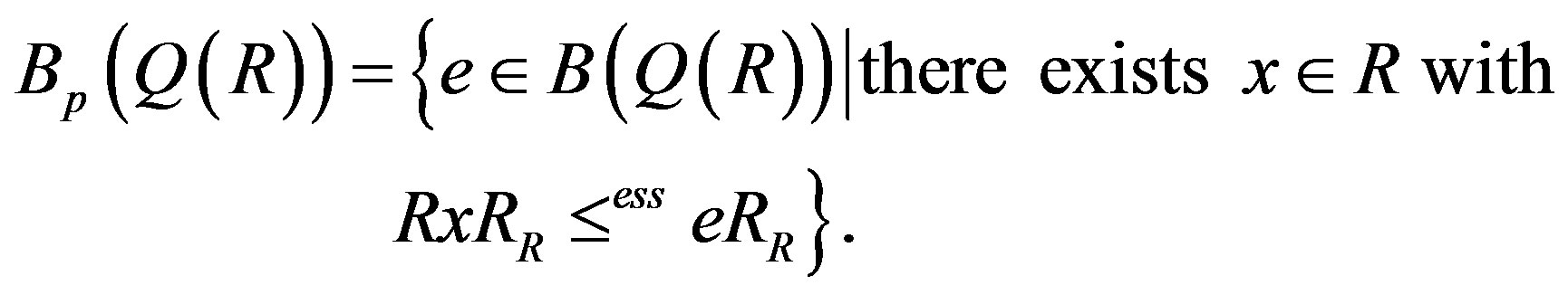
Let 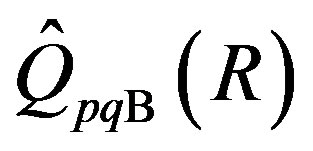be the subring of 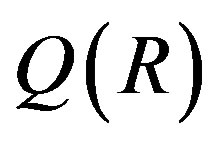generated by R and.

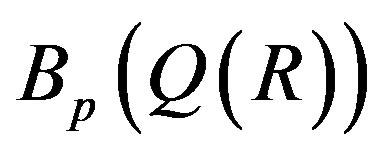Lemma 2.9. [16] Assume that R is a semiprime ring. Then:

1) The ring 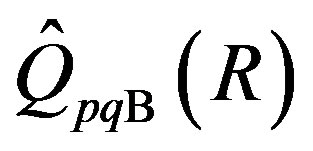is the smallest right ring of quotients ofwhich is p.q.-Baer;

2) R is p.q.-Baer if and only if.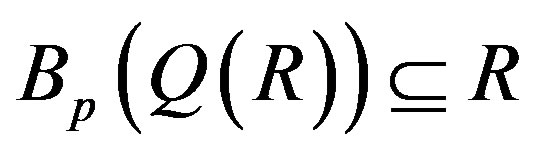

With these preparations, in spite of Example 1, we have the following result for p.q.-Baer property of R^G on a semiprime ring R for the case when G is finite and X-outer.

3. Main Results

Theorem 3.1. Let R be a semiprime ring with a finite group G of X-outer ring automorphisms of R. Then:

1) If R is G-p.q.-Baer, then R^G is p.q.-Baer.

2) If R is reduced G-p.q.-Baer, then R^G is Rickart.

Proof. 1) Assume that R is G-p.q.-Baer. To show that R^G is p.q.-Baer, it is enough to see that 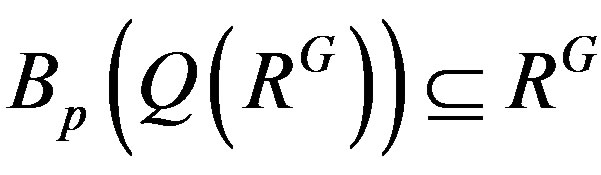by Lemma 9 since R^G is semiprime from Lemma 3. Let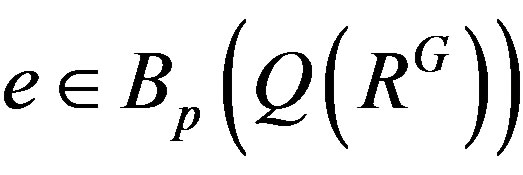. Then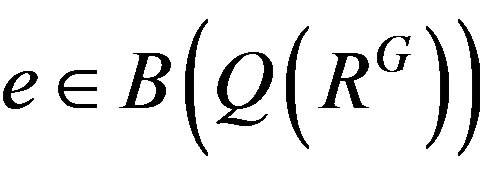, so 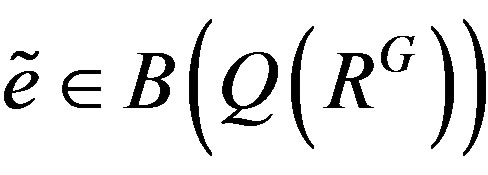by Lemma 7. From Lemma 9, there exists 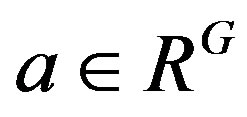such that because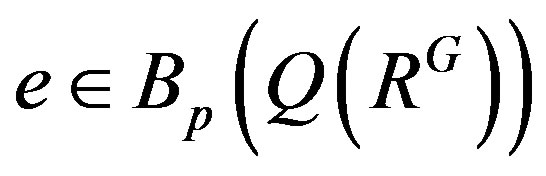. Note that.

We show that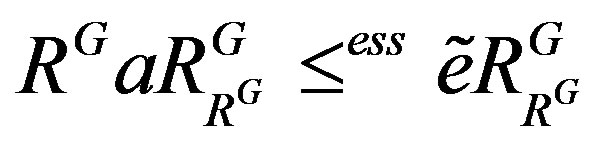. To see this, say

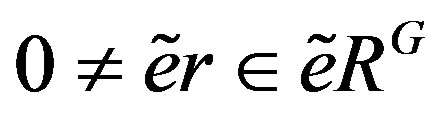with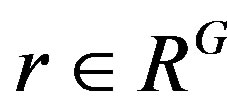. Then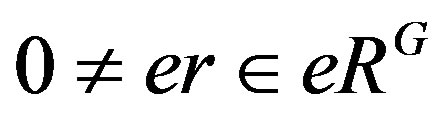. So there exists 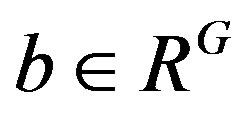such that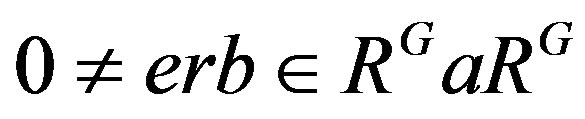. Hence .

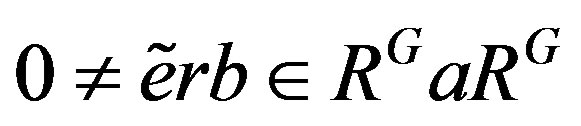Observe that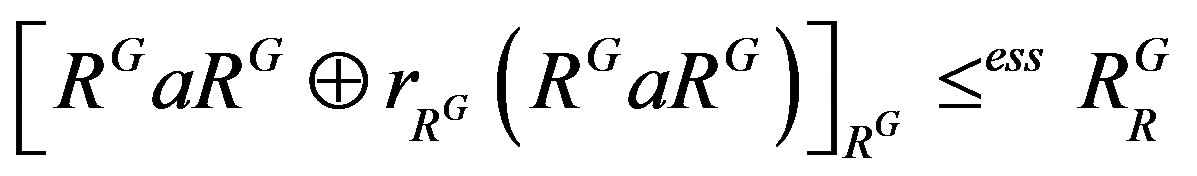, as R^G is semiprime from Lemma 3. So 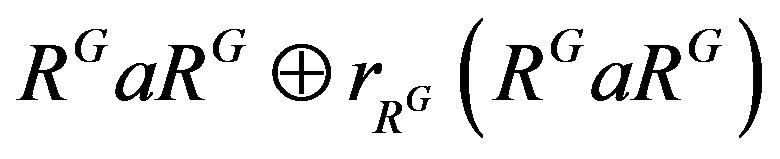is a dense right ideal of R^G since R^G is semiprime. By Lemma 7, 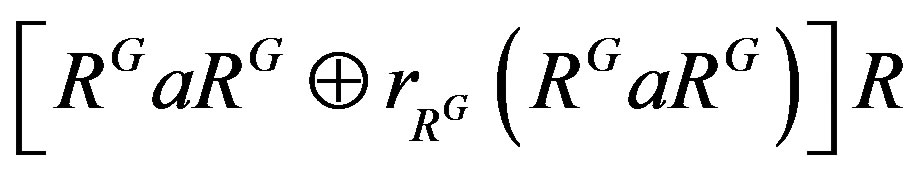is a dense right ideal of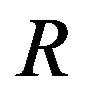. So it is essential in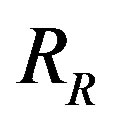. Hence

.

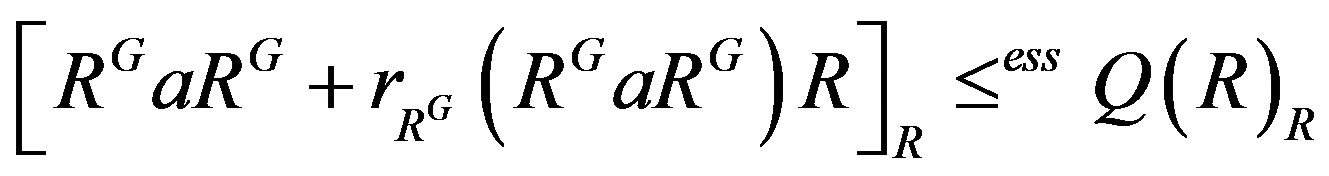We claim that.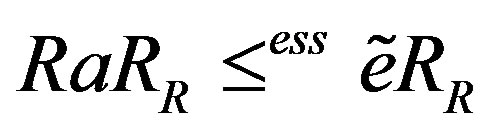

First note that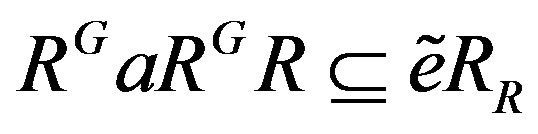. For the claim, it is enough to show that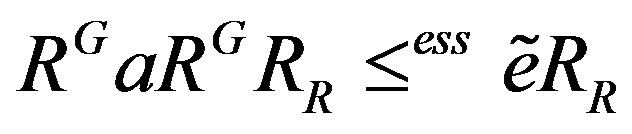. Take

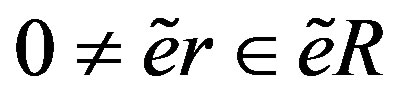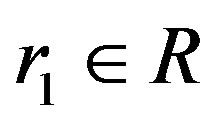with. Then there existssuch that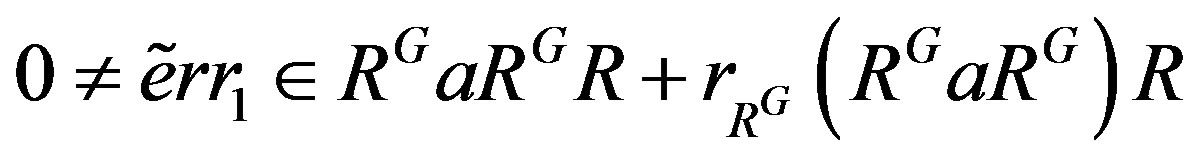. Say

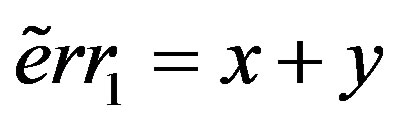, where 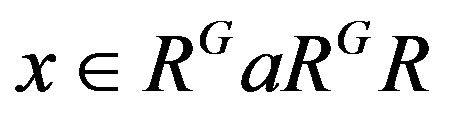and .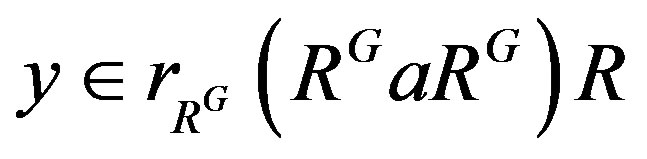

Then.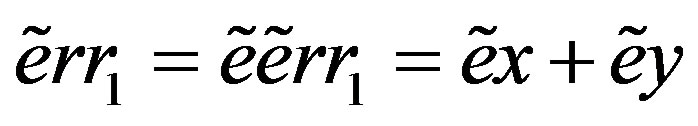

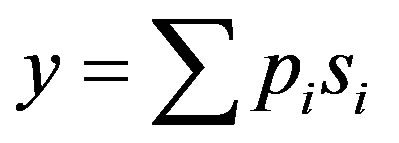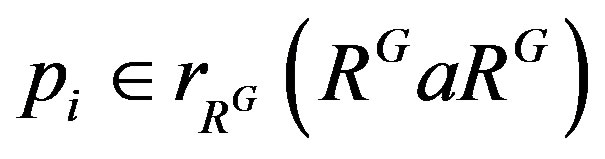Put with and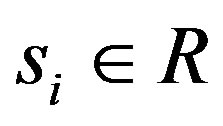. Then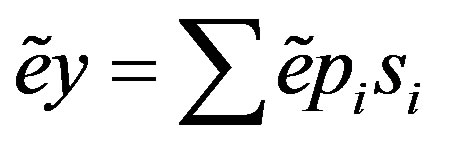. In this case, 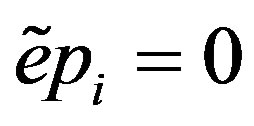for all i. To see this, assume on the contrary that there is 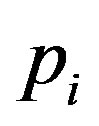such that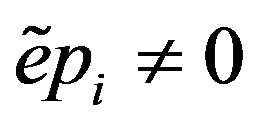. Note that.

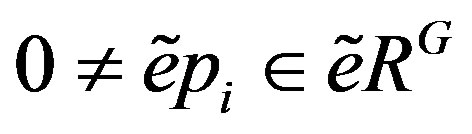Thus there exists 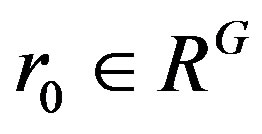such that 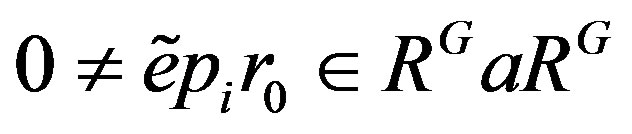because.

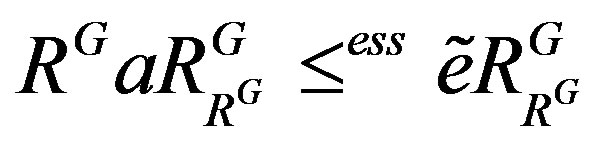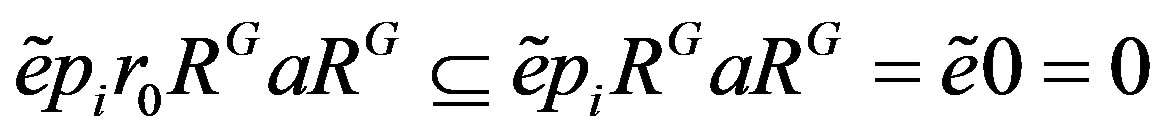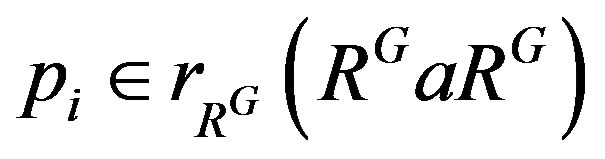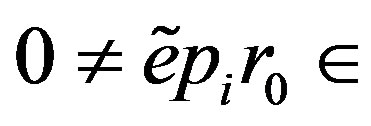
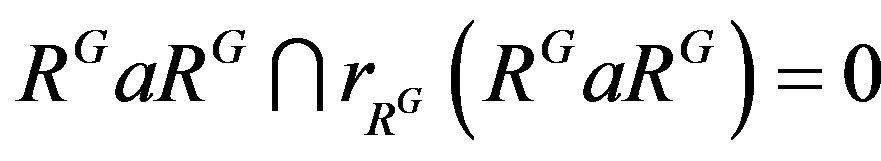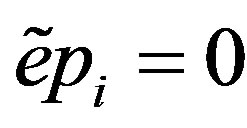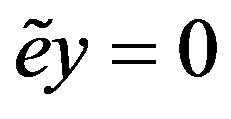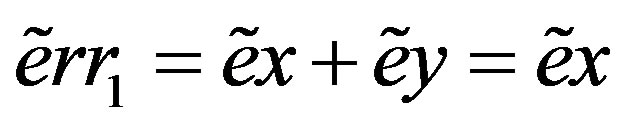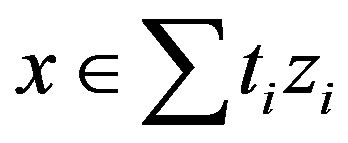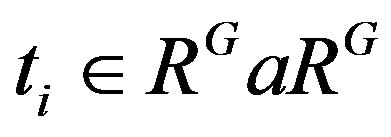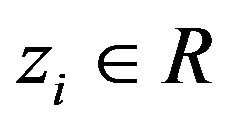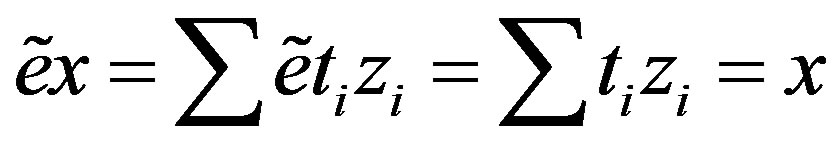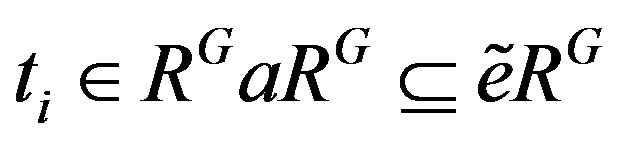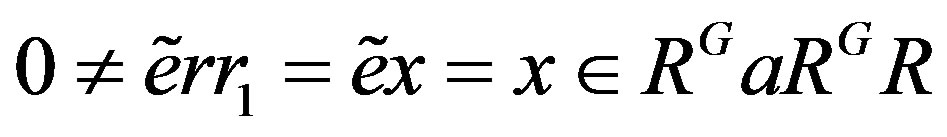Also because . Therefore we have that , a contradiction. Thus for all i, so. Hence. Now since, write with and. Then because. So. Therefore. Note that

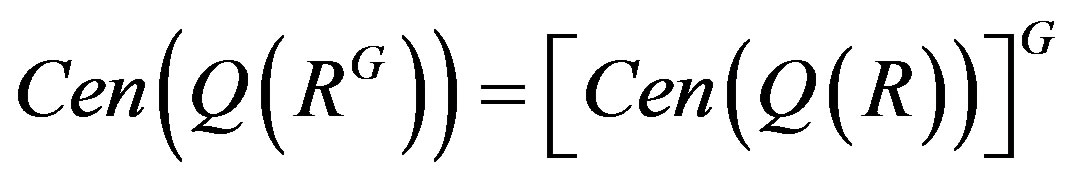by Lemma 5. Therefore

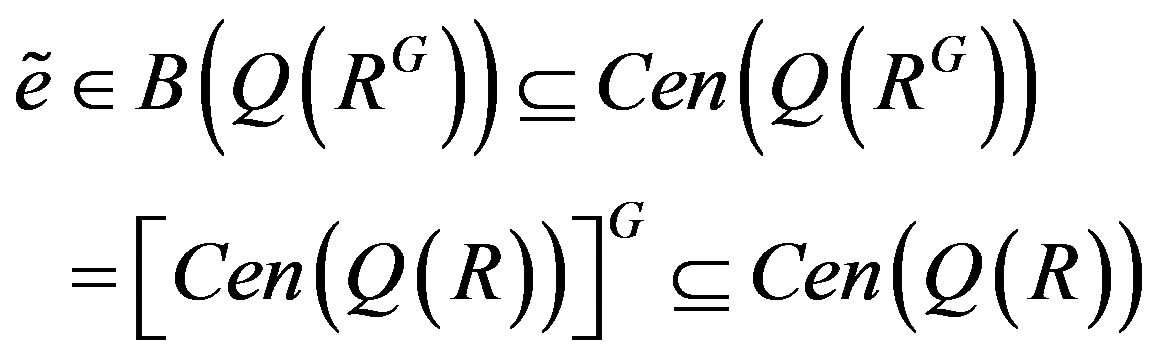
Hence.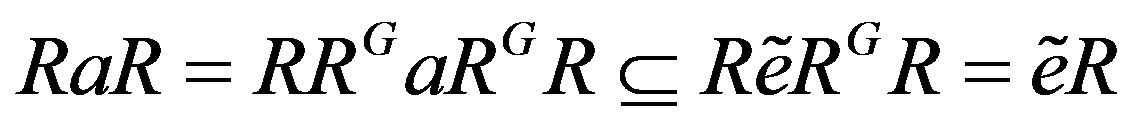

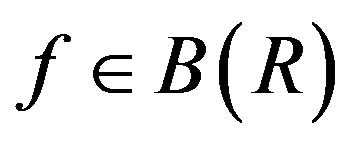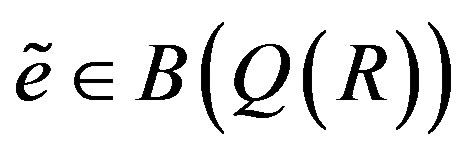As RaR is a G-invariant two-sided ideal of R and R is R-p.q.-Baer, there is such that . From [9],. As and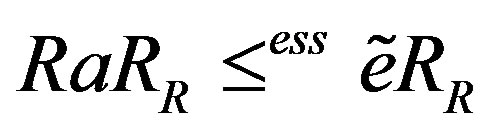, it follows that 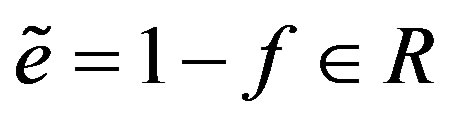by Lemma 6, so.

Therefore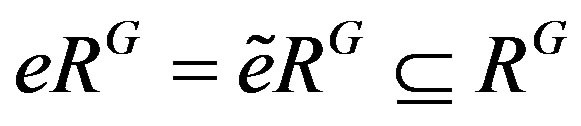, and thus.

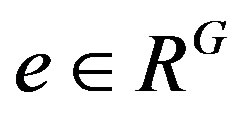So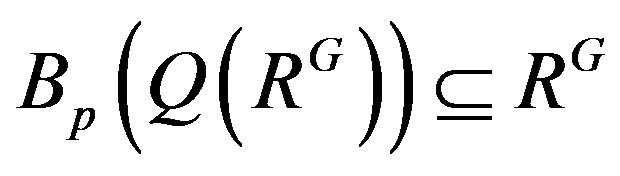, and hence R^G is p.q.-Baer by Lemma 9.

2) We recall that a reduced p.q.-Baer ring is Rickart. Thus if R is reduced G-p.q.-Baer, then R^G is Rickart from 1).

4. Conclusion

In [14], the quasi-Baer property of fixed rings under finite group actions on a semiprime ring and their applications to C^*-algebras have been studied (see also [17,18]). Motivated by investigations in [14], in this paper we investigate the right p.q.-Baer property of fixed rings under finite group actions on a given semiprime ring. Assume that R is a semiprime ring with a finite group G of X-outer ring automorphisms of R. Then we show that if R is G-p.q.-Baer, then R^G is p.q.-Baer. Thus if R is a semiprime p.q.-Baer ring with finite group G of X-outer ring automorphisms of R, then R^G is p.q.-Baer.

REFERENCES

NOTES

^*Corresponding author.