ABSTRACT

The aim of this paper is to provide an advanced analysis of the shear stresses exerted on vessel walls by the flowing blood, when a limb or the whole body, or a vessel prosthesis, a scaffold… is placed in an external static magnetic field B₀. This type of situation could occur in several biomedical applications, such as magnetic resonance imaging (MRI), magnetic drug transport and targeting, tissue engineering, mechanotransduction studies… Since blood is a conducting fluid, its charged particles are deviated by the Hall effect, and the equations of motion include the Lorentz force. Consequently, the velocity profile is no longer axisymmetric, and the velocity gradients at the wall vary all around the vessel. To illustrate this idea, we expand the exact solution given by Gold (1962) for the stationary flow of blood in a rigid vessel with an insulating wall in the presence of an external static magnetic field: the analytical expressions for the velocity gradients are provided and evaluated near the wall. We demonstrate that the derivative of the longitudinal velocity with respect to the radial coordinate is preponderant when compared to the θ-derivative, and that elevated values of B₀ would be required to induce some noteworthy influence on the shear stresses at the vessel wall.

Keywords:

Magnetohydrodynamic Flow of Blood, Wall Shear Stresses, Magnetic Fields in Biomedical Applications

1. Introduction

The aim of this paper is to provide an advanced analysis of the shear stresses exerted on vessel walls by the flowing blood, when a limb or the whole body, or a vessel prosthesis, a scaffold… is placed in an external static magnetic field B₀.

As explained in [1] [2] , such a situation may occur in several biomedical applications:

・ magnetic resonance imaging (MRI) [3] [4] [5] [6] [7] .

・ magnetic drug transport and targeting [8] - [13] : magnetic particles containing or coated with therapeutics are injected into the bloodstream and concentrated to sites of disease under the influence of the magnetic field.

・ tissue engineering [14] [15] [16] [17] [18] : magneto-responsive particles are guided by the magnetic force in order to enhance cellular invasion in the scaffolds.

・ mechanotransduction studies and applications for regenerative medicine strategies (for example, with stem cells) [19] [20] .

This analysis would also provide a risk assessment for the vessel wall (plaque rupture in case of atherosclerotic lesion [21] , severity of some aneurysms [22] , ...) or for other cells attachment and/or transmigration (white blood cells, tumor cells, cells seeded in vascular substitutes [23] , ...).

Since blood is a conducting fluid, its charged particles are deviated by the Hall effect thus inducing electrical currents and voltages along the vessel walls and in the neighboring tissues. The equations of motion include the Lorentz force j^B, where j is the electric current density. Consequently, the velocity profile is no longer axisymmetric, even in a cylindrical vessel; and the velocity gradients at the wall vary all around the vessel.

To illustrate this idea, we chose to expand the exact solution given by Gold [24] for the stationary flow of blood in a rigid vessel with an insulating wall in the presence of an external static magnetic field. This analysis completes previous ones [25] [26] . In the present paper, we provide the analytical expressions for the velocity gradients and evaluate them near the vessel wall.

2. Unidirectional Steady Blood Flow in a Rigid Cylindrical Vessel with Insulating Walls

As explained by Gold [24] and by Abi-Abdallah et al. [25] , the Navier-Stokes equations including the Lorentz force (Equation (1)), coupled with the induction equation (Equation (2)) govern the flow of a conducting, incompressible, Newtonian fluid in an externally applied static magnetic field B₀.

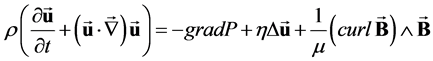(1)

(2)

where u and P are the fluid velocity and pressure; μ is the magnetic permeability; ρ, η and σ are the fluid density, viscosity and conductivity and the electric current density is expressed as $j=\left(curlB\right)/\mu$ .

Gold [24] then considered the case of a unidirectional steady blood flow in a rigid circular vessel with insulating walls and radius R (Figure 1, [25] [26] ). The

velocity and magnetic field are defined in the cylindrical frame (e_r, e_θ, e_z) as:

(3)

The induced magnetic field, B_I, is parallel to the flow and guarantees $\text{div}B=0$ . The continuity equation $\text{div}u=0$ is also satisfied.

The longitudinal projections (along e_z) of Equations ((1) and (2)) in the cylindrical frame are thus:

(4)

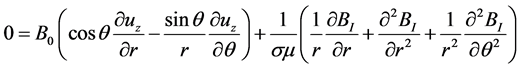(5)

The boundary conditions associated with this problem are:

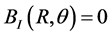because the wall is non-conducting (6a)

and $u_z\left(R,\theta \right)=0$ , because of the no-slip condition at the rigid wall (6b)

The equation system (4) to (6) is expressed in a non-dimensional form, using the following definitions:

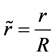$\tilde{U}=\frac{u_z}{u_0}$ , , $\tilde{B}=\frac{B_I}{B_0}$ , and (where u₀ is some characteristic mean velocity).

The numerical values of the different quantities are taken from Abi-Abdallah et al. [25] :

$\eta =4\times {10}^{-3}\text{Pa}\cdot \text{s}$ , $u_0=0.4\text{m}/\text{s}$ , $\partial P/\partial z=-128\text{Pa}/\text{m}$ , and $R=0.01\text{m}$ ; then G equals +8.

The non-dimensional solution given by Gold [24] for Equation (4) and (5), associated with the boundary conditions (6) is:

(7)

and

$\tilde{U}\left(\tilde{r},\theta \right)=\frac{G}{2H_a}\left[E_1\left(\tilde{r},\theta \right))A_1\left(\tilde{r},\theta \right)+E_2\left(\tilde{r},\theta \right)A_2\left(\tilde{r},\theta \right)\right]$ (8)

with:

$E_1\left(\tilde{r},\theta \right)={\text{e}}^{-\frac{H_a}{2}\tilde{r}\cos \theta }$ ,

$A_1\left(\tilde{r},\theta \right)={\alpha }_0{I}_0\left(\frac{H_a}{2}\tilde{r}\right)+{\displaystyle \underset{n=1}{\overset{\infty }{\sum }}2{\alpha }_n{I}_n\left(\frac{H_a}{2}\tilde{r}\right)}\cos \left(n\theta \right)$ ,

$E_2\left(\tilde{r},\theta \right)={\text{e}}^{\frac{H_a}{2}\tilde{r}\cos \theta }$ ,

$A_2\left(\tilde{r},\theta \right)={\alpha }_0{I}_0\left(\frac{H_a}{2}\tilde{r}\right)+{\displaystyle \underset{n=1}{\overset{\infty }{\sum }}2{\left(-1\right)}^n{\alpha }_n{I}_n\left(\frac{H_a}{2}\tilde{r}\right)}\cos \left(n\theta \right)$ ,

and

${\alpha }_0=\frac{{I^{\prime }}_0\left(\frac{H_a}{2}\right)}{I_0\left(\frac{H_a}{2}\right)};{\alpha }_n=\frac{{I^{\prime }}_n\left(\frac{H_a}{2}\right)}{I_n\left(\frac{H_a}{2}\right)}$ (9)

The Hartmann number, H_a, is defined as: $H_a=B_{0}R\sqrt{\frac{\sigma }{\eta }}$ , the magnetic Reynolds number, R_em, as: $R_{em}=R{u}_0\sigma \mu$ , and the functions I_n are the n^th order modified Bessel functions of the first kind.

In order to evaluate the derivatives of the I_n functions, the following identities are used:

${I^{\prime }}_0\left(X\right)=I_1\left(X\right)\text{and}{I^{\prime }}_n\left(X\right)=I_{n-1}\left(X\right)-\frac{n}{X}{I}_n\left(X\right)$ (10).

In such flow configuration, the classical definition of the dimensional shear stresses would yield:

${\tau }_{rz}=\eta \frac{\partial u_z}{\partial r};{\tau }_{\theta z}=\frac{\eta }{r}\frac{\partial u_z}{\partial \theta }$ (11)

The corresponding non-dimensional expressions would be: ${\tilde{\tau }}_{rz}=\frac{\partial \tilde{U}}{\partial \tilde{r}};{\tilde{\tau }}_{\theta z}=\frac{1}{\tilde{r}}\frac{\partial \tilde{U}}{\partial \theta }$ , where ${\tilde{\tau }}_{rz}=\frac{{\tau }_{rz}}{{\tau }_0};{\tilde{\tau }}_{\theta z}=\frac{{\tau }_{\theta z}}{{\tau }_0}$ , and ${\tau }_0=\frac{\eta u_0}{R}$ ( ${\tau }_0=0.\text{16}\text{Pa}$ , with the numerical data of this study).

It is thus necessary to calculate the velocity gradients (from Equation (8)). This can be done as follows:

$\frac{\partial E_1}{\partial \tilde{r}}=-\frac{H_a}{2}\cos \theta {\text{e}}^{-\frac{H_a}{2}\tilde{r}\cos \theta }$ and $\frac{\partial E_2}{\partial \tilde{r}}=\frac{H_a}{2}\cos \theta {\text{e}}^{\frac{H_a}{2}\tilde{r}\cos \theta }$ (12)

$\frac{\partial A_1}{\partial \tilde{r}}=\frac{H_a}{2}\left[{\alpha }_0{I}_1\left(\frac{H_a}{2}\tilde{r}\right)+{\displaystyle \underset{n=1}{\overset{\infty }{\sum }}2{\alpha }_n\cos \left(n\theta \right)\left(I_{n-1}\left(\frac{H_a}{2}\tilde{r}\right)-\frac{2n}{H_a\tilde{r}}{I}_n\left(\frac{H_a}{2}\tilde{r}\right)\right)}\right]$ (13)

$\begin{array}{l}\frac{\partial A_2}{\partial \tilde{r}}\\ =\frac{H_a}{2}\left[{\alpha }_0{I}_1\left(\frac{H_a}{2}\tilde{r}\right)+{\displaystyle \underset{n=1}{\overset{\infty }{\sum }}2{\left(-1\right)}^n{\alpha }_n\cos \left(n\theta \right)\left(I_{n-1}\left(\frac{H_a}{2}\tilde{r}\right)-\frac{2n}{H_a\tilde{r}}{I}_n\left(\frac{H_a}{2}\tilde{r}\right)\right)}\right]\end{array}$ (14)

$\frac{\partial E_1}{\partial \theta }=\frac{H_a}{2}\tilde{r}\sin \theta {\text{e}}^{-\frac{H_a}{2}\tilde{r}\cos \theta }$ and $\frac{\partial E_2}{\partial \theta }=-\frac{H_a}{2}\tilde{r}\sin \theta {\text{e}}^{\frac{H_a}{2}\tilde{r}\cos \theta }$ (15)

$\frac{\partial A_1}{\partial \theta }=-{\displaystyle \underset{n=1}{\overset{\infty }{\sum }}2n{\alpha }_n{I}_n\left(\frac{H_a}{2}\tilde{r}\right)}\sin \left(n\theta \right)$ and $\frac{\partial A_2}{\partial \theta }={\displaystyle \underset{n=1}{\overset{\infty }{\sum }}2n{\left(-1\right)}^{n+1}{\alpha }_n{I}_n\left(\frac{H_a}{2}\tilde{r}\right)}\sin \left(n\theta \right)$ (16)

Gathering all, one obtains:

$\frac{\partial \tilde{U}}{\partial \tilde{r}}=\frac{G}{2H_a}\left[\frac{\partial E_1}{\partial \tilde{r}}{A}_1+E_1\frac{\partial A_1}{\partial \tilde{r}}+\frac{\partial E_2}{\partial \tilde{r}}{A}_2+E_2\frac{\partial A_2}{\partial \tilde{r}}\right]$ (17)

and:

$\frac{\partial \tilde{U}}{\partial \theta }=\frac{G}{2H_a}\left[\frac{\partial E_1}{\partial \theta }{A}_1+E_1\frac{\partial A_1}{\partial \theta }+\frac{\partial E_2}{\partial \theta }{A}_2+E_2\frac{\partial A_2}{\partial \theta }\right]$ (18)

3. Results

The term $\frac{\partial \tilde{U}}{\partial \tilde{r}}\left(1,\theta \right)$ , $0<\theta <2\text{π}$ , represents the change of velocity in the radial direction, all around the vessel wall.

The term $\frac{\partial \tilde{U}}{\partial \theta }\left(\tilde{r},\theta \right)$ represents the change of velocity in the azimuthal direction, at a given value of $\tilde{r}$ . The velocity is zero everywhere at the wall (r = R); consequently the velocity gradient $\frac{\partial \tilde{U}}{\partial \theta }\left(1,\theta \right)$ is also zero. The interesting quantities are thus $\frac{\partial \tilde{U}}{\partial \theta }\left(\tilde{r},\theta \right)$ , for $\tilde{r}$ close to 1, but lower than 1.

The dependence of the non-dimensional velocity $\tilde{U}$ upon θ (for $–\text{π}<\theta <+\text{π}$ ) is presented in Figure 2. It has been computed for $\tilde{r}=0.5$ . In the absence of magnetic field (H_a = 0), the situation is axisymmetric and the velocity does not depend upon θ. The flow is the classical Poiseuille flow and, as expected, $\tilde{U}\left(\tilde{r}=0.5\right)=3/2$ . When the Hartmann number increases, the flow is furthermore reduced (this is the decelerating effect due to the Lorentz force), and the dependence upon θ (asymmetry of the flow) is more and more pronounced. The velocity is maximal in the direction θ = 0 and θ = π (or −π), according to the fact that the profile is flattened and stretched parallel to the direction of B₀ (along Ox) [25] . For the same reason, the velocity is minimal in the direction θ = π/2 or −π/2.

The same type of results is shown in Figure 3, where the dependence of the velocity upon θ has been illustrated at $\tilde{r}=0.99$ (near the vessel wall). Of course, the velocities are very small, since at the wall, they are exactly zero. As in Figure 2, we can observe that the curve obtained for the case H_a = 0.16 is superimposed with the curve H_a = 0, meaning that the influence of a magnetic field B₀ =1.5 T (corresponding to H_a = 0.16) remains negligible. Moreover, when H_a = 0, the

value obtained for the non-dimensional velocity at r = 0.99 * R is 0.0398, which is the Poiseuille value.

The dependence of the non-dimensional velocity gradient $\partial \tilde{U}/\partial \tilde{r}$ upon θ (for $–\text{π}<\theta <+\text{π}$ ), at $\tilde{r}=0.99$ is illustrated in Figure 4 and at $\tilde{r}=1$ in Figure 5. These gradients are negative, since the value of the velocity $\tilde{U}$ decreases when going towards the vessel wall ( $\tilde{U}=0$ , when $\tilde{r}=1$ ). As previously noted, the influence of a 1.5 T magnetic field (H_a = 0.16) is not discernible, and the absolute values of the gradients $\partial \tilde{U}/\partial \tilde{r}$ are maximum for θ = 0, and θ = π, or −π (due to the fact that the profile is stretched along Ox).

In the absence of a magnetic field (H_a = 0), the Poiseuille value ( $\partial \tilde{U}/\partial \tilde{r}=-4$ , at the wall) is obtained, and no dependence upon θ is observed (axisymmetric situation). The maximum values of $\partial \tilde{U}/\partial \tilde{r}$ are increased by about 25% in the case of a very strong magnetic field (H_a = 4.47, B₀ = 40 T), when compared to the case H_a = 0.

The dependence of the non-dimensional velocity gradient $\partial \tilde{U}/\partial \theta$ upon θ (for $–\text{π}<\theta <+\text{π}$ ), at $\tilde{r}=0.5$ and at $\tilde{r}=0.99$ is illustrated in Figure 6 and Figure 7 respectively. Since we have $\tilde{U}=0$ , everywhere at the vessel wall ( $\tilde{r}=1$ ), we also have $\partial \tilde{U}/\partial \theta =0$ , for $\tilde{r}=1$ . Consequently, the absolute values of the $\partial \tilde{U}/\partial \theta$ velocity gradients decrease when $\tilde{r}$ tends towards 1.

In the absence of a magnetic field (H_a = 0), the situation is axisymmetric, and there is no dependence upon θ.

The non-dimensional shear stress, ${\tilde{\tau }}_{\theta z}$ , could be obtained dividing $\partial \tilde{U}/\partial \theta$ by the value of the corresponding $\tilde{r}$ (Equation (11)). For example, if we look at the maximum value of ${\tilde{\tau }}_{\theta z}$ for $\tilde{r}=0.99$ , we obtain $\left|{\tilde{\tau }}_{\theta z}\right|<0.02$ , which is negligible when compared to ${\tilde{\tau }}_{rz}$ (Figure 4).

4. Conclusion

In this paper, we demonstrate that the quantities ${\tilde{\tau }}_{rz}=\frac{\partial \tilde{U}}{\partial \tilde{r}}$ and ${\tilde{\tau }}_{\theta z}=\frac{1}{\tilde{r}}\frac{\partial \tilde{U}}{\partial \theta }$

both depend upon θ, but that this dependence may be considered negligible for low values of B₀ (B₀ < 3 T). We also demonstrate that, at the vessel wall, ${\tilde{\tau }}_{\theta z}$ is several orders of magnitude smaller than ${\tilde{\tau }}_{rz}$ , and that, in the presence of a very strong magnetic field (H_a = 4.47, B₀ = 40 T), the maximum value of ${\tilde{\tau }}_{rz}$ is only increased by 25%, when compared to its value in the absence of a magnetic field (H_a = 0). Consequently, in most of the situations encountered in biomedical applications, the classical calculation (η(∂u/ ∂r)) remains a good approximation to evaluate the shear stresses at the wall.

Declarations

Competing interests: none.

Funding: none.

Ethical approval: not required.

Cite this paper

Drochon, A, Beuque, M. and Abi-Abdallah Rodriguez, D. (2017) Impact of an External Magnetic Field on the Shear Stresses Exerted by Blood Flowing in a Large Vessel. Journal of Applied Mathematics and Physics, 5, 1493- 1502. https://doi.org/10.4236/jamp.2017.57122

References