Open Journal of Discrete Mathematics
Vol.06 No.02(2016), Article ID:65436,6 pages
10.4236/ojdm.2016.62010
Double Derangement Permutations
Pooya Daneshmand1, Kamyar Mirzavaziri2, Madjid Mirzavaziri3
1Ferdowsi University of Mashhad, International Campus, Mashhad, Iran
2National Organization for Development of Exceptional Talents (NODET) I, Mashhad, Iran
3Department of Pure Mathematics, Ferdowsi University of Mashhad, Mashhad, Iran

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 28 September 2015; accepted 9 April 2016; published 12 April 2016
ABSTRACT
Let n be a positive integer. A permutation a of the symmetric group  of permutations of
of permutations of  is called a derangement if
is called a derangement if 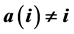 for each
for each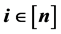 . Suppose that x and y are two arbitrary permutations of
. Suppose that x and y are two arbitrary permutations of . We say that a permutation a is a double derangement with respect to x and y if
. We say that a permutation a is a double derangement with respect to x and y if  and
and 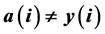 for each
for each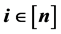 . In this paper, we give an explicit for- mula for
. In this paper, we give an explicit for- mula for , the number of double derangements with respect to x and y. Let
, the number of double derangements with respect to x and y. Let 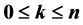 and let
and let  and
and 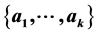 be two subsets of
be two subsets of 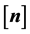 with
with 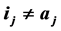 and
and . Suppose that
. Suppose that 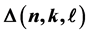 denotes the number of derangements x such that
denotes the number of derangements x such that







Keywords:
Symmetric Group of Permutations, Derangement, Double Derangement
1. Introduction
Let n be a positive integer. A derangement is a permutation of the symmetric group 



The number of derangements also satisfies the relation
exclusion principle that 


These facts and some other results concerning derangements can be found in [1] . There are also some generalizations of this notion. The problème des rencontres asks how many permutations of the set 
exactly k fixed points. The number of such permutations is denoted by 


concerning the permutations of 
Let e be the identity element of the symmetric group















2. The Result
In the following, we assume that n is a positive integer and the identity permutation of the symmetric group 






Definition 1. Suppose that x and y are two arbitrary permutations of



Proposition 1. Let 







Proof. Let


Case 1.




















Case 2.
















These considerations show that
We can therefore assume that





For a derangement x satisfying 


If the first case occurs then we have to evaluate the number of derangements of the set 



If the second case occurs then we have to evaluate the number of derangements of the set 



We now use induction on k to show that
For 
Now let the result be true for
Corollary 1. Let k be a positive integer. Then
Proof. Let










The following Table 1 gives some small values of
The following lemma can be easily proved.
Lemma 1. Let x and y be two arbitrary permutations and 






Theorem 2. Let 




Table 1. Values of 


where
Proof. Let 




and 
where
Our ultimate goal is to find an explicit formula for evaluating 


Lemma 2. Let 





Moreover, 


Proof. We can restate the problem as follows: We want to put k ones and 



the 


the i-th block be


Now suppose that we write 

Lemma 3. Let 





Moreover, 


Proof. Similar to the above argument, we want to put k ones and 

Case 1. There is no block of ones before the first zero and after the last zero. In this case we put 
and choose 


ways. Let the number of ones in the i-th block be

solutions for the latter equation is
Case 2. There is no block of ones before the first zero but there is a block after the last zero. In this case we put 



ones in 



Case 3. There is a block of ones before the first zero but there is no block after the last zero. This is similar to the above case.
Case 4. There is a block of ones before the first zero and a block of ones after the last zero. In this case we must have 




places for putting 




These considerations prove that
A straightforward computation gives the result.
The following Table 2 gives some small values of
Table 2. Values of 


Theorem 3. Let c be be a cycle of length
Proof. Let 






Using the notations of Theorem 2, 








Example 1. We evaluate 


and 

Applying Theorem 3 with 
and 

The above example shows that how can we evaluate 


Cite this paper
Pooya Daneshmand,Kamyar Mirzavaziri,Madjid Mirzavaziri, (2016) Double Derangement Permutations. Open Journal of Discrete Mathematics,06,99-104. doi: 10.4236/ojdm.2016.62010
References
- 1. Graham, R.L., Knuth, D.E. and Patashnik, O. (1988) Concrete Mathematics. Addison-Wesley, Reading.
- 2. Pitman, J. (1997) Some Probabilistic Aspects of Set Partitions. American Mathematical Monthly, 104, 201-209.
http://dx.doi.org/10.2307/2974785 - 3. Knopfmacher, A., Mansour, T. and Wagner, S. (2010) Records in Set Partitions. The Electronic Journal of Combinatorics, 17, R109.























