ABSTRACT

In this paper, we prove a common fixed point theorem in Intuitionistic fuzzy metric space by using pointwise R-weak commutativity and reciprocal continuity of mappings satisfying contractive conditions.

1. Introduction

Atanassove [1] introduced and studied the concept of intuitionistic fuzzy sets as a generalization of fuzzy sets. In 2004, Park [2] defined the notion of intuitionistic fuzzy metric space with the help of continuous t-norms and continuous t-conorms. Recently, in 2006, Alaca et al. [3] defined the notion of intuitionistic fuzzy metric space by making use of Intuitionistic fuzzy sets, with the help of continuous t-norm and continuous t-conorms as a generalization of fuzzy metric space due to Kramosil and Michalek [4]. In 2006, Turkoglu [5] et al. proved Jungck’s [6] common fixed point theorem in the setting of intuitionistic fuzzy metric spaces for commuting mappings. For more details on intuitionistic fuzzy metric space, one can refer to the papers [7-12].

The aim of this paper is to prove a common fixed point theorem in intuitionistic fuzzy metric space by using pointwise R-weak commutativity [5] and reciprocal continuity [9] of mappings satisfying contractive conditions.

2. Preliminaries

Definition 2.1 [13]. A binary operation 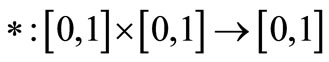is continuous t-norm if * satisfies the following conditions:

1) * is commutative and associative;

2) * is continuous;

3) 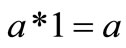for all;

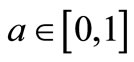4) 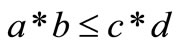whenever and 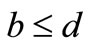for all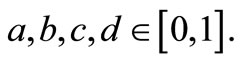

Definition 2.2 [13]. A binary operation is continuous t-conorm if ◊ satisfies the following conditions:

1) ◊ is commutative and associative;

2) ◊ is continuous;

3) 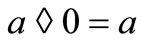for all;

4) 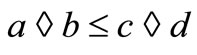whenever 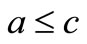and 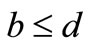for all

Alaca et al. [3] defined the notion of intuitionistic fuzzy metric space as:

Definition 2.3 [3]. A 5-tuple 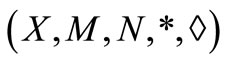is said to be an intuitionistic fuzzy metric space if X is an arbitrary set, * is a continuous t-norm, ◊ is a continuous tconorm and 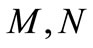are fuzzy sets on X² × [0, ∞) satisfying the conditions:

1) 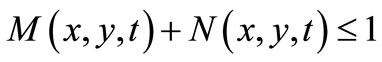for all 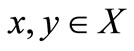and;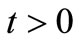

2) 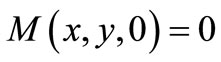for all;

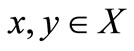3) 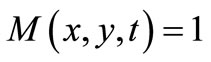for all 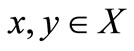and 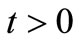if and only if;

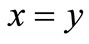4) 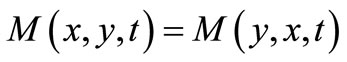for all and t > 0;

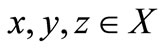5) for all and;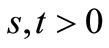

6) is left continuous, for all;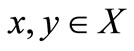

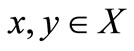7) for all and;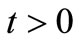

8) for all;

9) 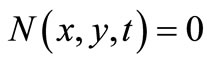for all 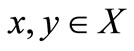and 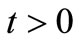if and only if;

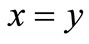10) 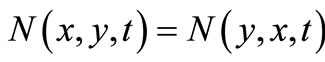for all 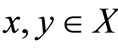and t > 0;

11) 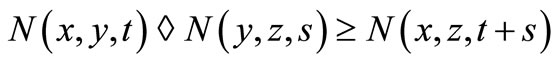for all 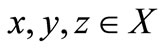and;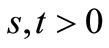

12) 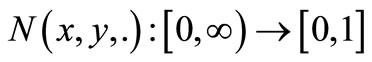is right continuous, for all;

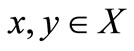13) for all.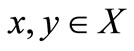

The functions 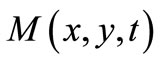and denote the degree of nearness and the degree of non-nearness between x and y w.r.t. t respectively.

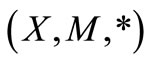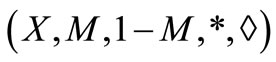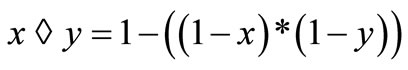Remark 2.1 [12]. Every fuzzy metric space is an intuitionistic fuzzy metric space of the form such that t-norm * and t-conorm are associated as for all.

Remark 2.2 [12]. In intuitionistic fuzzy metric space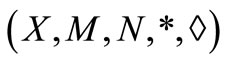, 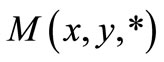is non-decreasing and 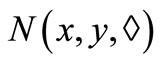is non-increasing for all.

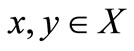Definition 2.4 [3]. Let 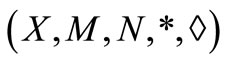be an intuitionistic fuzzy metric space. Then

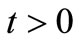1) A sequence in X is said to be Cauchy sequence if, for all and,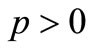 

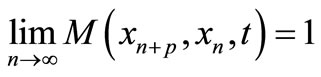
and

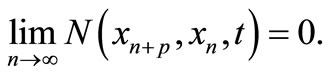
2) A sequence 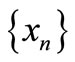in X is said to be convergent to a point 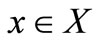if, for all,

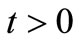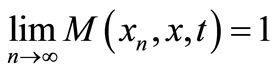
and

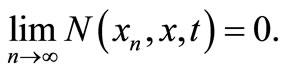
Definition 2.5 [3]. An intuitionistic fuzzy metric space 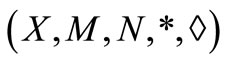is said to be complete if and only if every Cauchy sequence in X is convergent.  

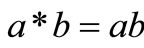Example 2.1 [3]. Let and let * be the continuous t-norm and ◊ be the continuous tconorm defined by and 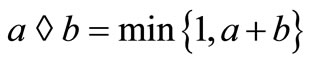respectively, for all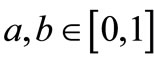. For each 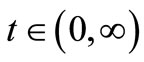and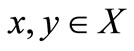, define M and N by  

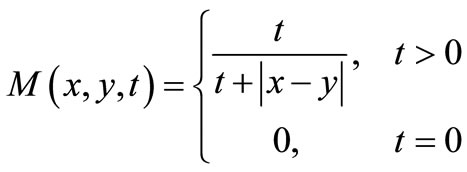
and 

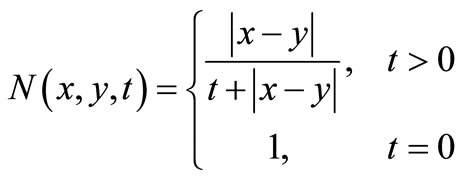
Clearly, 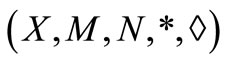is complete intuitionistic fuzzy metric space.

Definition 2.6 [3]. A pair of self mappings 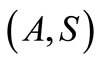of a intuitionistic fuzzy metric space 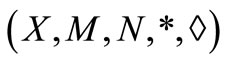is said to be commuting if 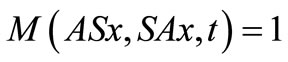and 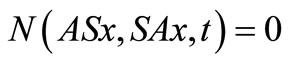for all.

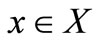Definition 2.7 [3]. A pair of self mappings 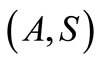of a intuitionistic fuzzy metric space is said to be weakly commuting if and 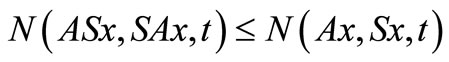for all and.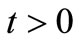

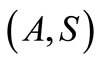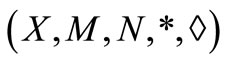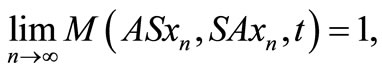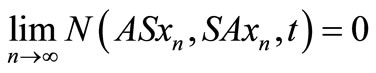Definition 2.8 [12]. A pair of self mappings of a intuitionistic fuzzy metric space is said to be compatible if and for all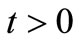, whenever 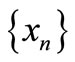is a sequence in X such that 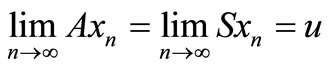for some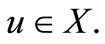

Definition 2.9 [5]. A pair of self mappings 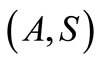of a intuitionistic fuzzy metric space 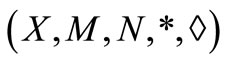is said to be pointwise R-weakly commuting, if given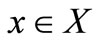, there exist such that for all

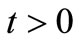
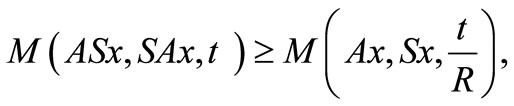
and

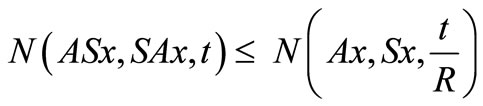
Clearly, every pair of weakly commuting mappings is pointwise R-weakly commuting with.

Definition 2.10 [9]. Two mappings A and S of a Intuitionistic fuzzy metric space 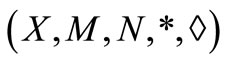are called reciprocally continuous if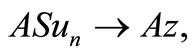 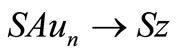, whenever 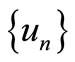is a sequence such that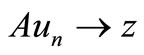, for some z in X.

If A and S are both continuous, then they are obviously reciprocally continuous but converse is not true.

3. Lemmas

The proof of our result is based upon the following lemmas of which the first two are due to Alaca et al. [12]:  

Lemma 3.1 [12]. Let 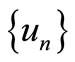is a sequence in a intuitionistic fuzzy metric space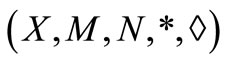. If there exists a constant 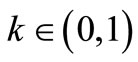such that

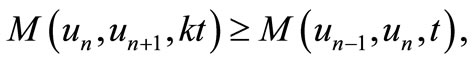
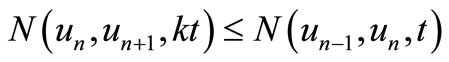
for all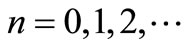

Then 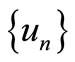is a Cauchy sequence in X.

Lemma 3.2 [12]. Let 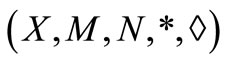be intuitionistic fuzzy metric space and for all, 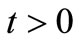and if for a number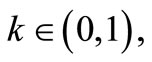 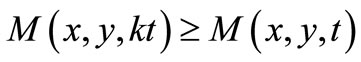and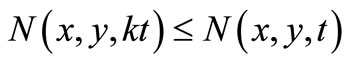. Then x = y.

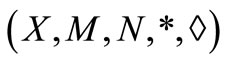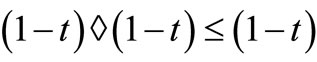Lemma 3.3. Let be a complete intuitionistic fuzzy metric space with continuous t-norm * and continuous t-conorm ◊ defined by and for all 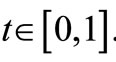Further, let 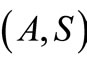and 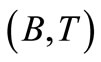be pointwise R-weakly commuting pairs of self mappings of X satisfying:

(3.1)

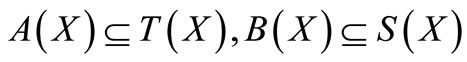
(3.2) there exists a constant 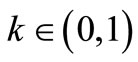such that

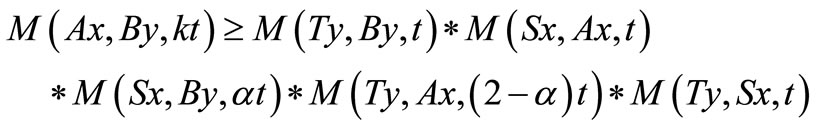
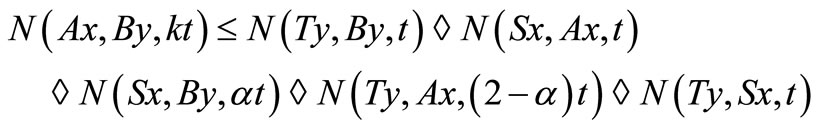
for all, 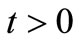and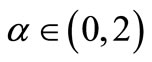. Then the continuity of one of the mappings in compatible pair 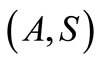or on implies their reciprocal continuity.

Proof. First, assume that A and S are compatible and S is continuous. We show that A and S are reciprocally continuous. Let 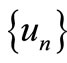be a sequence such that and 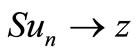for some 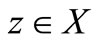as.

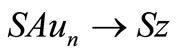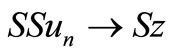Since S is continuous, we have and as 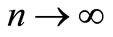and since 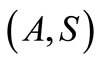is compatible, we have

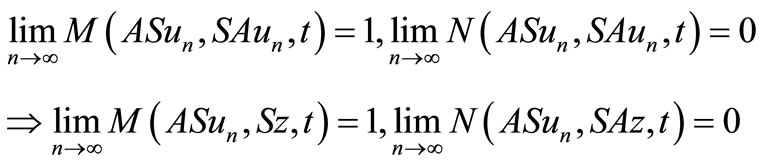
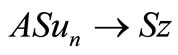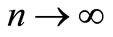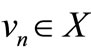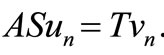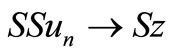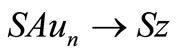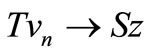That is as. By (3.1), for each n, there exists such that Thus, we have, , and as 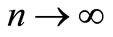whenever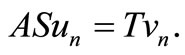

Now we claim that 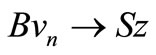as.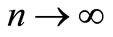

Suppose not, then taking in (3.2), we have

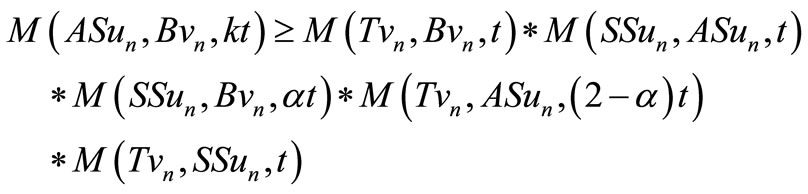
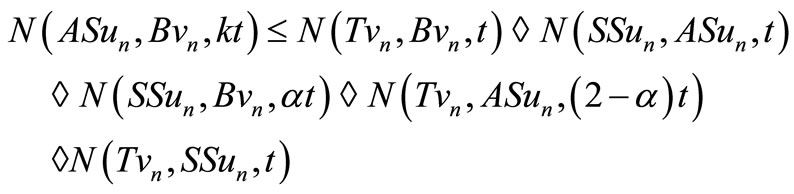
Taking, we get

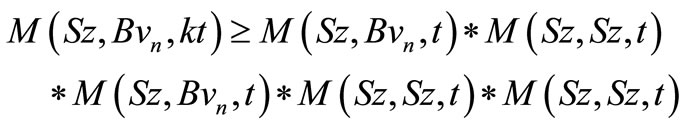
That is,

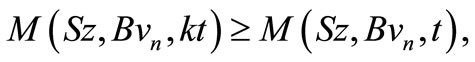
by the use of Lemma 3.2, we have 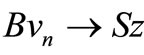as.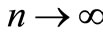

Now, we claim that 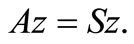Again take 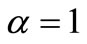in (3.2), we have

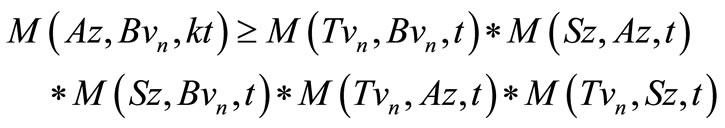
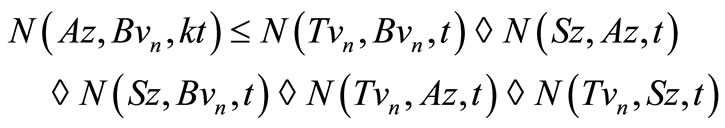
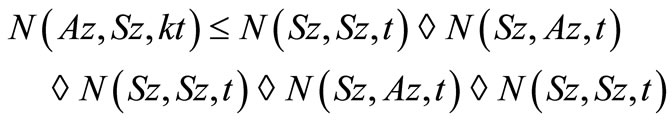
i.e.

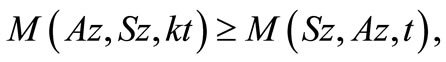
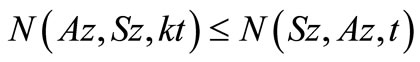
therefore, by use of Lemma 3.2, we have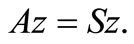

Hence, , 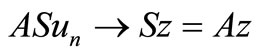as.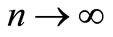

This proves that A and S are reciprocally continuous on X. Similarly, it can be proved that B and T are reciprocally continuous if the pair 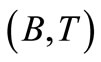is assumed to be compatible and T is continuous.

4. Main Result

The main result of this paper is the following theorem:

Theorem 4.1. Let 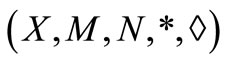be a complete intuitionistic fuzzy metric space with continuous t-norm * and continuous t-conorm defined by and 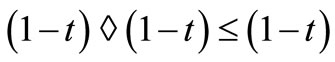for all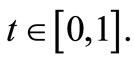  

Further, let 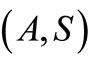and 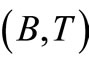be pointwise R-weakly commuting pairs of self mappings of X satisfying (3.1), (3.2). If one of the mappings in compatible pai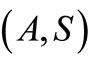r or 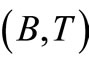is continuous, then A, B, S and T have a unique common fixed point.

Proof. Let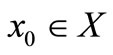. By (3.1), we define the sequences 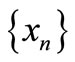and 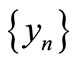in X such that for all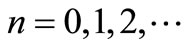

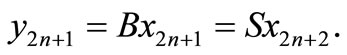
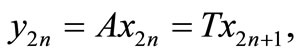We show that 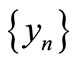is a Cauchy sequence in X. By (3.2) take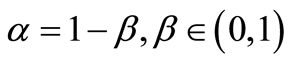, we have

Now, taking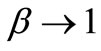, we have

Similarly, we can show that

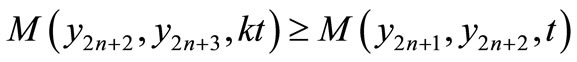
Also,

Taking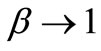, we get

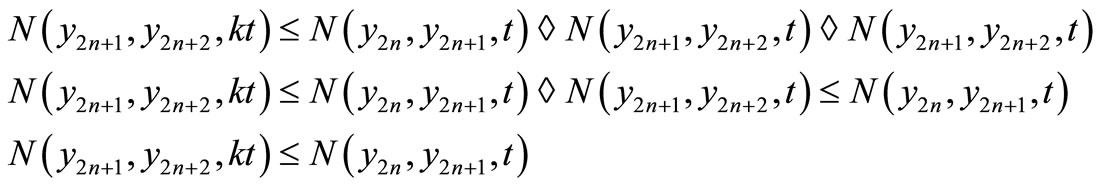
Similarly, it can be shown that

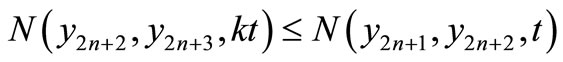
Therefore, for any n and t, we have

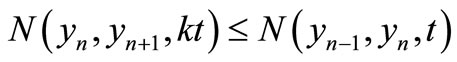
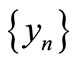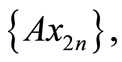
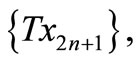
Hence, by Lemma 3.1, is a Cauchy sequence in X. Since X is complete, so converges to z in X. Its subsequences 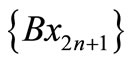and 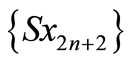also converge to z.

Now, suppose that 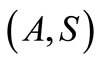is a compatible pair and S is continuous. Then by Lemma 3.2, A and S are reciprocally continuous, then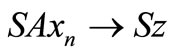, as.

As, 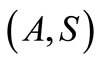is a compatible pair. This implies

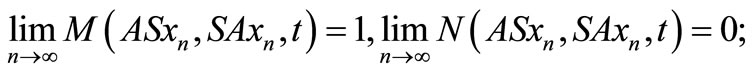
This give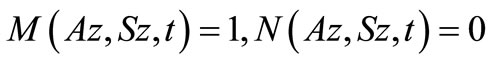s as.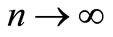

Hence,.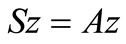

Since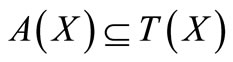, therefore there exists a point 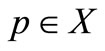such that

Now, again by taking 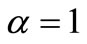in (3.2), we have

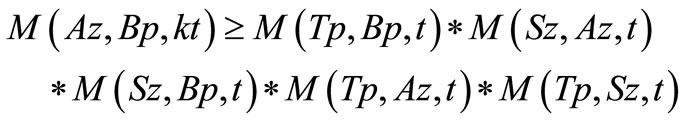
and

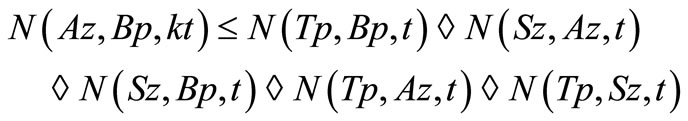
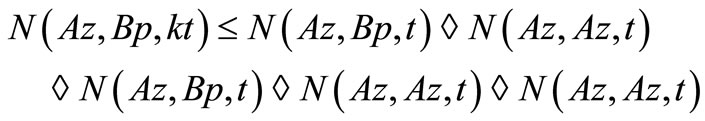
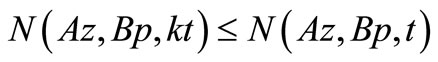
Thus, by Lemma 3.2, we have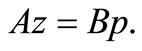

Thus,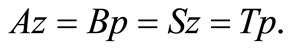

Since, A and S are pointwise R-weakly commuting mappings, therefore there exists, such that

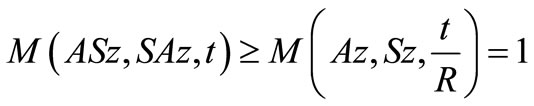
and

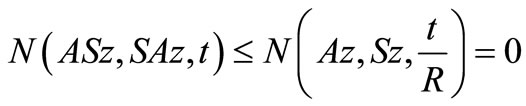
Hence, 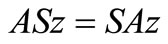and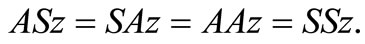

Similarly, B and T are pointwise R-weakly commuting mappings, we have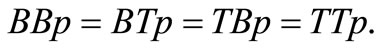  

Again, by taking 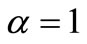in (3.2),

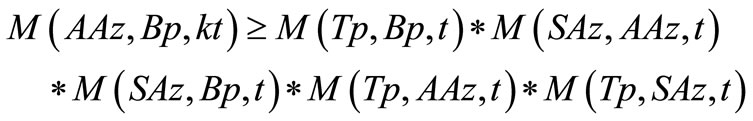
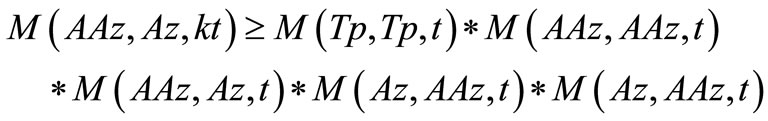
and

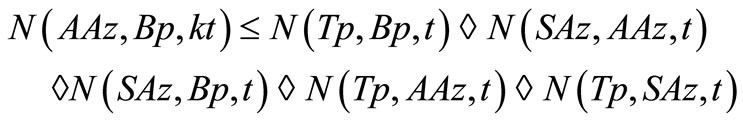
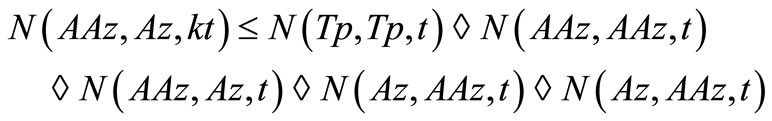
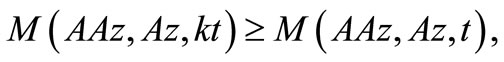
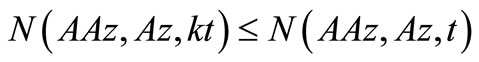
By Lemma 3.2, we hav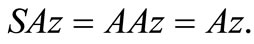e Hence 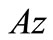is common fixed point of A and S. Similarly by (3.2), 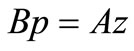is a common fixed point of B and T. Hence, 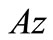is a common fixed point of A, B, S and T.

Uniqueness: Suppose that 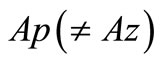is another common fixed point of A, B, S and T.

Then by (3.2), take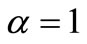

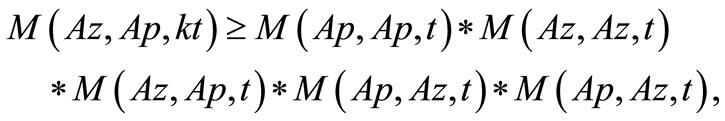and

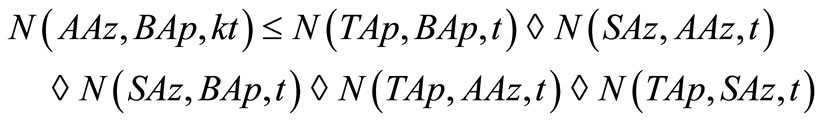
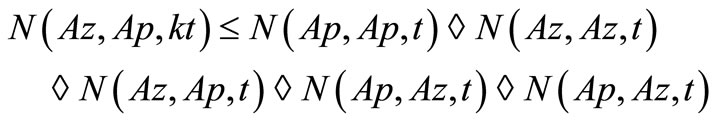
This gives

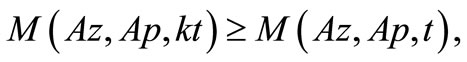and

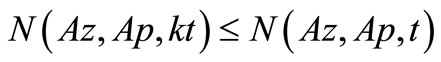
By Lemma 3.2,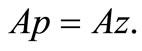

Thus, uniqueness follows.

Taking 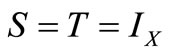in above theorem, we get following result:

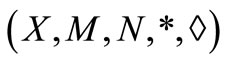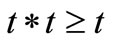Corollary 4.1. Let be a complete intuitionistic fuzzy metric space with continuous t-norm * and continuous t-conorm defined by and 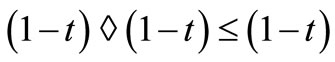for all 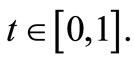Further, let A and B are reciprocally continuous mappings on X satisfying

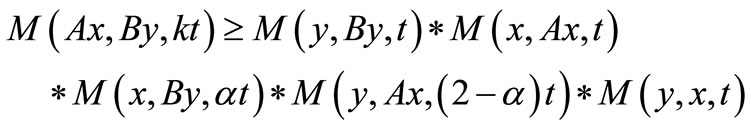
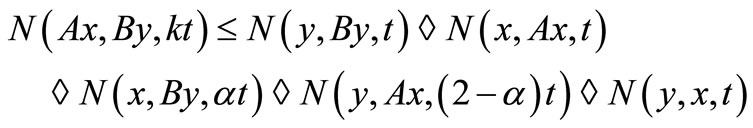
for all, 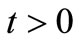and 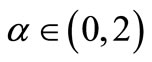then pair A and B has a unique common fixed point.

We give now example to illustrate the above theorem:

Example 4.1. Let 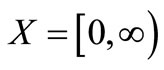and let 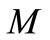and 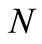be defined by

and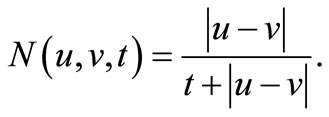

Then 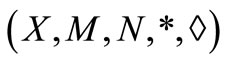is complete intuitionistic fuzzy metric space. Let A, B, S and T be self maps on X defined as:

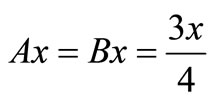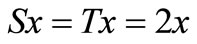and for all.

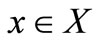Clearly

1)    either of pair (A, S) or (B, T) be continuous self-mappings on X;

2)    ;

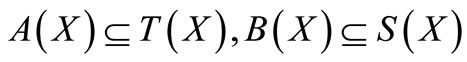3)    {A, S} and {B, T} are R-weakly commuting pairs as both pairs commute at coincidence points;

4)    {A, S} and {B, T} satisfies inequality (3.2), for all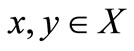, where.

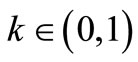Hence, all conditions of Theorem 4.1 are satisfied and x = 0 is a unique common fixed point of A, B, S and T.

5. Acknowledgements

We would like to thank the referee for the critical comments and suggestions for the improvement of my paper.

REFERENCES

NOTES

^*Corresponding author.