Advances in Pure Mathematics
Vol.3 No.9(2013), Article ID:41127,5 pages DOI:10.4236/apm.2013.39098
Value Distribution of L-Functions with Rational Moving Targets
1Intelligent Medical Objects, Inc., Northbrook, USA
2Department of Mathematical Sciences, Northern Illinois University, DeKalb, USA
Email: mcardwell@e-imo.com, *ye@math.niu.edu
Copyright © 2013 Matthew Cardwell, Zhuan Ye. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. In accordance of the Creative Commons Attribution License all Copyrights © 2013 are reserved for SCIRP and the owner of the intellectual property Matthew Cardwell, Zhuan Ye. All Copyright © 2013 are guarded by law and by SCIRP as a guardian.
Received August 26, 2013; revised September 26, 2013; accepted October 1, 2013
Keywords: Value Distribution; Moving Target; L-Function; Selberg Class
ABSTRACT
We prove some value-distribution results for a class of L-functions with rational moving targets. The class contains Selberg class, as well as the Riemann-zeta function.
1. Introduction
We define the class 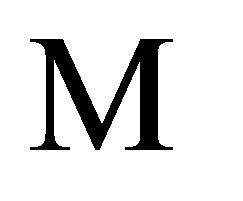 to be the collection of functions
to be the collection of functions
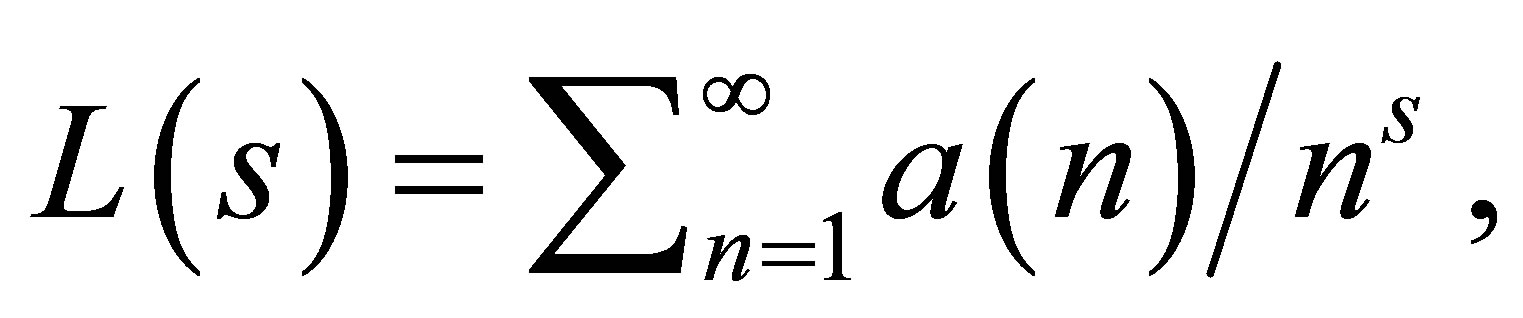 satisfying Ramanujan hypothesisAnalytic continuation and Functional equation. We also denote the degree of a function
satisfying Ramanujan hypothesisAnalytic continuation and Functional equation. We also denote the degree of a function 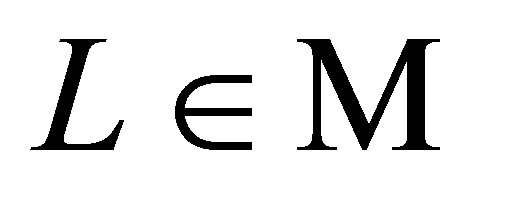 by
by 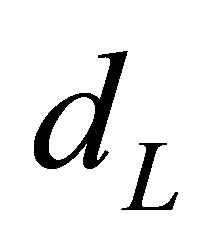 which is a non-negative real number. We refer the reader to Chapter six of [1] for a complete definitions. Obviously, the class
which is a non-negative real number. We refer the reader to Chapter six of [1] for a complete definitions. Obviously, the class 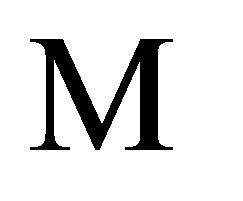 contains the Selberg class. Also every function in the class
contains the Selberg class. Also every function in the class 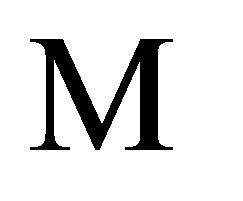 is an
is an  -function and the Riemann-zeta function is in the class. In this paper, we prove a value-distribution theorem for the class
-function and the Riemann-zeta function is in the class. In this paper, we prove a value-distribution theorem for the class 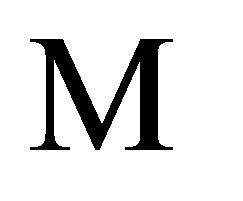 with rational moving targets. The theorem generalizes the value-distribution results in Chapter seven of [1] from fixed targets to moving targets.
with rational moving targets. The theorem generalizes the value-distribution results in Chapter seven of [1] from fixed targets to moving targets.
Theorem. Assume that 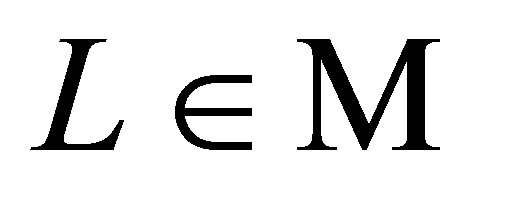 and
and 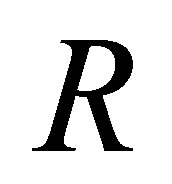 is a rational function with
is a rational function with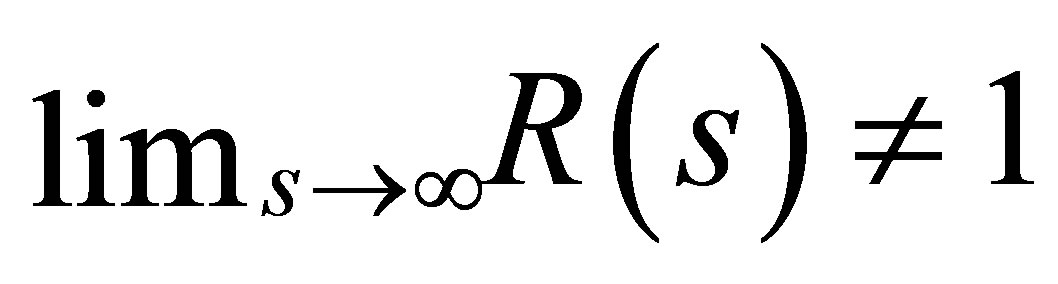 . Let the roots of the equation
. Let the roots of the equation 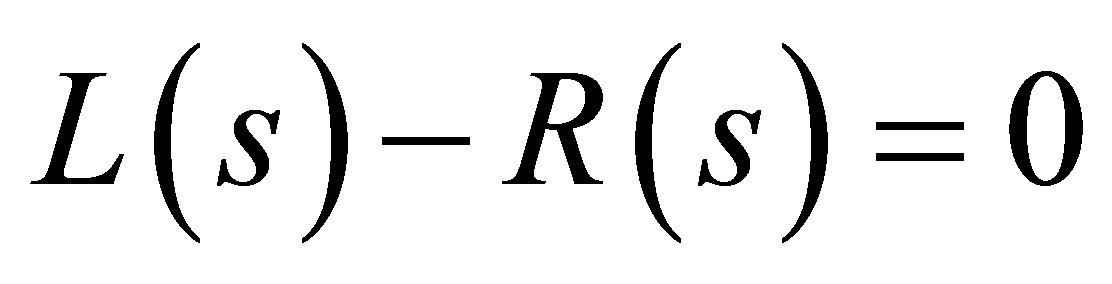 be denoted by
be denoted by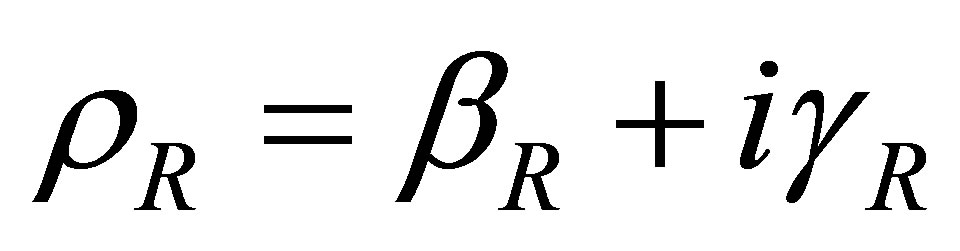 . Then
. Then
(I) For any ,
,
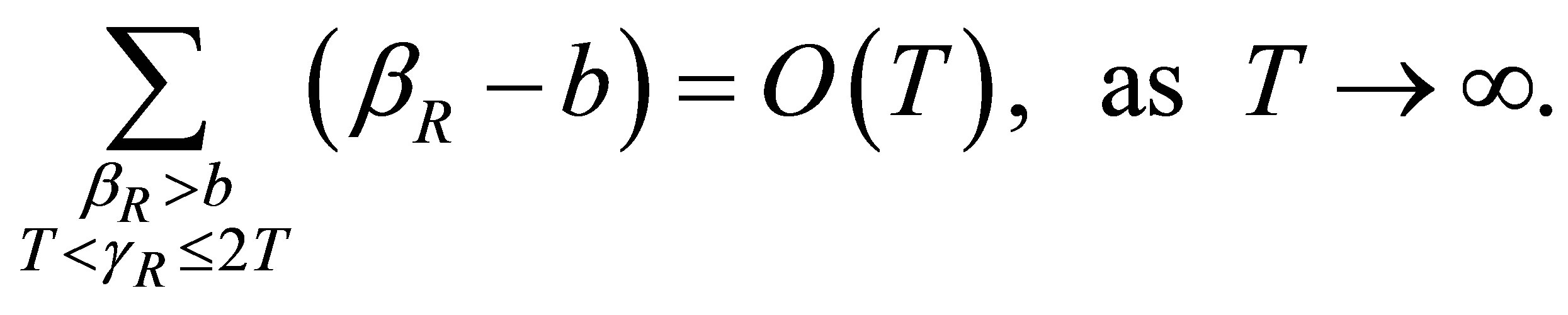
(II) For sufficiently large negative ,
,
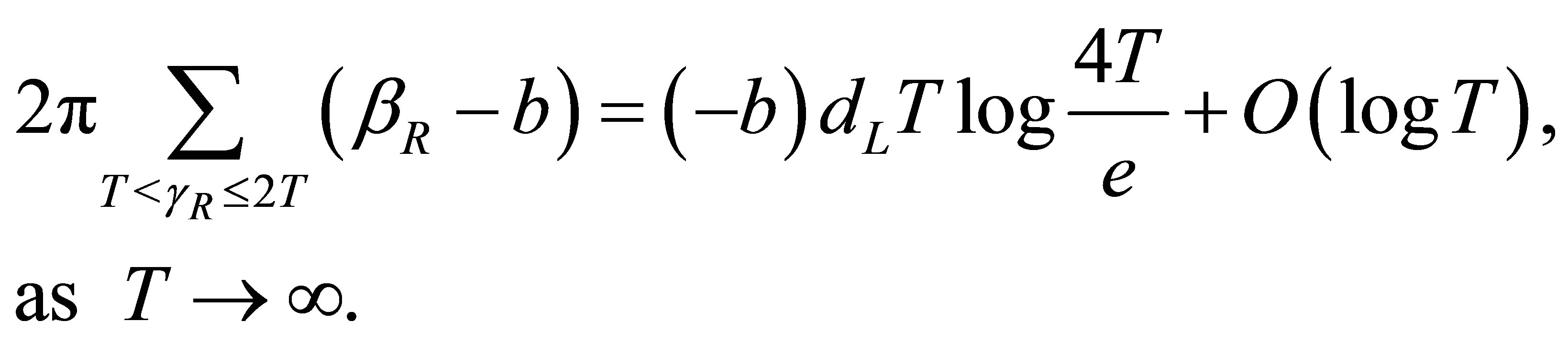
Proof of (I). It is known that if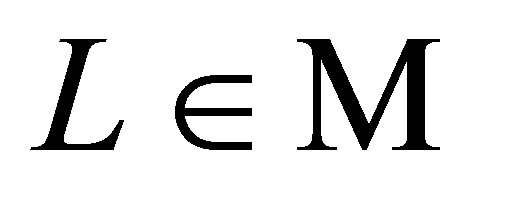 , then
, then
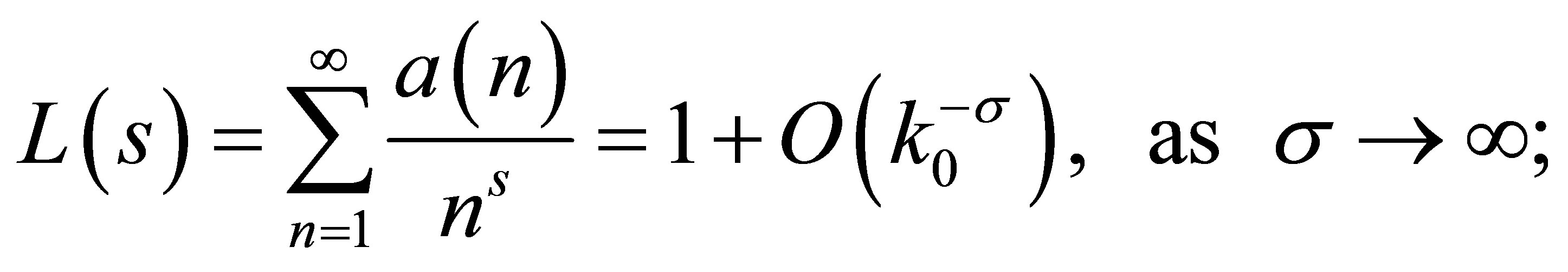
where 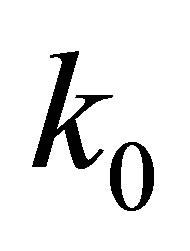 is the index of the first non-zero term of the sequence of
is the index of the first non-zero term of the sequence of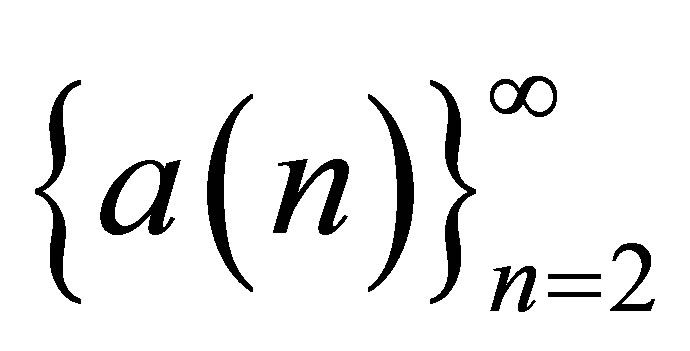 ,
, 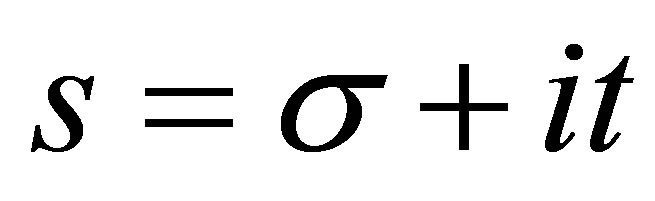 with
with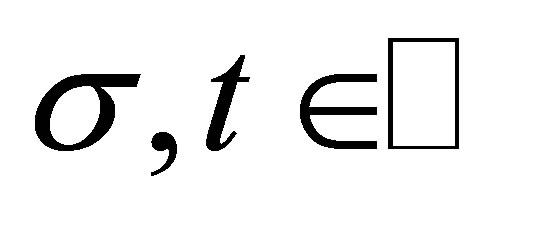 . Since
. Since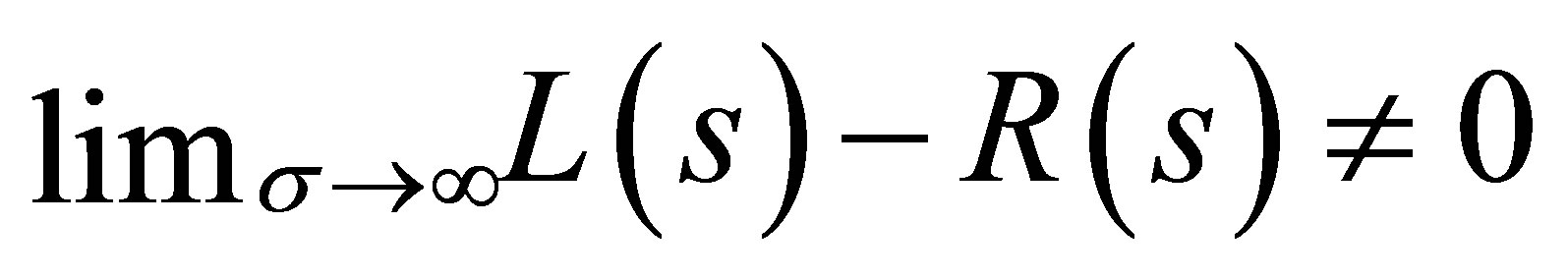 , there exists
, there exists  such that
such that 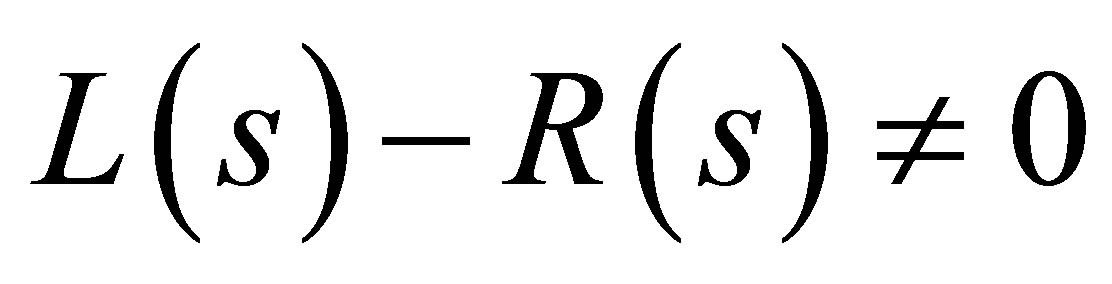 for
for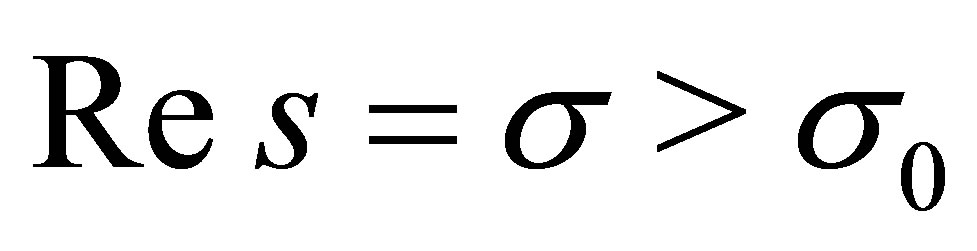 . It follows that
. It follows that 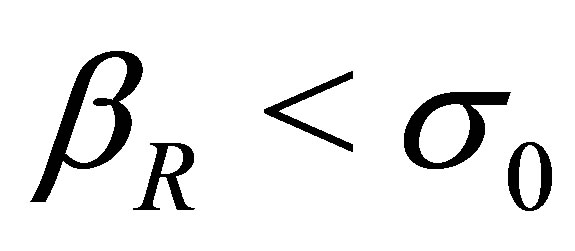 for all real part of zeros of the function
for all real part of zeros of the function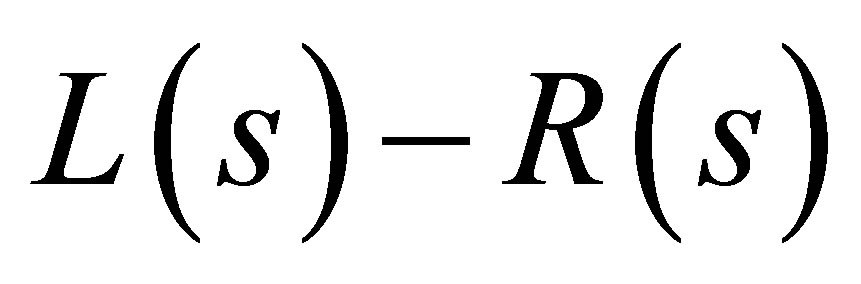 . We set
. We set  where the degrees of
where the degrees of 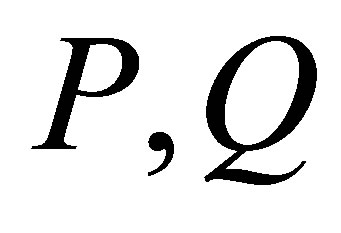 are
are , respectively; and define
, respectively; and define
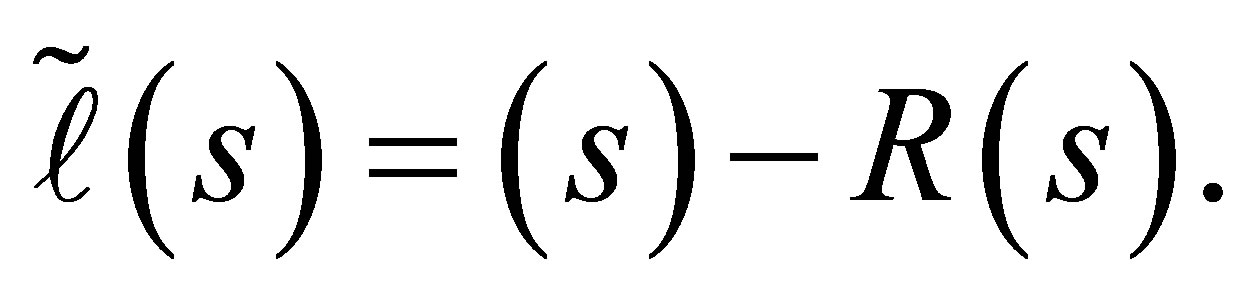
Thus, there is 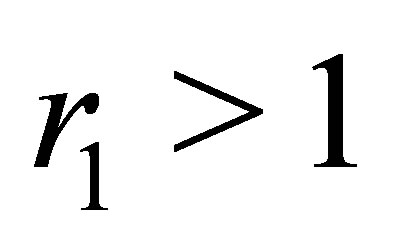 such that
such that  is analytic in the region
is analytic in the region  since
since 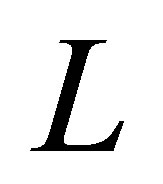 is a meromorphic function in
is a meromorphic function in  with the only pole at
with the only pole at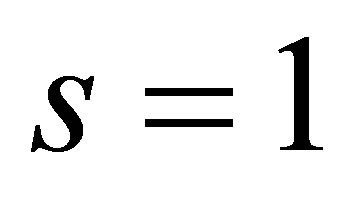 . We apply Littlewood’s argument principle [3] to
. We apply Littlewood’s argument principle [3] to  in the rectangle
in the rectangle  where
where 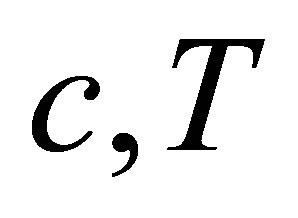 are parameters satisfying
are parameters satisfying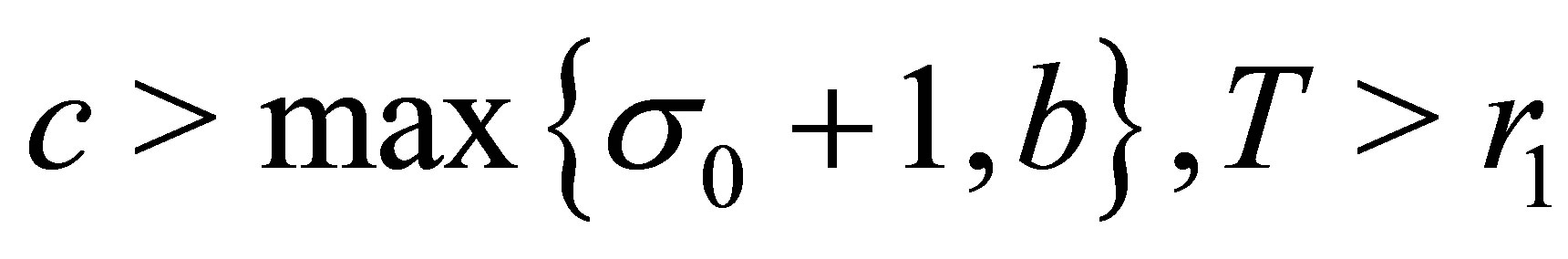 . Thus,
. Thus,
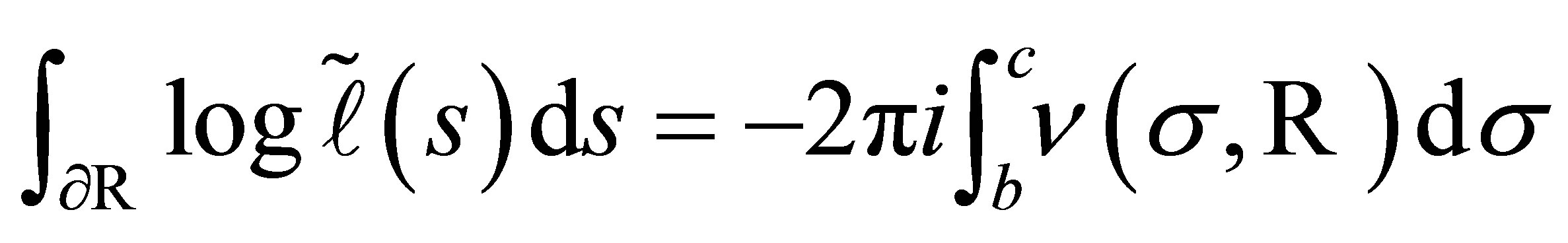
where the given logarithm is defined as in Littlewood’s argument principle [3]. To prove our result, however, we first decompose our auxiliary function by
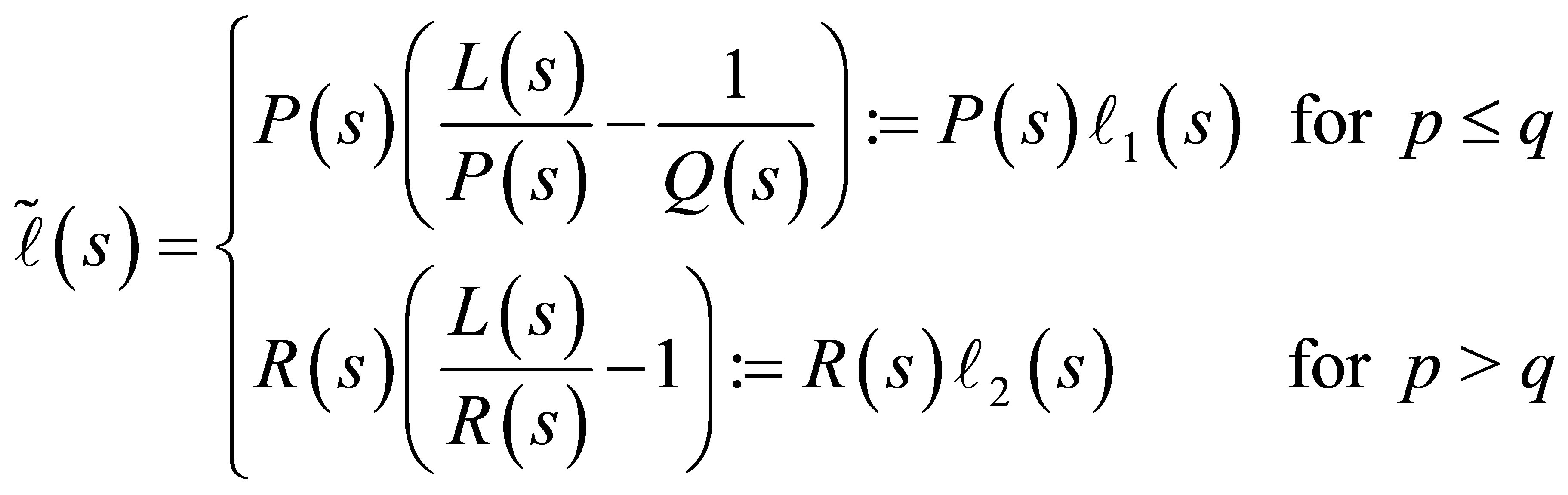 (1)
(1)
Without loss of generality, we may assume that  whenever
whenever 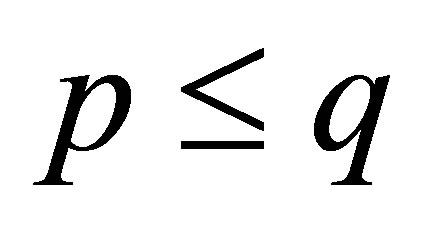 since we can always write
since we can always write  for
for 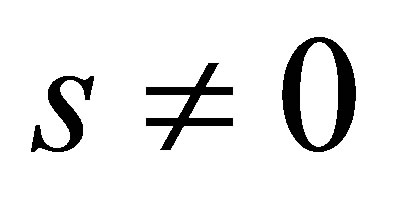 due to our choice of the parameters which define the rectangle
due to our choice of the parameters which define the rectangle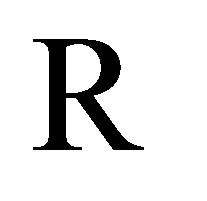 . However, the modification will guarantee in the case of
. However, the modification will guarantee in the case of 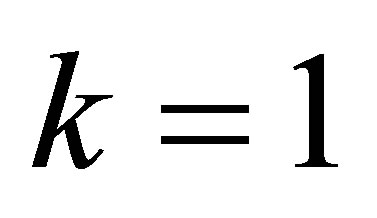 that
that 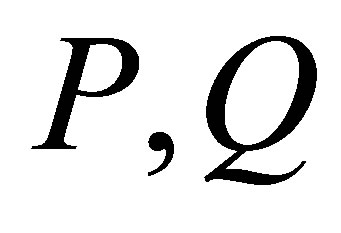 exhibit polynomial growth, which is necessary for our proof. In the case of
exhibit polynomial growth, which is necessary for our proof. In the case of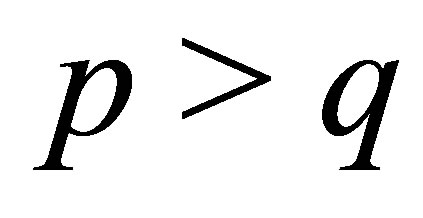 ,
, 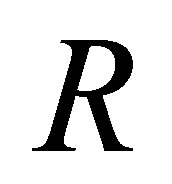 already exhibits polynomial growth, and no such adjustment is necessary. We now integrate the logarithm of
already exhibits polynomial growth, and no such adjustment is necessary. We now integrate the logarithm of  to get
to get
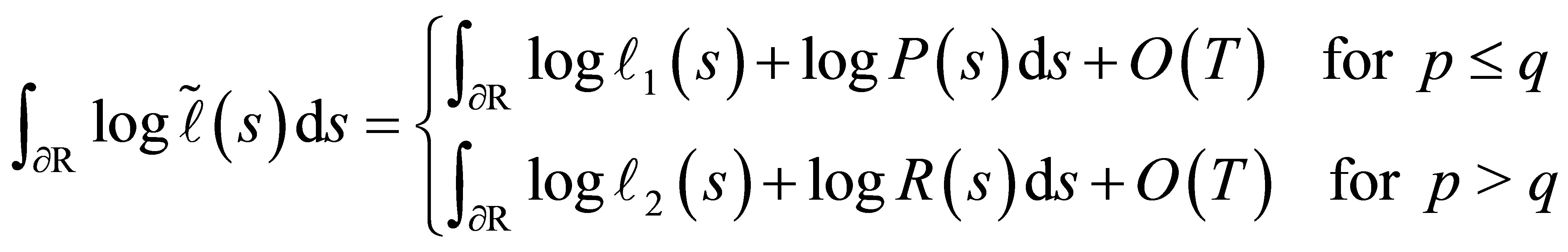
where the 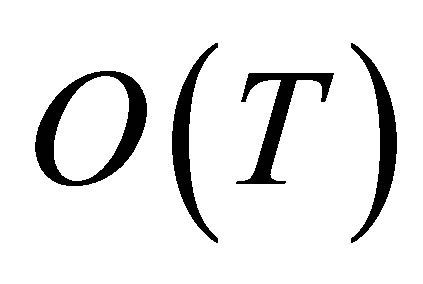 terms are the integrals of the maximum contribution from writing
terms are the integrals of the maximum contribution from writing 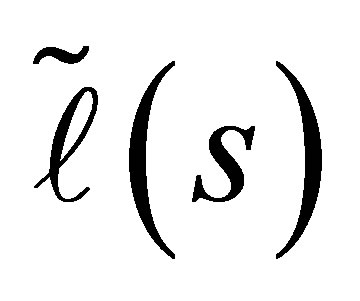 as a sum of logarithms. By our choice of
as a sum of logarithms. By our choice of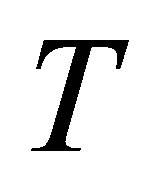 , both
, both 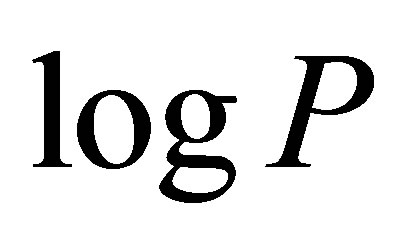 and
and 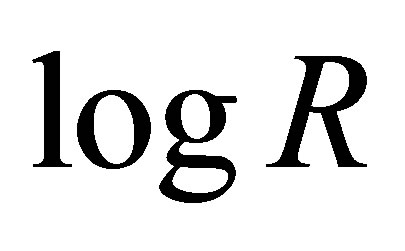 are analytic in
are analytic in 
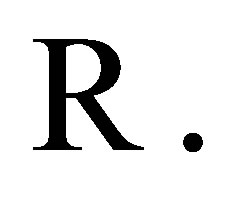 Hence, Cauchy’s Theorem gives
Hence, Cauchy’s Theorem gives
 (2)
(2)
To connect this integral with Littlewood’s argument principle [3], we note that the definition of  guarantees that
guarantees that
 (3)
(3)
In light of (2) and because the quantity given in (3) is imaginary-valued, we get for 
 (4)
(4)
for instance.
We now estimate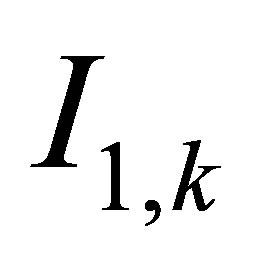 . For
. For 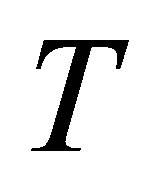 large enough, we have for
large enough, we have for 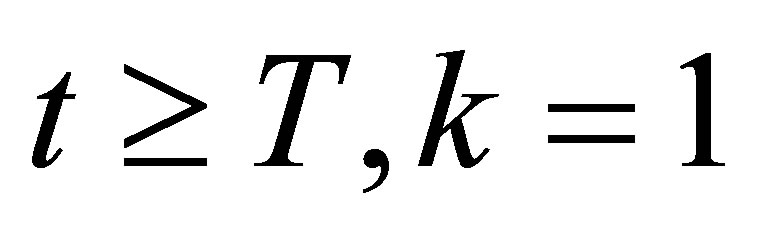 (since
(since ),
),

Then for 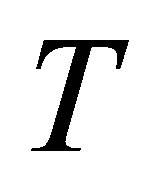 large enough,
large enough, 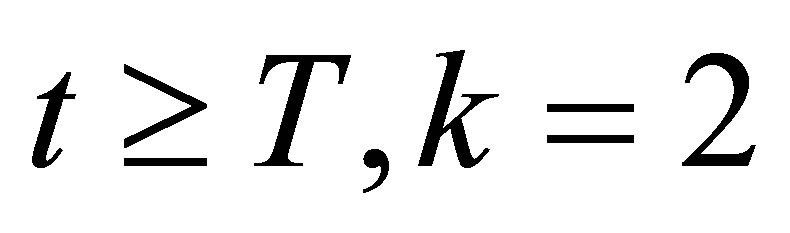 , we find in a similar fashion that
, we find in a similar fashion that
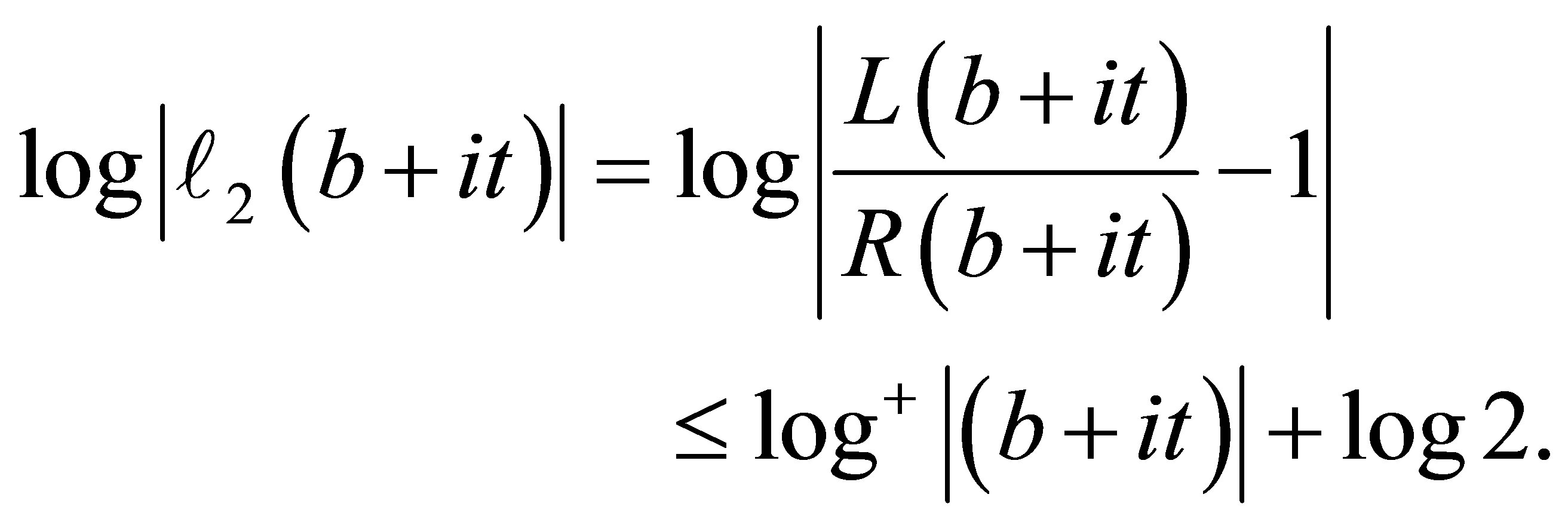
Since we have the same estimate for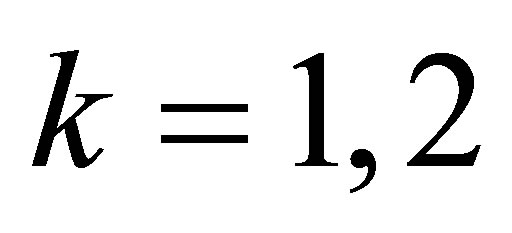 , we find that
, we find that
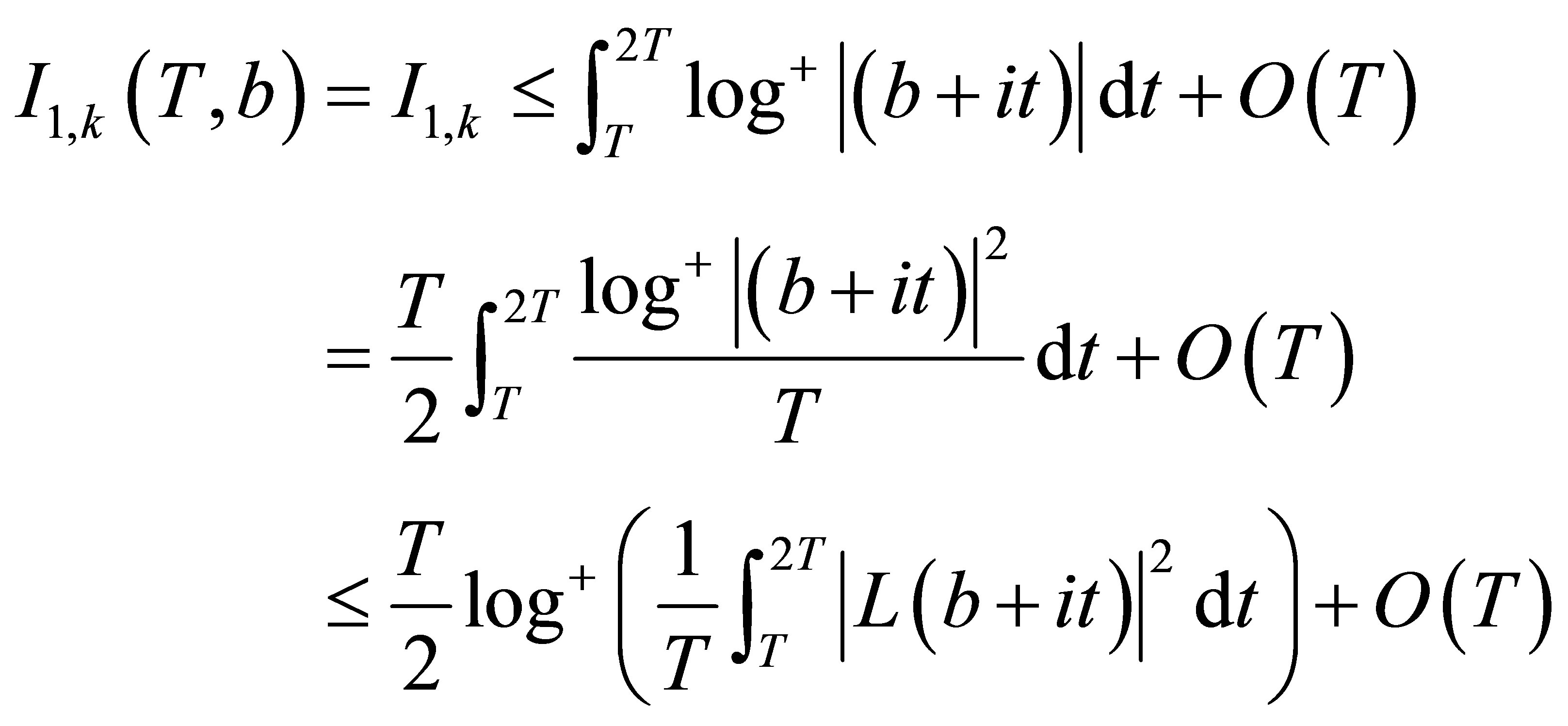
where the final bound follows from Jensen’s inequality. It is known [2] that for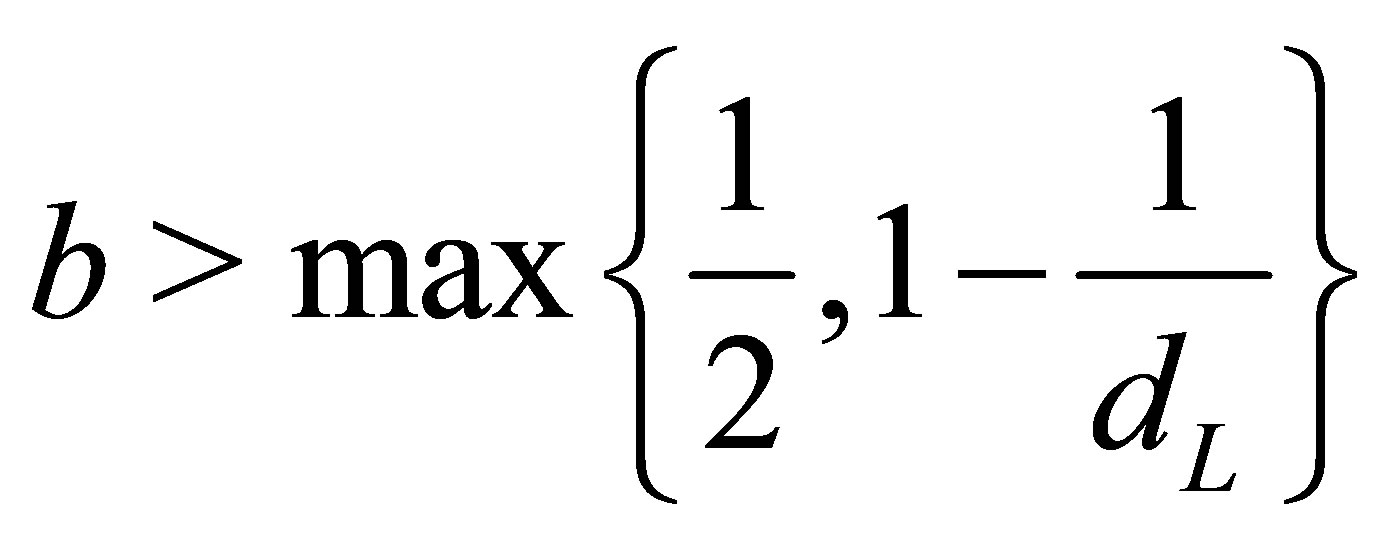 ,
,

Hence, 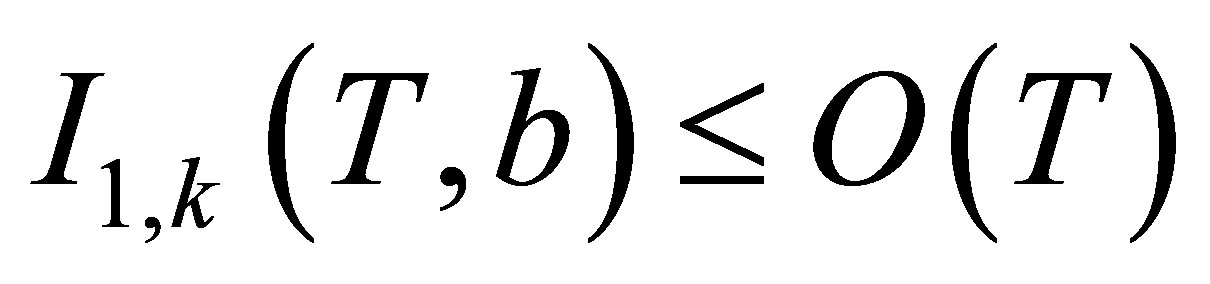 uniformly in
uniformly in  .
.
We next move to estimate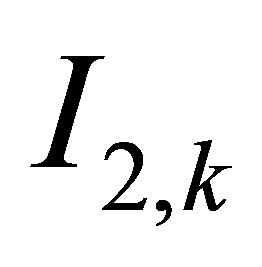 . For sufficiently large positive real number
. For sufficiently large positive real number , we have
, we have
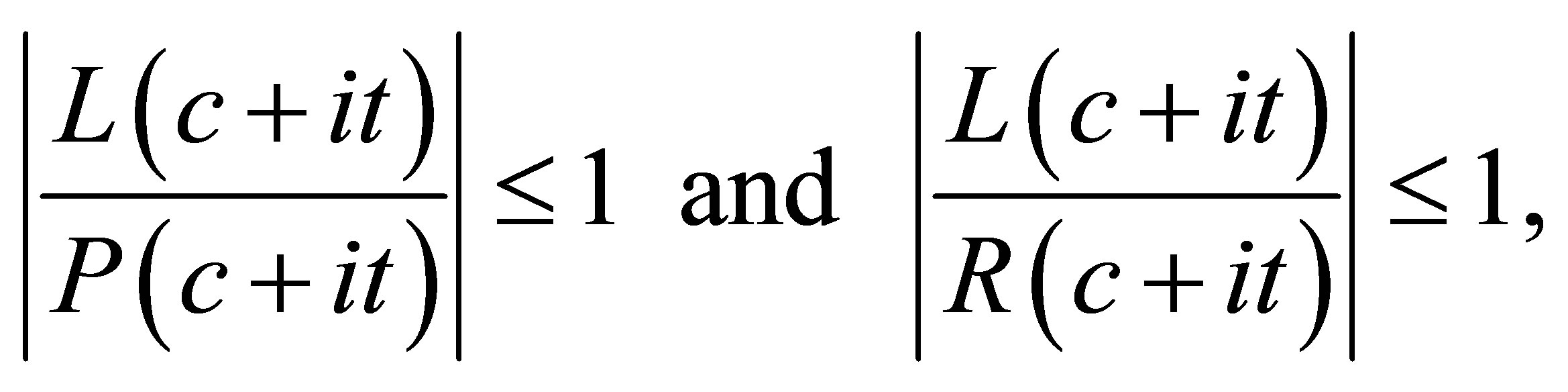 (5)
(5)
so

since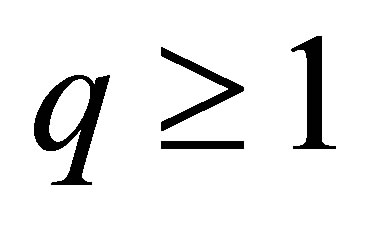 . Furthermore,
. Furthermore,
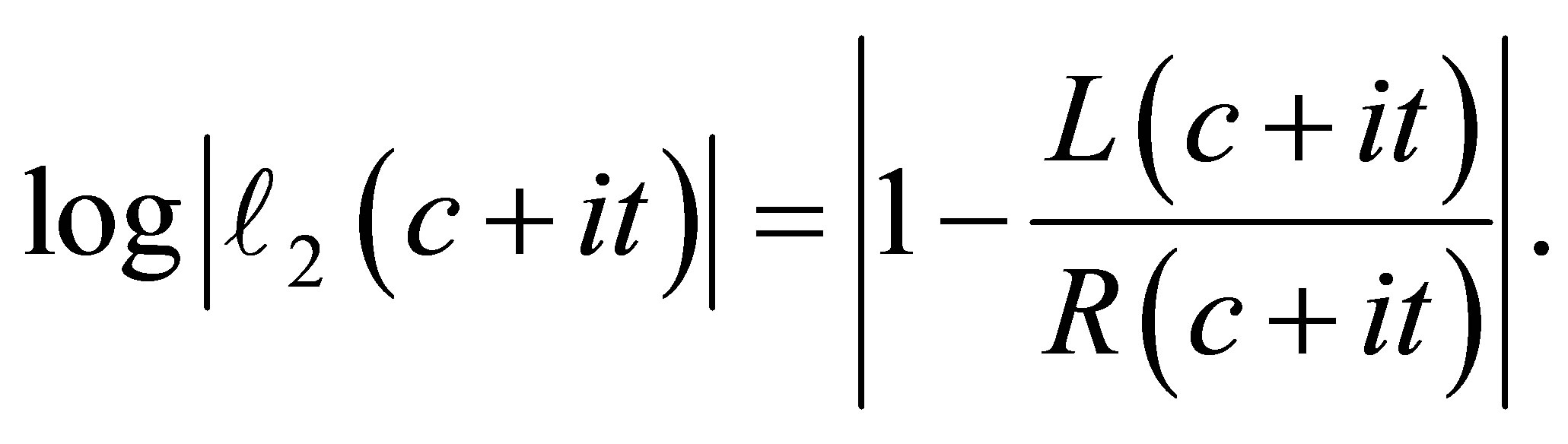
Since we may take  large enough so that
large enough so that
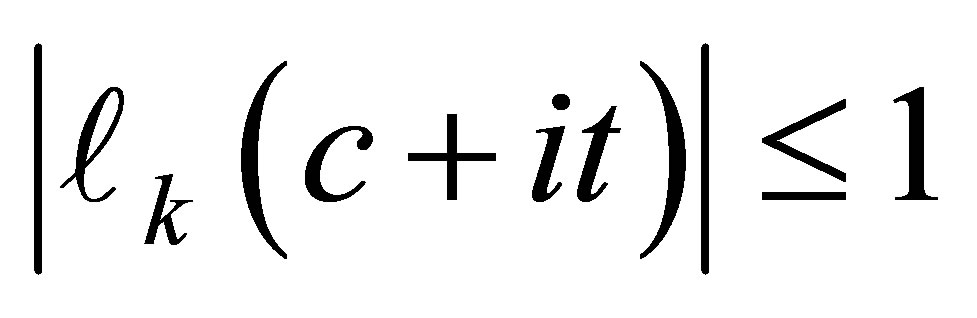 , we may write
, we may write 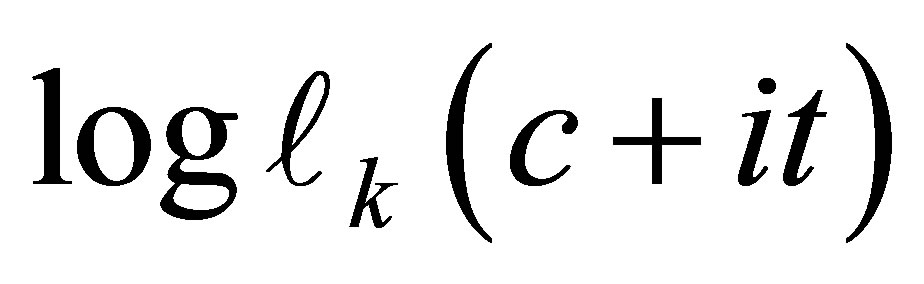 using a Taylor series expansion in the rectangle
using a Taylor series expansion in the rectangle . For
. For , we have after taking real parts that
, we have after taking real parts that

We now observe that for sufficiently large T and some constant M we have
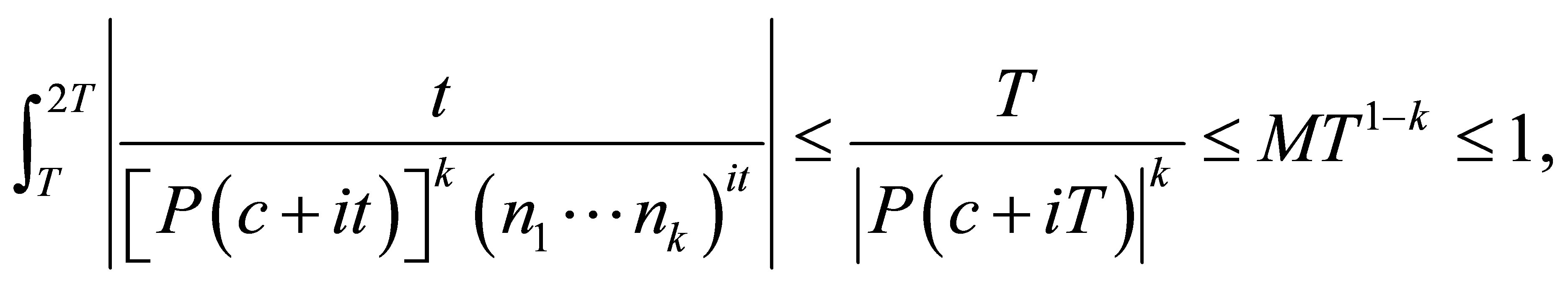
for 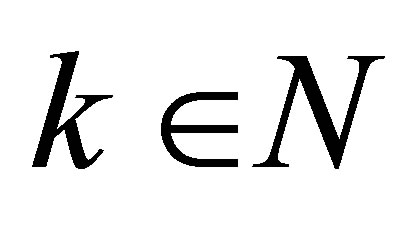 and
and
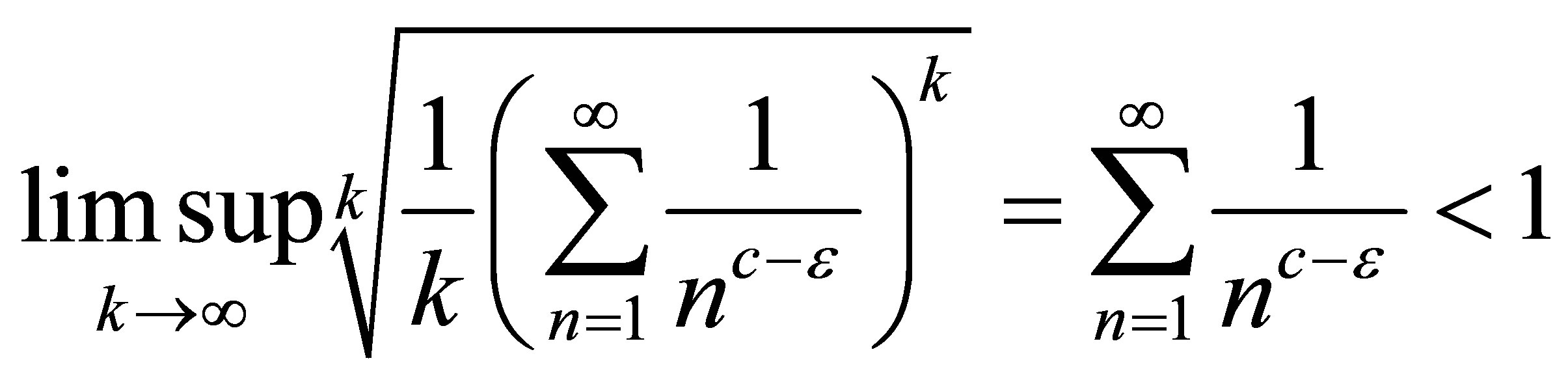
for sufficiently large . In light of these bounds and the definition of
. In light of these bounds and the definition of , we have (6)
, we have (6)
where the last equality holds because  could be sufficiently large. Replacing
could be sufficiently large. Replacing 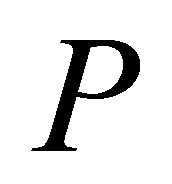 by
by 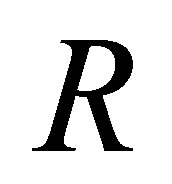 in the above computations, we see analogously that
in the above computations, we see analogously that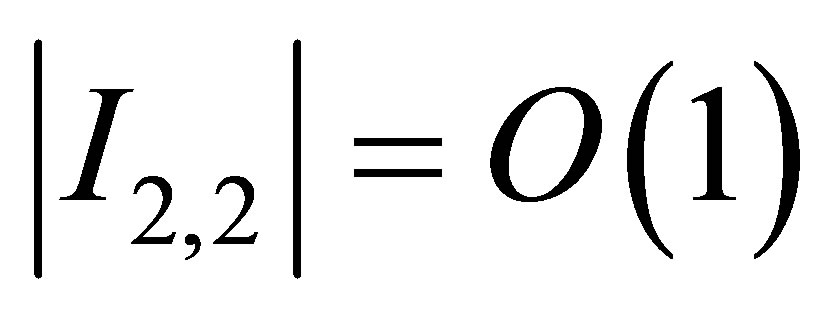 .
.
Finally, we estimate 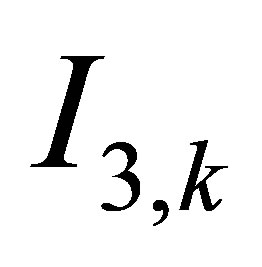 and
and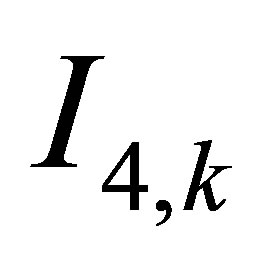 . We show the computation for
. We show the computation for 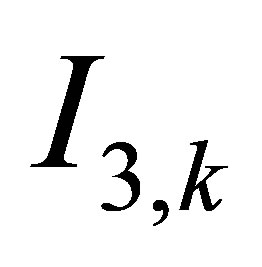 explicitly and note that the bound for
explicitly and note that the bound for  follows analogously. We first suppose that
follows analogously. We first suppose that 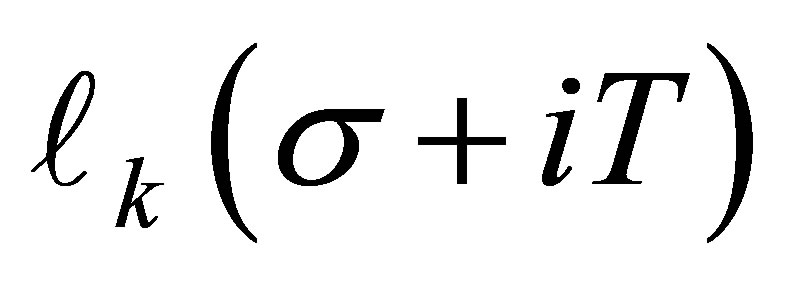 has exactly
has exactly 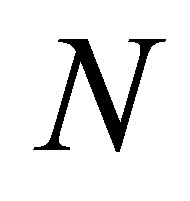 zeros for
zeros for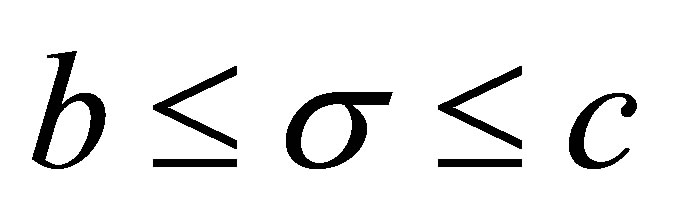 . Then, there are at most
. Then, there are at most 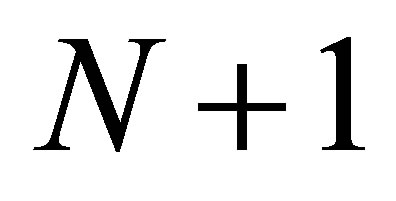 subintervals, counting for multiplicities, in which
subintervals, counting for multiplicities, in which 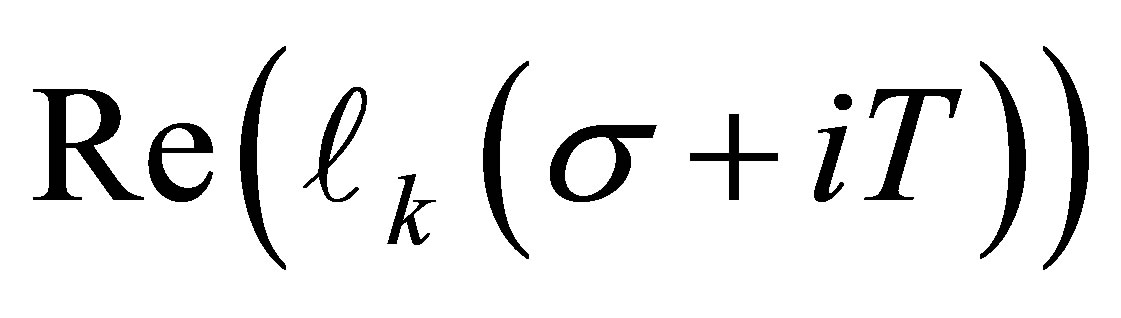 is of constant sign. Thus,
is of constant sign. Thus,
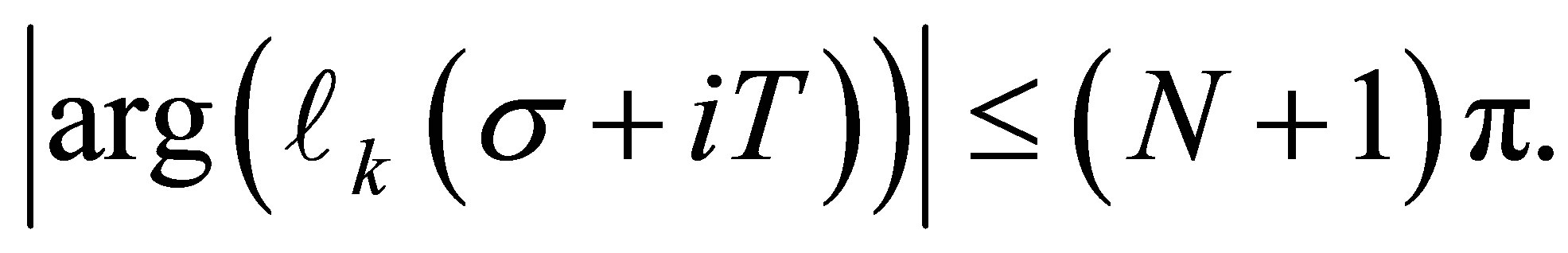 (7)
(7)
It remains to estimate . To this end, we define
. To this end, we define
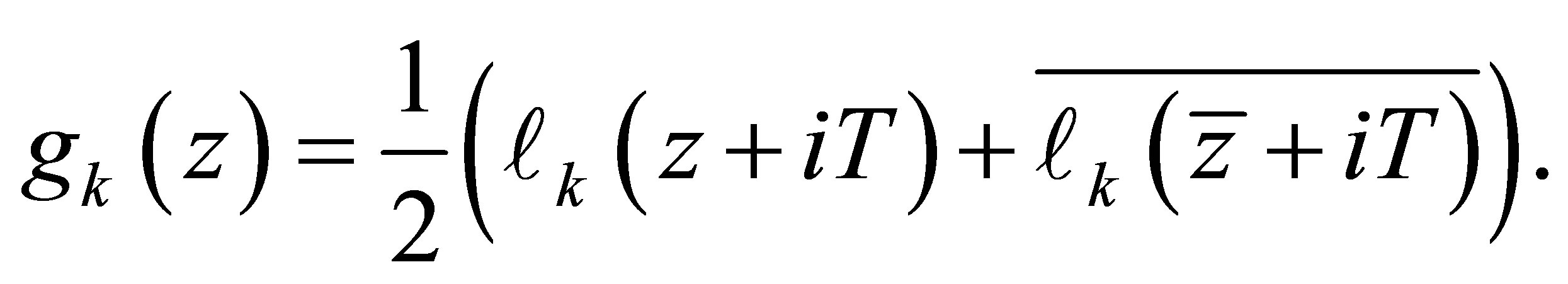
Then
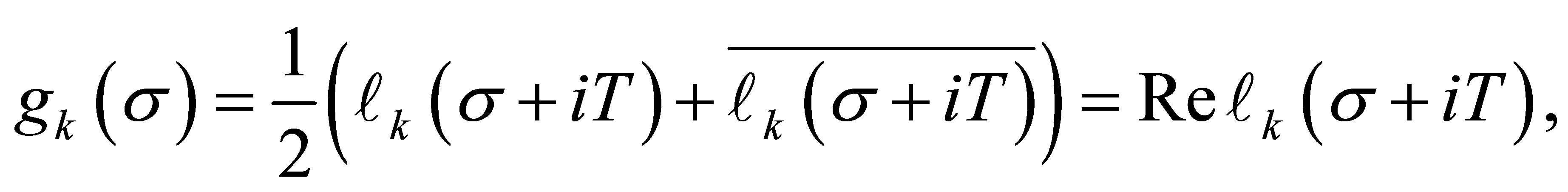
so that if 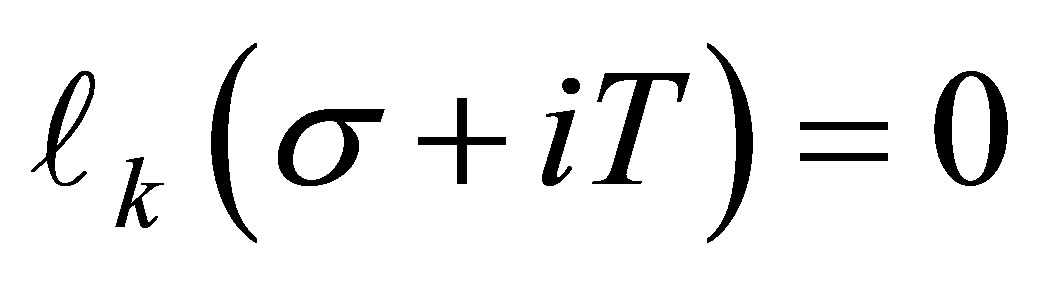 for
for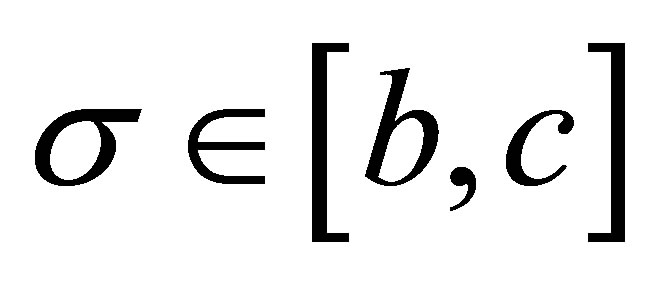 , then
, then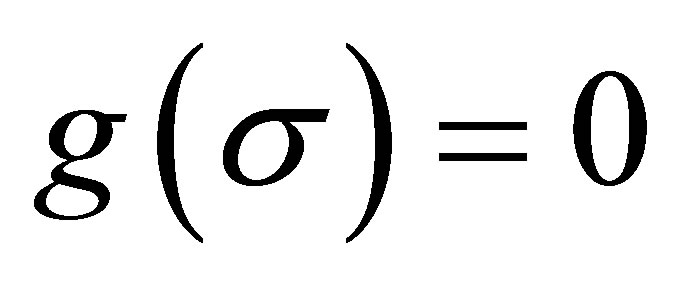 .
.
Now let  and
and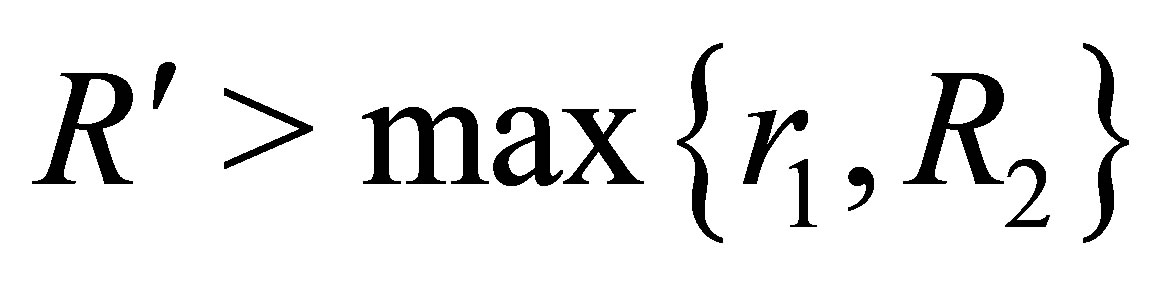 , and choose
, and choose 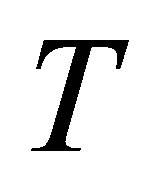 large enough so that
large enough so that . Then
. Then  for
for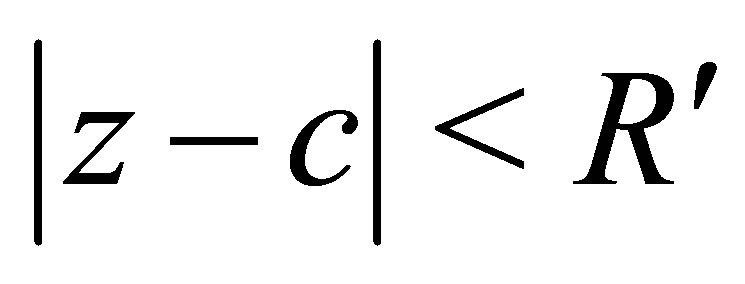 , showing that no zeros or poles of
, showing that no zeros or poles of 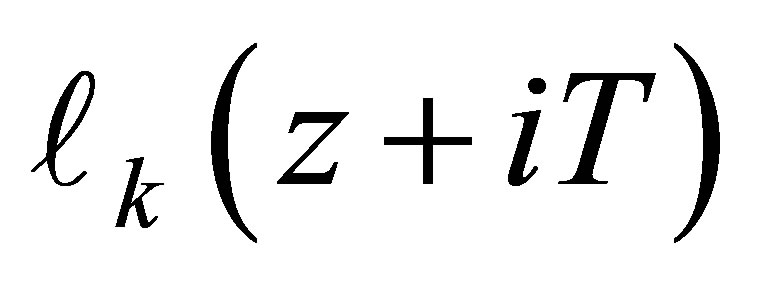 are located in
are located in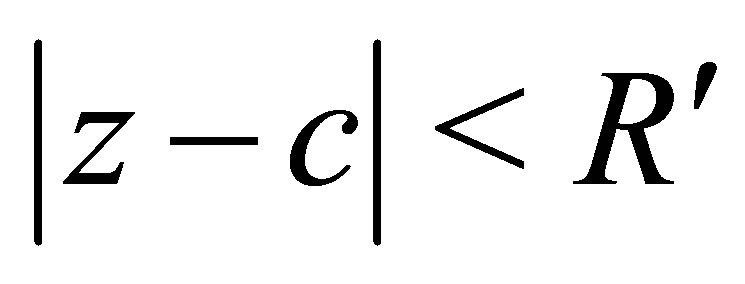 . Thus, both
. Thus, both 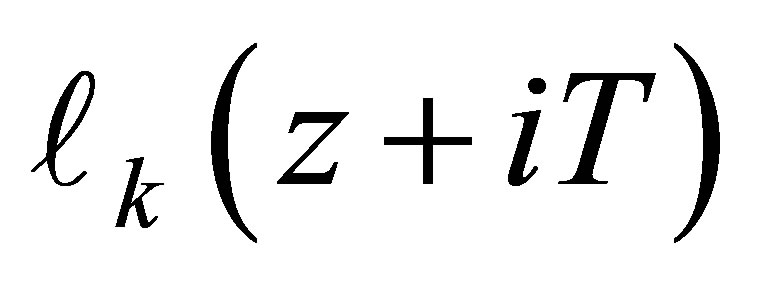 and
and 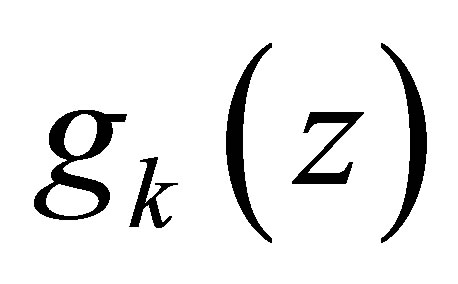 are analytic in
are analytic in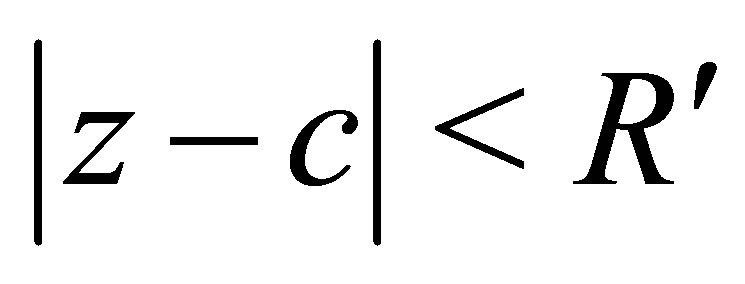 . Letting
. Letting 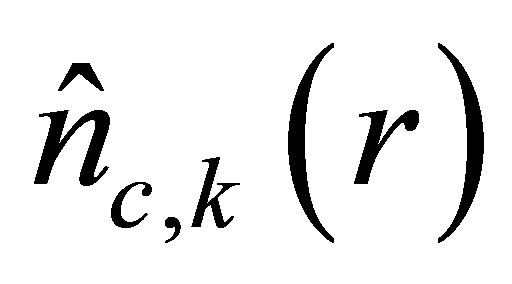 denote the number of zeros of
denote the number of zeros of 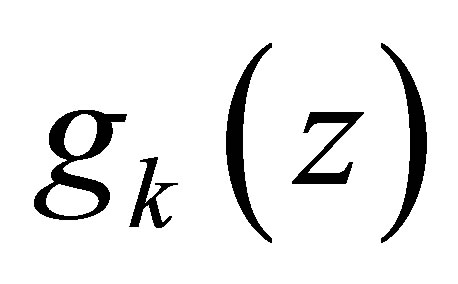 in
in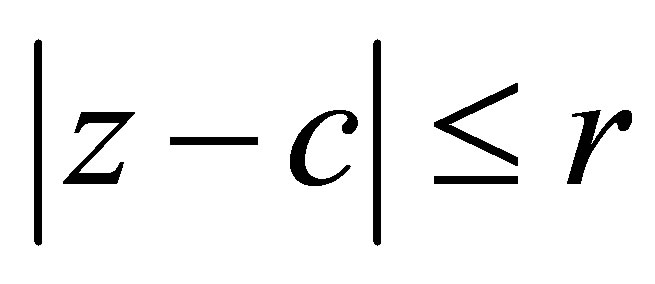 , we have
, we have
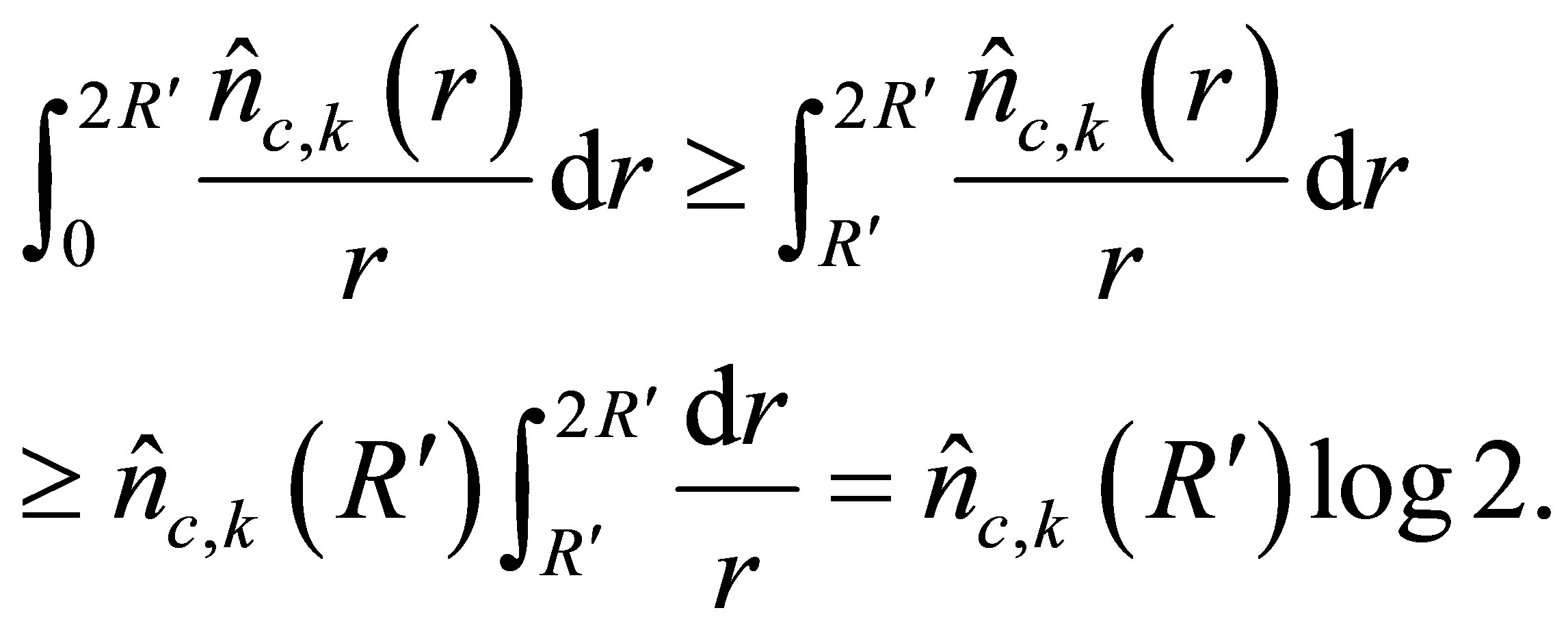
By Jensen’s formula

and so
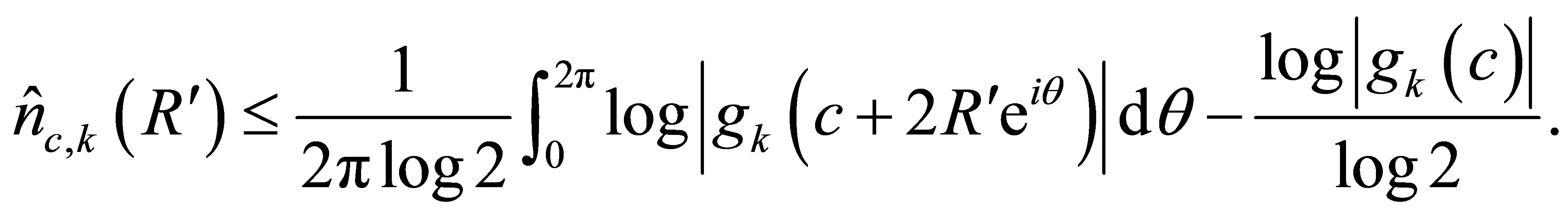 (8)
(8)
| |
By (5), 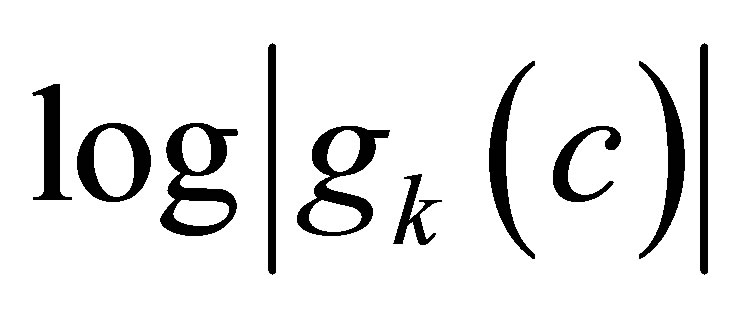 is bounded. Further, it is clear from a property of
is bounded. Further, it is clear from a property of  functions that we have
functions that we have
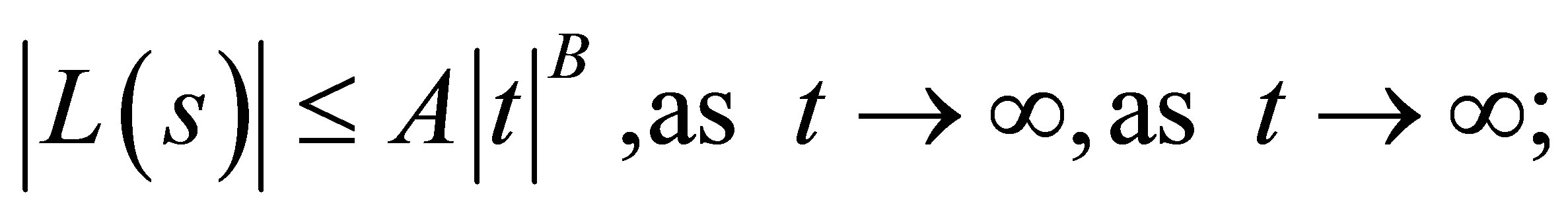
for some positive absolute numbers 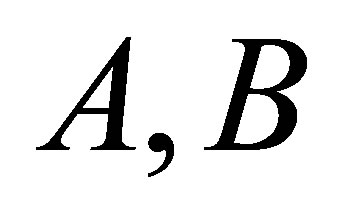 in any vertical strip of bounded width. The same estimate must hold for
in any vertical strip of bounded width. The same estimate must hold for 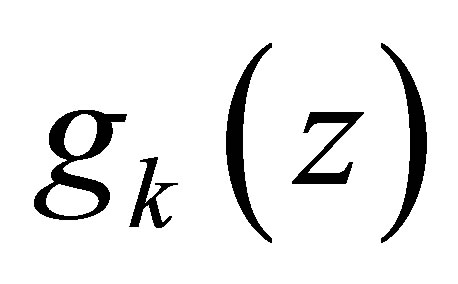 as well. Thus, the integral in (8) is
as well. Thus, the integral in (8) is , implying that
, implying that  . Since the interval
. Since the interval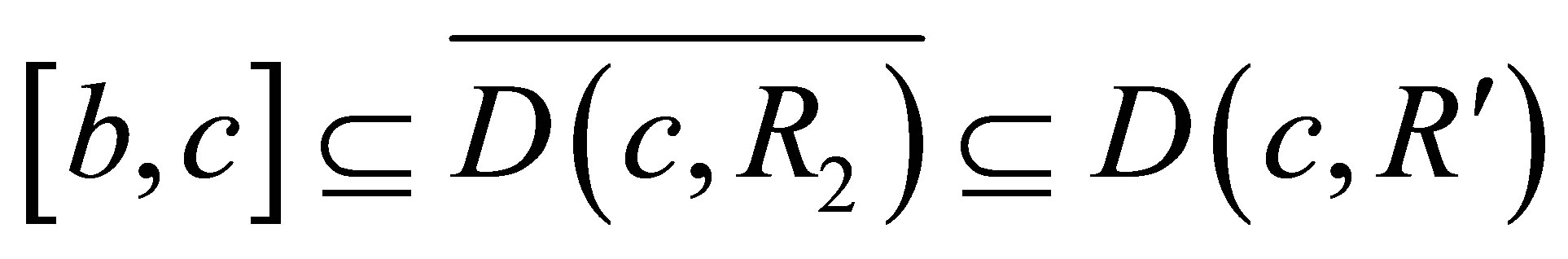 , it follows that
, it follows that
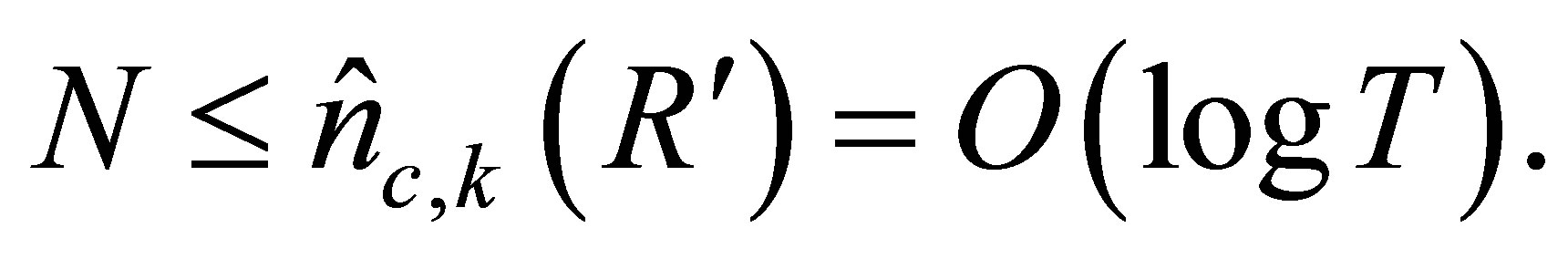
With this bound, we integrate (7) to deduce that
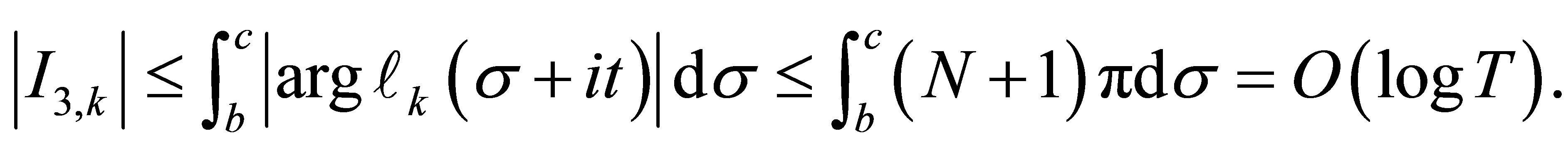
As previously noted, we may bound 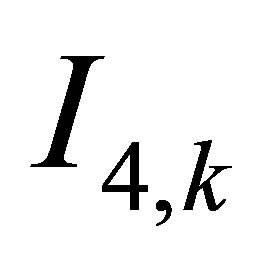 in the same way. Thus, we attain the desired bounds for
in the same way. Thus, we attain the desired bounds for  and
and . Consequently, the first part of the theorem is proved by using (4).
. Consequently, the first part of the theorem is proved by using (4).
Proof of (II). As in the proof of the first part of the theorem, we conclude that there exists a real number 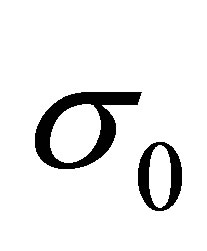 for which the real parts
for which the real parts 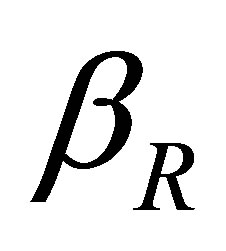 of all
of all 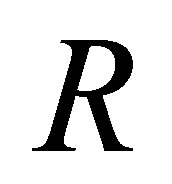 -values satisfy
-values satisfy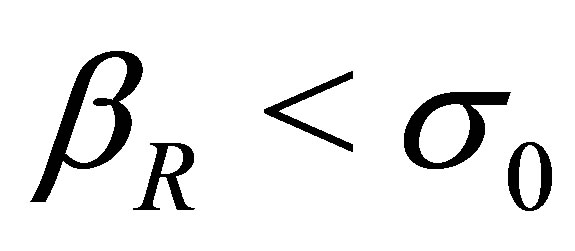 ; and also, there exist
; and also, there exist 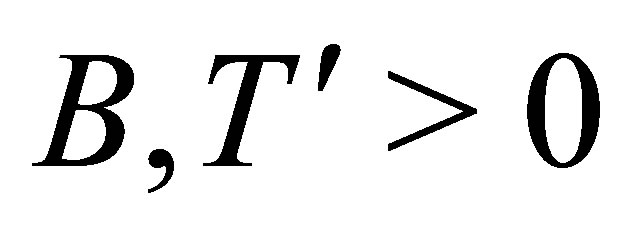 for each rational function
for each rational function 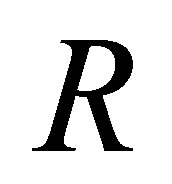 such that no zeros of
such that no zeros of  lie in the quarter-plane
lie in the quarter-plane . As before, we define the rectangle
. As before, we define the rectangle 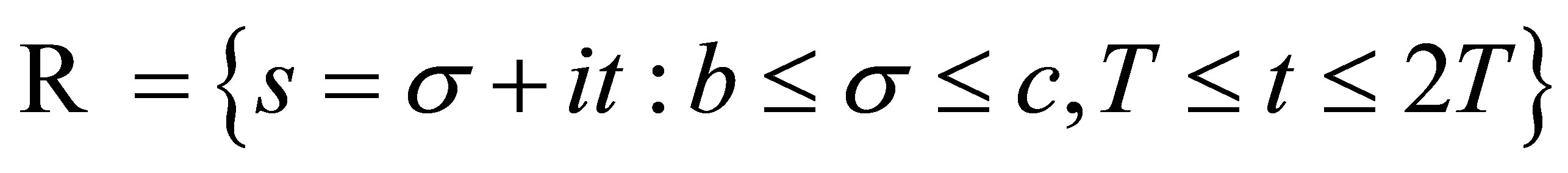 where
where 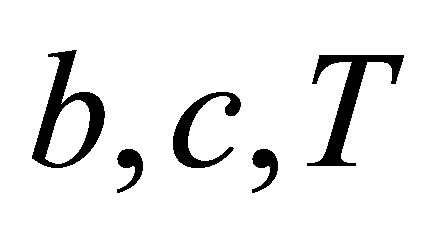 are parameters satisfying
are parameters satisfying 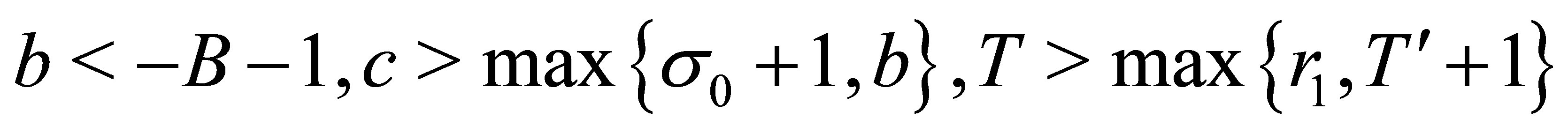 .
.
Proceeding as in the proof of the first part of the theorem, we see that
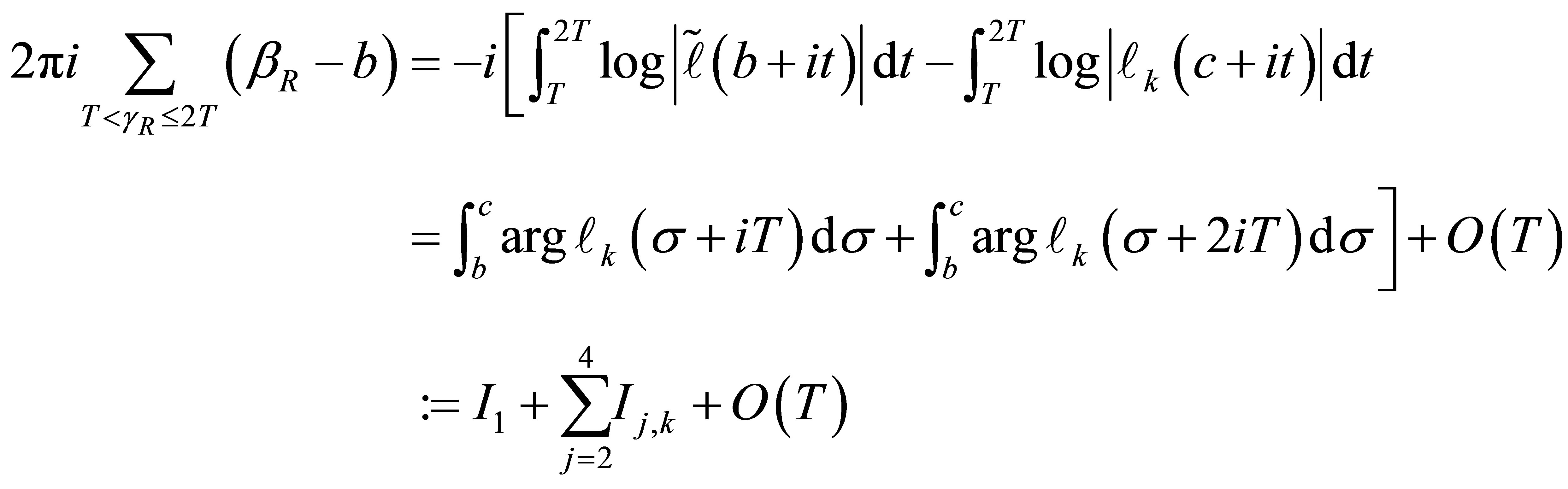
for 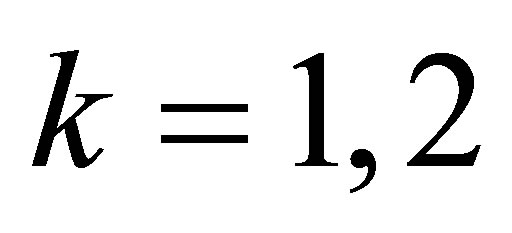 where
where 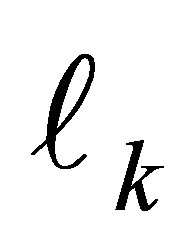 is defined as in (1). In the equation above, we note that we have chosen to compute
is defined as in (1). In the equation above, we note that we have chosen to compute 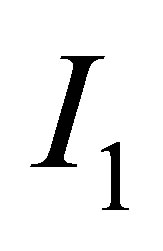 separately. Indeed, this is the only estimate that we will need. For the integrals
separately. Indeed, this is the only estimate that we will need. For the integrals ,
, 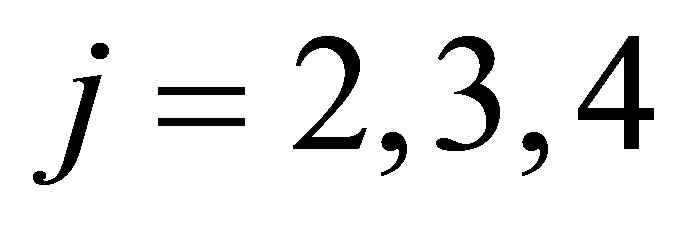 and
and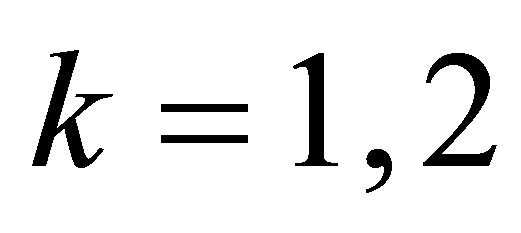 , the bounds given as in the proof of the first part of the theorem still hold. First, integral
, the bounds given as in the proof of the first part of the theorem still hold. First, integral 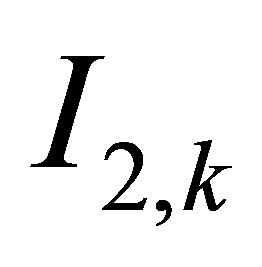 is unchanged. On the other hand, the integrals
is unchanged. On the other hand, the integrals 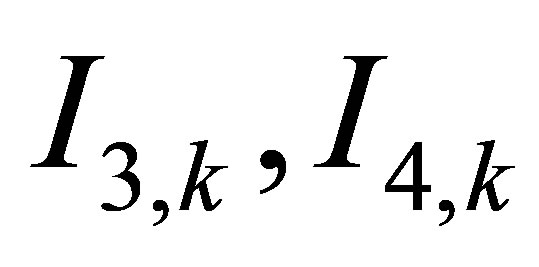 have changed by our choice of
have changed by our choice of , but, as we have done as before, we still have the desired bound since the only requirement is that we consider
, but, as we have done as before, we still have the desired bound since the only requirement is that we consider 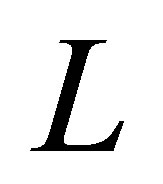
 in a vertical strip of fixed width, which we have in this case.
in a vertical strip of fixed width, which we have in this case.
We now bound . Since
. Since , we have by the functional equation in the definition of
, we have by the functional equation in the definition of 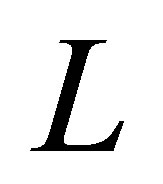 function,
function,
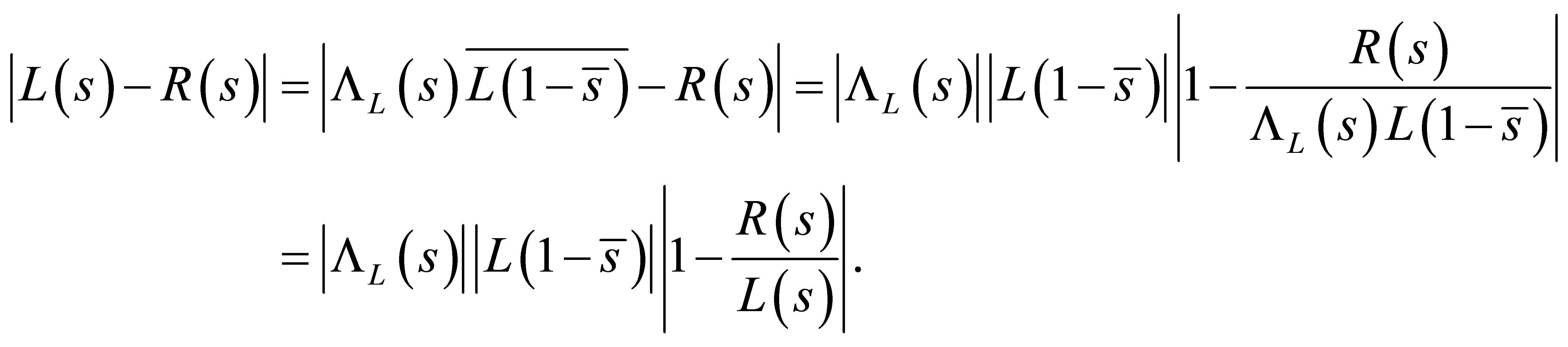
Taking logarithms, we get
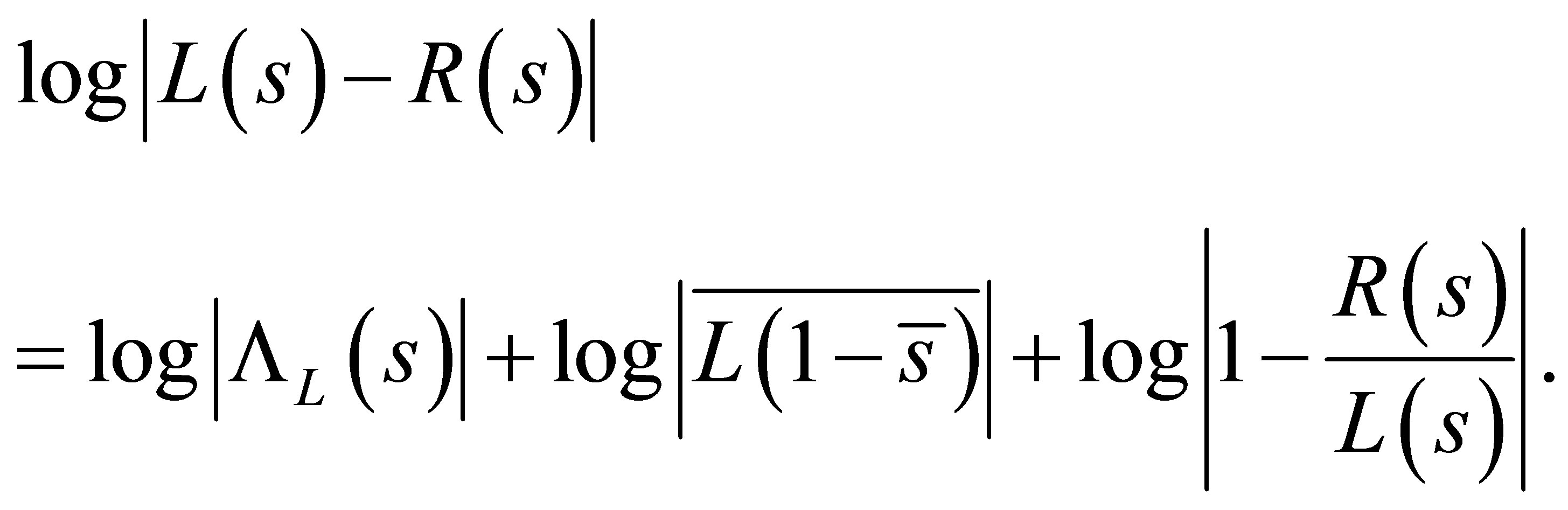 (9)
(9)
Since, for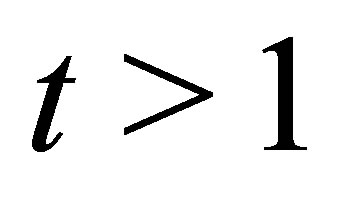 , we have, uniformly in
, we have, uniformly in ,
,
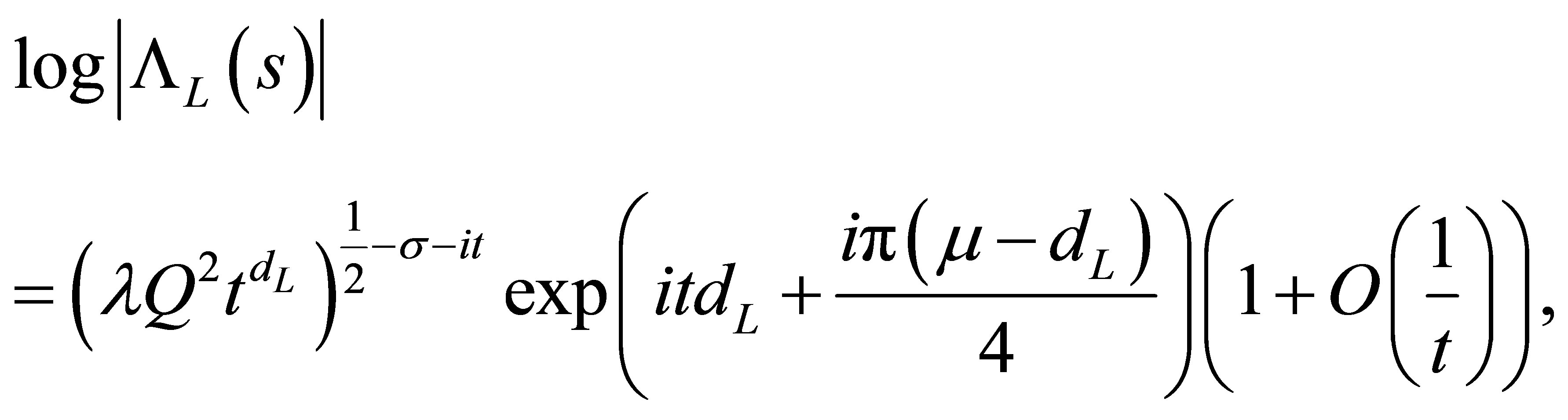
where 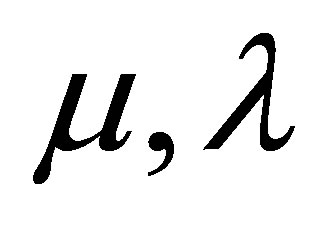 are two constants. It follows, for
are two constants. It follows, for 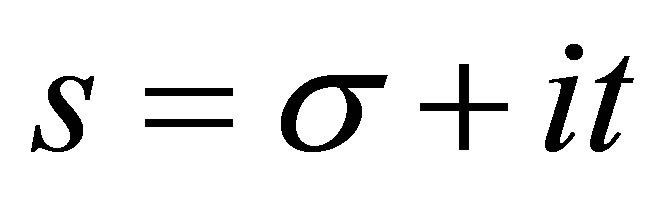 as
as , that
, that

We now consider the last term in (9). Since,
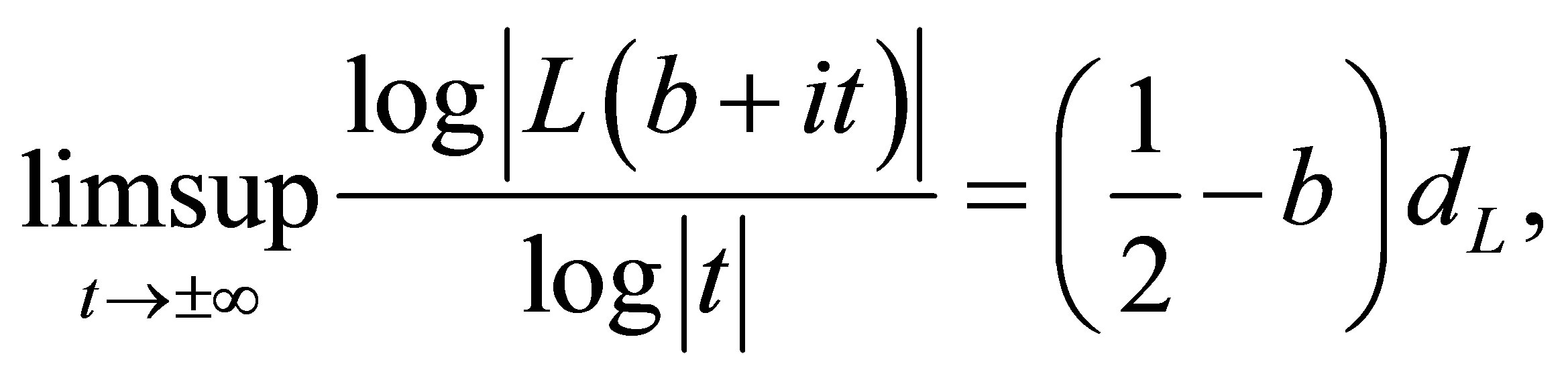
and noting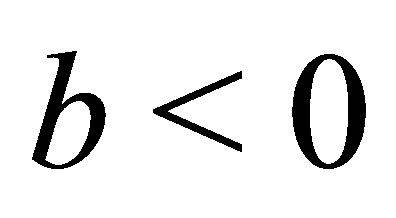 , we have for any
, we have for any  and
and 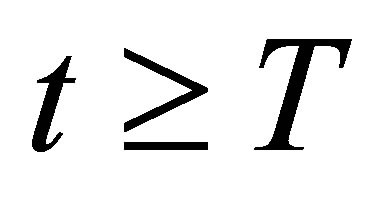
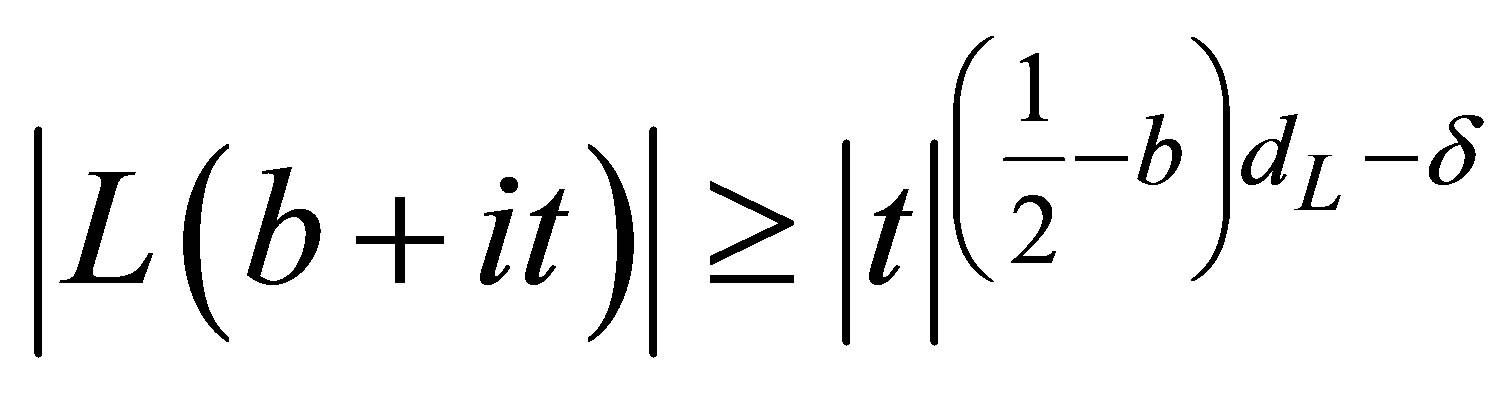
for sufficiently large . Then we see the quotient
. Then we see the quotient

when  is large enough so that
is large enough so that
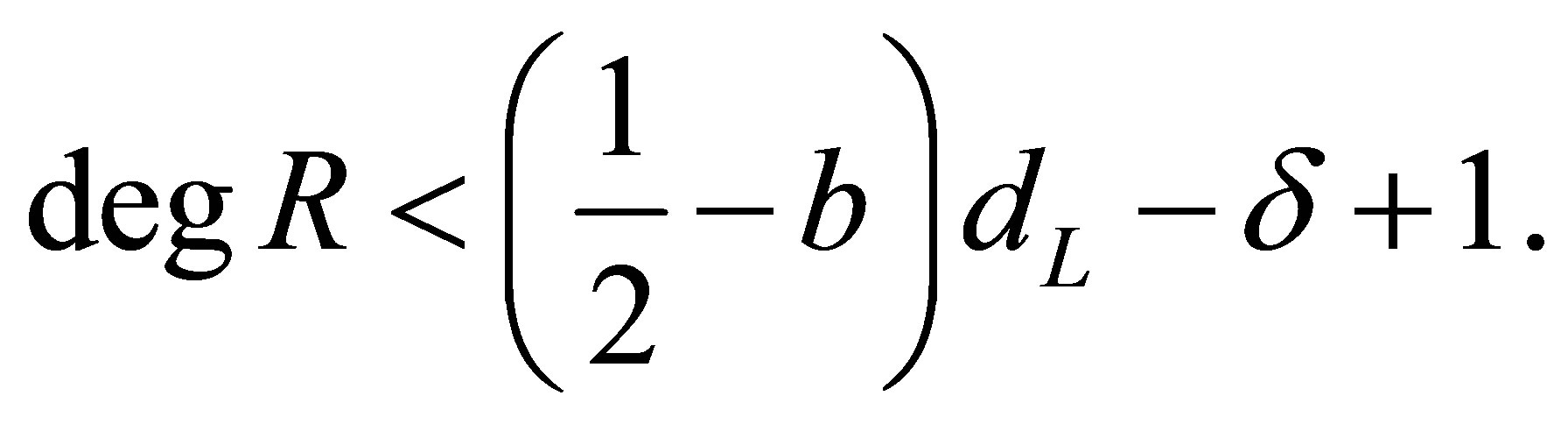
Therefore, we find that

Integrating in light of these estimates, we see
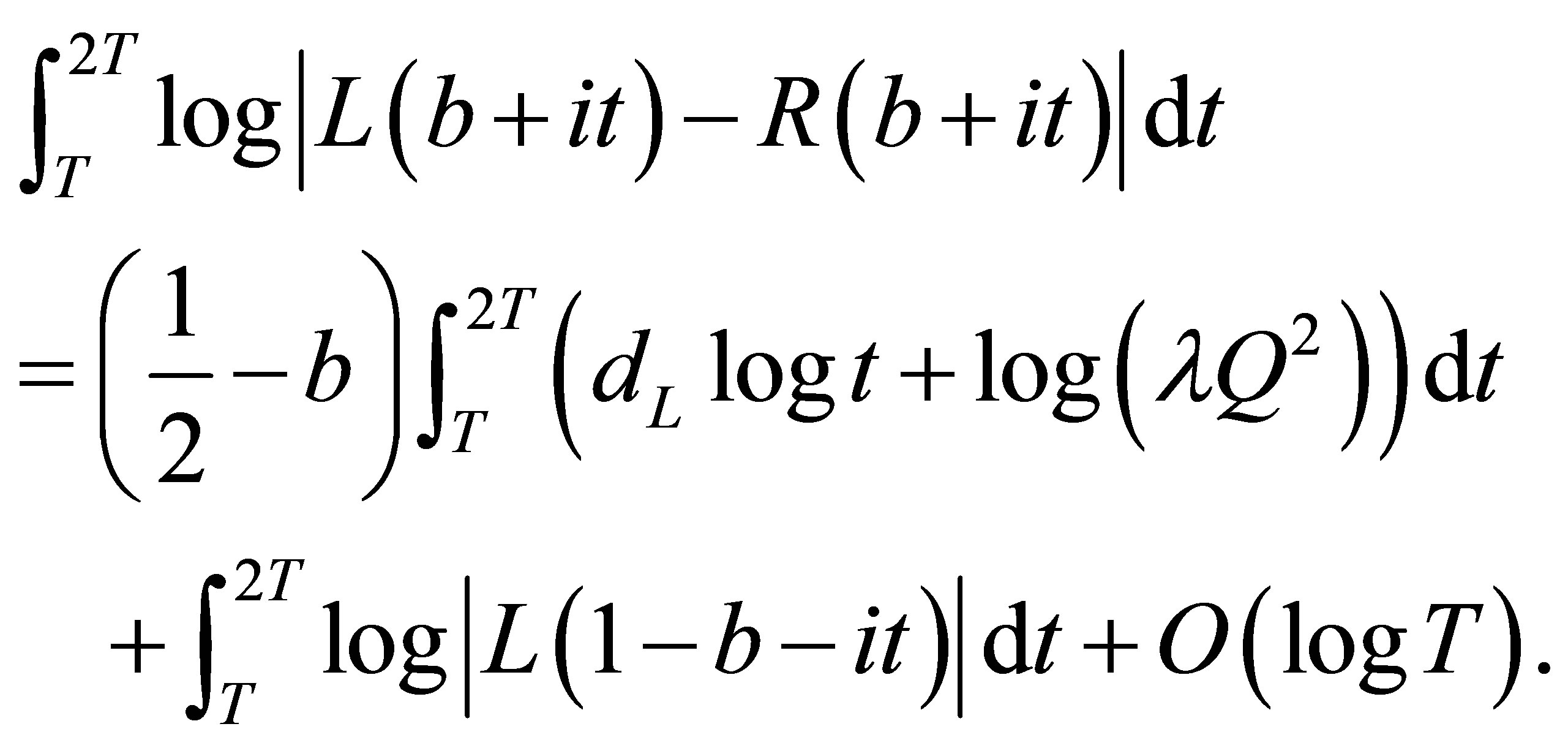
The first integral is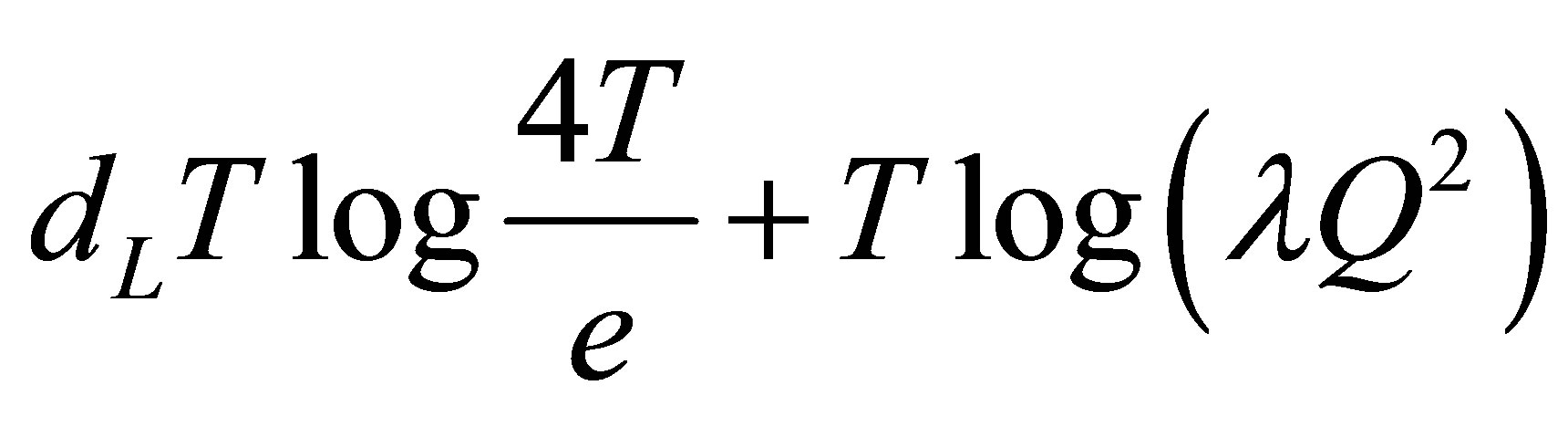 , and the second integral is
, and the second integral is 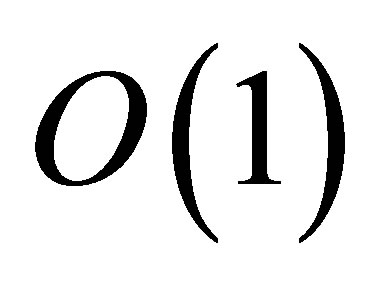 for sufficiently large and negative
for sufficiently large and negative  by the method used to derive (6). Hence,
by the method used to derive (6). Hence,
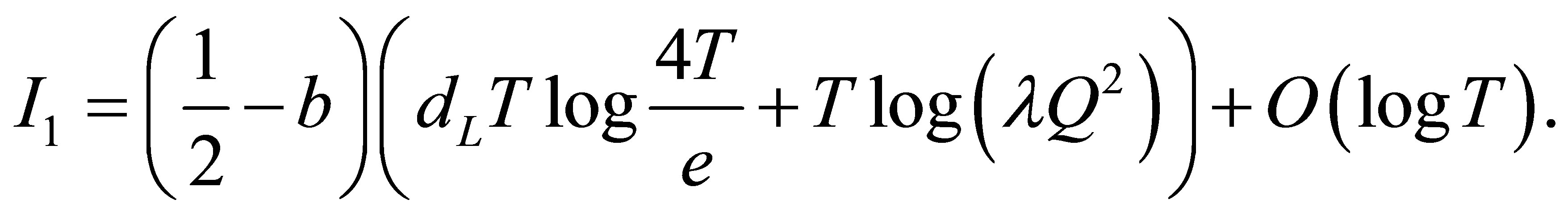
With the estimates for the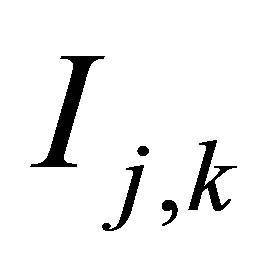 ’s, we have proved the second part of the theorem.
’s, we have proved the second part of the theorem.
REFERENCES
- J. Steuding, “Value Distribution of L-Functions,” Number 1877 in Lecture Notes in Mathematics, Springer, 2007.
- H. S. A. Potter, “The Mean Values of Certain Dirichlet Series I,” Proceedings London Mathematical Society, Vol. 46, No. 2, 1940, pp. 467-468. http://dx.doi.org/10.1112/plms/s2-46.1.467
- E. C. Titchmarsh, “The Theory of Functions,” 2nd Edition, Oxford, 1939.
NOTES
*Corresponding author.