 Applied Mathematics, 2011, 2, 874-882 doi:10.4236/am.2011.27117 Published Online July 2011 (http://www.SciRP.org/journal/am) Copyright © 2011 SciRes. AM On Certain Theta Function Identities Analogous to Ramanujan’s P-Q Eta Function Identities Kaliyur R. Vasuki, Abdulrawf Abdulrahman Abdullah Kahtan Department of St udi es in Mat hemat i c s, University of Mysore, Mysore, India E-mail: vasuki_kr@hotmail.com, raaofgahtan@yahoo.co.in Received February 12, 2011; revised May 23, 2011; accepted M ay 26, 2011 Abstract The purpose of this paper is to provide direct proofs of certain theta function identities analogous to Ra- manujan’s P-Q eta functions identities. Keywords: Eta Function Identities, Theta Function, P-Q Modular Equations 1. Introduction In the unorganized pages of his second notebook [1,2], Ramanujan recorded 23 identities involving ratio of Dedekind’s eta function of which have been proved by B. C. Berndt and L.-C. Zhang [3] by employing Ramanu- jan’s modular equations of various degree, or via his mixed modular equations or via the theory of modular forms. Similar 14 identities involving ratio of Dede- kind’s eta function found on page 55 of Ramanujan’s lost notebook [4] have been proved by Berndt [5] employing the above methods. Berndt and H. H. Chan [6], Berndt, Chan and Zhang [7], have employed some of the above mentioned P-Q modular equations for the explicit evaluation of Rogers-Ramanujan’s continued fractions, and Ramanujan-weber-class invariants. Motivated by their works, several new P-Q eta functions identities have been discovered and employed them in finding the explicit evaluation of continued fractions, class invariant, and ratio of theta functions by many mathematics. For example see [8-20]. The purpose of this paper is to provide direct proofs of some of P-Q eta functions identities. In Section 2 of this paper, we found convenient to gather some definitions and lemmas which are required to prove P-Q eta function identities. In Section 3, we derive some P-Q eta function identities. 2. Preliminary Results First we shall provide some useful notations and definition. In Chapter 16 of his second notebook [2,21,22] Ra- manujan develops theory of theta function and his theta function is defined by theta function and his theta func- tion defined by 11 22 ,: ,,,,ab nn nn n faba b a abb abab ab 1, . where we employ the customary notation 1 1 ;1 k k ab aq If we set , and π where z is complex and Im(j) > 0, then f (a, b) = Ԃ 3 (z, j), where Ԃ 3 (z, j) denote the classical theta function in its standard notation [23]. Following Ramanujan, we define 2iz aqe2iz bqe , ij qe 22 222 3 11 222 2 0 2 312 0 :, ;; :, ;; :, 1(;) n n nn n nnn n qfqq qqqqq qfqq qqqqq fq fqq qqq , , and 2 . :;qqq For convenience, we denote n q by n for positive integer n. It is easy to see that 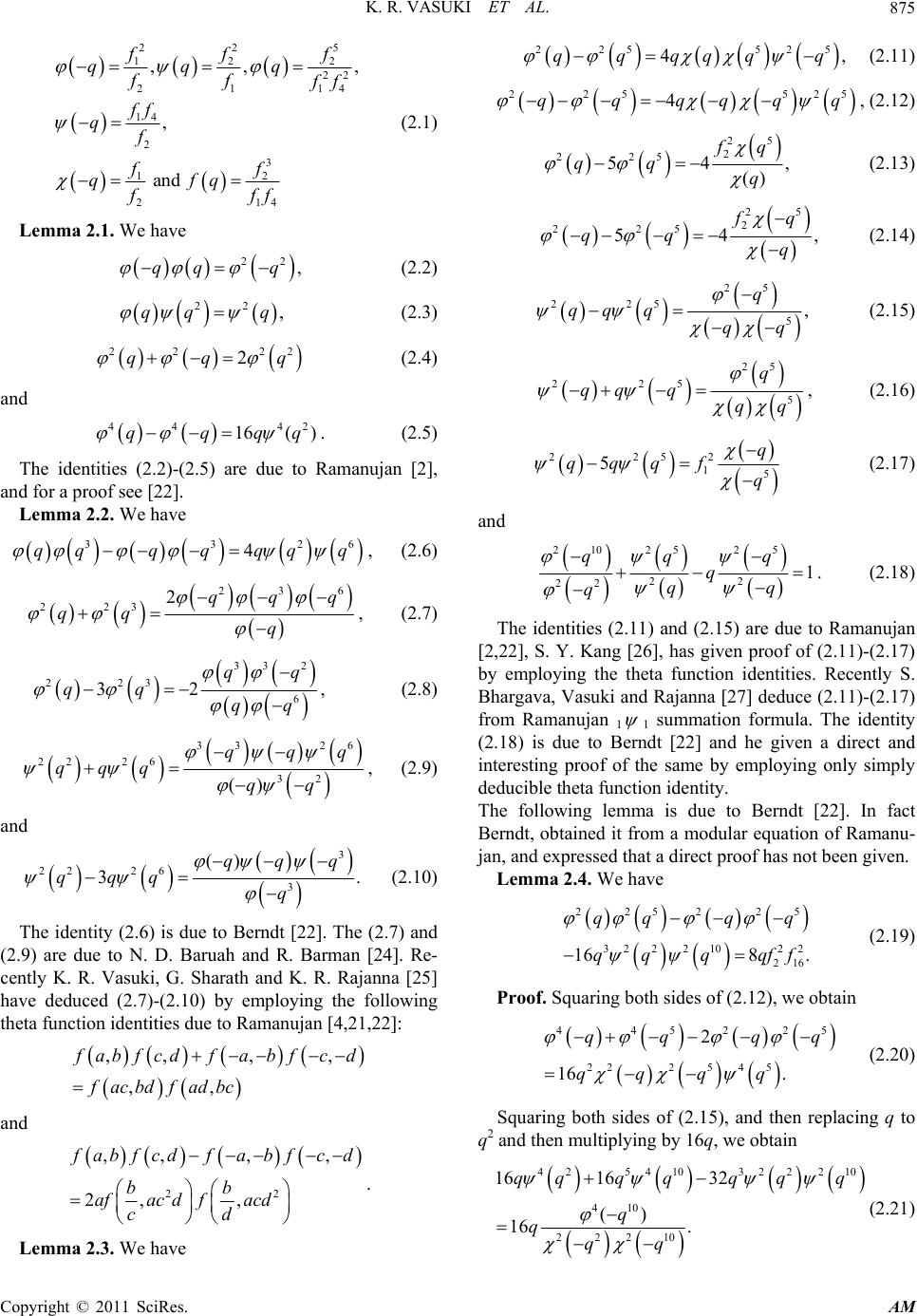 K. R. VASUKI ET AL.875 22 12 22 21 14 14 2 3 12 214 ,, , and ff qqq ff 5 2 , f f ff qf ff qfq fff (2.1) Lemma 2.1. We have 22 ,qqq (2.2) 22 ,qq q (2.3) 22 2 2qq 2 q 2 q 6 (2.4) and 44 4 16( )qqq . (2.5) The identities (2.2)-(2.5) are due to Ramanujan [2], and for a proof see [22]. Lemma 2.2. We have 332 4qqqqqqq , (2.6) 236 223 2qqq qq q , (2.7) 33 2 223 6 32 qq qq qq , (2.8) 33 26 22 26 32 () qqq qqqqq , (2.9) and 3 22 26 3 () 3. (2.10) qqq qqq q The identity (2.6) is due to Berndt [22]. The (2.7) and (2.9) are due to N. D. Baruah and R. Barman [24]. Re- cently K. R. Vasuki, G. Sharath and K. R. Rajanna [25] have deduced (2.7)-(2.10) by employing the following theta function identities due to Ramanujan [4,21,22]: ,,, , ,, ab f cdfabfcd facbdfadbc and 22 ,,, , 2, , ab f cdfab fcd bb afac dfacd cd . Lemma 2.3. We have 225 52 4,qqqqqq 5 (2.11) 225 52 4qqqqq 5 q, (2.12) 25 2 225 54 () , q qq q (2.13) 25 2 225 54, q qq q (2.14) 25 225 5, q qq qqq (2.15) 25 225 5 q qq qqq , (2.16) 2252 15 5q qqq fq (2.17) and 210 2525 22 22 1 qq q q qq q . (2.18) The identities (2.11) and (2.15) are due to Ramanujan [2,22], S. Y. Kang [26], has given proof of (2.11)-(2.17) by employing the theta function identities. Recently S. Bhargava, Vasuki and Rajanna [27] deduce (2.11)-(2.17) from Ramanujan 1 1 summation formula. The identity (2.18) is due to Berndt [22] and he given a direct and interesting proof of the same by employing only simply deducible theta function identity. The following lemma is due to Berndt [22]. In fact Berndt, obtained it from a modular equation of Ramanu- jan, and expressed that a direct proof has not been given. Lemma 2.4. We have 2252 2 32221022 216 168 . qqq q qqq qff 5 (2.19) Proof. Squaring both sides of (2.12), we obtain 44522 22254 5 2 16 . qq q qqqq 5 q (2.20) Squaring both sides of (2.15), and then replacing q to q2 and then multiplying by 16q, we obtain 4254 103222 10 410 22210 16 1632 () 16 . qqqqqq q q qqq (2.21) Copyright © 2011 SciRes. AM  K. R. VASUKI ET AL. 876 Adding (2.20) and (2.21) and then employing (2.5), we deduce that 44522 3222 10 2254 410 22210 252 10 22 210 222 2 32 16 16 qq qq qq q qq qqq q qq qq qf fqqq 5 5 upon using (1.1). Now employing (2.18) and then using (2.16), in the right side of the above, we obtain 44522 322210 225 2520 14 2 32 16 . qq qq qq q fffq qff 5 (2.22) From (2.11) and (2.13), it follows that 2222 52 52 210 165 ,qf fqqqq which is equivalent to 2222 5 210 44522 16 2 54 qf fqq qq qq 5 . (2.23) From (2.23) and (2.22), we deduce that 2222 5 210 322210225 22 2520 22545 14 2 25 2252520 14 16 2 32 2 4416 44 qf fqq qq qqq 5 0 ff q qqq q ff fff qqqq ff upon using (2.11) and (1.1), This completes the proof. 3. P-Q Eta Function Identities Lemma 3.1. Let 2 :, q Pq and 2 :. q Qq Then, 4 2 2 1PQ . (3.1) Proof. We have 442 4 42 2 22 42 222 42 22 2 2 1 2 2 2 qq Pq qqq q qq q q q Q where we have used (2.2) and (2.4). This completes the proof. Theorem 3.1. Let 2 :, q Pq and 3 6 :q Qq . Then, 44 2 2 1 64 . PQ PQ QP PQ (3.2) Proof. By (2.2) and (2.6), 23 2 2226 3 3 3 2 3 6 3 () 111 () ()( ) () 4. qq qq PQ qq qq qqqq qq qq q qq 3 Taking forth power on both sides and then employing (2.5), we have 42 46 4 23 443 43 4 443 83 8 8286 8 11616 11 () 11 11, qq PQ qq qq q q qq q q qq PQ Copyright © 2011 SciRes. AM  K. R. VASUKI ET AL.877 . where, we have used (2.2). Thus, we have 4 288 111PQP Q Now expanding both sides and then dividing through- out by (PQ)4, we obtain the required result. Corollary 3.1. Let 3 26 :,and:. q q PQ qq Then, 42 42 2 2 36 2 4816 138. PQ PQ QPQP PQ PQ PQ PQQ PPQ 4 Proof. Squaring both sides of (3.2) and then employ- ing (3.1) and after some simplification, we obtain the required result. Corollary 3.2. Let 3 3 : and : q q PQ qq . Then, 221 64 . PQ PQ QP PQ Proof. We have 2 22 . qq q q Using this in (3.2), we obtain the required result. Corollary 3.1 and 3.2 are due to Vasuki and Srivatsa- kumar [19]. Theorem 3.2. Let 3 :, q Pq and 2 6 :q Qq . Then, 2 2 2 2 3. QP QPQ Q (3.3) Proof. By (2.7), (2.8) and (2.2), 23 2 2 2 2 223 223 42 22 4 2323 46 3( 3 1 ()(). ()() qq q P PPqqq q qq Q qq q The required result follows from the above after some algebraic manipulations. Corollary 3.3. Let 33 : and :. qq PQ qq Then, 3. QP PQ PQP Q (3.5) Proof. We have from (2.2) 2 6. qPQ q Using this in (3.3), we obtain (3.5). Theorem 3.3. Let 2 36 : and : q q PQ qq . Then, 2 2 22 93 2 12. PQ PQPQ PQQ P PQ PQ QP (3.6) Proof. By (2.2), (2.7) and (2.8), 23 2 2 2232 22 3 32 6 2 22 3() 3 1 () () () . qq P Pqq qqq qq q q q (3.7) Changing q to in (3.7), we obtain 2 q 22 2 226 3. 1 q Q Qq (3.8) From (3.7), (2.2) and (3.8), we deduce that 22 2 2 223 23 4 22 26 2 2 2 3 1 3. 1 qq P PPqq q q Q Q ) (3.4) Copyright © 2011 SciRes. AM  K. R. VASUKI ET AL. 878 Thus, we have 2 22 2 22 33 , 11 PQ PPQ which is equivalent to (3.6). Identities (3.3), (3.5) and (3.6) are due to Bhargava et al. [12]. Theorem 3.4. Let 3 13 2 88 : and :. q q PQ qq qq 6 Then, 44 2 2 16 49. (3.9) PQ PQ QP PQ Proof. By (2.3) and (2.5), 8 8 82 4 42 4 42 () 16 16 () 16 () . q Pqq q qq q qq (3.10) Changing q to in (3.10), we have 3 q 43 8 34 6 16 . q Qqq (3.11) By (2.3) and (2.6), 223 2 22 26 3 226 3 226 4 4 qq PQ qq q qq qq q qq qq q 4 From (3.10), (3.11) and the above, it is easy to see that 4 88 2 1616() 4PQ PQ , which equivalent to (3.9). Corollary 3.4. Let 3 13 26 44 : and : q q PQ qq qq . Then, 22 16 49 PQ PQ PQQ P . (3.12) Proof. By from (2.3), we have 2 11 22 2 44 , qq qqqq using this in (3.9), we obtain (3.12). The identity (3.12) is due to Vasuki and Srivatsakumar [18]. Theorem 3.5. Let 2 11 36 42 () : and :. q q PQ qq qq Then, 2 2 2 2 3. QP PPQP (3.13) Proof. By (2.3), (2.9) and (2.10), we have 22 243 2 2 2264326 22 2 26 23 4 43 4 3 1 () () . qqq Q QQqqq q qq qq q q qq P This is equivalent to (3.13). Theorem 3.6. Let 5 21 () : and :. q q PQ qq 0 Then, 33 2 2 1 45 PQ PQ PQ QP QP PQ . (3.14) Proof. By (2.2) and (2.5), 8 8 82 4 4 42 4 11 1 16 . q Pq q q qq q (3.15) Copyright © 2011 SciRes. AM  K. R. VASUKI ET AL.879 Changing q to in (3.15), we obtain 2 q 54 10 8 45 16 1 qq Qq . (3.16) From (3.15) and (3.16), we have 3222 10 88 225 16 11 qqq PQ qq . (3.17) By (2.2), we have 445225 4 42410225 . ()() qq qq PQ qq qq (3.18) By (2.1), (3.17) and (3.18), we deduce that 12 414 520 88 3 24 210 11 16 ffff PQPQq ff . (3.19) By (2.1), (2.19), (3.17), and (3.18), we also deduce that 488 225225 32221 225 22 210 225 4 14520 8 210 111 16 ()() 8 ()() 8. PQPQ qqqq qq q qq qf f qq ffff qff 0 (3.20) From (3.19) and (3.20), we obtain 3 488 488 111 3211 , PQP Q PQP Q which implies 32 422 3, 32 3 AAB B PQ BA where 4 1 PQ and 88 11BPQ . 0, 6 6 , Squaring the above on both sides and then factoring using maple, we obtain ,,,CPQDPQEPQ where 5 62442 5 62442 ,5544 ,5544 C PQPPQPQPQPQQ D PQPPQPQPQPQQ and 10 1210 28 4 648 210 212 ,101615 2015 10 16 . EPQPPQPQPQ PQPQPQ PQ Q It is easy to see that, P and Q have the following series expansion 23456 12 246812Pqqqqqq and 510152025 30 12 246812Qqqqqq q Using these in C(P,Q), D(P,Q) and E(P,Q), we obtain 11 121314 , 2560 1376049600149760 CPQ qqq q 23 45 , 64284 14084352 12096 DPQ qq qqq and 23 4 , 64 768q 537628672129024 EPQ qq q One can see that q–1 D(P,Q) and q–1 E(P,Q) does not tends to 0 as q tends to 0, whereas q–1C(P,Q) tends to 0 as q tends to 0. Hence q–1C(P,Q) = 0 in some neighbor- hood q = 0. By analytic continuation q–1C(P,Q) = 0 in 1q . Thus we have ,0CPQ. Dividing the above throughout by , we obtain (3.14). 3 PQ Corollary 3.5. Let 5 5 : and : q q PQ qq . Then, 31 31 22 22 1 45. PQ PQ PQ PQQP QP Corollary 3.5 follows from (2.2) and (3.14). The fol- lowing theorem is due to Adiga et al. [9]. Theorem 3.7. Let 2 21 : and :. q q PQ qq 0 Copyright © 2011 SciRes. AM 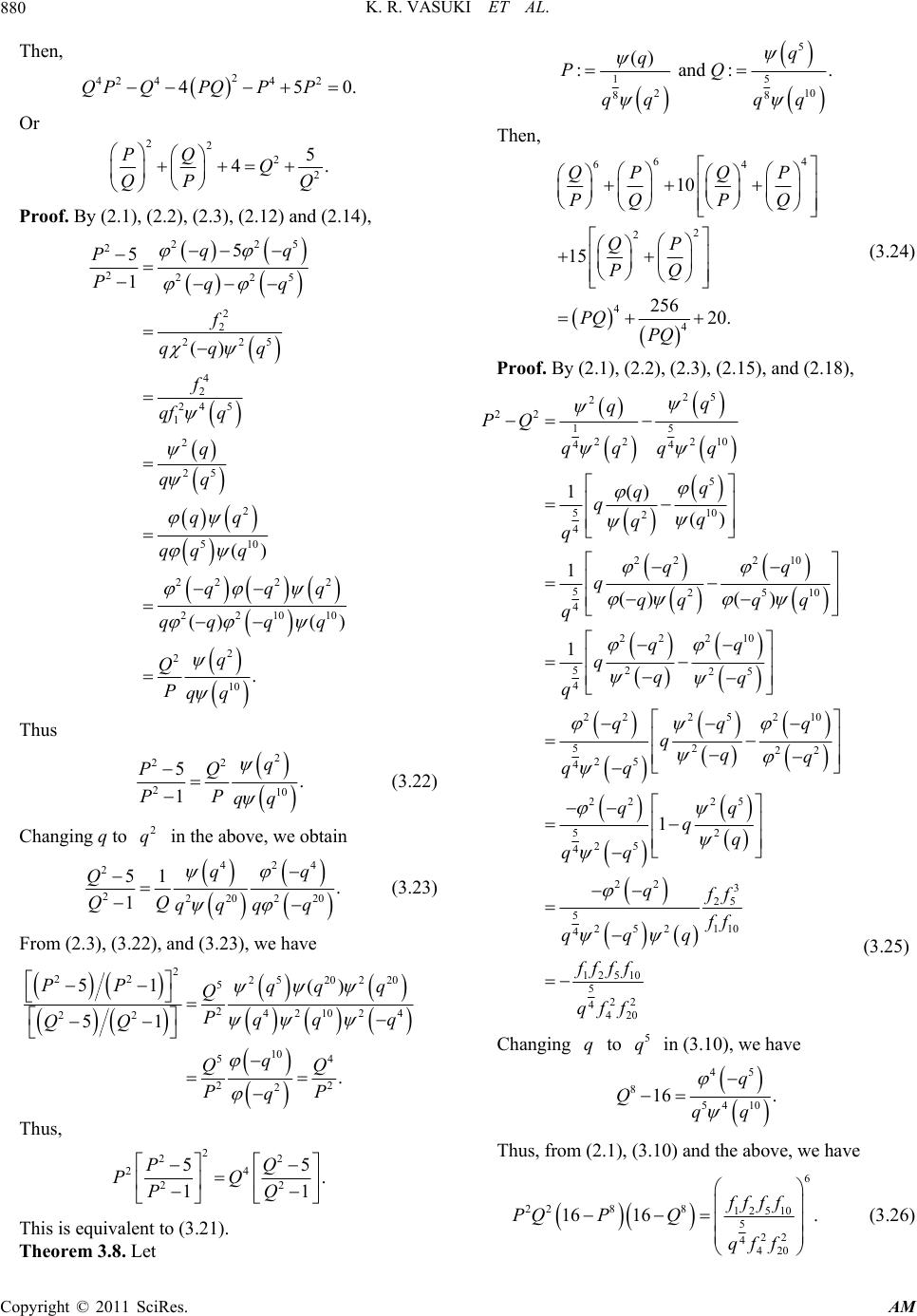 K. R. VASUKI ET AL. 880 0. Then, 2 42 442 45QP QPQPP Or 22 2 2 5 4. PQ Q QPQ Proof. By (2.1), (2.2), (2.3), (2.12) and (2.14), 225 2 2225 2 2 225 4 2 24 5 1 2 25 2 510 222 2 22 1010 2 2 10 5 5 1 () () () () . qq P Pqq f qqq f qf q q qq qq qqq qqq qqq q q Q Pqq Thus 2 22 210 5. 1 q PQ P Pqq (3.22) Changing q to in the above, we obtain 2 q 424 2 2220 220 51 . 1 qq Q Q Qqqq q (3.23) From (2.3), (3.22), and (3.23), we have 2 22 2520 2 20 5 242102 4 22 10 54 22 2 51 () 51 . PP qqq Q Pqqq QQ q QQ PP q Thus, 2 22 24 22 55 . 11 PQ PQ PQ This is equivalent to (3.21). Theorem 3.8. Let 5 15 21 88 () : and :. q q PQ qq qq 0 Then, 64 64 2 2 4 4 10 15 256 20. QP QP PQ PQ QP PQ PQ PQ (3.24) Proof. By (2.1), (2.2), (2.3), (2.15), and (2.18), 25 2 22 15 22 210 44 5 510 2 4 22 210 5251 4 22 210 52 25 4 2225 210 52 22 25 4 22 5 25 4 1() () 1 ()( ) 1 1 q q PQ qqqq q q qq q q qq qqqq q q qq qqq q qq qqq qq q qq 0 q 25 2 22 3 25 5 252110 4 12 510 5 22 4 420 q qq qff ff qqq ffff qff (3.25) Changing to in (3.10), we have q5 q 45 8 54 10 16 . q Qqq Thus, from (2.1), (3.10) and the above, we have 6 228812510 5 22 4 420 16 16. ffff PQ PQ qff (3.26) Copyright © 2011 SciRes. AM  K. R. VASUKI ET AL.881 Comparing (3.25) and (3.26), we obtain 6 22 2288 1616 ,PQ PQPQ which is equivalent to the required result. The following corollary is due to Vasuki and Srivat- saKumar [18]. Corollary 3.6. Let 5 15 21 44 () : and : q q PQ qqqq 0 . Then, 32 32 2 2 10 15 256 20. QP QPQP PQ PQPQ PQ PQ Corollary 3.6 follows from (2.3) and (3.24). The fol- lowing theorem is due to Adiga et al. [9]. Theorem 3.9. Let 2 110 5 2 : and :. q q PQ qq qq 0 Then, 42222 44 45PQPQQP Q. (3.27) Proof. By (2.15), (2.17) and (2.1), 22 2 222 25 22 1 25 2 5 15 . qq q P Pqqq q fq q q 5 5 (3.28) Changing to in (3.28), we obtain q2 q 210 2 222 1. 5 q Q Qq (3.29) By (2.2), (2.3), (3.28), and (3.29), 22542 22 222 410 24 2 25 15 51 . qq PQ PQ qq qP Q q Thus, we have 22 22 2422 15 51QP QPPQ0. Factorizing the above, we find that ,,CPQDPQ0, where 42 422 42 ,4CPQPQPPQQQ 5 and 22 22 ,Q5DPQ PQP. If ,DPQ0, then 2 2 2 225 5 1 q P QPq by (3.28). This is not true by the definition of Q. Hence ,DPQ0 . Thus we most have ,0CPQ . This completes the proof. 4. Acknowledgements The authors are thankful to DST, New Delhi for award- ing research project [No. SR/S4/MS:517/08] under which this work has been done. 5. References [1] B. C. Berndt, “Ramanujan’s Notebooks, Part IV,” Sprin- ger-Verlag, New York, 1994. [2] S. Ramanujan, “Notebooks (2 Volumes),” Tata Institute of Fundamental Research, Bombay, 1957. [3] B. C. Berndt and L.-C. Zhang, “Ramanujan’s Identities for Eta Functions,” Mathematische Annalen, Vol. 292, No. 1, 1992, pp. 561-573. doi:10.1007/BF01444636 [4] S. Ramanujan, “The Lost Notebook and Other Unpub- lished Paper,” Narosa, New Delhi, 1988. [5] B. C. Berndt, “Modular Equations in Ramanujan’s Lost Notebook,” In: R. P. Bamvah, V. C. Dumir and R. S. Hans-Gill, Eds., Number Theory, Hindustan Book Co., Delhi, 1999, pp. 55-74. [6] B. C. Berndt and H. H. Chan, “Some Values for Rogers- Ramanujan Continued Fraction,” Canadian Journal of Mathematics, Vol. 47, 1995, pp. 897-914. doi:10.4153/CJM-1995-046-5 [7] B. C. Berndt, H. H. Chan and L.-C. Zhang, “Ramanujan’s Class Invariants and Cubic Continued Fraction,” Acta Arithmetica, Vol. 73, 1995, pp. 67-85. [8] C. Adiga, K. R. Vasuki and M. S. M. Naika, “Some New Explicit Evaluations of Ramanujan’s Cubic Continued Fraction,” New Zealand Journal of Mathematics, Vol. 31, 2002, pp. 109-114. [9] C. Adiga, K. R. Vasuki and K. Shivashankara, “Some Copyright © 2011 SciRes. AM  K. R. VASUKI ET AL. Copyright © 2011 SciRes. AM 882 Theta Function Identities and New Explicit Evaluation of Rogers-Ramanujan Continued Fraction,” Tamsui Oxford Journal of Mathematical Sciences, Vol. 18, No. 1, 2002, pp. 101-117. [10] N. D. Baruah, “On Some of Ramanujan’s Identities for Eta Functions,” Indian Journal of Mathematics, Vol. 43, 2000, pp. 253-266. [11] N. D. Baruah and N. Saikia, “Some General Theorems on the Explicit Evaluations of Ramanujan’s Cubic Contin- ued Fraction,” Journal of Computational and Applied Mathematics, Vol. 160, No. 1-2, 2003, pp. 37-51. doi:10.1016/S0377-0427(03)00612-5 [12] S. Bhargava, K. R. Vasuki and T. G. Sreeramamurthy, “Some Evaluations of Ramanujan’s Cubic Continued Fraction,” Indian Journal of Pure and Applied Mathemat- ics, Vol. 35, 2004, pp. 1003-1025. [13] S.-Y. Kang, “Ramanujan’s Formulas for Explicit Evalua- tion of the Rogers-Ramanujan Continued Fraction and Theta Functions,” Acta Arithmetica, Vol. 90, 1999, pp. 49-68. [14] M. S. M. Naika and B. N. Dharmendra, “On Some New General Theorem for the Explicit Evaluations of Ra- manujan’s Remarkable Product of Theta Function,” Ra- manujan Journal, Vol. 15, No. 3, 2008, pp. 349-366. doi:10.1007/s11139-007-9081-1 [15] K. R. Vasuki, “On Some Ramanujan’s P-Q Modular Equations,” Journal of the Indian Mathematical Society, Vol. 73, No. 3-4, 2006, pp. 131-143. [16] K. R. Vasuki and K. Shivashankara, “A Note on Explicit Evaluations of Products and Ratios of Class Invariants,” Math Forum, Vol. 13, 2000, pp. 45-46. [17] K. R. Vasuki and T. G. Sreeramamurthy, “A Note on Explicit Evaluations of Ramanujan’s Continued Frac- tion,” Advanced Studies in Contemporary Mathematics, Vol. 9, 2004, pp. 63-80. [18] K. R. Vasuki and B. R. Srivasta Kumar, “Certian Identi- ties for Ramanujan-G¨ollnitz-Gordan Continued Frac- tion,” Journal of Computational and Applied Mathemat- ics, Vol. 187, No. 1, 2006, pp. 87-95. doi:10.1016/j.cam.2005.03.038 [19] K. R. Vasuki and B. R. S. Kumar, “Evaluations of the Ramanujan-Gollnitz-Gordon Continued Fraction H(q) by Modular Equations,” Indian Journal of Mathematics, Vol. 48, No. 3, 2006, pp. 275-300. [20] J. Yi, “Evaluations of the Rogers-Ramanujan’s Continued Fraction R(Q) by Modular Equations,” Acta Arithmetica, Vol. 97, No. 2, 2001, pp. 103-127. doi:10.4064/aa97-2-2 [21] C. Adiga, B. C. Berndt, S. Bhargava and G. N. Watson, “Chapter 16 of Ramanujan’s Second Notebook: Theta Functions and Q-Series,” Memoirs of the American Mathematical Society, Vol. 53, No. 315, 1985, pp. 55-74. [22] B. C. Berndt, “Ramanujan’s Notebooks, Part III,” Springer-Verlag, New York, 1991. [23] E. T. Whittaker and G. N. Watson, “A Course of Modern Analysis,” 4th Edition, Cambridge University Press, Cambridge, 1966. [24] N. D. Baruah and R. Barman, “Certain Theta Function Identities and Ramanujan’s Modular Equations of De- gree 3,” Indian Journal of Mathematics, Vol. 48, No. 3, 2006, pp. 113-133. [25] K. R. Vasuki, G. Sharath and K. R. Rajanna, “Two Modular Equations for Squares of the Cubic-Functions with Applications,” Note di Mathematica, In Press. [26] S.-Y. Kang, “Some Theorems in Rogers-Ramanujan Con- tinued Fraction and Associated Theta Functions in Ra- manujan’s Lost Notebook,” Ramanujan Journal, Vol. 3, No. 1, 1999, pp. 91-111. doi:10.1023/A:1009869426750 [27] S. Bhargava, K. R. Vasuki and K. R. Rajanna, “On Cer- tain Identities of Ramanujan for Ratios of Eta Functions,” Preprint.
|