Applied Mathematics, 2011, 2, 764-770
doi:10.4236/am.2011.26102 Published Online June 2011 (http://www.SciRP.org/journal/am)
Effect of Slip Velocity on Blood Flow through a
Catheterized Artery
Narendra Kumar Verma 1, Shailesh Mishra1, Shafi Ullah Siddiqui1, Ram Saran Gupta2
1Department of Mat hem at i cs, Harcourt Butler Technological Institute, Kanpur, In di a
2Department of Mat hem at i cs, Kamla Nehru Institute of Technology, Sultanpur, India
Received April 17, 2011; revised May 4, 2011; accepted May 7, 2011
Abstract
A mathematical model for pulsatile flow of blood in a catheterized artery in presence of an axisymmetric
stenosis with a velocity slip at the constricted wall is proposed. The expressions for the flow characteristics,
velocity profiles, the flow resistance, the wall shear stress, the effective viscosity are obtained in the present
analysis. The effects of slip velocity on the blood flow characteristics are shown graphically and discussed
briefly.
Keywords: Pulsatile, Stenosis, Catheter, Flow Resistance, Wall Shear Stress, Slip Velocity
1. Introduction
Atherosclerosis is the leading cause of death in many
countries. There is considerable evidence that vascular
fluid dynamics plays an important role in the develop-
ment and progression of arterial stenosis, which is one of
the most widespread diseases in human beings. The fluid
mechanical study of blood flow in artery bears some
important aspects due to the engineering interest as well
as the feasible medical applications. The hemodynamic
behavior of the blood flow is influenced by the presence
of the arterial stenosis. If the stenosis is present in an
artery, normal blood flow is disturbed. The actual causes
of stenosis are not well known but its effects on the car-
diovascular system can be understood by studying the
blood flow in its vicinity [1-4]. Ahmed et al. [5] de-
scribed the effect of stenosis at moderate Reynolds
number with a reference to monkey aorta with induced
atherosclerosis. Siouffi et al. [6] studied experimental
analysis of unsteady flows through a stenosis, on the
basis of the changes induced by the waveform on post
stenostic flow characteristic in a 75% severe stenosis.
The study of pulsatile flow through a stenosis is moti-
vated by the need to obtain a better understanding of the
impact of flow phenomena on atherosclerosis and stroke.
In order to understand the effect of stenosis on blood
flow through and beyond the narrowed segment of the
artery, many studies have been undertaken experimen-
tally and theoretically. Liu and Yamaguchi [7] find out a
systematic study of a pulsatile flow in a stenosed channel
to identify how the waveform affects the generation, de-
velopment and breakdown of the vortex wave. Numeri-
cal solutions of pulsatile flow have been reported by
several investigators [8,9], which has been done assum-
ing the blood as a Newtonian fluid. A number of re-
searchers have studied the flow of non-Newtonian fluids
with the pulsation through arterial stenosis [10-13].
The flow through an annulus with mild constriction at
the outer wall can be used as a model for the blood flow
through the catheterized stenotic artery. The insertion of
a catheter (a long flexible cylindrical tube) into a con-
stricted tube (i.e. stenosed artery) results in an annular
region between the walls of the catheter and artery. This
will alter the flow field, modify the pressure distribution
and increase the resistance. Even though the catheter tool
devices are used for the measurement of arterial blood
pressure or pressure gradient and flow velocity or flow
rate, X-ray angiography and intravascular ultrasound
diagnosis and coronary balloon angioplasty treatment of
various arterial diseases, a little attention has been given
in the literature to the flow in catheterized arteries. Roose
and Lykoudis [14] studied the fluid mechanics of the
ureter with an inserted catheter by considering the peri-
staltic wave moving along the stationary cylinder.
McDonald [15] considered the pulsatile blood flow in a
catheterized artery and obtained theoretical estimates for
pressure gradient corrections for catheters, which are
positioned eccentrically, as well as coaxially with the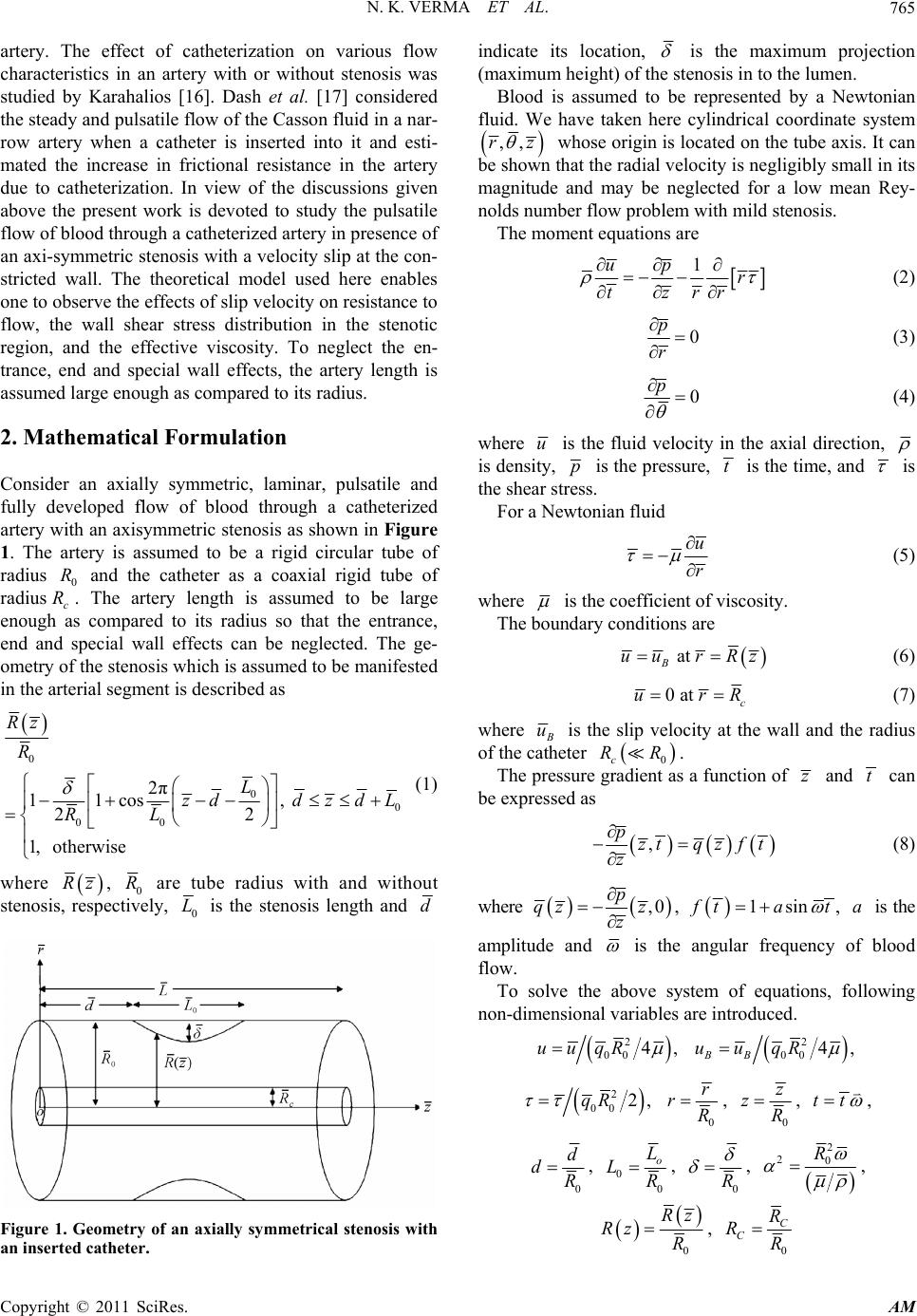

N. K. VERMA ET AL.765
artery. The effect of catheterization on various flow
characteristics in an artery with or without stenosis was
studied by Karahalios [16]. Dash et al. [17] considered
the steady and pulsatile flow of the Casson fluid in a nar-
row artery when a catheter is inserted into it and esti-
mated the increase in frictional resistance in the artery
due to catheterization. In view of the discussions given
above the present work is devoted to study the pulsatile
flow of blood through a catheterized artery in presence of
an axi-symmetric stenosis with a velocity slip at the con-
stricted wall. The theoretical model used here enables
one to observe the effects of slip velocity on resistance to
flow, the wall shear stress distribution in the stenotic
region, and the effective viscosity. To neglect the en-
trance, end and special wall effects, the artery length is
assumed large enough as compared to its radius.
2. Mathematical Formulation
Consider an axially symmetric, laminar, pulsatile and
fully developed flow of blood through a catheterized
artery with an axisymmetric stenosis as shown in Figure
1. The artery is assumed to be a rigid circular tube of
radius 0 and the catheter as a coaxial rigid tube of
radius c. The artery length is assumed to be large
enough as compared to its radius so that the entrance,
end and special wall effects can be neglected. The ge-
ometry of the stenosis which is assumed to be manifested
in the arterial segment is described as
R
R

0
0
0
00
2π
11cos,
22
1, otherwise
Rz
R
L
zdd zdL
RL



 






(1)
where

Rz, 0 R are tube radius with and without
stenosis, respectively, 0
L is the stenosis length and d
Figure 1. Geometry of an axially symmetrical stenosis with
an inserted catheter.
indicate its location,

is the maximum projection
(maximum height) of the stenosis in to the lumen.
Blood is assumed to be represented by a Newtonian
fluid. We have taken here cylindrical coordinate system


,,rz  whose origin is located on the tube axis. It can
be shown that the radial velocity is negligibly small in its
magnitude and may be neglected for a low mean Rey-
nolds number flow problem with mild stenosis.
The moment equations are

1up r
tzrr


 
 
 (2)
0
p
r


 (3)
0
p



 (4)
where u is the fluid velocity in the axial direction,

is density, p is the pressure, t is the time, and

is
the shear stress.
For a Newtonian fluid
u
r




(5)
where

is the coefficient of viscosity.
The boundary conditions are

at
B
uu rRz (6)
0 at c urR

 (7)
where
B
u is the slip velocity at the wall and the radius
of the catheter


0c
RR.
The pressure gradient as a function of z and t can
be expressed as
 
,
pztqzf t
z


 (8)
where
 
,0
p
qz z
z

,

1sin
f
tat

 , is the a
amplitude and

is the angular frequency of blood
flow.
To solve the above system of equations, following
non-dimensional variables are introduced.


2
00 4,uuqR



2
004,
BB
uuqR




2
002,qR
 
0
r
rR
,
0
z
zR
, tt


,
0
d
dR , 0
0
o
L
LR ,
0
R

 ,

2
20
R




,



0
Rz
Rz R ,
0
C
C
R
RR 

N. K. VERMA ET AL. 766
where

is the pulsatile Reynolds numbers for Newto-
nian fluid and 0 q is the pressure gradient in a uniform
tube without catheter.
 

4
π
e
pRz
z
Qt







 (16)
Using non-dimensional variables Equations (2)-(5)
reduce to It can be expressed in dimensionless form as
 
22
4
uqzft r
trr






(9)
 
  
4
e
Rz qzft
Qt
  (17)
1
2
u
r


  (10) where


Qt is defined in Equation (15).
An application of Equation (10) in to (9), yields
 
21
4
uu
qzft r
trr






3. Solution
r




(11)
Consider the Womersley parameter to be small. The ve-
locity u can be expressed in the following form
where

1sin
f
ta t

,

0
qzqz q,
,

qz

0ft




2
01
,, ,,,,u rzturzturzt 

 (18)
The boundary conditions in their non-dimensional
form are now expressed as




2
01
,, ,,,,zrt zrtzrt  

 (19)

at
B
uu rRz (12) Substituting the expression of u from Equation (18) in
(11), we get
0 at c urR (13)
 
04
u
rrqz
rr






The geometry of the stenosis in dimensionless form is
given by
ft (20)

0
0
0
00
2π
11cos,
22
1, otherwise
Rz
R
L
zdd zdL
RL



 






(14)
01
1
uu
r
trr t







(21)
Substituting u from Equation (18) into conditions (12)
in (13) we get

01
, 0 at
B
uuurRz (22)
01
0, 0 at c uu rR

 (23)
The non-dimensional volumetric flow rate is given by
 

4,,
c
Rz
R
QtrurztrIntegrating Equations (20) and (21) and using the
boundary conditions (22) and (23), we have the expres-
sions for and u as in Equations (24) and (25).
d (15)
0 1
The expression for velocity can easily be obtained
from Equations (18), (24) and (25).
u
where
 

4
00
p
8
Qt
Qt Rq

 and u
The wall shear stress w

(as a result of Equations (10)
and (18)) becomes,



2π,,
c
Rz
R
Qt rurzt

is the volumetric flow rate.

01
1
2
w
rRz
uu
rr




 



(26)
The effective viscosity e

is defined as


 





22
22
0
log
1l
log log
c
B
cc
RR
rR
uuqzftRr
RR RRog
rR














(24)
 







 

22
22 44222
1
22
1
22 44222
43 4log33
16 log
log( )
434log33
log( )log
c
c
ccc cc
cc
RR
qzf t
uRrrRrrRrR
RR
RR
rR RRRRRR RRR
RR RR


 







 





(25)

N. K. VERMA ET AL. 767
which is determined, by substituting velocity Equations (24) and (25) into the Equation (26), in the form

 



 






22 22
2
3
22
224422 2
42
2 log2 log32log
1
434log33
log log
cc
B
wcc
cc
ccc c
cc
RR RR
uqzftRqzf tRR
R RRR RRRR
RR R
RRR RRR R
RRRRRR R





 
 







 

 


 





c
(27)
From Equations (15), (24) and (25) the expression for volumetric flow rate is given by





 


 

 


 

222
22
22
2
222 22
22
2
22622644422
2log
2log
2log
log
182128612log1218
48 log
cc c
cB
c
c
cccc
c
c
cccc ccc
c
RRRRR
QtR Ru
RR
RR
qzftRRRRR RR
RR
RR
qzftRRRRRRRRR RRRR
RR














 







 
 
22 22
22244222
634324log18
log log
cc
ccc ccc
cc
RR RR
RRRRRRRRRR
RR RR

 

 

 
 

 
 

(28)
The effective viscosity e

can be found out with the
help of Equations (17) and (28).
If steady flow is considered, then Equation (28) re-
duces to








 

22222
2
22222 22
2log
22log
log log
cc cc
scB cccc
cc
RRRRRRR
QRRuqzRRRRRRR
RR RR

 

 






(29)
where
s
Q is the steady flow rate.
The value of can be found from Equation (29),
taking .

qz
1
s
Q
In absence of catheter, (i.e. when ), the Equa-
tions (24), (25), (27), (28) reduce to
0
c
R
 

22
0B
uuqzftRr 

(31)
 

22 44
14
16
qzf t
uRrr


3
R
(32)
 
2
3
8
wqzftRqzf tR



 (33)
     
  
2
24
2
6
B
QtRzuqz f tRz
qzf t Rz






 

4. Result and Discussions
With a view to examining the applicability of the present
mathematical model, a specific numerical illustration has
been undertaken with the use of the existing data for the
various physical parameters encountered in the analysis.
The following data have been made use of in order to
carry out the numerical computations:
0.5a

; 0, 0.1, 0.2, 0.3, 0.4, 0.5
c
R

;
0.5


; 0, 0.1, 0.2

.
2
(34)
For the present steady simulation, the profiles of the
velocity-field are computed and plotted in Figures 2 and
3. Figure 2 shows the variations of axial velocity, u with
radial distance, r for different time periods, t and fixed
stenosis height,

, c and R

. It is seen that velocity
increases rapidly with time, t as t goes from t = 0˚ to t =
90˚ and then decreases sharply when t goes from t = 90˚
to t = 270˚. It further increases in the time cycle from t =

N. K. VERMA ET AL. 768
Figure 2. Variation of axial velocity with radial distance.
Figure 3. Variation of axial velocity with radial distance.
270˚ to t = 360˚..
Axial velocity decreases with increasing stenosis
height,

for different slip velocity,
B
u and for fixed
values of c, R

and t (Figure 3). It can be clearly
observed that the axial velocity assumes higher magni-
tude in a uniform artery than that in a stenosed artery.
Also the axial velocity increases with increasing slip
velocity,
B
u in both the stenotic and uniform artery.
Both these figures also include the corresponding pro-
files in the absence of stenosis.
The variations of the wall shear stress, w

with the
axial distance, z for different values of catheter radius,
c and slip velocity, R
B
u for fixed

, and t

are
presented in Figure 4. The blood flow characteristic, w

increases with axial distance, r in the stenotic region in
the upstream of the stenosis throat and attains its maxi-
mum at the throat and then decreases sharply. The wall
shear stress, w

decreases with increasing slip velocity
for any value of c. One notices that the flow charac-
teristic, w
R

assumes higher values in a catheterized ar-
tery than that in an uncatheterized artery.
Figure 5 demonstrates the variations of the blood flow
characteristic, w

with catheter radius for different val-
ues of slip velocity,
B
u in stenosed and normal artery.
It is noticed that increase in catheter radius increases the
wall shear stress. On the other hand, increase in slip ve-
locity reduces the wall shear stress in both the normal
and the stenosed artery. The variations of effective vis-
cosity, e

with the catheter radius, c for different
values of slip velocity,
R
B
u and fixed stenosis height,

and time, are illustrated in Figure 6. The effective
viscosity, e
t

increases with increasing catheter radius,
c significantly while it decreases with increasing slip
velocity,
R
B
u.
Figure 7 reveals the variations of effective viscosity,
e

with the catheter radius, c for different stenosis
heights,
R

and t for fixed time. An increase in steno-
sis height

increases the effective viscosity e

. It is
observed that the magnitude of effective viscosity e

is
less in a normal artery in comparison to that of the
stenosed artery.
Figure 4. Variation of wall shear stress with axial distance.
Figure 5. Variation of wall shear stress with catheter ra-
dius.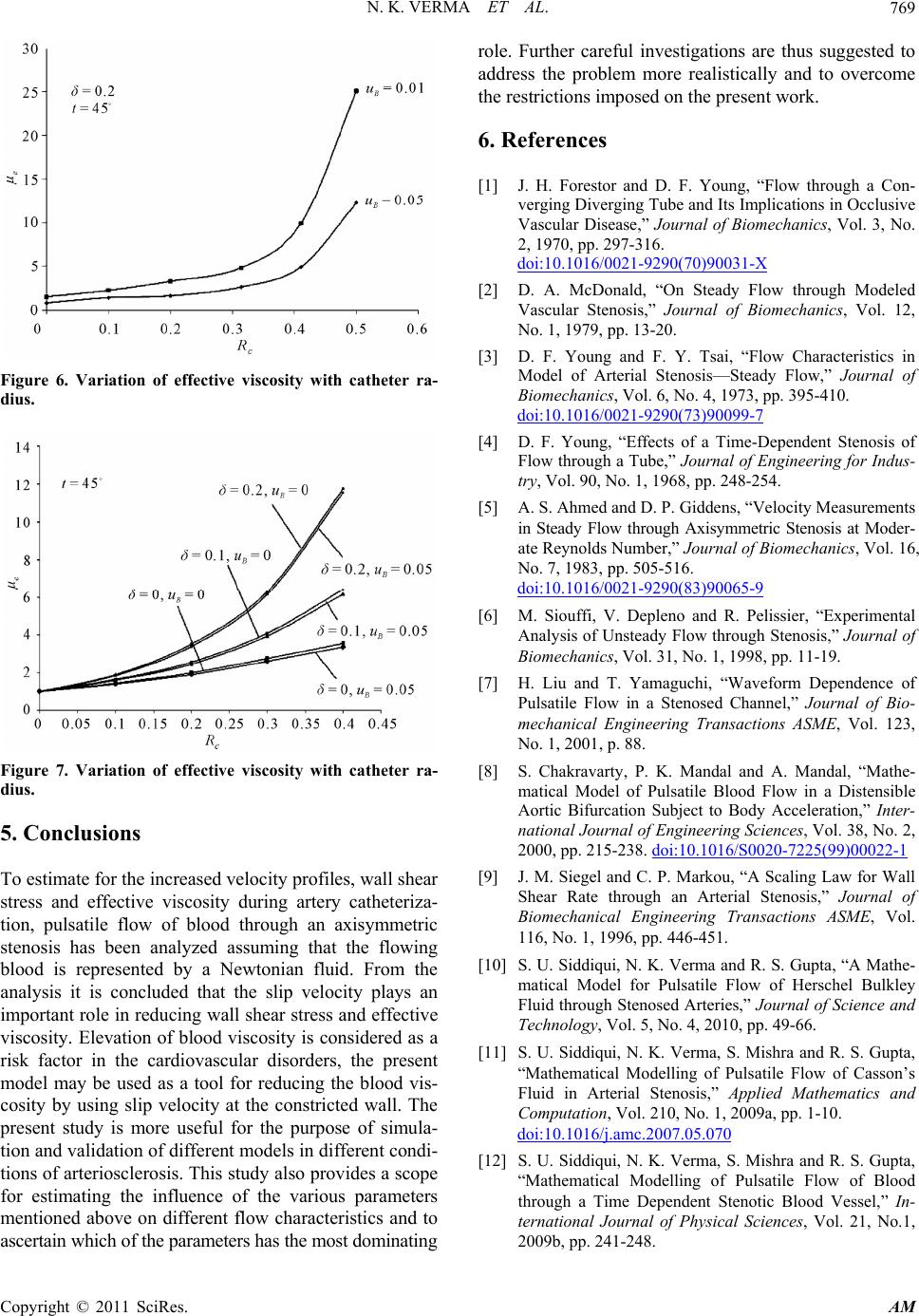

N. K. VERMA ET AL.769
Figure 6. Variation of effective viscosity with catheter ra-
dius.
Figure 7. Variation of effective viscosity with catheter ra-
dius.
5. Conclusions
To estimate for the increased velocity profiles, wall shear
stress and effective viscosity during artery catheteriza-
tion, pulsatile flow of blood through an axisymmetric
stenosis has been analyzed assuming that the flowing
blood is represented by a Newtonian fluid. From the
analysis it is concluded that the slip velocity plays an
important role in reducing wall shear stress and effective
viscosity. Elevation of blood viscosity is considered as a
risk factor in the cardiovascular disorders, the present
model may be used as a tool for reducing the blood vis-
cosity by using slip velocity at the constricted wall. The
present study is more useful for the purpose of simula-
tion and validation of different models in different condi-
tions of arteriosclerosis. This study also provides a scope
for estimating the influence of the various parameters
mentioned above on different flow characteristics and to
ascertain which of the parameters has the most dominating
role. Further careful investigations are thus suggested to
address the problem more realistically and to overcome
the restrictions imposed on the present work.
6. References
[1] J. H. Forestor and D. F. Young, “Flow through a Con-
verging Diverging Tube and Its Implications in Occlusive
Vascular Disease,” Journal of Biomechanics, Vol. 3, No.
2, 1970, pp. 297-316.
doi:10.1016/0021-9290(70)90031-X
[2] D. A. McDonald, “On Steady Flow through Modeled
Vascular Stenosis,” Journal of Biomechanics, Vol. 12,
No. 1, 1979, pp. 13-20.
[3] D. F. Young and F. Y. Tsai, “Flow Characteristics in
Model of Arterial Stenosis—Steady Flow,” Journal of
Biomechanics, Vol. 6, No. 4, 1973, pp. 395-410.
doi:10.1016/0021-9290(73)90099-7
[4] D. F. Young, “Effects of a Time-Dependent Stenosis of
Flow through a Tube,” Journal of Engineering for Indus-
try, Vol. 90, No. 1, 1968, pp. 248-254.
[5] A. S. Ahmed and D. P. Giddens, “Velocity Measurements
in Steady Flow through Axisymmetric Stenosis at Moder-
ate Reynolds Number,” Journal of Biomechanics, Vol. 16,
No. 7, 1983, pp. 505-516.
doi:10.1016/0021-9290(83)90065-9
[6] M. Siouffi, V. Depleno and R. Pelissier, “Experimental
Analysis of Unsteady Flow through Stenosis,” Journal of
Biomechanics, Vol. 31, No. 1, 1998, pp. 11-19.
[7] H. Liu and T. Yamaguchi, “Waveform Dependence of
Pulsatile Flow in a Stenosed Channel,” Journal of Bio-
mechanical Engineering Transactions ASME, Vol. 123,
No. 1, 2001, p. 88.
[8] S. Chakravarty, P. K. Mandal and A. Mandal, “Mathe-
matical Model of Pulsatile Blood Flow in a Distensible
Aortic Bifurcation Subject to Body Acceleration,” Inter-
national Journal of Engineering Sciences, Vol. 38, No. 2,
2000, pp. 215-238. doi:10.1016/S0020-7225(99)00022-1
[9] J. M. Siegel and C. P. Markou, “A Scaling Law for Wall
Shear Rate through an Arterial Stenosis,” Journal of
Biomechanical Engineering Transactions ASME, Vol.
116, No. 1, 1996, pp. 446-451.
[10] S. U. Siddiqui, N. K. Verma and R. S. Gupta, “A Mathe-
matical Model for Pulsatile Flow of Herschel Bulkley
Fluid through Stenosed Arteries,” Journal of Science and
Technology, Vol. 5, No. 4, 2010, pp. 49-66.
[11] S. U. Siddiqui, N. K. Verma, S. Mishra and R. S. Gupta,
“Mathematical Modelling of Pulsatile Flow of Casson’s
Fluid in Arterial Stenosis,” Applied Mathematics and
Computation, Vol. 210, No. 1, 2009a, pp. 1-10.
doi:10.1016/j.amc.2007.05.070
[12] S. U. Siddiqui, N. K. Verma, S. Mishra and R. S. Gupta,
“Mathematical Modelling of Pulsatile Flow of Blood
through a Time Dependent Stenotic Blood Vessel,” In-
ternational Journal of Physical Sciences, Vol. 21, No.1,
2009b, pp. 241-248.

N. K. VERMA ET AL. 770
[13] I. Takuji and L. F. R. Guimaraes, “Effect of Non-New-
tonian Property of Blood on Flow through a Stenosed
Tube,” Fluid Dynamics Research, Vol. 22, No. 5, 1998,
pp. 251-264. doi:10.1016/S0169-5983(97)00041-5
[14] R. Roos and P. S. Lykoudis, “The Fluid Mechanics of the
Ureter with an Inserted Catheter,” Journal of Fluid Me-
chanics, Vol. 46, No. 4, 1971, pp. 625-630.
doi:10.1017/S0022112071000752
[15] D. A. McDonald, “Pulsatile Flow in a Catheterized Ar-
tery,” Journal of Biomechanics, Vol. 19, No. 3, 1986, pp.
239-249. doi:10.1016/0021-9290(86)90156-9
[16] G. T. Karahalios, “Some Possible Effects of a Catheter on
the Arterial Wall,” Medical Physiology, Vol. 17, No. 5,
1990, pp. 922-925. doi:10.1118/1.596448
[17] R. K. Dash, G. Jayaraman and K. N. Mehta, “Estimation
of Increased Flow Resistance in a Narrow Catheterized
Artery—A Theoretical Model,” Journal of Biomechanics,
Vol. 29, No. 7, 1996, pp. 917-930.
doi:10.1016/0021-9290(95)00153-0