Received 31 October 2014; revised 30 November 2014; accepted 12 December 2014

ABSTRACT

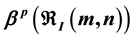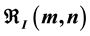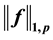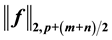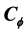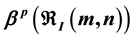In this paper, we introduce the weighted Bloch spaces on the first type of classical bounded symmetric domains, and prove the equivalence of the norms and. Furthermore, we study the compactness of composition operator from to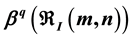, and obtain a sufficient and necessary condition for 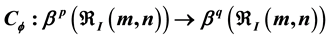to be compact.

Keywords:

Bloch Space, Classical Bounded Symmetric Domains, Composition Operators, Compactness, Bergman Metric

1. Introduction

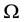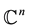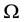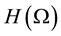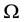Let be a bounded homogeneous domain in. The class of all holomorphic functions on will be denoted by. For a holomorphic self-map of and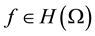, the composition is denoted by, and is called the composition operator with symbol.

The composition operators as well as related operators known as the weighted composition operators between the weighted Bloch spaces were investigated in [1] [2] in the case of the unit disk, and in [3] - [7] for the case of the unit ball. The study of the weighted composition operators from the Bloch space to the Hardy space was carried out in [8] [9] for the unit ball. Characterizations of the boundedness and the compactness of the composition operators and the weighted ones between the Bloch spaces were given in [10] - [12] for the polydisc case, and in [13] - [18] for the case of the bounded symmetric domains. Furthermore, we will give some results about the composition operators for the case of the weighted Bloch space on the bounded symmetric domains.

In 1930s all irreducible bounded symmetric domains were divided into six types by E. Cartan. The first four types of irreducible domains are called the classical bounded symmetric domains, the other two types, called exceptional domains, consist of one domain each (a 16 and 27 dimensional domain).

The first three types of classical bounded symmetric domains can be expressed as follows [19] :

,

where and is the identity matrix, is the transpose of;

Let and. The Kronecker product of and is defined as the

matrix such that the element at the -th row and -th column [19] . Then the Berg- man metric of is as follows (see [19] ):

(1.1)

where is a complex vector, is the conjugate transpose of, and

.

Following Timoney’s approach (see [18] ), a holomorphic function is in the Bloch space, if

Now we define a holomorphic function to be in the p-Bloch space, if

(1.2)

where

We can prove that is a Banach space with norm which is similar

with the case on

Let be a holomorphic self-map of. We are concerned here with the question of when

will be a compact operator.

Let denote a diagonal matrix with diagonal elements. In this work,we shall de- note by a positive constant, not necessarily the same on each occurrence.

In Section 2, we prove the equivalence of the norms defined in this paper and in [20] .

In Section 3, we state several auxiliary results most of which will be used in the proofs of the main results.

Finally, in Section 4, we establish the main result of the paper. We give a sufficient and necessary condition for the composition operator C_f from the p-Bloch space to the q-Bloch space to be compact, where and. Specifically,we prove the following result:

Theorem 1.1. Let be a holomorphic self-map of. Then is compact if and only if, for every, there exists a such that

(1.3)

for all whenever,.

The compactness of the composition operators for the weighted Bloch space on the bounded symmetric domains of is similar with the case of; we omit the details.

2. The Equivalence of the Norms

Denote [20] .

Lemma 2.1. (Bloomfield-Watson) [21] Let be an Hermitian matrix. Then

(2.1)

where is any matrix and satisfies.

Theorem 2.1. and are equivalent.

Proof. The metric matrix of is

For any, let with. Then

Denote then, and

Thus

Hence

Furthermore,

Since

Thus

(2.2)

For

then we have

(2.3)

Combining (2.2) and (2.3),

Next,

and

Therefore, the proof is completed. □

3. Some Lemmas

Here we state several auxiliary results most of which will be used in the proof of the main result.

Lemma 3.1. [18] Let be a bounded homogeneous domain. Then there exists a constant, depending only on, such that

(3.1)

for each whenever f holomorphically maps into itself. Here denotes the Bergman metric

on, denotes the Jacobian matrix of.

Lemma 3.2. Let be a holomorphic self-map of and a compact subset of.Then there exists a constant such that

(3.2)

for all whenever.

Proof. For, let

For any compact, there exists a constant such that. Then there exists

such that, whenever.

Thus

(3.3)

Combining Lemma 3.1 with (3.3) shows that (3.2) holds. □

Lemma 3.3. (Hadamard) [21] Let be an Hermitian matrix. Then

(3.4)

and equality holds if and only if is a diagonal matrix.

Lemma 3.4. Let. Then

(3.5)

Proof. For any, we have

Thus we have,

It follows from Lemma 3.3 that □

Lemma 3.5. Let be a classical bounded symmetric domain, and denote its metric matrix. Then a holomorphic function on is in if and only if

(3.6)

If (3.6) holds, then

(3.7)

Proof. We can get the conclusion by the process of the proof on Theorem 2.1. □

Lemma 3.6. [18] Let

where and are unitary matrices and

Denote,. Then

(1)

(2)

(3);

(4) for;

(5) for;

(6) for.

Lemma 3.7. is compact if and only if as for

any bounded sequence in that converges to 0 uniformly on compact subsets of.

Proof. The proof is trial by using the normal methods. □

4. Proof of Theorem 1.1

Proof. Let be a bounded sequence in with, and uniformly on compact subsets of.

Suppose (1.3) holds. Then for any, there exists a, such that

(4.1)

for all whenever and.

By the chain rule, we have.

If and, then we get. If and, then

(4.2)

It follows from (4.1) and (4.2) that

(4.3)

(4.4)

whenever and.

On the other hand, there exists a constant such that

So if, then

We assume that converges to 0 uniformly on compact subsets of. By Weierstrass Theorem, it is easy to see that converges to 0 uniformly on compact subsets of. Thus, for given, there exists large enough such that

(4.5)

for any, whenever and. Then by in- equalities (4.3) and (4.5) and Lemma 3.2, it follows that, for large enough,

(4.6)

whenever and.

Combining (4.4) and (4.6) shows that as large enough. So

is compact.

For the converse, arguing by contradiction, suppose is compact and

the condition (1.3) fails. Then there exist an, a sequence in with

as and a sequence in, such that

(4.7)

for all.

Now we will construct a sequence of functions 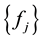satisfying the following three conditions :

(I) is a bounded sequence in;

(II) tends to 0 uniformly on any compact subset of;

(III)

The existence of this sequence will contradict the compactness of.

We will construct the sequence of functions according to the following four parts: A - D.

Part A: Suppose that

where is the matrix whose element at the row and column is 1 and the other elements are 0. Since maps into itself, and

Denote by Using formula (1.1), we have

Denote

then

(4.8)

We construct the sequence of functions according to the following three different cases.

Case 1. If for some,

(4.9)

then set

(4.10)

where is any positive number.

Case 2. If for some,

(4.11)

then set

(4.12)

where, if for some, or for some, , replace the corresponding term by 0 (the same below).

Case 3. If for some,

(4.13)

then set

(4.14)

Next, we will prove that the sequences of functions defined by (4.10), (4.12) and (4.14) all satisfy the conditions (I), (II) and (III).

To begin with, we will prove the sequence of functions defined by (4.10) satisfies the three con- ditions. We can get that

It follows from Lemma 3.5 that.

This proves that the sequence of functions defined by (4.10) satisfies condition (I).

Let be any compact subset of. Then there exists a such that

(4.15)

for any. By (4.10), we have

Since

But as. Thus, converges to 0 uniformly on. Therefore,

converges to 0 uniformly on as. Thus, the sequence of functions defined by (4.10) satisfies the condition (II).

Now (4.8) and (4.9) mean that

(4.16)

Combining (4.7) and (4.16), we have

Since

This proves that as, which means that the sequence of functions defined by (4.10) satisfies condition (III).

We can prove that the sequence of functions defined by (4.12) or (4.14) satisfies the conditions (I) - (III) by using the analogous method as above.

Part B: Now we assume that

It is clear that and for we can assume that and as, where.

If, we can use the same methods as in Part A to construct a sequence of functions satisfy- ing conditions (I)-(III).

Using formula (1.1), we have

Denote

Then,

(4.17)

We construct the sequence of functions according to the following six different cases.

Case 1. If for some,

then set

(4.18)

Case 2. If for some,

then set

(4.19)

Case 3. If for some,

then set

(4.20)

Case 4. If for some,

then set

(4.21)

Case 5. If for some,

then set

(4.22)

Case 6. If for some,

then set

(4.23)

By using the same methods as in Part A, we can prove the sequences of functions defined by (4.18)-(4.23) satisfying conditions (I) - (III).

Now, as an example,we will prove that the sequence of functions defined by (4.19) satisfying the conditions (I) - (III).

For any, we have

(4.24)

Thus

By Lemma, we have

(4.25)

It follows from Lemma 3.5 and (4.25) that. This proves that the sequence of functions defined by (4.19) satisfy the condition (I).

Let be any compact subset of. Since there exists a such that, Thus

Since

So as. Thus, converges to 0 uni-

formly on E. Therefore,the sequence of converges to 0 uniformly on as. Thus, the sequence of functions defined by (4.19) satisfies the condition (II).

For case 2,

(4.26)

Combining (4.7) and (4.26), we have

Since

This proves that as, which means that the sequence of functions defined by (4.19) satisfies condition (III).

If, then by Lemma 3.6, there exist and in such that

If we denote, then and

, where.

Denote, where the sequence of functions is the sequence obtained in Part A. We have

(4.27)

where and. Now (4.27) implies that

and

It is clear that, and combining the discussion in Part A,we can get that

as; that means the sequence of functions satisfies condition (III).

We prove that the sequence of functions is a bounded sequence in.

Since,

So is bounded.

Next we prove that converges to 0 uniformly on any compact subset of. Let

then by the definition of and Lemma 3.6, we can get a calculation directly that

It is clear that converges uniformly to in.

Since and, there similarly exist in such that

, and the first component of is. It is clear that is holo-

morphic on. Let for. For, we know

. We may choose such that. Thus, for large enough,

and from this it follows that

by the definition of, converges to 0 uniformly on.

Hence satisfies conditions (I)--(III), and this contradicts the compactness of.

Part C: Assume that

where. For we may assume that, , as, where,.

Just as in Part B, we can use the same methods to prove the conclusion. And for, , we may only show the sequence of functions which satisfy the conditions (I) - (III) here.

Using formula (1.1), we have

Denote

then,

(4.28)

We construct the sequence of functions according to the following three different cases.

Case 1. If for some,

then set

(4.29)

Case 2. If for some,

then set

(4.30)

Case 3. If for some,

then set

(4.31)

Using the same methods as in Part A and Part B, we can prove the sequences of functions defined by (4.29)-(4.31) satisfying conditions (I) - (III).

Part D: In the general situation. For, there exist an unitary matrix and an unitary matrix such that

We may assume that and as. Let and; means

that as for any,. Let and for

. Of course, P is an unitary matrix, is an unitary matrix, and con-

verges uniformly to on.

Let, where the sequence of are the functions obtained in Part C.

From the same discussion as that in Part B, we know that satisfies conditions (I) and (III). For the compact subset, is also a compact subset of, so we can choose an open sub-

set of such that. Since converges uniformly to

on, it follows that as. Since tends to 0 uniformly on, we know tends to 0 uniformly on. Thus, satisfies condition (II). □

Acknowledgements

We thank the Editor and the referee for their comments. Research is funded by the National Natural Science Foundation of China (Grant No. 11171285) and the Postgraduate Innovation Project of Jiangsu Province of China (CXLX12-0980).

Cite this paper

JianbingSu,HuijuanLi,XingxingMiao,RuiWang, (2014) Compactness of Composition Operators from the p-Bloch Space to the q-Bloch Space on the Classical Bounded Symmetric Domains. Advances in Pure Mathematics,04,649-664. doi: 10.4236/apm.2014.412074

References

NOTES

^*Corresponding author.