Advances in Pure Mathematics
Vol.4 No.3(2014), Article ID:44351,4 pages DOI:10.4236/apm.2014.43014
Heredity of Lower Separation Axioms on Function Spaces
Njuguna E. Muturi
Department of Mathematics, Egerton University, Egerton, Kenya
Email: edward.njuguna@gmail.com
Copyright © 2014 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/


Received 23 January 2014; revised 23 February 2014; accepted 28 February 2014
ABSTRACT
The set of continuous functions from topological space 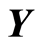 to topological space
to topological space  endowed with a topology forms the function space. For
endowed with a topology forms the function space. For  subset of
subset of , the set of continuous functions from the space
, the set of continuous functions from the space 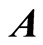 to the space
to the space 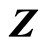 forms the underlying function space with an induced topology. The function space has properties of topological space dependent on the properties of the space
forms the underlying function space with an induced topology. The function space has properties of topological space dependent on the properties of the space , such as the
, such as the ,
, 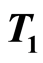 ,
, 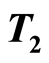 and
and 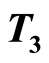 separation axioms. In this paper, we show that the underlying function space inherits the
separation axioms. In this paper, we show that the underlying function space inherits the ,
, 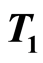 ,
, 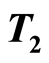 and
and 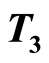 separation axioms from the function space, and that these separation axioms are hereditary on function spaces.
separation axioms from the function space, and that these separation axioms are hereditary on function spaces.
Keywords:Function Space; Underlying Function Space; Hereditary Properties

1. Introduction
The set of continuous functions from the space 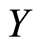 to the space
to the space  is denoted by
is denoted by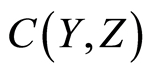 . The set open topology
. The set open topology 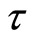 defined on the set
defined on the set 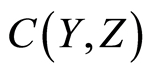 generated by the sets of the form
generated by the sets of the form where the sets
where the sets 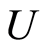 and
and  ranges over the class
ranges over the class  of compact subsets of
of compact subsets of 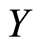 and
and 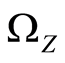 class of open subsets of
class of open subsets of 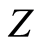 respectively, is called the compact open topology. The sets of the form
respectively, is called the compact open topology. The sets of the form  forms subbases for the compact open topology
forms subbases for the compact open topology  on
on 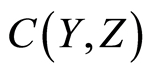 (see [1] ). The set open topology
(see [1] ). The set open topology 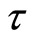 defined on the set
defined on the set 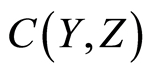 generated by the subbases
generated by the subbases 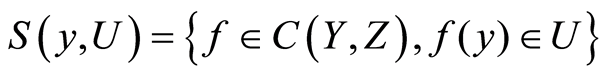 where
where  and
and 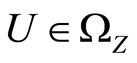 is called point open topology (see [2] ).
is called point open topology (see [2] ).
Let 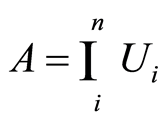 for
for 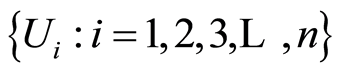 family of non-empty open subsets of
family of non-empty open subsets of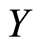 . The set
. The set  consist of continuous functions of the form
consist of continuous functions of the form  where
where  is an inclusion mapping (see [3] ).
is an inclusion mapping (see [3] ).
Let the topological space 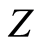 be a
be a  -space for
-space for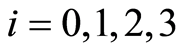 , then the function space
, then the function space 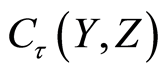 with compact open topology
with compact open topology 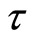 inherits the
inherits the  -separation axioms for
-separation axioms for 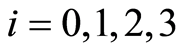 (see [4] and [5] ).
(see [4] and [5] ).
Definition 1.1 For , the sets of the form
, the sets of the form
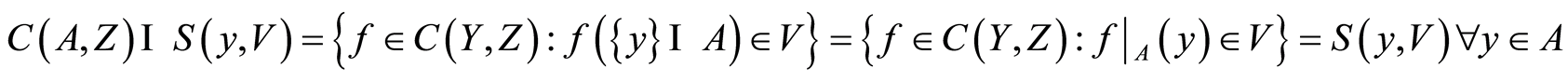 as defined in [3] , forms the subbases for point open topology on the set
as defined in [3] , forms the subbases for point open topology on the set .
.
Definition 1.2 The sets of the form
 where
where 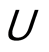 is open in
is open in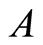 ,
, 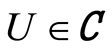 and
and , defines the subbases for the set open topology on the set
, defines the subbases for the set open topology on the set 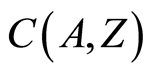 (see [3] ). This topology is referred to as open-open topology (see [6] ). If
(see [3] ). This topology is referred to as open-open topology (see [6] ). If 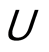 is compact, then
is compact, then 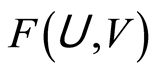 defines the subbases for the compact open topology on the set
defines the subbases for the compact open topology on the set .
.
The point open topology and the compact open topology are also open-open topologies. The set  endowed with set open topology
endowed with set open topology 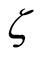 is written as
is written as 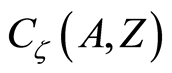 and is referred to as the underlying function space of the space
and is referred to as the underlying function space of the space  (see [3] ).
(see [3] ).
Definition 1.3 Let 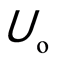 and
and  be open subsets of
be open subsets of  and
and  respectively. The set
respectively. The set 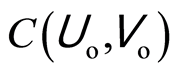 forms the subspace of the function space
forms the subspace of the function space  with the induced topology
with the induced topology 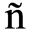 generated by the subbases
generated by the subbases  (see [7] ).
(see [7] ).
The following lemma and theorem are important for our consideration.
Lemma 1.4 In a regular space, if  is compact,
is compact, 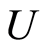 an open subset of a regular space and
an open subset of a regular space and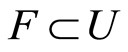 , then for some open set
, then for some open set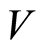 ,
, 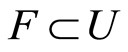 and
and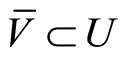 .
.
From the above lemma, the following inference is made. Let  where
where  is a class of compact subsets of
is a class of compact subsets of  and
and . Then for the space
. Then for the space  with compact open topology
with compact open topology ,
, 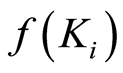 is a compact subset of
is a compact subset of . Since
. Since 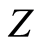 is a regular space, there exist open sets
is a regular space, there exist open sets , such that
, such that  and
and .
.
This implies that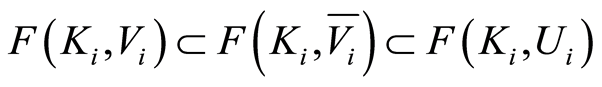 , in which the assertion
, in which the assertion 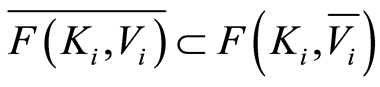 can be made (see [5] ).
can be made (see [5] ).
Theorem 1.5 The function 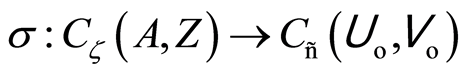 defined by
defined by 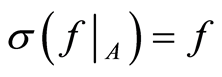 is a homeomorphism (see [7] ).
is a homeomorphism (see [7] ).
2. Lower Separation Axioms on the Underlying Function Space 
In this section, we show that the underlying function space 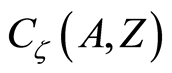 inherits the
inherits the  -separation axioms for
-separation axioms for 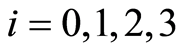 from the space
from the space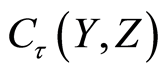 . Topologies
. Topologies 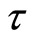 and
and 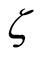 are both compact open.
are both compact open.
Theorem 2.1 Let the function space 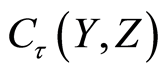 be a
be a  space. The function space
space. The function space  for
for  is a
is a  space.
space.
Proof. Let 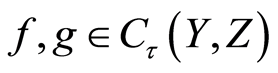 be distinct maps such that
be distinct maps such that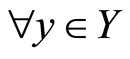 ,
,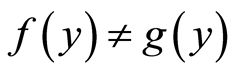 . Then
. Then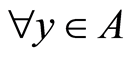 ,
, 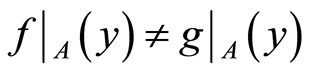 . For the open set
. For the open set  containing
containing 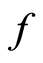 but not
but not 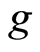 in
in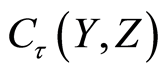 , the open set
, the open set
 in
in  contains
contains 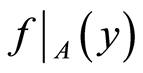 but not
but not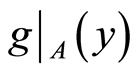 . Therefore the space
. Therefore the space  is a
is a 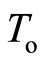 space. □
space. □
Theorem 2.2 Let the function space 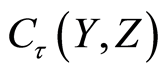 be a
be a 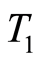 space. The function space
space. The function space 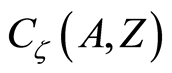 for
for  is a
is a  space.
space.
Proof. Let  be distinct maps such that
be distinct maps such that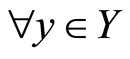 ,
, . Then
. Then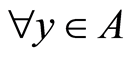 ,
,
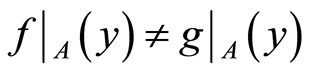 . For the open sets
. For the open sets  containing
containing  but not
but not  and
and 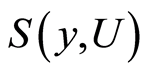 containing
containing 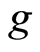 but not
but not  in
in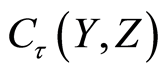 , the open sets
, the open sets

and

in  are neighborhoods of
are neighborhoods of  but not
but not 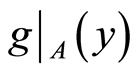 and
and  but not
but not 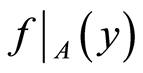 respectively. Therefore the space
respectively. Therefore the space  is a
is a 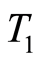 space. □
space. □
Theorem 2.3 Let the function space 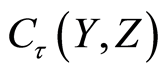 be a
be a  space. The function space
space. The function space  for
for  is a
is a 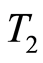 space.
space.
Proof. Let  be distinct maps such that
be distinct maps such that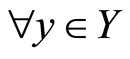 ,
,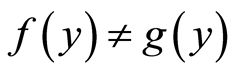 . Then
. Then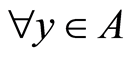 ,
,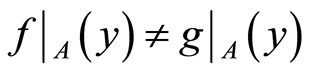 . For the disjoint open sets
. For the disjoint open sets  and
and 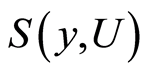 neighborhoods of
neighborhoods of 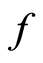 and
and  respectively in
respectively in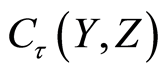 , the open sets
, the open sets

and

in  are disjoint neighborhoods of
are disjoint neighborhoods of 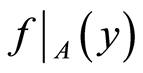 and
and  respectively. Therefore the space
respectively. Therefore the space 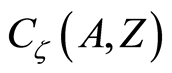 is a
is a 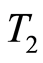 space. □
space. □
Theorem 2.4 Let the function space  be a regular space for a regular space
be a regular space for a regular space . The function space
. The function space 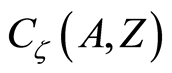 for
for  is a regular space.
is a regular space.
Proof. The space 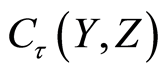 is regular for a regular space
is regular for a regular space  if for the open cover
if for the open cover 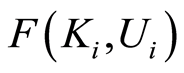 of
of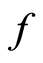 , there exist open sets
, there exist open sets  neighborhoods of
neighborhoods of 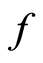 such that for
such that for 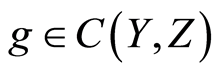 and
and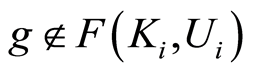 ,
, 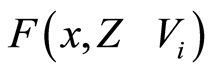 for some
for some  is a neighborhood of
is a neighborhood of  which does not intersect
which does not intersect  and
and
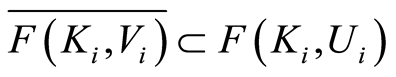 . For
. For ,
, 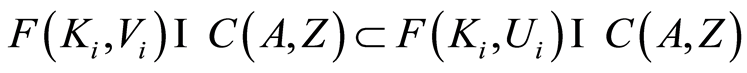 implying that
implying that , where
, where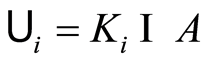 . For
. For 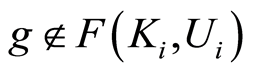 we have that
we have that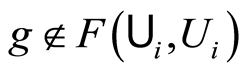 , implying that
, implying that  and for
and for ,
, . Therefore
. Therefore 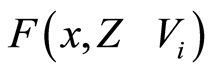 is a neighbourhood of
is a neighbourhood of 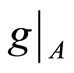 not intersecting
not intersecting .
. 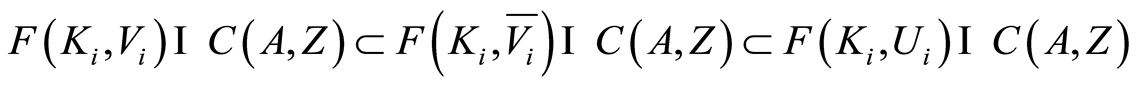 implies that
implies that . From the assertion
. From the assertion 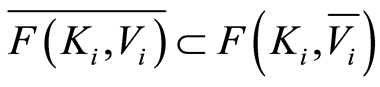 in Lemma 1.4, we have that
in Lemma 1.4, we have that . Therefore
. Therefore  and
and  are two disjoint open sets neighborhoods of
are two disjoint open sets neighborhoods of 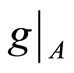 and
and  respectively. Hence the set
respectively. Hence the set 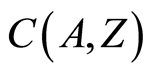 with the induced topology
with the induced topology 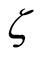 is a regular space. □
is a regular space. □
3. Conclusion
The underlying function space  inherits the
inherits the 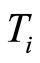 -separation axioms for
-separation axioms for 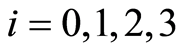 from the function space
from the function space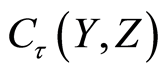 . From theorem 1.5, the underlying function space
. From theorem 1.5, the underlying function space 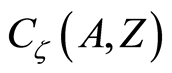 is homeomorphic to the subspace
is homeomorphic to the subspace  of the function space
of the function space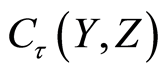 . This implies that the subspace
. This implies that the subspace 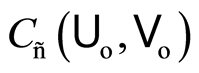 is a
is a  -space for
-space for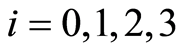 , if the function space
, if the function space 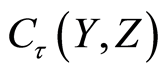 is a
is a 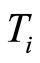 -space for
-space for . Therefore the
. Therefore the  -separation axioms for
-separation axioms for 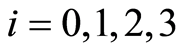 are hereditary on function spaces.
are hereditary on function spaces.
References
- Fox, R.H. (1945) On Topologies for Function Spaces. American Mathematical Society, 27, 427-432.
- Kelley, J.L. (1955) General Topology. Springer-Verlag, Berlin.
- Muturi, N.E., Gichuki, M.N. and Sogomo, K.C. (2013) Topologies on the Underlying Function Space. International Journal of Management, IT and Engineering, 3, 101-113.
- Arens, R.F. (1946) A Topology for Spaces of Transformations. The Annals of Mathematics, 2, 480-495. http://dx.doi.org/10.2307/1969087
- Willard, S. (1970) General Topology. Addison-Wesley Publishing Company, United States of America.
- Kathryn, F.P. (1993) The Open-Open Topology for Function Spaces. International Journal Mathematics and Mathematical Sciences, 16, 111-116. http://dx.doi.org/10.1155/S0161171293000134
- Muturi, N.E. (2014) Homeomorphism between the Underlying Function Space and the Subspace of the Function Space. Journal of Advanced Studies in Topology, 1, 57-60.

