ABSTRACT

The generalized Zakharov equation is a coupled equation which is a classic nonlinear mathematic model in plasma. A series of new exact explicit solutions of the system are obtained, by means of the first integral method, in the form of trigonometric and exponential functions. The results show the first integral method is an efficient way to solve the coupled nonlinear equations and get rich explicit analytical solutions.

Keywords:Generalized Zakharov Equation, First Integral Method, Exact Explicit Solutions

1. Introduction

The generalized Zakharov equation have been the focus of many researchers due to two facts: the system is a classic nonlinear mathematic model in plasma physics; the exact solutions to the system are widely applied in many scientific and engineering fields. The generalized Zakharov equation is a coupled equation written as 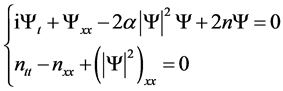[1]

(1)

where 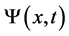is the envelope of the high-frequency electric field, 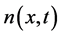is the plasma density measured from its equilibrium value, 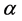is a real coefficient, x and t are 1-dimensional space and time coordinate, respectively. Up to now, many methods have been used to solve the exact solution of the system (1) such as rational auxiliary equation method [2] , F-expansion method [3] , and Li et al. obtained the generalized solitary solutions by exp-function method [4] [5] , Hong got the doubly periodic solutions by the generalized Jacobi elliptic function expansion method [6] , M. Javidi constructed dark and bright solitary wave solutions by a variational iteration method [7] . Besides, Guo discussed the existence and uniqueness of smooth solution [8] , Gambo investigates the dynamical behavior [9] , S. Abbasbandy solved the numerical solutions [10] of the system (1).

The first integral method is based on the ring theory of commutative algebra, the pioneer work can be traced to Feng, he first proposed the first integral method for solving Burgers-KdV equation [11] and then further developed it. Recently, Bin Lu applied this method to construct travelling wave solutions of the (2 + 1)-dimensional BKK system and (3 + 1)-dimensional Burgers equation [12] , Hodsein et al. also reported new solutions of the Davey-Stewartson equation by using this method [13] , the method has also been successfully adopted for solving some important complex partial differential equations in [14] -[23] .

In order to explore new analysis solutions to the system (1), we attempted to use the first integral method to solve the generalized Zakharov equation for the first time. The rest of the paper proceeds as follows: In Section 2, we briefly introduce the first integral method. In Section 3, we apply the method to the generalized Zakharov equation, and give the exact explicit solutions under two different cases. Finally, some conclusions are given in Section 4.

2. The First Integral Method

Consider the nonlinear partial differential equation (NLPDE) in the form:

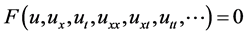(2)

where 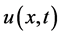is the solution of Equation (2).

First, we use the travelling wave transformation

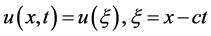(3)

where c is a constant to be determined later, then the NLPDE is reduced to a nonlinear ordinary differential system

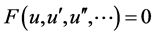(4)

where the prime denotes the differential with respect to.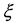

Next, we introduce new independent variables

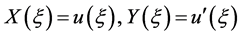(5)

then (4) can be converted to a system of the nonlinear ordinary differential system as follows

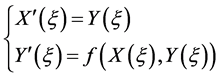(6)

According to the qualitative theory of ordinary differential equations, the general solutions to (6) can be solved directly if we can find two integrals to (6) under the same conditions. However, it is really difficult to realize this even for one first integral, because for a given autonomous system, there is no systematic theory about how to find its first integral, nor is there a logical way could tell what these first integrals are. A key idea of our approach here is to apply the Division Theorem to obtain one first integral to (6) which reduces (4) to a first-order integrable ODE, then the exact solutions for (2) will be obtained by solving this equation. Now, let’s recall the Division Theorem.

Division Theorem Suppose that are polynomials in complex domain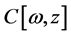, and 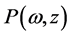is irreducible in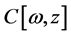. If vanishes at all zero points of, then there exists a polynomial 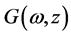in 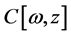such that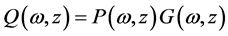

3. New Exact Explicit Solutions of the Generalized Zakharov Equation

In order to seek the exact solutions of system (1), we assume

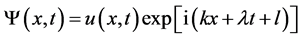(7)

Substituting (7) into (1) and yields:

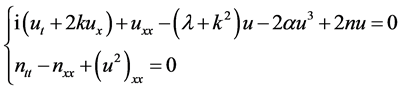(8)

Using the transformations

(9)

where 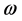is a nonzero constant, then (8) further reduced to

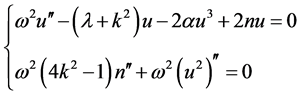(10)

where “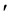” denotes.

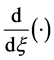Integrating Equation (10b) with respect to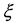, and taking the integration constant as zero yields

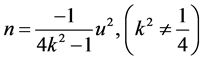(11)

Substituting (11) into (10a) and yields:

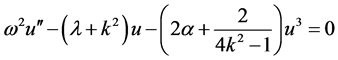(12)

Now, we introduce new independent variables 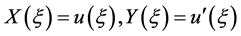which change Equation (12) to a dynamical system given by

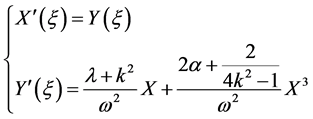(13)

Applying the Division Theorem to seek the first integral to (13). Suppose that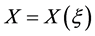, 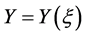are nontrivial solutions to (13), and 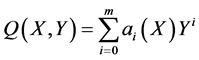is an irreducible polynomial such that

(14)

where are polynomials of X and all relatively primes,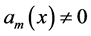. Equation (14) is called the first integral to (13). Note that 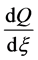is a polynomial of X and Y, and 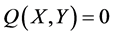implies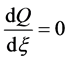. Due to the Division Theorem, there exists a polynomial 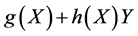such that

(15)

We will take two different cases into consideration in the following, assuming that 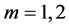in Equation (15).

Case A:

Suppose that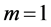, by equating the coefficients of 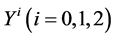on both sides of (15), we have

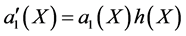(16a)

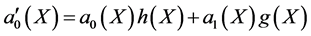(16b)

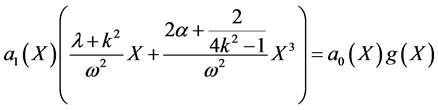(16c)

Since 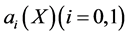are polynomials in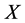, then from (16a) we deduce that 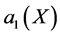is a constant and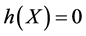. For simplicity, we take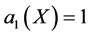. Balancing the degrees of 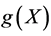and in (16b), we conclude that 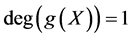only. Suppose that 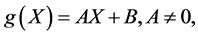then from Equation (16b) we find

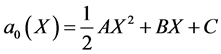(17)

where C is an arbitrary integration constant.

Substituting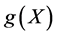, 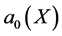and into Equation (16c) and setting all the coefficients of powers x to be zero, we obtain a system of nonlinear algebraic equations

(18)

By solving it, we obtain

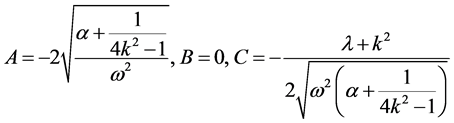(19a)

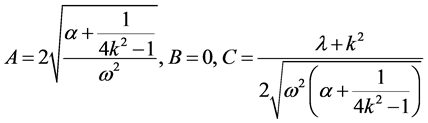(19b)

Using the conditions (19a), (19b) in (14) respectively, we obtain

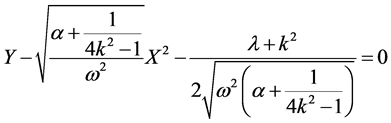(20a)

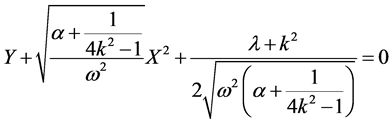(20b)

Combining (20a) with (13), we obtain the exact solutions to (13) as follows

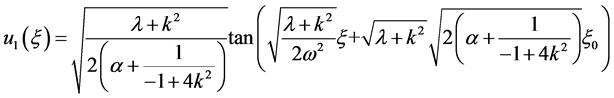(21)

where 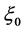is an arbitrary constant.

Then the exact solutions to the system (1) can be written as

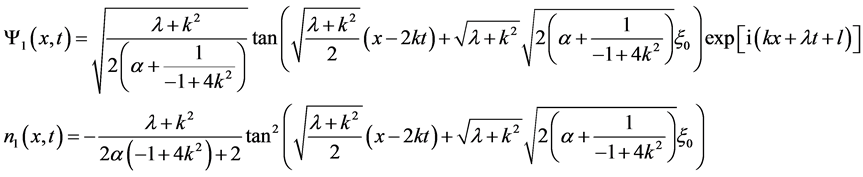(22)

Similarly, in the case of (20b), we obtain

(23)

where 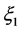is an arbitrary constant.

Then the exact solutions to the system (1) are given by

(24)

We can get distinctive solutions by giving different values to.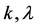

Case B:

Suppose that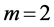, by equating the coefficients of 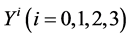on both sides of (15), we have

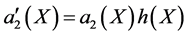(25a)

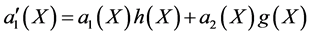(25b)

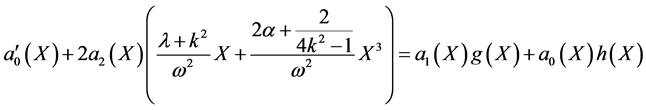(25c)

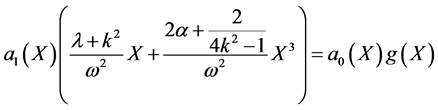(25d)

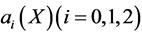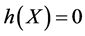Since are polynomials, then from (25a) we deduce that is a constant and. For simplicity, we take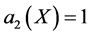. Balancing the degrees of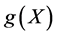, 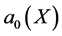and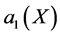, we conclude that 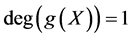or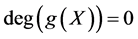. Actually, if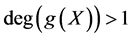, suppose that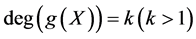, then from (25b)-(25c), we know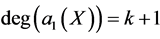, , 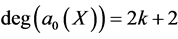and from (25d), we have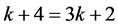, and then 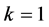which is contrary to.

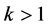Case 1 When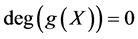, we take, then from (25b)-(25c), we find

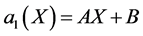
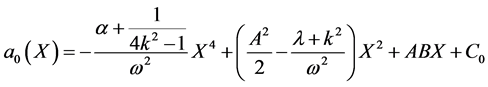
where B, C₀ is arbitrary integration constant.

Substituting, 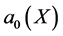and 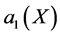into (25d) and setting all the coefficients of powers of X to be zero, we obtain a system of nonlinear algebraic equations

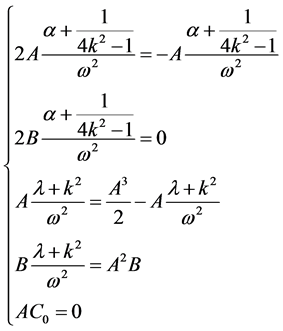(26)

By solving it, we obtain

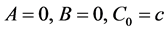(27)

where 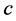is an arbitrary constant.

Using the conditions (27) in (14), we obtain

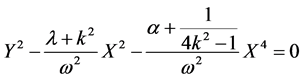(28)

Combining (28) with (13), we obtain the exact solutions to (13) as follows

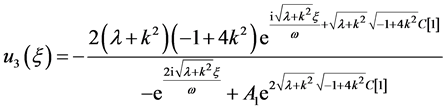(29a)

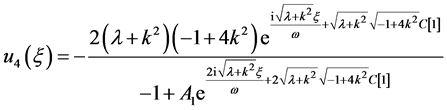(29b)

Then the exact solutions to the generalized Zakharov equation can be written as

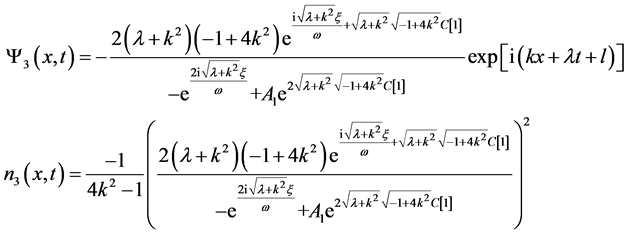(30)

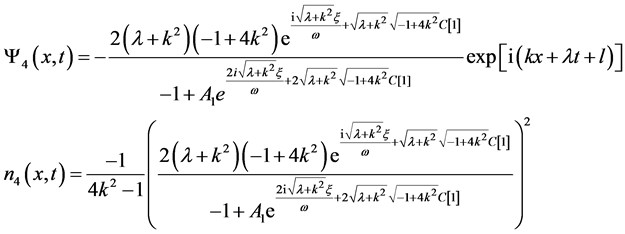(31)

Comparing the results with the works studied before, it can be seen these are new results for the system (1).

Case 2 When, take, then from (25b)-(25c), we find

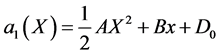
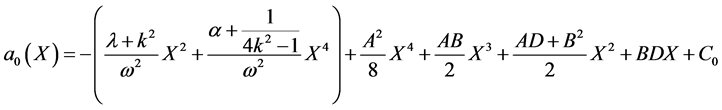
Substituting, 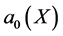and 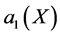into (25d), and setting all the coefficients of powers of X to be zero, we obtain a system of nonlinear algebraic equation

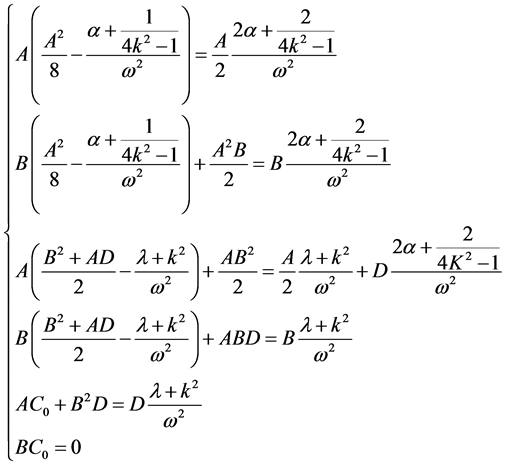(32)

By solving it, we obtain

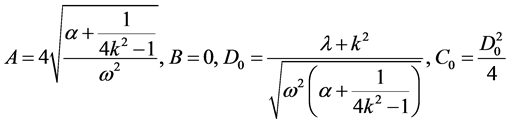(33a)

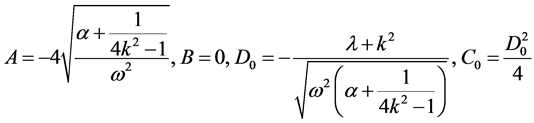(33b)

Using the conditions (33a), (33b) in (14) respectively, we obtain

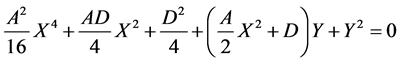(34)

i.e.

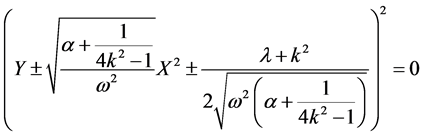(35)

Combining (35) with (13), we obtain the exact solutions to (13) which were same with the case m = 1.

4. Conclusion

In this paper, we discussed how to construct the exact solutions for the generalized Zakharov equation by using the first integral method. Many new exact explicit solutions with arbitrary constant, peaked wave solutions are obtained, they may be important for the explanation of some practical physical problems. The performance of the method shows it is reliable and effective to give more exact solutions, we deduce that the method can be extended to solve many systems of nonlinear PDE which are arising in the theory of soliton and other fields.

Acknowledgements

This study was technically supported by the National Natural Science Foundation of China under Grant No. 11371267 and the Scientific Research Foundation of the Education Department of Sichuan Province of China under Grant No. 12ZA135.

References