 Theoretical Economics Letters, 2013, 3, 283-287 http://dx.doi.org/10.4236/tel.2013.35047 Published Online October 2013 (http://www.scirp.org/journal/tel) Matched Charitable Contributions: Comparative Statics and Equilibrium Daniel K. Biederman Department of Economics, University of North Dakota, Grand Forks, USA Email: daniel.biederman@business.und.edu Received August 8, 2013; revised September 6, 2013; accepted September 13, 2013 Copyright © 2013 Daniel K. Biederman. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. ABSTRACT A small optimizing agent max imizes his utility by allocati ng his inco me to private consumption an d to a contribution to a particular charity. The agent’s contribution may be matched, at a particular rate, by a large ag ent. We provid e a com- prehensive comparative-statics analysis of the agent’s prob lem, allowing for change s in the agent’s income, the agent’s conjecture about other agents’ contributions, and the match rate. A Nash equilibrium among n such agents is shown to exist if private consumption is a normal good for all agents. The equilibrium is unique if private consumption and charitable giving are normal goods for all agents. Keywords: Charitable Contributions; Match Rate; Monotonic Transformation; Nash Equilibrium 1. Introduction Fundraising efforts by charities sometimes feature an offer to match individual contributions at a particular rate, commonly called the match rate. Solicitations may in- form potential contributors that a certain benefactor has offered to contribute, say, 50 cents or one dollar or two dollars for every dollar contributed by others. To the ex- tent, match offers and other fundraising techniques are successful in generating higher levels of contributions than otherwise would be observed, they enhance the sup- ply of certain privately-provided public goods, thereby mitigating th e undersupp ly of such goo ds. How ever, only in recent years, economists began to devote considerable efforts to the study of fundraising methods. Karlan and List [1, p. 1774] note that fundraisers are “typically long on rules of thumb and short on hard scientific evidence, divided as to the most efficient means to attract ... dol- lars.” We are concerned, in this paper, with matched contri- butions.1 We formulate a model in which each of “small,” purely-altruistic, optimizing agents allocates his income to private consumption and a contribution to a particular charitable organization. The agent is aware that his contribution, as well as the contributions of others, will be matched at a particular rate. They formulate a Nash conjecture about the total ch aritable contribution of the other n 1n small agents. Given values of his in- come, his Nash conjecture, and the match rate, the agent maximizes his utility subject to his budget constraint. The purpose of the paper is twofold. First, we aim to fill a gap in the existing literature by providing a com- prehensive comparative-statics analysis of the problem faced by an agent whose charitable contribution is matched. We derive relatively simple, elasticity-based expressions for the partial derivatives of the agent’s charitable contribution with respect to a change in his income, a change in his conjecture about other agents’ contributions, and a change in the match rate. The signs of these partial derivatives are directly related to the elas- ticities of the agent’s marginal rate of substitution with respect to changes in the agent’s private consumption and in the total contribution to the charity. Second, we apply our comparative-statics results to establish conditions that ensure existence and uniqueness of Nash equilibrium among the agents. These condi- tions overlap, in some measure, the conditions obtained by Bergstrom, Blume, and Varian [9] for the existence and uniqueness of Nash equilibriu m for a class of models in which a public go od is privately-provided . Our results generalize the Bergstrom et al. resu lts in th e sen se th at, i f we set the net match rate equal to zero in our model, we obtain, essentially, the Bergstrom et al. model. Further- n 1Previous theoretical and empirical studies of matched contributions include Andreoni [2], Eckel and Grossman [3], Gong and Grundy [4], Huck and Rasul [5], Karlan, List, and Shafir [6], Meier [7], and Ron- deau and List [8]. C opyright © 2013 SciRes. TEL 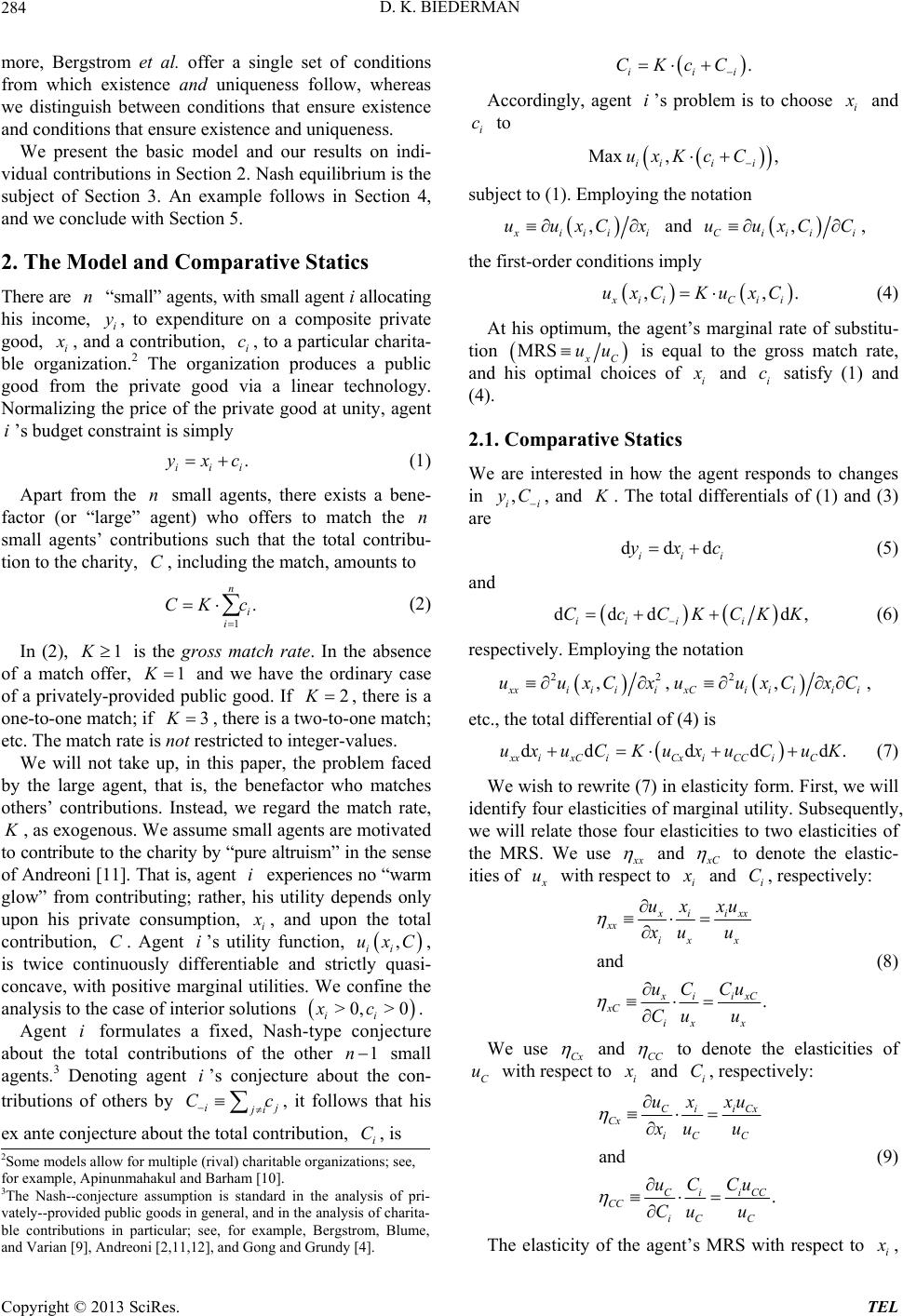 D. K. BIEDERMAN 284 more, Bergstrom et al. offer a single set of conditions from which existence and uniqueness follow, whereas we distinguish between conditions that ensure existence and conditions that ensure existence and uniqueness. We present the basic model and our results on indi- vidual contributions in Section 2. Nash equilibrium is the subject of Section 3. An example follows in Section 4, and we conclude with Section 5. 2. The Model and Comparative Statics There are “small” agents, with small age nt i allocating his income, i, to expenditure on a composite private good, i ny , and a contribution, i, to a particular charita- ble organization.2 The organization produces a public good from the private good via a linear technology. Normalizing the price of the private good at unity, agent ’s budget constraint is simply c i . (1) ii yxc i Apart from the small agents, there exists a bene- factor (or “large” agent) who offers to match the small agents’ contributions such that the total contribu- tion to the charity, , including the match, amounts to n C n 1. n i i CK c (2) In (2), 1 is the gross match rate. In the absence of a match offer, 1 and we have the ordinary case of a privately-prov ided public good. If 2 , there is a one-to-one match; if , there is a two-to-one match; etc. The match rate is not restricted to integer-values. 3K We will not take up, in this paper, the problem faced by the large agent, that is, the benefactor who matches others’ contributions. Instead, we regard the match rate, , as exogenous. We assume small agents are motivated to contribute to the charity by “pure altruism” in the sens e of Andreoni [11]. That is, agent experiences no “warm glow” from contributing; rather, his utility depends only upon his private consumption, i i , and upon the total contribution, . Agent ’s utility function, C i , ii uxC >0, >0 ii xc , is twice continuously differentiable and strictly quasi- concave, with positive marginal utilities. We confine the analysis to the case of interior solutions . Agent formulates a fixed, Nash-type conjecture about the total contributions of the other i1n small agents.3 Denoting agent ’s conjecture about the con- i tributions of others by ij i C c , it follows that his ex ante conjecture about the total contribution, , is i C . ii CKcC i Accordingly, agent ’s problem is to choose ii and to i c Max ,, iii i uxKc C subject to (1). Employing the notatio n , iii i uuxCx and , Ciii uuxCC i , the first-order conditions imply , ,. ii Cii uxC KuxC (4) At his optimum, the agent’s marginal rate of substitu- tion MRS C uu is equal to the gross match rate, and his optimal choices of i and satisfy (1) and (4). i c 2.1. Comparative Statics We are interested in how the agent responds to changes in , i yC i , and . The total differentials of (1) and (3) are K ddd ii yx i c (5) and ddd d ii ii CcCKCK ,K (6) respectively. Employing th e notation 222 ,, , xiiii xCiiiii uuxCxuuxCxC , etc., the total differential of (4) is dddd d xxixCiCxi CCiC uxu CKuxuCuK . (7) We wish to rewrite (7) in elasticity form. First, we will identify four elasticities of marginal utility. Sub sequently, we will relate those four elasticities to two elasticities of the MRS. We use x and C to denote the elastic- ities of u with respect to i and , respectively: i C and . xi ixx xx ix x xi ixC xC ix x ux xu xuu uC Cu Cu u (8) We use Cx and CC to denote the elasticities of with respect to C ui and , respectively: i C and . Ci iCx Cx iC C Ci iCC CC iCC ux xu xu u uC Cu Cu u (9) 2Some models allow for multiple (rival) charitable organizations; see, for example, Apinunmahakul and Barham [10]. 3The Nash--conjecture assumption is standard in the analysis of pri- vately--provided public goods in general, and in the analysis of charita- le contributions in particular; see, for example, Bergstrom, Blume, and Varian [9], Andreoni [2,11,12], and Gong and Grundy [4]. The elasticity of the agent’s MRS with respect to i , Copyright © 2013 SciRes. TEL  D. K. BIEDERMAN 285 which we denote by , is equal to xCx : 2. xC i xixC CxxxCx iC xCx xC uu x xuu uuuu xu uu C (10) Similarly, the elasticity of the agent’s MRS with re- spect to , which we denote by i C , is equal to CCC : 2. xC i CixC CxCxCC iC CCC xC uu C Cuu uuuu Cu uu (11) Using (4) and (8)-(11), we can r ewrite (7) as follows: dd d. ii xC ii xC CK (12) We use (5), (6), and (12) to obtain d 1d ii KxC K dd , ixiiC Cii cCy Cx K (13) where . Ci xi xC (14) Using (13), we easily obtain the partial derivatives that are central to our comparative-statics analysis: , iii x cy C (15) , Cii i cC Kx (16) 1. Ciii cK CxK (17) The second-order sufficient condition for a maximum is >0 , and that condition is ensured by the assump- tion of strictly-quasiconcave utility.4 In view of (15), >0 ii cy if and only if (“iff”) <0 x . This is the case when the agent’s MRS, at the optimum, decreases with his private consumption. Fur- thermore, using (14) and (16), we can determine that <0 x iff >1 ii cC . Thus, to say that an agent’s charitable contribution, i, is normal is to say that when that agent conjectures an increase in the contributions of other agents, his best reply is such that his conjecture about the total contribution, , increases. c i C Noting that 1 iiii yc y , it follows from (14) and (15) that >0 ii xy iff C>0. This is the case when the agent’s MRS, at the optimum, increases with his conjecture about the total contribution. Using (16), it follows as well that <0 ii cC iff >0. C To say that an agent’s private consumption is normal, then, is to say that an increase in his conjecture about others’ con- tributio n s prompts him to decreas e h i s own contribution. From (17) it is clear that the agent will increase his contribution in response to an increase in the match rate iff his MRS is inelastic with respect to his conjecture about the total contribution (that is, iff <1 C ). We showed directly above that >0 C is necessary and sufficient for normality of private consumption and for a decreasing best reply. However, we have no a priori knowledge about the sign of 1C c . Indeed, it seems entirely reasonable to believe that, depending on an agent’s income and preferences, i may increase, de- crease, or remain unchanged in response to an increase in the match rate.5 We can summarize our comparative-statics results as follows. For agent , we have i 0< <1,0< <1 ii ii xy cy , and 1< <0 ii cC iff <0 x and >0 C . It is worth noting that these last two inequalities also ensure satisfaction of the second- order sufficient condition for the agent’s problem. 2.2. ALEP Complementarity For a particular agent, private consumption and the total charitable contribution are said to be Auspitz-Lieben- Edgeworth-Pareto (ALEP) complements if , and weak ALEP complements if . It has been widely-acknowledged that the primary limitation of this notion of complementarity, which originated with Aus- pitz and Lieben [13], is that, in general, the signs of the second-order partial derivatives of a utility function are not invariant with respect to monotonic transformations. Nevertheless, in many problems the assumption of ALEP complementarity (or weak ALEP complementarity) al- lows for stronger comparative-statics results than are obtainable without the assumption; see, for example, Chipma n [14] and Weber [15]. >0 xC u 0 xC u In our model, if we assume that marginal utilities are diminishing ( and ) and that , then <0 xx u<0 CC u0 xC u <0 x and >0 C and, consequently, all of the desirable” comparative-statics results—normality of i and of , etc.—are ensured. However, the assumption that is too strong. One can find strictly-quasi- concave utility functions for which and for which i C0 xC u ,uxC <0 xC u <0 x and >0 C . Moreover, the elasticities and C are invariant to monotonic transformations. Suppose we start with a utility function ,uxC x for which CC , and , so that <0, <0uu xx 0 xC u<0 and >0 C . A monotonic 4Ordinarily, the quasiconcavity condition for the problem at hand would be written as . It can be shown that 2 2 xxxC CC uKuKu 5Without probing the matter in detail, Gong and Grundy [4] note that individual contributions can either increase or decrease with the match rate. We provide an illustrative example in Section 4 below. >0 2 2xC CC Ku Ku ii xxx Cx uu . Copyright © 2013 SciRes. TEL  D. K. BIEDERMAN 286 transformation ,uxC uxC , (where >0 ) may or may not pr eserve the inequality , but it is certain to preserve the values of the MRS elasticities. In turn, it follows that the transformation preserves the agent’s demand func t ions. 0 xC u C 3. Nash Equilibrium In this section we apply the comparative-statics results derived in Section 2 to the existence and uniqueness of Nash equilibrium among the agents. n cPropo sition 1. Suppose i is continuous in i for all . Then, for given values of 1,2, ,in 2, ,1, i in and : K (A) a Nash equilibrium exists if private consumption is a normal good for all agents; (B) a unique Nash equilibrium exists if private con- sumption and the charitable contribution are normal goods for all agents. Proof. We apply Theorems 2.7 and 2.8 from Vives [16]. In addition to the continuity requirement, it is re- quired that agents’ best replies depend only on the ag- gregate actions of other agents. This requirement is clearly met. A further requirement is that agents’ strategy spaces must be compact. This requirement is met since i for all i. Then Vives’ Theorem 2.7 asserts existence of an equilibrium if best replies are strictly de- creasing, as is the case when i 0i cy is normal. This proves (A). Vives’ Theorem 2.8 asserts existence and unique- ness of equilibrium, provided 1< i <cC 0 ii for all , as is the case when i and are normal. This proves (B). Q.E.D. i c Note that Proposition 1 was established without regard to the sign or magnitude of i cK . However, we can establish existence of equilibrium for cases in which agents respond to an increase in the match rate by de- creasing their individual contributions or by leaving them unchanged. Proposition 2. Suppose is continuous in ii c C and 0 i cK for all . Then, for given values 1, ,i 2,n of 1,2, , i in and , a Nash equilibrium exists. K Proof. It follows from (16) and (17) that if 0 i cK, then <0 ii cC . Applying Vives’ [16] Theorem 2.7, we then have existence of an equilibrium. Q.E.D. As we noted in Section 1, our results on Nash equilib- rium are related to the results obtained by Bergstrom, Blume, and Varian [9] for the case of a privately-pro- vided good. Again, however, the Bergstrom et al. model does not focus specifically on charitable contributions, and as such there is no match rate in that model. Fur- thermore, Bergstrom et al. offered a single set of condi- tions that ensures existence and uniqueness of equilib- rium, whereas we have disentangled that set of condi- tions. 4. An Example Consider the constant-elasticity (CE) utility function: 11 1 if 01 or if 1 ,1 lnln if 1 i i i xC uxC xC where >0 . Let 1 . Using (1) and (4), agent ’s optimal contribution is given by i 1 1. i i yKC cK i (18) 4.1. Comparative Statics It is straightforward to show, for the CE function, that x and C . Expressions for the partial de- rivatives , iii i cycC , and i cK can be ob- tained either through direct differentiation of (18) or by the application of (14) - (17). We present the partials in both forms: 1, i ii ii C cy xC K (19) 1 1i ii ii x K cC xC K (20) 2 1 11. ii ii iii yC Cx cK Kx C KK It is clear that 0< <1 ii cy and 1< <0 ii cC for all values of , and that 0 i cK as (that is, as ). 1 1 4.2. ALEP Complementarity The CE utility function is additively separable, with u 0 xC u . Accordingly, we refer to and as ALEP independents in this case. Now let C 1 uuu . This increasing (and concave) transformation results in 3 2 xCi i uuxC 0 , so x and are now ALEP C substitutes. Nevertheless, the MRS elasticities are un- changed (xx and CC ), leaving the agent’s demand functions u nchange d as well. 4.3. Nash Equilibrium If all agents’ utility functions are of the CE type, then private consumption and the charitable contribution are Copyright © 2013 SciRes. TEL  D. K. BIEDERMAN Copyright © 2013 SciRes. TEL 287 [3] C. C. Eckel and P. J. Grossman, “Subsidizing Charitable Contributions: A Natural Field Experiment Comparing Matching and Rebate Subsidies,” Experimental Econom- ics, Vol. 11, No. 3, 2008, pp. 234-252. http://dx.doi.org/10.1007/s10683-008-9198-0 normal for all agents, and Proposition 1, Part B asserts existence and uniqueness of Nash equilibrium. Suppose, for instance, that the agents are identical in that they all have the same CE utility function and the same level of income, . Setting n y 1 i Cn i in (18), we ob- tain the Nash-equilibrium contribution, , for each agent: c* c[4] N. Gong and B. D. Grundy, “Charitable Fund Raising: Matching Grants or Seed Money,” Unpublished Manu- script, 2011. * 1. y cnK [5] S. Huck and I. Rasul, “Matched Fundraising: Evidence from a Natural Fie ld Expe riment,” Journal of Public Eco- nomics, Vol. 95, No. 5-6, 2011, pp. 351-362. http://dx.doi.org/10.1016/j.jpubeco.2010.10.005 It can be verified that the equilibrium total contribu- tion, , increases with , irrespective of the value of * CKnc[6] D. Karlan, J. A. List and E. Shafir, “Small Matches and Charitable Giving: Evidence from a Natural Field Expe- riment,” Journal of Public Economics, Vol. 95, No. 5-6, 2011, pp. 344-350. http://dx.doi.org/10.1016/j.jpubeco.2010.11.024 *K . 5. Summary and Conclusions [7] S. Meier, “Do Subsidies Increase Charitable Giving in the Long Run? Matching Contributions in a Field Experi- ment,” Journal of the European Economic Association, Vol. 5, No. 6, 2007, pp. 1203-1222. http://dx.doi.org/10.1162/JEEA.2007.5.6.1203 We have focused on purely-altruistic, utility-maximizing agents who allocate their incomes to private consumption and to charitable contributions that are matched at an exogenous rate. W e showed that the nature of an agent’s response to a change in his income is inexorably linked to the nature of his best reply to a change in the contribu- tions of other agents. We also derived a general charac- terization of an agen t’s response to a chang e in the match rate in terms of the elasticity of the agent’s MRS with respect to a change in his conjecture about the total con- tribution. These comparative-statics results are useful in establishing conditions that ensure existence and unique- ness of Nash equilibrium for the case of agents. n [8] D. Rondeau and J. A. List, “Matching and Challenge Gifts to Charity: Evidence from Laboratory and Natural Field Experiments,” Experimental Economics, Vol. 11, No. 3, 2008, pp. 253-257. http://dx.doi.org/10.1007/s10683-007-9190-0 [9] T. Bergstrom, L. Blume and H. Varian, “On the Private Provision of Public Goods,” Journal of Public Economics, Vol. 29, 1986, pp. 25-49. http://dx.doi.org/10.1016/0047-2727(86)90024-1 [10] A. Apinunmahakul and V. Barham, “Strategic Interaction and Charitable Fundraising,” Modern Economy, Vol. 3, 2012, pp. 338-345. http://dx.doi.org/10.4236/me.2012.33044 A more general model of matched contributions might allow for an endogenous match rate. For example, the match rate might be chosen by a utility-maximizing benefactor who is cognizant of how small contributors respond to changes in that rate. In addition, agents may be driven to contribute by motives other than pure altru- ism. Finally, in an environment that features heteroge- neous agents, so me of those ag ents may find it op timal to refrain altogether from contributing at certain match rates. Consideration of these factors makes for ongoing re- search. [11] J. Andreoni, “Giving with Impure Altruism: Applications to Charity and Ricardian Equivalence,” Journal of Politi- cal Economy , Vol. 97, No. 6, 1989, pp. 1447-1458. http://dx.doi.org/10.1086/261662 [12] J. Andreoni, “Toward a Theory of Charitable Fund- Raising,” Journal of Political Economy, Vol. 106, No. 6, 1998, pp. 1186-1213. http://dx.doi.org/10.1086/250044 [13] R. Auspitz and R. Lieben, “Untersuchungen über die Theorie des Preises,” Duncker & Humblot, Leipzig, 1889. [14] J. S. Chipman, “An Empirical Implication of Auspitz- Lieben-Edgeworth-Pareto Complementarity,” Journal of Economic Theory, Vol. 14, No. 1, 1977, pp. 228-231. http://dx.doi.org/10.1016/0022-0531(77)90096-5 REFERENCES [1] D. Karlan and J. A. List, “Does Price Matter in Charitable Giving? Evidence from a Large-Scale Natural Field Ex- periment,” American Economic Review, Vol. 97, No. 5, 2007, pp. 1774-1793. http://dx.doi.org/10.1257/aer.97.5.1774 [15] C. E. Weber, “Two Further Implications of Auspitz-Lie- ben-Edgeworth-Pareto Complementarity,” Economics Let- ters, Vol. 67, No. 3, 2000, pp. 289-295. http://dx.doi.org/10.1016/S0165-1765(99)00277-3 [2] J. Andreoni, “Leadership Giving in Charitable Fund-Rais- ing,” Journal of Public Economic Theory, Vol. 8, No. 1, 2006, pp. 1-22. http://dx.doi.org/10.1111/j.1467-9779.2006.00250.x [16] X. Vives, “Oligopoly Pricing: Old Ideas and New Tools,” MIT Press, Cambridge, 1999.
|