Advances in Pure Mathematics
Vol.06 No.13(2016), Article ID:72773,18 pages
10.4236/apm.2016.613073
Infinite Number of Twin Primes
S. N. Baibekov, A. A. Durmagambetov
L.N. Gumilyov Eurasian National University, Astana, Kazakhstan

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: October 6, 2016; Accepted: December 12, 2016; Published: December 15, 2016
ABSTRACT
This work is devoted to the theory of prime numbers. Firstly it introduced the concept of matrix primes, which can help to generate a sequence of prime numbers. Then it proposed a number of theorems, which together with theorem of Dirichlet, Siegel and Euler allow to prove the infinity of twin primes.
Keywords:
Prime Numbers, Twin Primes, Composite Numbers, Natural Numbers, Algorithms, Arithmetic Progression, Prime Numbers Matrix, Special Factorial, Generation of Prime Numbers

1. Introduction
A problem of twin prime numbers infinity formulated at the 5th International Mathematical Congress is one of the main problems of the theory of prime numbers that has not been solved for 2000 years. It has been known that twin prime numbers are pairs of prime numbers which differ from each other by 2. For example, numbers 11 and 13, and numbers 17 and 19 are twin primes, but next adjacent prime numbers 37 and 41 are not twin primes. This problem is also known as the second Landau’s problem.
In the year 2013 American mathematician Zhang Yitang from the University of New Hampshire has proved that there are an infinite number of pairs of prime numbers, separated by a fixed distance is greater than 2 but less than 70 million. That is, the number of pairs of twin primes 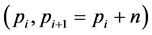 is infinite, where
is infinite, where 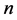 is greater than 2 but less than or equal to 70,000,000. James Maynard later improved this result to 600. In 2014, scientists led by Terence Tao (Polymath project) this result was improved up to 246 [1] .
is greater than 2 but less than or equal to 70,000,000. James Maynard later improved this result to 600. In 2014, scientists led by Terence Tao (Polymath project) this result was improved up to 246 [1] .
A goal of this paper is to prove infinity of twin primes.
To solve this problem, we have proposed a new method, which allows to empirically estimate infinity of twin primes [2] . In this paper we present another simple proof, which provides more correct results for twin primes infinity.
First, for convenience we introduce the following notation. As is known, a sequential
multiplication of all integers up to a certain number n is called as factorial: 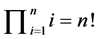
Hereafter a sequential multiplication of prime numbers will be occurred frequently, therefore for such cases we use the following notation:

Here 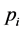 is a prime number with index number
is a prime number with index number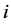 . A combination of symbols
. A combination of symbols 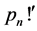 means a sequential multiplication of prime numbers from 2 to
means a sequential multiplication of prime numbers from 2 to  only. We shall call it as a special factorial of a prime number
only. We shall call it as a special factorial of a prime number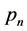 . For example,
. For example,  is a special factorial of prime number
is a special factorial of prime number 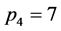 or
or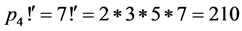 .
.
2. Matrices of Prime Numbers
In this paper we try to prove the infinity of number of twin primes. The proof will be on the basis of properties matrix of prime numbers.
The development of these matrices is implemented as follows.
Let we represent a set of natural numbers in a form of  matrices family with elements a(k, I, j), where i is a row index number, j is a column index number, and k is an indexing number of matrix
matrices family with elements a(k, I, j), where i is a row index number, j is a column index number, and k is an indexing number of matrix .
.
Here, a maximum number of rows of matrix  must be equal to the special factorial
must be equal to the special factorial 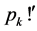 i.e.
i.e. . This means, that for every matrix with index number k there is a specific set of prime number sequence:
. This means, that for every matrix with index number k there is a specific set of prime number sequence: 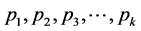 (note that the last prime number, which corresponds to this matrix is
(note that the last prime number, which corresponds to this matrix is
Here and further it is supposed that we don’t know any prime number. Prime numbers will be generated in the course of creating matrices
First, we show how matrix 






The numbers located in the second row of new matrix, also form an arithmetic progression. The first term and common difference of this progression are 3 and 2 respectively, i.e.
Figure 1. Matrices of prime numbers.
As previously defined, number 2 is a prime number. Therefore, all numbers divisible by 2 are composite numbers. In view of this, all numbers, except 2, which are located in the first row of the considered matrix (Figure 1, 

This implies that number 1 is not a prime number, otherwise all numbers divisible by 1 would be composite numbers. Number 1 is also not a composite number, since it is not divided by other numbers. That is why number 1 is located separately in the upper left corner in this and other matrices.
3. Algorithm for Matrix Transformation from One to Another Type
It is seen from matrix 


Thus, we transform matrix 





For transforming a matrix from one into another type a simple method is used. Implementation of this method lies in a simple transposition of numbers of certain rows and columns of the original matrix into corresponding rows and columns of a new matrix. For example, for forming a first column of matrix 





Note, that in the new matrix (Figure 1, 

In the new matrix all numbers located in each row, as in the case of matrix

The common difference of this arithmetic progression is equal to

As you can see, all the numbers of this row are divisible by 3. Therefore, they (except number 3) are composite numbers. In a view of this they are dark painted in matrix 


It should be pointed out that here and in all next figures, letter a denotes those matrices (e.g. 





It should be noted that during the process of the second matrix transformation, all numbers divisible by 3, are finally determined and dark painted accordingly.
It is seen from matrix 







For example, for forming the first column of matrix 




For forming a second column of matrix


A maximum number of rows in third matrix should be equal to a special factorial of the third prime number

For matrix 
From this expression, we obtain that all the numbers located in the fourth row are divisible by 5, and those numbers that located in the 24th row are also divisible by 5. In this context, all of them are accordingly transposed into the series of composite numbers and repainted into dark color (except a prime number 5). Here, in the case of the third matrix (Figure 1, 
Note that in all cases, there is no any strict regularity for location of painted and unpainted numbers within one column of any considered matrix. But a picture of mutual arrangement of these numbers within one column is repeated with perfect precision in the next columns (starting from the second column). This regularity of repeating pictures by columns is appeared when each previous matrix 



In matrix 




In this case, carrying out a number of similar operations, as in previous cases, we can finally identify a set of all composite numbers, which should be divisible by 7. Similarly, we can build other matrices.
Here we have presented a procedure that allows to perform mechanical transformation of prime numbers matrices from one type to another. In general case an algorithm of this transformation is as follows.
Let there be given matrix 

And a maximum number of matrix 




Then the algorithm of building next matrix 
Again, in this case a maximum number of matrix 




In a similar way matrix 

Here, based on the Dirichlet’s theorem on prime numbers in arithmetic progressions, it follows that if the first term and difference of the progression are not coprime numbers, then this progression will not contain any prime number or will contain only one prime number. And this prime number is the first term of the progression.
It also follows from the Dirichlet’s theorem that if the first term and difference of the progression are coprime numbers, then this progression contains prime numbers and composite numbers as well.
Therefore, in our case, first we determine if the first term and difference of the progression that consists of the numbers located in considered row of given matrix are coprime numbers. If they are not coprime numbers, then we conclude that all numbers of this row are composite numbers and they are dark painted for illustration purposes.
If the first term and difference of the considered progression are coprime numbers, then as mentioned above, this row contains both prime and composite numbers. Therefore, the numbers of these rows remain unpainted. Note, that dark painted are only those rows that contain only composite numbers.
Now, using matrices
4. Infinite Number of Twin Primes
First, we set a number of definitions:
Definition 1. If in a certain row of a matrix there are only composite numbers, then the row is dark painted for illustration purposes and for convenience we call it as a painted row.
Definition 2. If in a certain row of a matrix there are both prime and composite numbers, the row is not painted and for convenience we call such rows as not painted row.
Definition 3. If the first number of a row is not painted but the rest numbers are painted, then this not painted number is a prime number and the rest numbers are composite.
Definition 4. If a difference between index numbers of two neighbor and not painted rows is equal to 2, then such rows we call a pair of twin rows or twin rows. For the numbers located in different rows but in one column of twin rows pairs, an equation 
Definition 5. If an index number of a certain painted row differs from an index number of the nearest not painted row by greater than 2, then the raw is called as a single row.
From these definitions it follows that twin prime numbers can be only in twin rows.
A goal of the paper is determine a total number of prime numbers. Therefore, hereafter we will put main emphasis on pairs of twin rows.
Theorem 1. A number of twin rows pairs in matrix 
As is known, all twin prime numbers can be located in paired twin rows only. Moreover, if at some point, for example when considering matrix
We will analyze whether such case is possible and prove Theorem 1 conjointly.
Proof of Theorem 1.
Let suppose that some matrix has only one single pair of twin rows (for example, as in the case of Figure 1, 
For example, in matrix 


Here signs “−” and “+” correspond to upper and lower row of the pair of twin rows respectively.
But this only one pair of rows generates 5 

A set of numbers located in each row of 5 new pairs of rows of matrix 


where
From expression (3) we obtain that if at some value of 

then all numbers of this row are divided by 



All the numbers of each row of 3 newly formed pairs of twin rows, as stated above, form an arithmetic progression and in each of them the first term and difference of the arithmetic progression are coprimes, i.e.:
In virtue of this, it follows from Dirichlet theorem on prime numbers in arithmetic progressions, that in each row of these three pairs of twin rows there is an infinite number of prime numbers.
Now we consider a transformation of matrix 





where
It can be seen from (5), that a set of numbers lying in each row of newly created 21 pairs of rows, separately forms an arithmetic progression with the difference of 

We now consider which of these 21 pairs of rows of matrix 



where
Let we consider divisibility of the first terms 

In this case, in the same way as for case (4), we find that within an interval of 





Besides, all first terms and difference of the arithmetic progression formed from the numbers lying in each row of the newly created 15 pairs of twin rows of matrix

where
In view of this, it follows from Dirichlet theorem for prime numbers in arithmetic progression, that in each row of 15 newly formed pairs of twin rows there is an infinite number of prime numbers.
If we consider further similar transformations of matrices into the next following matrices, for example, matrix 






or

where-an index number of matrix 

It follows from (7) that a number of twin rows pairs is monotonically increased while moving to the next matrices, i.e. with increasing of index number k of matrix

The theorem 1 is proved.
As is shown in (7), a number of twin row pairs will be progressively increasing during the process of moving to the next matrices. But a number of ordinary rows of each next matrix is increased as a special factorial
row pairs is progressively decreased along with the matrices since the ratio
is progressively reduced with rising of
Theorem 2. There are prime twin numbers in any pair of twin rows of any matrix
As shown above, all twin prime numbers can be located in twin rows only. But the question arises are there cases where in some pair of twin rows no any pair of two prime numbers is located in one column. Then, due to the asymmetry (i.e. due to the skewness of prime numbers location) there will be no any pair of prime twin numbers in this pair of twin rows. If such skewness happens in all twin rows pairs of this matrix, then this and all next matrices will no longer contain prime twin numbers. Therefore we can definitely say that a number of prime twins should be limited.
We will analyze this case now and prove the Theorem 2.
Proof of the Theorem 2.
Let consider matrix 

A simple analysis shows that pairs of twin prime numbers conform to some simple rules. In particular, the last digit of any prime number (except 2 and 5) can not be an even number and it can not be equal to 5 as well. This means that last digits of the first and second number of any pair of twins should be respectively (1 and 3) and (7 and 9), and (9 and 1) as well. Therefore, a set of twin prime numbers should be divided into 3 subsets on these grounds. In fact, when we form matrix



Consequently, the fact that in each pair of twin rows of matrix 

Now we consider next matrix


Here we suppose that an average distance by columns between cells, where adjacent prime numbers are located in one pair of twin rows of matrix

If this is so, then in each pair of twin rows of matrix 



To verify this, first we enter a new parameter 

where 
Figure 2. Location of prime numbers in a pair of twins rows.
On the other hand, if two adjacent prime numbers are located in two neighboring and adjoining cells lying in the same column (i.e. vertically as shown in Figure 2, в), then a distance between these cells is equal to zero:
In this case these two prime numbers are twins.
As an example, we now consider a fragment of one pair of twin rows of any matrix. Let suppose that this fragment contains n prime numbers, as shown in Figure 2(c). In this figure light circles denote cells, in which composite numbers are located and dark circles mark cells with prime numbers. Then, above mentioned average distance by columns 

In this case, as shown above, a distance by columns between cells, where adjacent prime numbers with index numbers (1 and 3) and (4 and 6) are located, is equal 1, i.e.:
On the other hand a distance by columns between cells of adjacent prime numbers with index numbers (1 and 2), (5 and 6) and (7 and 8) is equal to zero, that is
Note, that the numbers with index numbers (1 and 2), (5 and 6), and (7 and 8) are twins (Figure 2(c)).
It should be noted that the first prime number can be located in a cell that is lying not in the first column of the considered fragment (Figure 2(c)). Similarly, we can say that a cell in which the last prime number with index number n is located, may be also situated not in the last column. These cases are not taken into account in (9), parameters 




It should be noted that a value of the considered parameter 
If we also add the parameter 
Here, a number of summands in the numerator of expression (9) will be greater by 1, i.e. a number of prime numbers in question is supposedly increased by 1 and becomes equal to

Now we consider a real case of matrix 







Let 




Then when applying (10) for the case of matrix 

where 

Note that here and further the first index of the parameter in question (in this case index k − 1) will correspond to index number of the considered matrix, and second index of this parameter (in this case index l) is an index number of the analyzed pair of twin rows.
Above we made an assumption that prime numbers in pairs of twin rows of each new matrix must be spaced more closely than in pairs of twin rows of previous matrix. To analyze and evaluate the assumption we will analyze a value of 
From the papers of Siegel [6] [7] [8] and other researchers [9] [10] [11] [12] it follows that if the constants (common differences) of different arithmetic progressions are equal to each other and the first term and common difference of each arithmetic progressions are co-primes, then prime numbers are distributed similarly and identically in these progressions. On the other hand, as shown above, a sequence of numbers located in any row of any pair of twin rows of matrix 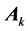


where 

Then from this and expression (12) we obtain:

or

where

On the other side, it follows from (13) that

Here, we call attention to the following:
1) A certain selected row of any pair of twin rows in any matrix, excepting matrices 



2) If two prime numbers, as shown above, are located in one column within one pair of twin rows, then they are twins. In this case a distance by columns between cells, where these prime twin numbers are located should be equal to zero.
3) Considered distance 
As shown in a case of proving Theorem 1, it follows from (7), that while transforming matrix 









In brief, a set of 





Therefore, a number of prime numbers, located in one selected pair of twin rows of the considered fragment of new matrix

On other hand, while transforming a fragment of 



Then from (14), (15) and (16) we obtain


Here we note the following. In the numerator (10) parameter 



If using this expression, enter appropriate simple changes to (12) and (14), then the expression (17) eventually goes into the following form:
From the inequality (11) get that
Therefore, with this in mind, we obtain that the expression for the parameter 



From this it follows that with increase of index number k of matrix 


As can be seen, “a density”, i.e. closeness of prime numbers is increasing. Therefore due to infinity of prime numbers and identity of their distribution in any pair of twin rows of matrix
In particular, due to the fact that general skewness of prime numbers in pairs of twin rows of matrix 


The Theorem 2 is proved.
Now we consider a problem posed in front of this paper.
Theorem 3. A number of twin primes is infinite.
Proof of the Theorem 3.
As is shown above, each matrix 


It follows from the Theorem 1 and expression (7) that with rising growth of matrix
It also follows from the Theorem 2, that in any pair of twin rows there are prime twin numbers. This entire means that a number of prime twins is infinite.
This conclusion is also unavoidably followed from the expression (17).
If in (17) we express the parameter 


then
Next, in the same manner, we transform parameter 






where 



It follows from (18), that with rising of index number 





Here, the infinite series containing reciprocals of prime numbers diverges, as was shown by Euler [13] , i.e.:
Therefore,

This means that with a growth of matrix


The Theorem 3 is proved.
5. Conclusion
Study authors introduce the concept of matrix primes for researching of the properties of prime numbers. After, a number of theorems were proved in the work. Using these theorems and the theorems of Dirichlet, Siegel and Euler the proof of the infinity of twin primes was offered.
Cite this paper
Baibekov, S.N. and Durmagambetov, A.A. (2016) Infinite Num- ber of Twin Primes. Advances in Pure Mathematics, 6, 954-971. http://dx.doi.org/10.4236/apm.2016.613073
References
- 1. A Prime Number. Wikipedia.
https://ru.wikipedia.org/wiki/Простое_число#cite_note-27 - 2. Baibekov, S.N. (2016) About Infinity of Prime Twins. Poisk, No, 203-213.
- 3. Baibekov, S.N. (2015) Development of New Method for Generating Prime Numbers, Eurasian Gumilev University Bulletin, No. 4, 14-21.
- 4. Baibekov, S.N. and Altynbek, S.A. (2015) Development of New Method for Generating Prime Numbers. Natural Science, 7, 416-423.
- 5. Baibekov, S.N. (2015) Generation and Test of Prime Numbers. Eurasian Gumilev University Bulletin, No. 6, Part 2, 263-365.
- 6. Siegеl, С.L. (1935) Acta Arithmetica, v. 1, 83-86.
- 7. Numbers of Arithmetic Progression.
universal_ru_en.academic.ru/...копия - 8. Karatsuba, A.A. (1975) The Fundamentals of Analytical Number Theory. M., Chapter 9.
- 9. Linnik, Yu.V. (1961) The Dispersion Method in Binary Additive Problems. L.: LGU Publishing.
- 10. Linnik, Yu., Barban, M. and Tshudakov, N. (1964) On Prime Numbers in an Arithmetic Progression with a Prime-Power Difference. Acta Arithmetica, 9, 375-390.
- 11. Gritsenko, S.A. and Dhevtsova, M.V. (2011) On Distribution of primes in Arithmetic Progressions with a Difference of Special Type. Scientific Bulletin, Mathematics and Physics Series,. No (100), n. 22, 17-37.
- 12. German-Yevtushenko, M.S. (2013) About a Sum of Values of Divisor Function by Numbers Lying in Arithmetic Progressions with a Difference of Special Type. Scientific Bulletin, Mathematics and Physics Series,. No 12(155), n. 31, 12-19.
- 13. Zagier, D. (1977) The First 50 Million Prime Numbers.
http://ega-math.narod.ru/Liv/Zagier.htm