ABSTRACT

The main purpose of the present work is to introduce necessary conditions for a map on a non-metric space, defined by using a map on a metric space, to have a fixed point.

1. Introduction

Let X denote a complete (or compact) metric space and also 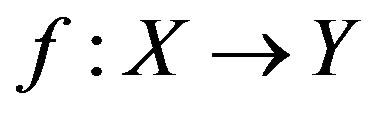a continuous map of X onto Y, where Y is a bounded closed topological normal space with a countable base.

What must be the conditions, in the means of the meric space X, such that the continuous map 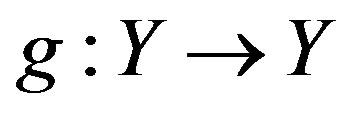from Y onto Y will have a fixed point?

We suppose that (see [1-3]):

the continuous map 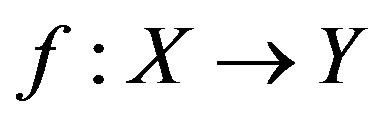(not one to one) and the continuous map 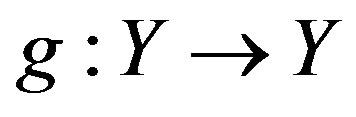are given and the continuous inverse map of f, 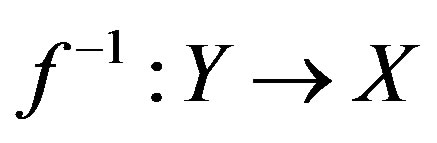exists.

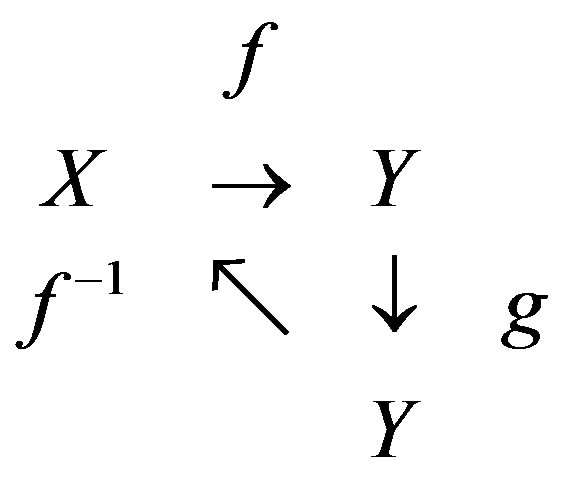
We remind that Banach contraction principle for multivalued maps is valid and also the next Theorem, proved by H. Covitz and S. B. Nadler Jr. (see [4]).

Theorem 1. Let be a complete metric space and 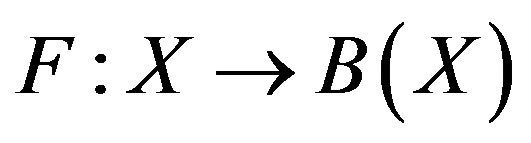a conraction map (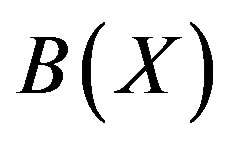denotes the family of all nonempty closed bounded (compact) subsets of X). Then there exists 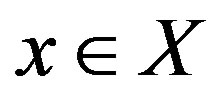such that.

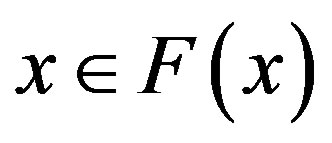2. Main Result

We consider now the next theorem:

Theorem 2. Let 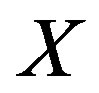denote a complete (or compact) metric space 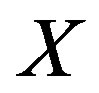and also:

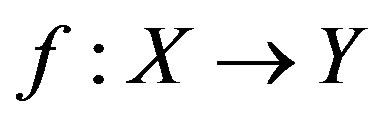a continuous map of 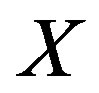onto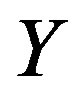, where 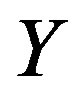is a bounded closed topological normal space with a countable base.

We suppose also that the maps:

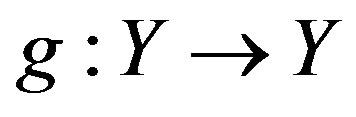is continuous and onto.

and

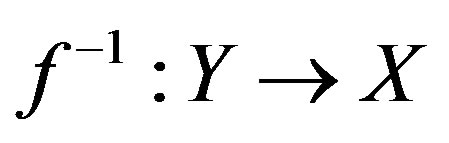exists and it is continuous.

If 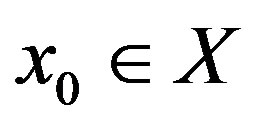is a point from 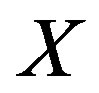and if we suppose also that.

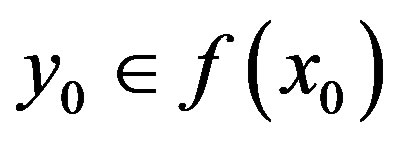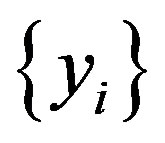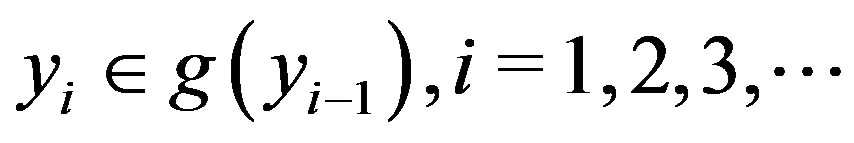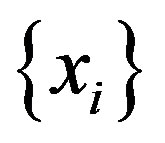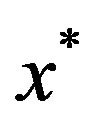Then if the rest terms of the sequence are received from and the rest of the terms of the sequence are determined by and if also is a Cauchy sequence and therefore convergent to a fixed point in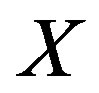, then the sequence 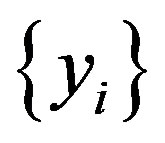will be also convergent to a fixed point 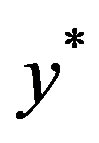in.

Proof. Let 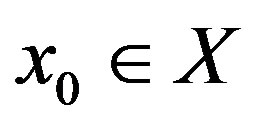is a point from 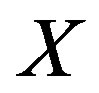and let us suppose also that and let the rest terms of the sequence 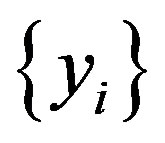are received from .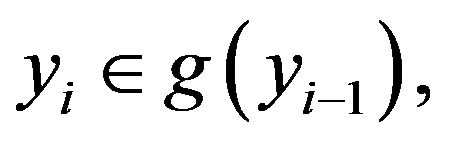

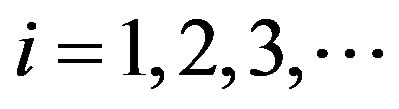Let also the rest of the terms of the sequence 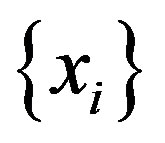are determined by .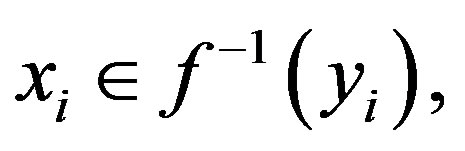

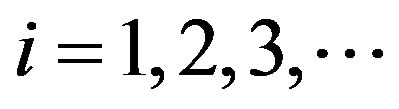If 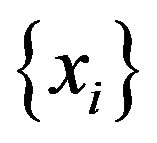is a Cauchy sequence then for any 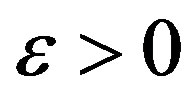there exists an integer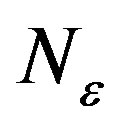, such that for all integers i and k, 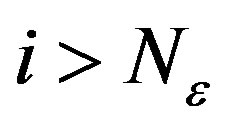and will be satisfied the inequality

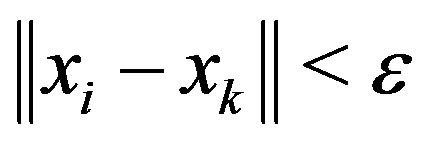
and therefore the Cauchy sequence 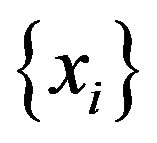will be convergent with a fixed point 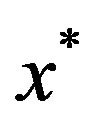in X, and because X is complete (or compact), i.e.

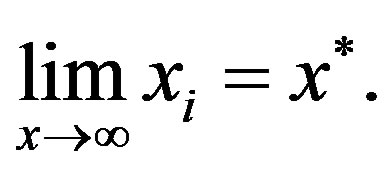
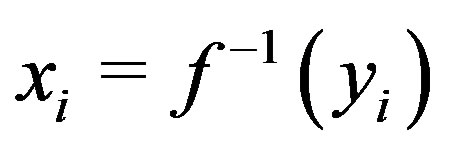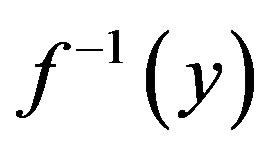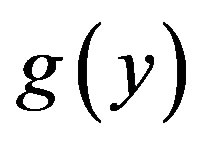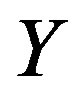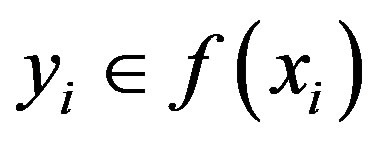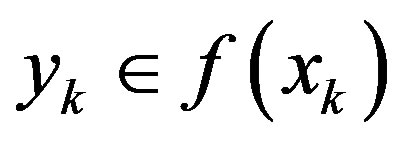Since and and is a continuous map and is continuous map onto the closed and bounded space, and also and, therefore the sequence 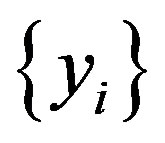will be also convergent with a fixed point 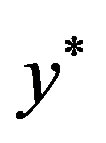in, such that 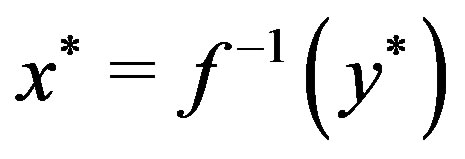and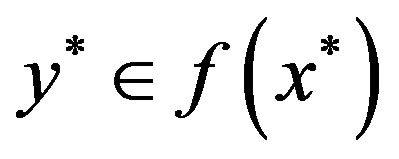, i.e.

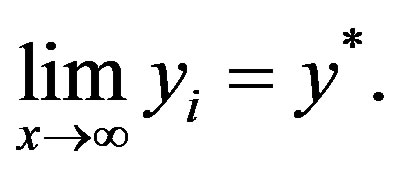
Q.E.D.

3. Acknowledgements

We express our gratitude to Professor Alexander Arhangelskii from OU-Athens for creating the problem and to Professor Jonathan Poritz and Professor Frank Zizza from CSU-Pueblo for the precious help for solving this problem, and to Professor Darren Funk-Neubauer and Professor Bruce Lundberg for correcting some grammatical and spelling errors.

REFERENCES