Journal of Signal and Information Processing
Vol.5 No.1(2014), Article ID:42874,3 pages DOI:10.4236/jsip.2014.51003
The Regularized Low Pass Filter
Math Department, University of Georgia, Athens, GA 30602, USA
Email: wchen@math.uga.edu
Copyright © 2014 Weidong Chen. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. In accordance of the Creative Commons Attribution License all Copyrights © 2014 are reserved for SCIRP and the owner of the intellectual property Weidong Chen. All Copyright © 2014 are guarded by law and by SCIRP as a guardian.
Received July 14th, 2013; revised August 17th, 2013; accepted August 21st, 2013
KEYWORDS
Noise; Low Pass Filter; Regularization
ABSTRACT
In this paper the low pass filter is discussed in the noisy case. And a regularized low pass filter is presented. The convergence property of the regularized low pass filtering algorithm is proved in theory and tested by numerical results.
1. Introduction
Filtering is widely applied in engineering [1-5]. In this paper, the problem of the low pass filtering is analyzed in theory and by examples in detail. A regularized low pass filtering algorithm is presented with the proof of the convergence property and numerical results.
First, we describe the band-limited signals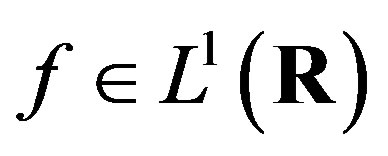 . The details can be seen in [6].
. The details can be seen in [6].
Definition: A function  is said to be
is said to be  band-limited if
band-limited if

Here 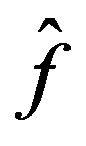 is the Fourier transform of
is the Fourier transform of :
:
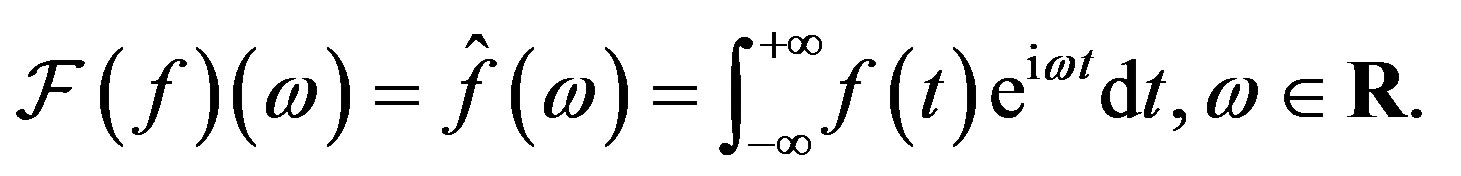 (1)
(1)
We then have the inversion formula:
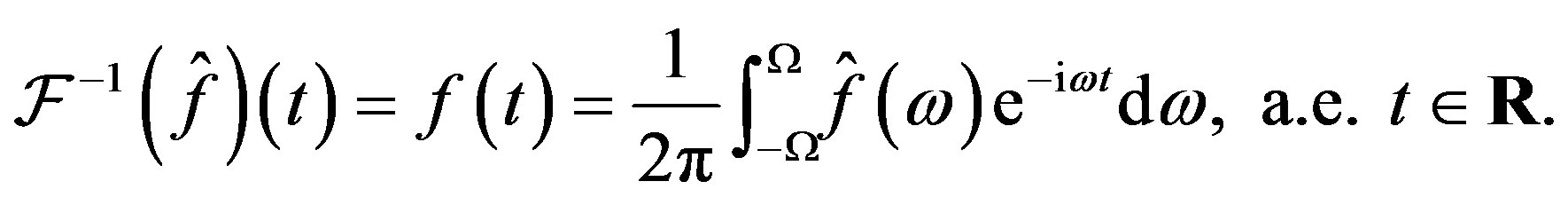
In many practical problems, the signal 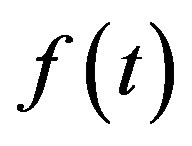 is noisy:
is noisy:
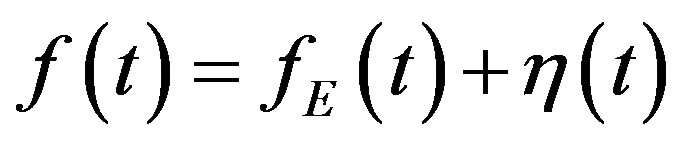 (2)
(2)
where 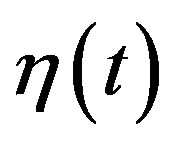 is the noise
is the noise
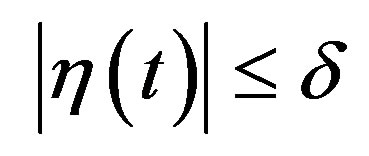 (3)
(3)
and 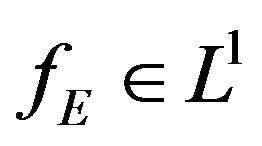 is the exact band-limited signal.
is the exact band-limited signal.
In this paper, we will consider the problem low pass filtering:
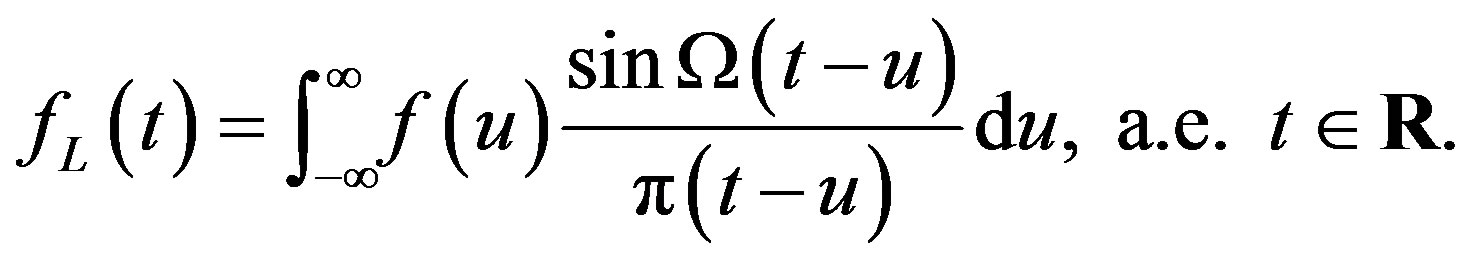 (4)
(4)
If the signal is noisy however, the filter is not reliable. We will give an example to show that the noise can become very large after the low pass filtering process. So this filter is not reliable in the noisy case. And a regularized low pass filtering algorithm will be presented.
In section 2 we give the property of the low pass filter. A regularized filtering algorithm and the proof of its convergence are in section 3. The numerical results of some examples are given in section 4. Finally, the conclusion is given in section 5.
2. The Property of the Low Pass Filter
In this section, we discuss the property of the low pass filter.
Example. Assume the noise is 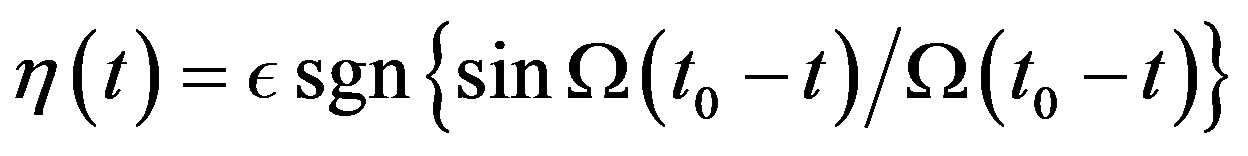 where
where 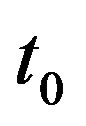 is a given point in the time domain and
is a given point in the time domain and 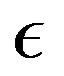 is close to zero. Then the noise signal after the filtering is
is close to zero. Then the noise signal after the filtering is
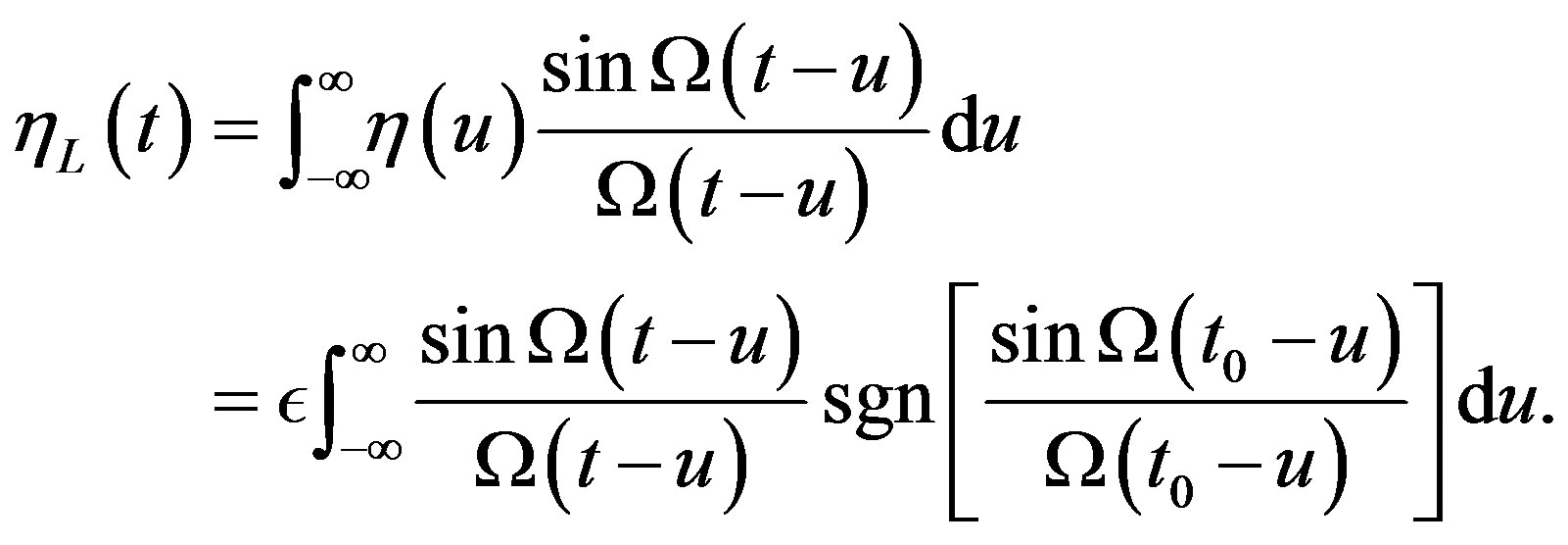
We can see that . However, the noise at
. However, the noise at  after the filtering is
after the filtering is
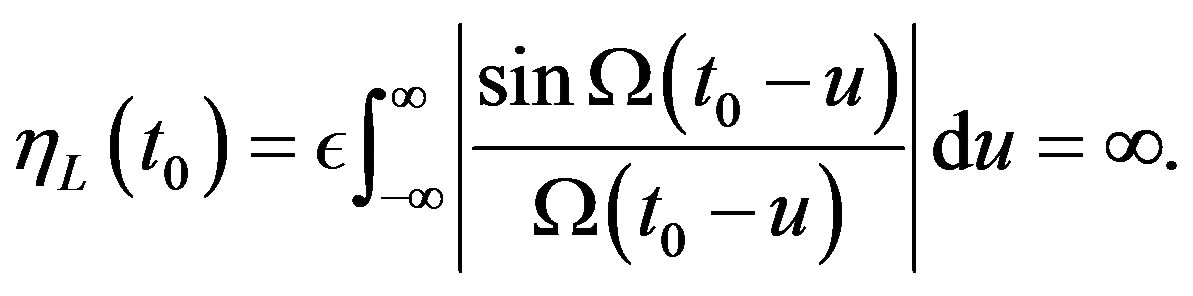
Also at any point ,
, 

So the error after the filtering becomes .
.
Remark. This is only an example for analysis. In the section of numerical results we will show that the low pass filter (4) is not very effective for white noise.
3. The Regularized Filtering Algorithm
First, we consider the regularized Fourier transform [7]:
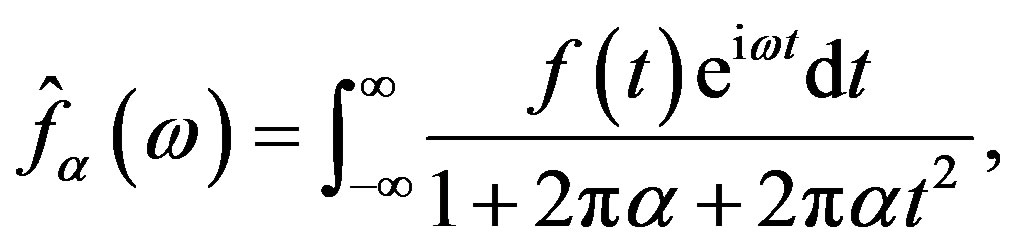
where  is the regularization parameter. Here
is the regularization parameter. Here 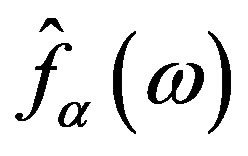 is the minimizer of a smoothing functional. We have proved
is the minimizer of a smoothing functional. We have proved 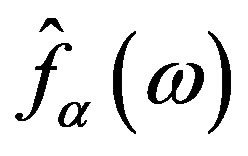 converges to the exact Fourier transform as the error of
converges to the exact Fourier transform as the error of  approaches to zero. In [7], we have successfully used the regularized Fourier transform in extrapolation. So the weight function
approaches to zero. In [7], we have successfully used the regularized Fourier transform in extrapolation. So the weight function
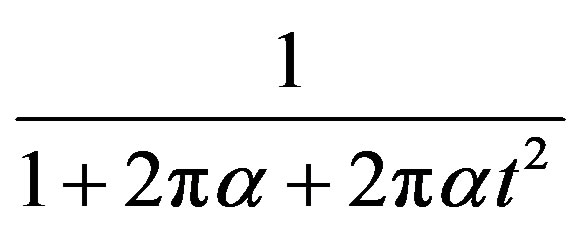
is helpful to solve ill-posed problems.
Based on the regularized Fourier transform we present the regularized filtering formula:
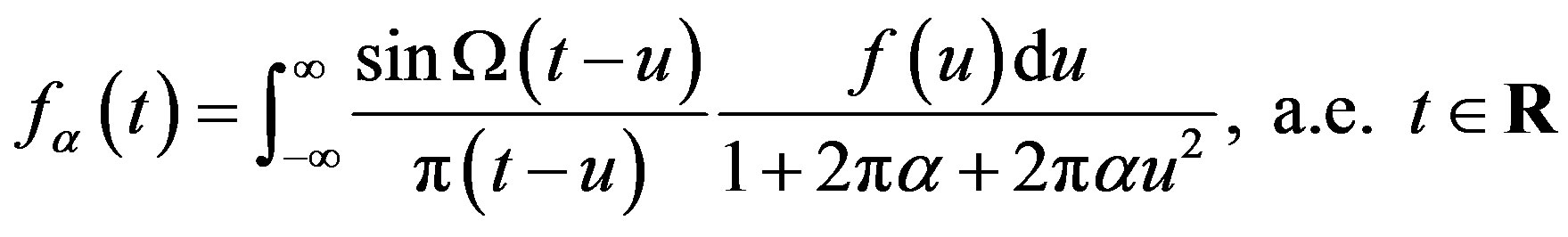 (5)
(5)
where 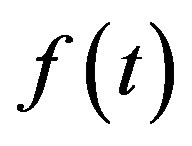 is given in (2).
is given in (2).
The convergence property of this regularized filtering formula is given in the theorem below.
Theorem 3.1. For , if
, if  and
and 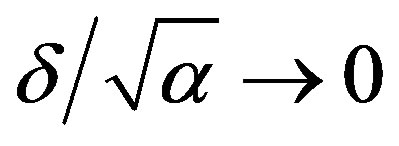 as
as , then
, then  according to the maximum norm as
according to the maximum norm as .
.
 Proof.
Proof.
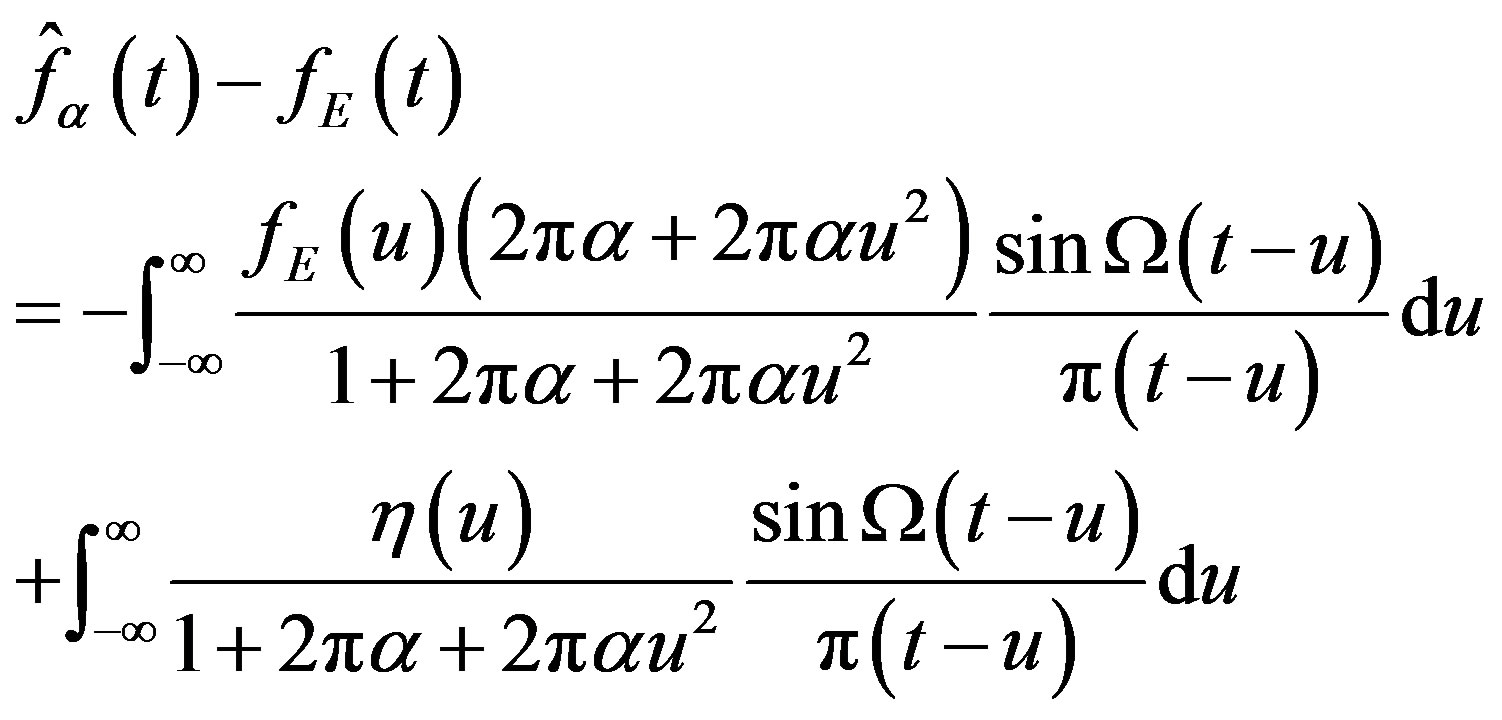
where

For each , there exists
, there exists 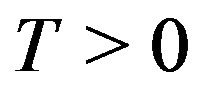 such that
such that

Then
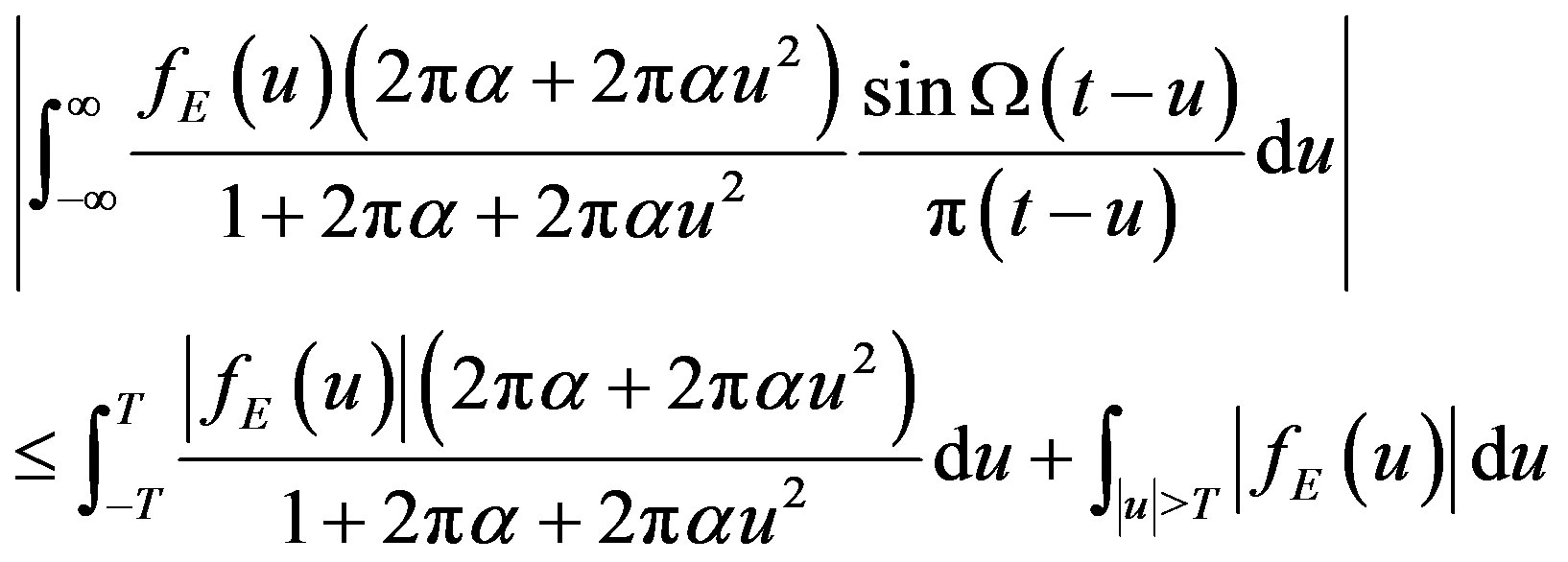
where
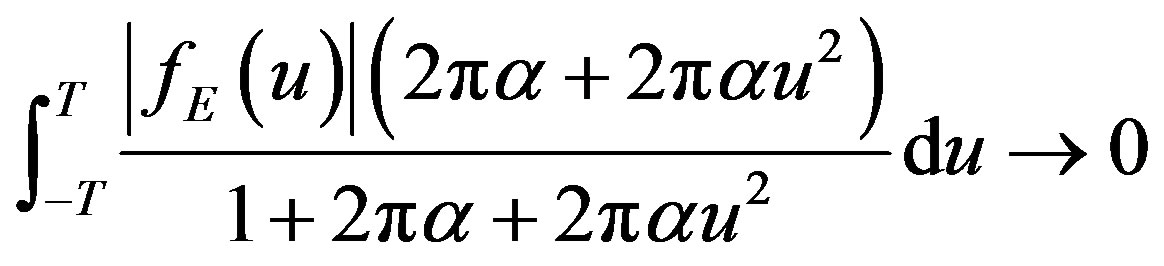
as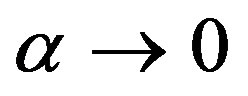 .
.
4. Numerical Results
In this section, we give some examples to show that the regularized filtering algorithm (5) is more effective in reducing the noise than the convolution (4).
Suppose the exact signal in example 1 and 2 is
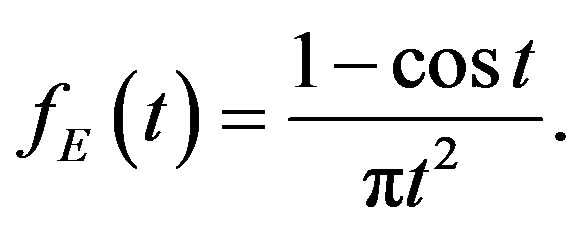
Then construct
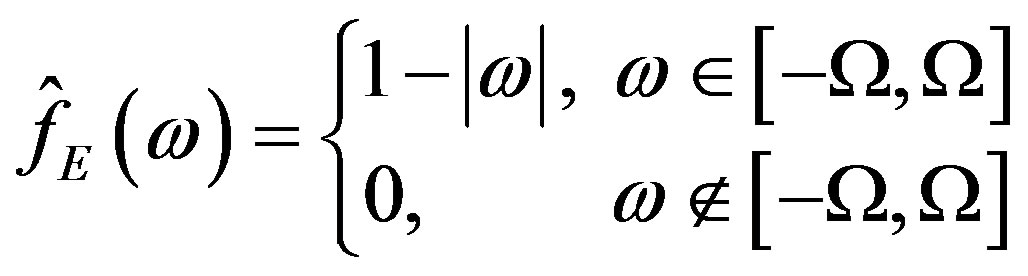
where .
.
Example 1. We consider the noise

where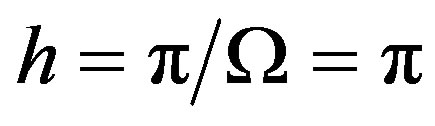 ,
, 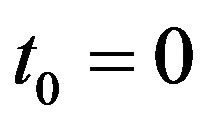 , and
, and . This noise is used in the analysis of the stability in Section 2.
. This noise is used in the analysis of the stability in Section 2.
The result of (4) and the result of the regularized filtering algorithm with  are in figure 1.
are in figure 1.
Example 2. We consider the noise to be white noise that is Gauss distribution whose variance is 0.01. The result of (4) and the result of the regularized sampling algorithm with 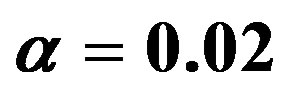 are in figure 2.
are in figure 2.
5. Conclusion
The filter of convolution with sinc function is not stable. For some noises the results of the filtering are not reliable.
Regularized filtering algorithm is more effective in reducing the noise.
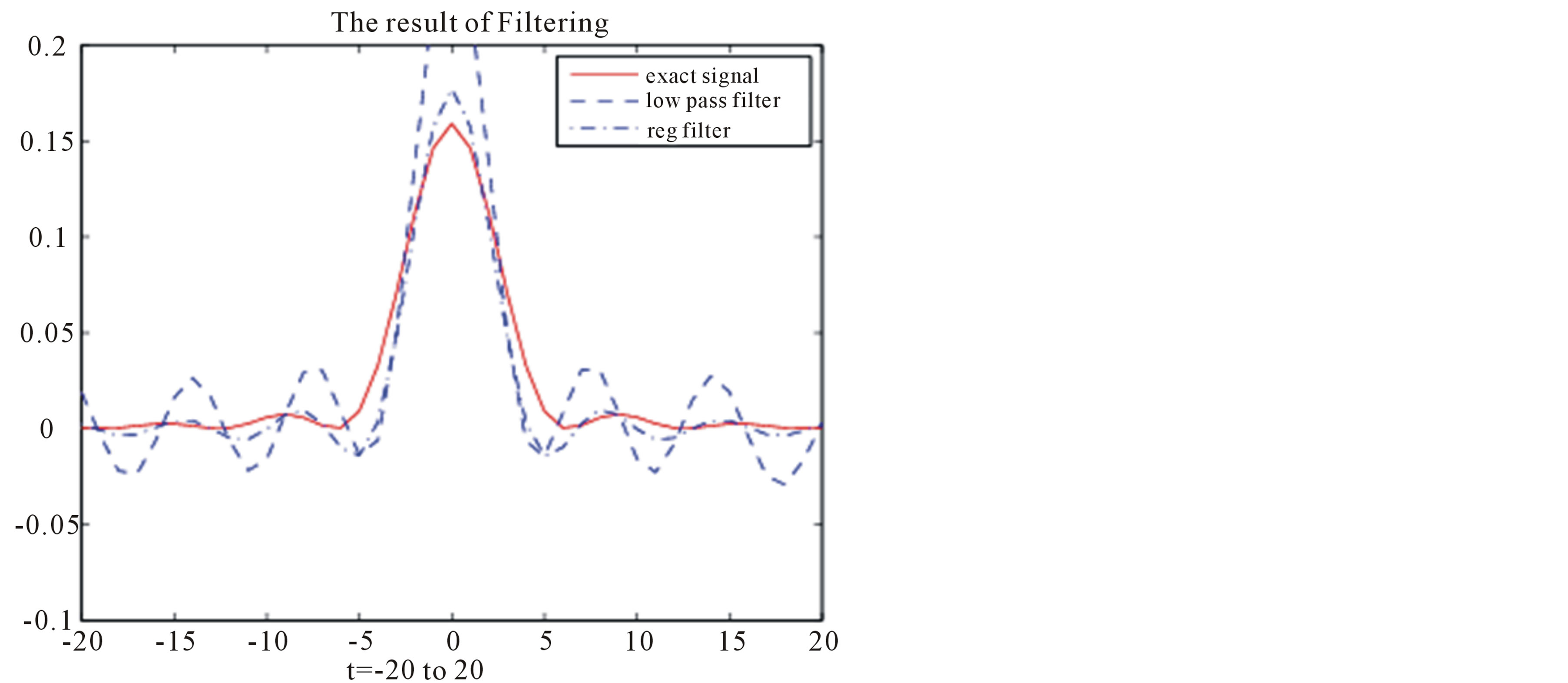
Figure 1. The numerical results of example 1.
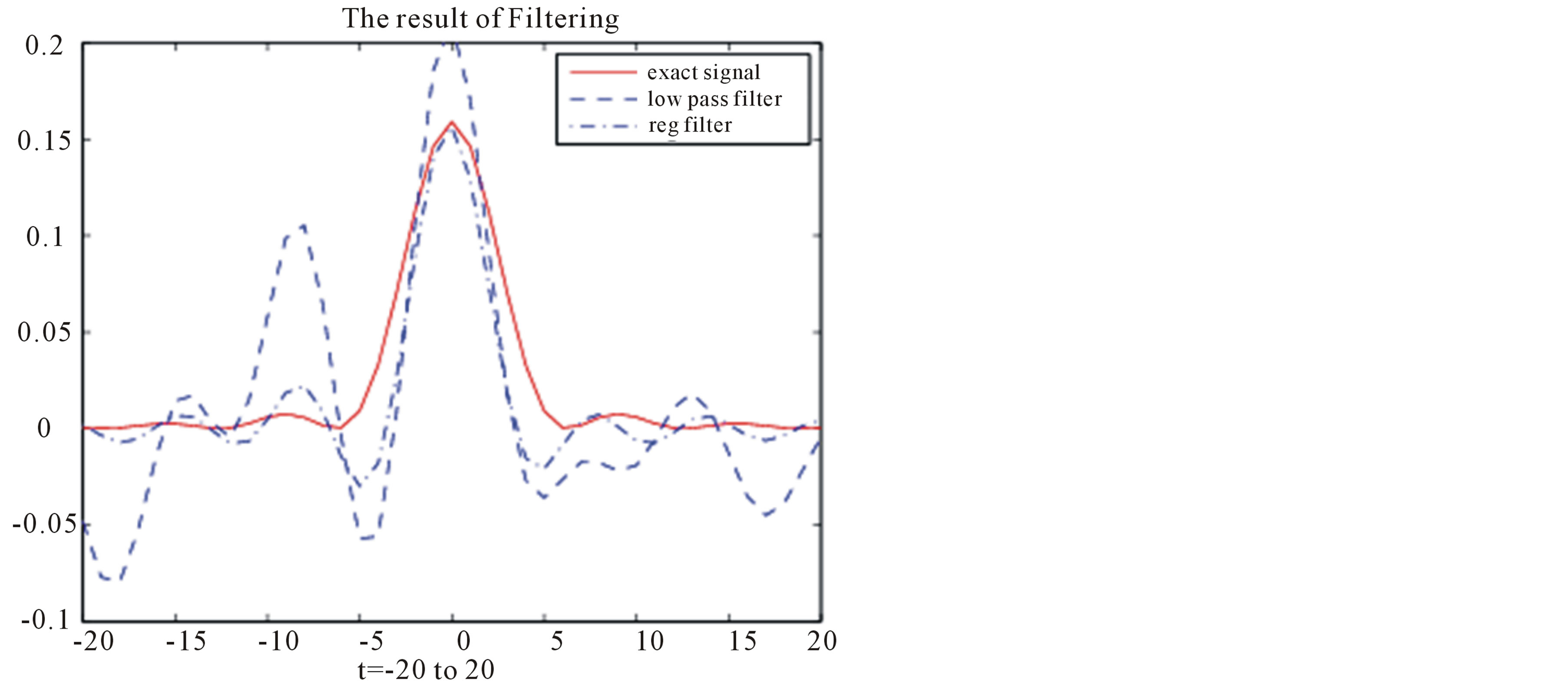
Figure 2. The numerical results of example 2.
Acknowledgements
I would like thank University of Georgia for supporting my post doctoral work.
REFERENCES
- D. Georgakopoulos and W. Q. Yang, “Circuit Noise Reduction by Analogue Lowpass Filtering and Data Averaging,” Electronics Letters, Vol. 37 No. 19, 2001, pp. 1147-1148. http://dx.doi.org/10.1049/el:20010782
- P. Daripa and W. Hua, “A Numerical Study of an Ill-Posed Boussinesq Equation Arising in Water Waves and Nonlinear Lattices: Filtering and Regularization Techniques,” Applied Mathematics and Computation, Vol. 101, No. 2-3, 1999, pp. 159-207. http://dx.doi.org/10.1016/S0096-3003(98)10070-X
- O. Scherzer and J. Weickert, “Relations between Regularization and Diffusion Filtering,” Journal of Mathematical Imaging and Vision, Vol. 12, No. 1, 2000, pp. 43-63. http://dx.doi.org/10.1023/A:1008344608808
- T. Daboczi and I. Kollarl, “Multiparameter Optimization of Inverse Filtering Algorithms,” IEEE Transactions On Instrumentation and Measurement, Vol. 45, No. 2, 1996, pp. 417-421. http://dx.doi.org/10.1109/19.492758
- D. Tschumperle and R. Deriche, “Vector-Valued Image Regularization with PDEs: A Common Framework for Different Applications,” IEEE Transactions on Pattern Analysis and Machine Intelligence, Vol. 27, No. 4, 2005, pp. 506-517. http://dx.doi.org/10.1109/TPAMI.2005.87
- W. Chen, “An Efficient Method for An Ill-Posed Problem —Band-limited Extrapolation by Regularization,” IEEE Transaction on Signal Processing, Vol. 54, No. 12, 2006, pp. 4611-4618. http://dx.doi.org/10.1109/TSP.2006.881255
- W. Chen, “Band-Limited Extrapolation by Regularization,” LAP Lambert Academic Publishing, 2010.

