Applied Mathematics, 2010, 1, 326-333
doi:10.4236/am.2010.14043 Published Online October 2010 (http://www.SciRP.org/journal/am)
Effect of Non-Homogeneity on Thermally Induced
Vibration of Orthotropic Visco-Elastic Rectangular
Plate of Linearly Varying Thickness
Arun Kumar Gupta, Pooja Singhal
Department of Mathematics, M.S. College, Saharanpur, Uttar Pradesh, India
Received August 3, 2010; revised August 30, 2010; accepted September 3, 2010
Abstract
The analysis presented here is to study the effect of non-homogeneity on thermally induced vibration of
orthotropic visco-elastic rectangular plate of linearly varying thickness. Thermal vibrational behavior of non
-homogeneous rectangular plates of variable thickness having clamped boundary conditions on all the four
edges is studied. For non-homogeneity of the plate material, density is assumed to vary linearly in one direc-
tion. Using the method of separation of variables, the governing differential equation is solved. An approxi-
mate but quite convenient frequency equation is derived by using Rayleigh-Ritz technique with a two-term
deflection function. Time period and deflection at different points for the first two modes of vibration are
calculated for various values of temperature gradients, non-homogeneity constant, taper constant and aspect
ratio. Comparison studies have been carried out with non-homogeneous visco-elastic rectangular plate to
establish the accuracy and versatility.
Keywords: Non-Homogeneous, Orthotropic, Visco-Elastic, Variable Thickness, Rectangular Plate, Vibration,
Thermal Gradient
1. Introduction
Thermal effect on vibration of non-homogenous viscoe-
lastic plates are of great interest in the field of engineer-
ing such as for better designing of gas turbines, jet en-
gine, space craft and nuclear power projects, where met-
als and their alloys exhibits visco-elastic behavior. There-
fore, for these reason such structures are exposed to high
intensity heat fluxes and thus material properties undergo
significant changes, in particular the thermal effect on
the modules of elasticity of the material can not be taken
as negligible.
Plates of variable thickness have been extensively used
in Civil, Electronic, Mechanical, Aerospace and Marine
Engineering applications. The practical importance of
such plates has made vibration analysis essential espe-
cially since the vibratory response needs to be accurately
determined in design process in order to avoid resonance
excited by internal or external forces.
Visco-elastic, as its name implies, is a generalization
of elasticity and viscosity. The ideal linear elastic ele-
ment is the spring. When a tensile force is applied to it,
the increase in the distance between its two ends is pro-
portional to the force. The ideal linear viscous element is
the dashpot.
The plate type structural components in aircraft and
rockets have to operate under elevated temperatures that
cause non-homogeneity in the plate material i.e. elastic
constants of the materials becomes functions of space
variables. In an up-to-date survey of literature, authors
have come across various models to account for non-ho-
mogeneity of plate materials proposed by researchers
dealing with vibration but none of them consider non-ho-
mogeneity with thermal effect on orthotropic visco-elastic
plates.
Free vibration of visco-elastic orthotropic rectangular
plates was discussed by Sobotka [1].Gupta and Khanna
[2] discussed vibration of viscoelastic rectangular plate
with linearly thickness variations in both directions.
Leissa’s monograph [3] contains an excellent discussion
of the subject of vibrating plates with elastic edge sup-
port. Several authors [4,5] have studied the thermal ef-
fect on vibration of homogeneous plates of variable
thickness but no one considered thermal effect on vibra-

A. K. GUPTA ET AL. 327
tion on non-homogeneous rectangular plates of varying
thickness. Tomar and Gupta [6-8] solved the vibration
problem of orthotropic rectangular plate of varying
thickness subjected to a thermal gradient. Gupta, Lal and
Sharma [9] discussed the vibration of non-homogeneous
circular plate of nonlinear thickness variation by a quad-
rature method. Gupta, Johri and Vats [10] solved the
problem of thermal effect on vibration of non-homoge-
neous orthotropic rectangular plate having bi-directional
parabolically varying thickness. Gupta, Kumar and Gupta
[11] studied the vibration of visco-elastic orthotropic
parallelogram plate with a linear variation of thickness.
Recently, Gupta and Kumar [12] solved the vibration pro-
blem of non-homogeneous visco-elastic rectangular plate
of linearly varying thickness subjected to linearly ther-
mal effect. Free vibration of a clamped visco-elastic rec-
tangular plate having bi-direction exponentially varying
thickness were studied by Gupta, Khanna and Gupta
[13] .Gupta, Aggarwal, Gupta, Kumar and Sharma [14]
discussed the non-homogeneity on free vibration of
orthotropic visco-elastic rectangular plate of parabolic
varying thickness. Subsequent review article of Bhasker
and Kaushik [15] are best source for problems involving
rectangular plates fall into three distinct categories: 1)
plates with all edges simply supported; 2) plates with a
pair of opposite edges simply supported; 3) plates which
do not fall into any of the above categories.
Rectangular plates have wide applications in civil
structures, electrical engineering, marine industry and
mechanical engineering. The dynamic characteristics of
rectangular plates are important to engineering designs.
An analysis is presented in this paper is to study the ef-
fect of non-homogeneity on thermally induced vibration
of orthotropic visco-elastic rectangular plate of linearly
varying thickness. It is clamped supported on all the four
edges. The assumption of small deflection and linear
orthotropic visco-elastic properties are made. It is further
assumed that the visco-elastic properties of the plates are
of the Kelvin type. For this the material constants of al-
loy ‘Duralium’ is used for the calculation of numerical
values. Time period and deflection for the first two
modes of vibration are calculated for the various values
of thermal gradients, non-homogeneity constant, aspect
ratio and taper constant.
2. Analysis
The equation of motion of a visco-elastic orthotropic
rectangular plate of variable thickness may be written in
the form, as by Sobotka [1]
22
22
222
2=
xy y
xMM
Mw
h
xy
x
yt
r
¶¶
¶¶
++
¶¶
¶¶¶
(1)
Here
x
M
,
y
M
and
x
y
M
are moments per unit length
of plate, r is mass per unit volume, h is thickness of
plate and w is displacement at time t.
The expression for ,,
x
yxy
M
MM are given by
22
~'
1
22
22
~'
122
2
~
=
=
=2
xx
yy
xy xy
ww
MDD D
x
y
ww
MDDD
x
y
w
MDD
xy
é
ù
¶¶
ê
ú
-+
ê
ú
¶¶
ë
û
é
ù
¶¶
ê
ú
-+
ê
ú
¶¶
ë
û
¶
-¶¶
(2)
where
3
=12(1 )
x
x
x
y
Eh
Dvv-
is called the flexural rigidity of the plate in x-direction,
3
=12(1 )
y
y
x
y
Eh
Dvv
-
is called the flexural rigidity of the plate in y-direction,
3
=12
xy
xy
Gh
D
is called the torsion rigidity,
and
'
1=(=)
x
yyx
DvD vD
Here D
is Rheological operator and &
x
y
EE are the
modules of elasticity in x- and y-direction respectively,
x
ν and
y
ν are the Poisson ratios &
x
y
G is the shear
modulus.
Taking deflection w as a product of two functions as:
()()()
=,,= ,wwxyt WxyTt
(3)
where W(x, y) is the function of coordinates in x, y and T
(t) is a time function.
Using Equation (3) in Equations (1) & (2) and then
equating both sides of equation comes to a constant, say
p2, one gets two separate differential equations as fol-
lows:
44 43
44 222
333
233
2
22' 222
1
2222 22
2
2' 22
2
1
22
22
222
4=0
xy
y
x
y
x
xy
WW WHW
DD Hx
x
yxy xy
D
D HWW W
yxy xy xy
D
DD
WWW
xxyy xy
D
DWW
hp W
xy xy yx r
é
¶¶ ¶¶¶
ê++ +
ê¶
¶¶ ¶¶¶¶
ë
¶
¶
¶¶¶ ¶
+++
¶¶¶
¶¶ ¶¶
¶
¶¶
¶¶¶
++ +
¶¶¶¶¶¶
ù
¶
¶¶¶
ú
++ -
ú
¶¶ ¶¶
¶¶ ú
û
(4)

A. K. GUPTA ET AL. 328
and
.. 2=0TpDT+ (5)
where
'
1
=2
x
y
H
DD+
Equation (4) is a differential equation of motion for
orthotropic rectangular plate of variable thickness and (5)
is a differential equation of time functions of free vibra-
tion of viscoelastic rectangular orthotropic plate.
The temperature dependence of the modulus of elas-
ticity for orthotropic materials is given by
()
()
()
1
2
0
=1
=1
=1
x
y
xy
EE γτ
EE γτ
GG γτ
-
-
-
(6)
and temperature distribution along the length i.e. in the
x-direction,
()
=1 /
o
ττ
x
a- (7)
where τ denotes the temperature excess above the ref-
erence temperature at any point at distance /
x
a and
0
τ denotes the temperature excess above reference
temperature at the end, i.e. for x = 0. Here E1 and E2 are
values of the Young’s moduli respectively along the x
and y axis at the reference temperature i.e. at τ = 0
The modulus variation (6) in view of expressions (7)
becomes
()( )
()( )
()( )
1
2
0
=1 1/
=1 1/
=11/
x
y
xy
Ex Exa
Ex Exa
Gx Gxa
a
a
a
éù
--
ëû
éù
--
ëû
éù
--
ëû
(8)
where α = γ τo (0 ≤ α < 1), known as thermal gradient.
The expression for the strain energy V and Kinetic en-
ergy P in the plate are [3]
22
,,1,,
00
2
,
1() ()2
2
4( )
ab
x
xxyyyxx yy
xy xy
DW DWDWW
D Wdxdy




 (9)
22
00
1
=2
ab
PphWdxdyr
òò (10)
The thickness and density varies linearly in the
x-direction only, so let us assume
()
=1 /
o
hh xab+ (11)
and ()
1
=1 /
o
x
arr a+ (12)
where β is the taper constant and α1 is non-homogeneity
constant.
3. Solution and Frequency Equation
To find the solution, we use Rayleigh-Ritz technique. In
this method, one requires maximum strain energy be
equal to the maximum Kinetic energy. So, it is necessary
for the problem under consideration that
()
=0δVP- (13)
for arbitrary variations of W satisfying relevant geomet-
rical boundary conditions are
,
,
==0 at =0,
==0 at =0,
x
y
WWx a
WWyb
(14)
and the corresponding two term deflection function is
taken as [6]
 
 
2
12
=/ /1/1/
//1/1/
Wxaybxa yb
A
Axayb xayb










(15)
The non-dimensional variables are
=/, =/, =/, =/,
=/
X
xaYybWW ahha
a 
(16)


**
112 2
***
21
=/1, =/1
==
xy xy
xy
EE νν EE νν
EνEνE

(17)
By using Equations (8), (11) and (12) in (9) and
(10), one gets
2 1/
25
00
1
=[(1 + )(1)])
2
ba
oo
Ppha αXβ
X
WdXdY 

 (18)
and
1/ 3
00
2** 2
,,
21
**
,,
1
*2
,
1
[{1 - (1)}(1)
{() (/)()
(2 /)
(4/)() }]
ba
XX YY
XX YY
XY
o
VRX X
WEEW
EEWW
GEW dXdY
 



(19)
where 3
*
1
1
=( /12)
2o
REh a (20)
On substituting the values of P and V from Equations
(18) and (19) in Equation (13), we get
()
22
11
=0VλpP- (21)

1/ 3
00
2** 2
,,
21
**
,,
1
*2
,
1
{1(1)}(1)
{() (/)()
(2 /)
(4/)() }
ba
XX YY
XX YY
XY
o
VRX X
WEEW
EEW W
GEW dXdY
 





(22)

A. K. GUPTA ET AL. 329

2 1/
11
00
={1 + (1)
ba
PαXβ
X
WdXdY




 (23)
where
4
2
2
*
1
12
=o
o
a
λ
Eh
r (24)
Equation (21) involves the unknown A1 and A2 arising
due to the substitution of W(x,y) from Equation (15).
These two constants are to be determined from Equation
(21) as follows:
22
11
()=0
n
VλpP
A
¶-
¶ (25)
where n = 1,2
On simplifying (25) we get
112 2
=0
nn
bAbA+ (26)
where n = 1,2, bn1, bn2 involves parametric constants and
the frequency parameter p. For a non-trivial solution, the
determinant of the coefficient of Equation (26) must be
zero. So, we get the frequency equation as
11 12
21 22
=0
bb
bb (27)
On solving Equation (27) one gets a quadratic equa-
tion in p2, which gives two values of p2. On substituting
the value of A1 = 1, by choice, in Equation (15) one get
A2 = –b11/b12 and hence W becomes:
()
()
2
11
12
11
111
aa
WXYX Y
bb
baa
XYX Y
bb b
éù
æö
÷
ç
êú
=--
÷
ç÷
ç
êú
èø
ëû
éù
æö
æöæ ö
÷
ç÷÷
êú
çç
÷
+-- -
ç÷÷
çç
÷÷÷
êú ççç
÷ çèøè ø
èø
êú
ëû
(28)
4. Time Functions of Vibration of
Viscoelastic Plates
The expression for Time function of free vibrations of
visco-elastic plates of variable thickness can be derived
from Equation (5) that depends upon visco-elastic op-
erator D
 and which for Kelvin’s Model can be taken
as:
1d DGdt
h
ìü
æöæö
ïï
ïï
÷÷
çç
º+ ÷÷
íý
çç
÷÷
çç
ïï
èøèø
ïï îþ
 (29)
where  is visco-elastic constant and G is shear modulus.
Taking temperature dependence of viscoelastic constant
η and shear modulus G is the same form as that of
Young’s moduli, we have
() ()
() ()
01
02
=1,
=1
GτGγτ
τγτhh
-
- (30)
where G0 is shear modulus and 
0 is visco-elastic con-
stant at some reference temperature i.e. at  = 0, 
1 and

2
are slope variation of  with G and  respectively. Sub-
stituting the value of  from Equation (7) and using
Equation (16) in Equation (30), one gets:
()
05
=1 1GG α
X
é
ù
--
ë
û (31)
where 
5 =

1

0, 0 <

5 < 1
and
 = 
0 [1 –

4 (1 – X)]
where

4 =

2

0, 0 <

4 < 1
Here

4 and

5 are thermal constants.
Substituting Equations (29) and (31) in Equation (5),
one gets:
22
=0TpkTpT
·· ·
++ (32)
Where 04
05
[1(1 )]
[1(1 )]
α
X
kGG α
X
h h--
== -- (33)
Equation (32) is a second order differential equation in
time function T. The solution of which comes out to be
[
]
1
1121
()= cossin
at
TteCbt Cbt+ (34)
where 1 a = –p2k/2,
2
1=1 2
pk
bp æö
÷
ç
-÷
ç÷
ç
èø
(35)
where C1 and C2 are constants of integration, which can
be determined easily from initial conditions of the plate.
Let us take initial conditions as
T = 1 and T
 = 0 at t = 0 (36)
Using Equation (36) in Equation (34), we have C1 = 1
and
C2 = p2k/2b1 = –a1/b1 (37)
Using Equation (37) in Equation (34), one has
()
1
1111
()cos/sin
at
Ttebtabbt
é
ù
=+-
ë
û (38)
Thus deflection w may be expressed by using Equa-
tion (38) and Equation (28) in Equation (3)
()
()
()
1
2
11
12
1111
=11
111
cos/sin
at
aa
wXYX Y
bb
baa
XYX Y
bb b
ebtabbt
éù
æö
÷
ç
êú
--
÷
ç÷
ç
êú
èø
ëû
é
ù
æö
æöæ ö
÷
ç÷÷
ê
ú
çç
÷
+-- -
ç÷÷
çç
÷÷÷
ê
ú ççç
÷
çèøè ø
èø
ê
ú
ë
û
éù
´+-
ë
û
(39)
Time period of the vibration of the plate is given by
=2 /KπP (40)
where p is the frequency given by Equation (27).

A. K. GUPTA ET AL. 330
Figure 1. Variation of time period with taper constant of visco-elastic non homogeneous rectangular plate of line-
arly varying thickness.
Figure 2. Variation of time period with non homogeneity constant of visco-elastic non homogeneous rectangular
plate of linearly varying thickness.
Figure 3. Variation of time period with aspect ratio of visco-elastic non homogeneous rectangular plate of linearly
varying thickness.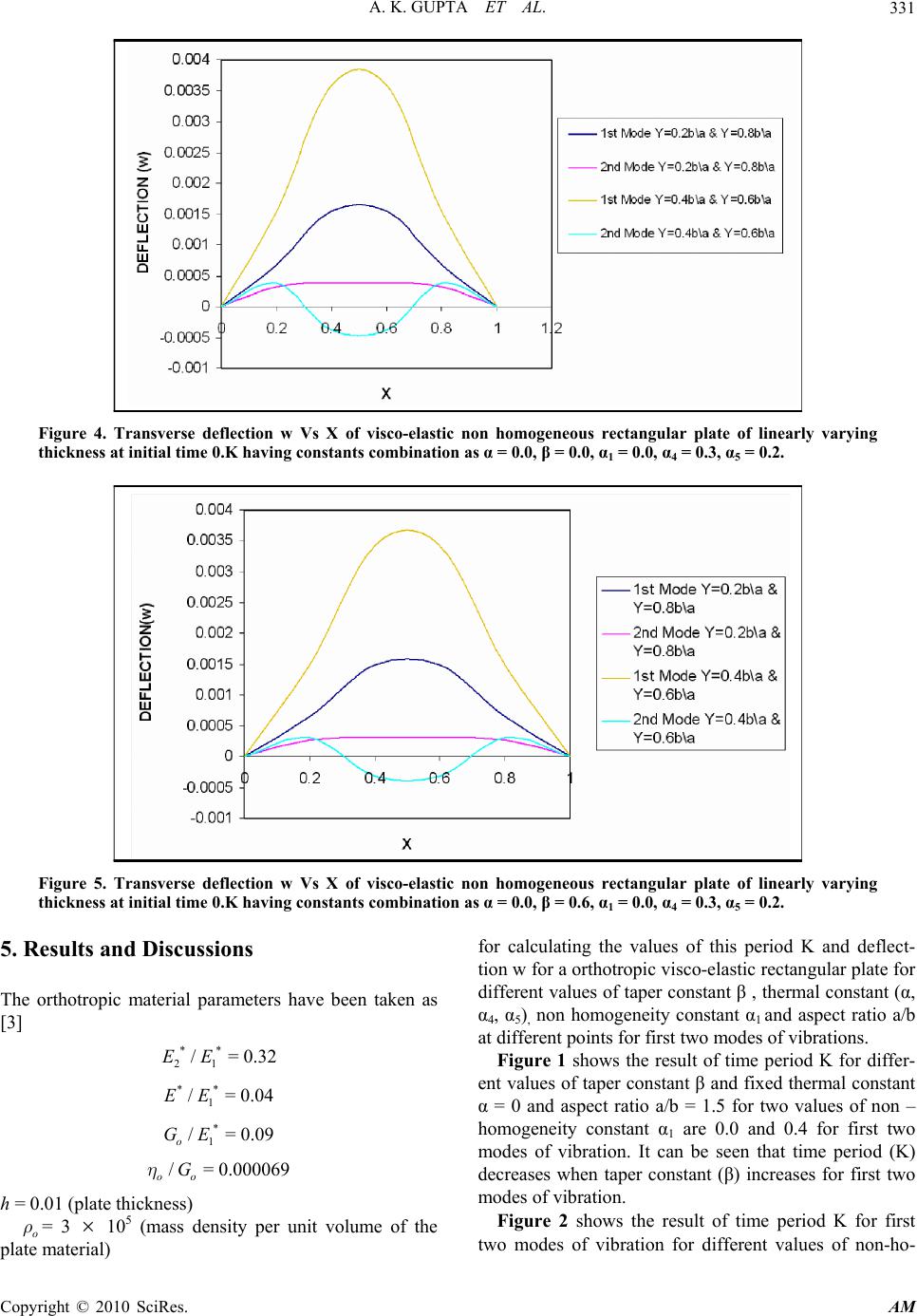

A. K. GUPTA ET AL. 331
Figure 4. Transverse deflection w Vs X of visco-elastic non homogeneous rectangular plate of linearly varying
thickness at initial time 0.K having constants combination as α = 0.0, β = 0.0, α1 = 0.0, α4 = 0.3, α5 = 0.2.
Figure 5. Transverse deflection w Vs X of visco-elastic non homogeneous rectangular plate of linearly varying
thickness at initial time 0.K having constants combination as α = 0.0, β = 0.6, α1 = 0.0, α4 = 0.3, α5 = 0.2.
5. Results and Discussions
The orthotropic material parameters have been taken as
[3]
**
21
/=0.32EE
**
1
/=0.04EE
*
1
/=0.09
o
GE
/= 0.000069
oo
Gh
h = 0.01 (plate thickness)
o
r= 3  105 (mass density per unit volume of the
plate material)
for calculating the values of this period K and deflect-
tion w for a orthotropic visco-elastic rectangular plate for
different values of taper constant β , thermal constant (α,
α4, α5), non homogeneity constant α1 and aspect ratio a/b
at different points for first two modes of vibrations.
Figure 1 shows the result of time period K for differ-
ent values of taper constant β and fixed thermal constant
α = 0 and aspect ratio a/b = 1.5 for two values of non –
homogeneity constant α1 are 0.0 and 0.4 for first two
modes of vibration. It can be seen that time period (K)
decreases when taper constant (β) increases for first two
modes of vibration.
Figure 2 shows the result of time period K for first
two modes of vibration for different values of non-ho-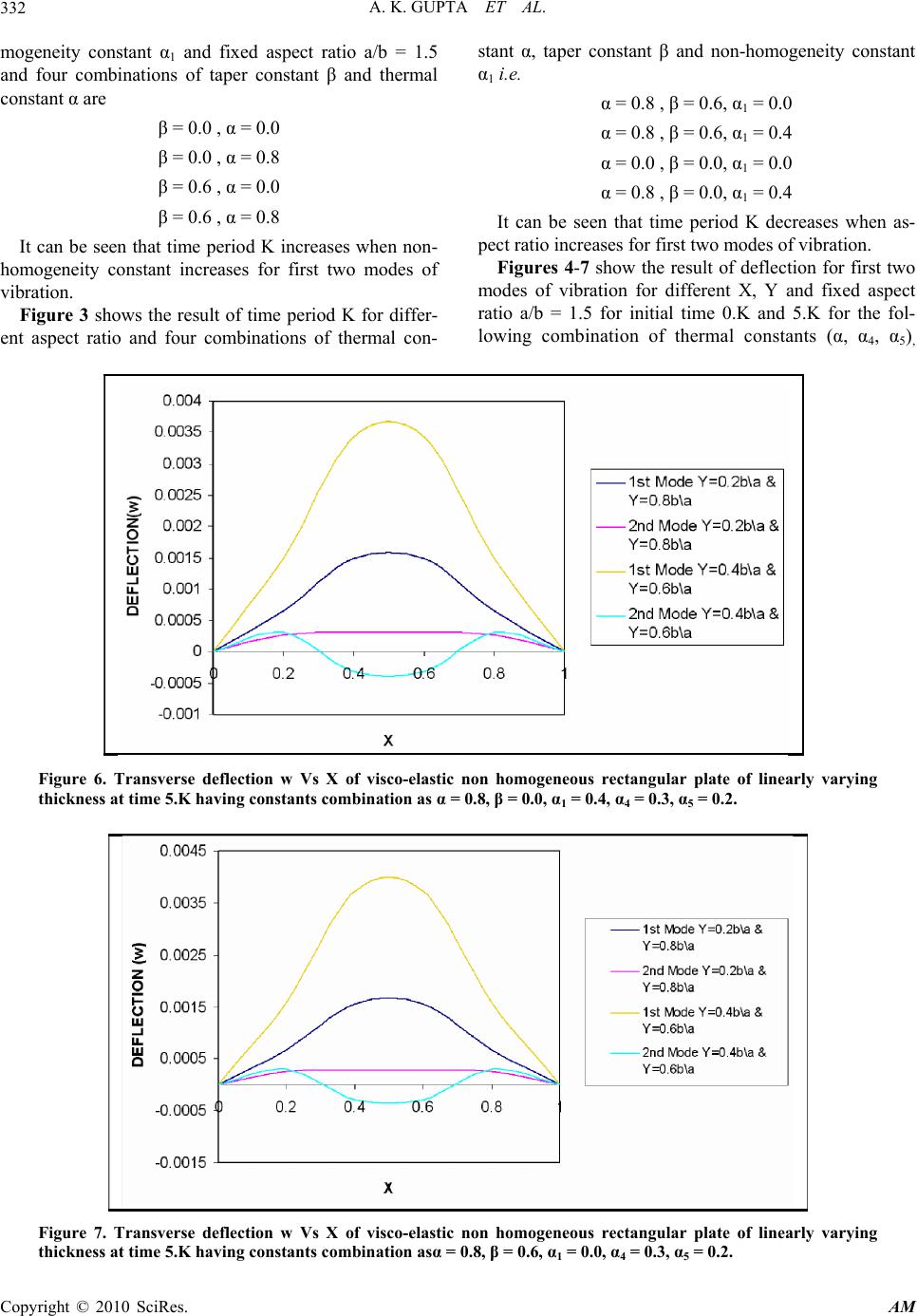

A. K. GUPTA ET AL. 332
mogeneity constant α1 and fixed aspect ratio a/b = 1.5
and four combinations of taper constant β and thermal
constant α are
β = 0.0 , α = 0.0
β = 0.0 , α = 0.8
β = 0.6 , α = 0.0
β = 0.6 , α = 0.8
It can be seen that time period K increases when non-
homogeneity constant increases for first two modes of
vibration.
Figure 3 shows the result of time period K for differ-
ent aspect ratio and four combinations of thermal con-
stant α, taper constant β and non-homogeneity constant
α1 i.e.
α = 0.8 , β = 0.6, α1 = 0.0
α = 0.8 , β = 0.6, α1 = 0.4
α = 0.0 , β = 0.0, α1 = 0.0
α = 0.8 , β = 0.0, α1 = 0.4
It can be seen that time period K decreases when as-
pect ratio increases for first two modes of vibration.
Figures 4-7 show the result of deflection for first two
modes of vibration for different X, Y and fixed aspect
ratio a/b = 1.5 for initial time 0.K and 5.K for the fol-
lowing combination of thermal constants (α, α4, α5),
Figure 6. Transverse deflection w Vs X of visco-elastic non homogeneous rectangular plate of linearly varying
thickness at time 5.K having constants combination as α = 0.8, β = 0.0, α1 = 0.4, α4 = 0.3, α5 = 0.2.
Figure 7. Transverse deflection w Vs X of visco-elastic non homogeneous rectangular plate of linearly varying
thickness at time 5.K having constants combination asα = 0.8, β = 0.6, α1 = 0.0, α4 = 0.3, α5 = 0.2.

A. K. GUPTA ET AL. 333
taper constant β and non-homogeneity constant α1.
Results are compared with isotropic plate [12] and
found to be in very close agreement.
6. References
[1] Z. Sobotka, “Free Vibration of Visco-Elastic Ortho-
tropic Rectangular Plates,” Acta Technica, Vol. 23, No. 6,
1978, pp. 678-705.
[2] A. K. Gupta and A. Khanna, “Vibration of Viscoelastic
Rectangular Plate with Linearly Thickness Variations in
Both Directions,” Journal of Sound and Vibration, Vol.
301, No. 3-5, 2007, pp. 450-457.
[3] A. W. Leissa, “Vibration of Plate,” NASA SP-60, 1969.
[4] S. R. Li and Y. H. Zhou, “Shooting Method for Non Lin-
ear Vibration and Thermal Buckling of Heated Orthotropic
Circular Plates,” Journal of Sound and Vibration, Vol.
248, No. 2, 2001, pp. 379-386.
[5] D. V. Bambill, C. A. Rossil, P. A. A. Laura and R. E.
Rossi, “Transverse Vibrations of an Orthotropic Rectan-
gular Plate of Linearly Varying Thickness and with a
Free Edge,” Journal of Sound and Vibration, Vol. 235,
No. 3, 2000, pp. 530-538.
[6] J. S. Tomar and A. K. Gupta, “Effect of Thermal Gradi-
ent on Frequencies of Orthotropic Rectangular Plate
Whose Thickness Varies in Two Directions,” Journal of
Sound and Vibration, Vol. 98, No. 2, 1985, pp. 257-262.
[7] J. S. Tomar and A. K. Gupta, “Thermal Effect on Fre-
quencies of an Orthotropic Rectangular Plate of Linearly
Varying Thickness,” Journal of Sound and Vibration, Vol.
90, No. 3, 1983, pp. 325-331.
[8] J. S. Tomar and A. K. Gupta, “Effect of Exponential
Temperature Variation on Frequencies of an Orthotropic
Rectangular Plate of Exponentially Varying Thickness,”
Proceeding of the Workshop on Computer Application in
Continum Mechanics, Roorkee, 11-13 March 1986, pp.
183-188.
[9] U. S. Gupta, R. Lal and S. Sharma, “Vibration Analysis
of Non-Homogenous Circular Plate of Nonlinear Thick-
ness Variation by Differential Quadrature Method,” Journal
of Sound and Vibration, Vol. 298, No. 4-5, 2006, pp. 892
-906.
[10] A. K. Gupta, T. Johri and R. P. Vats, “Thermal Effect on
Vibration of Non-Homogeneous Orthotropic Rectangular
Plate Having Bi-Directional Parabolically Varying Thick-
ness,” Proceeding of International Conference in World
Congress on Engineering and Computer Science 2007
(WCECS 2007), San Francisco, 24-26 October 2007, pp.
784-787.
[11] A. K. Gupta, A. Kumar and D. V. Gupta, “Vibration of
Visco-Elastic Orthotropic Parallelogram Plate with Line-
arly Thickness Variation,” Proceeding of International
Conference in World Congress on Engineering and
Computer Science 2007 (WCECS 2007), San Francisco,
24-26 October 2007, pp. 800-803.
[12] A. K. Gupta and L. Kumar, “Thermal Effect on Vibration
of Non-Homogenous Visco-Elastic Rectangular Plate of
Linear Varying Thickness,” Meccanica, Vol. 43, No. 1,
2008, pp. 47-54.
[13] A. K. Gupta, A. Khanna and D. V. Gupta, “Free Vibration of
Clamped Visco-Elastic Rectangular Plate Having Bi-
Directional Exponentially Thickness Variations,” Journal
of Theotrocial and Applied Mechanics, Vol. 47, No. 2,
2009, pp. 457-471.
[14] A. K. Gupta, N. Aggarwal, D. V. Gupta, S. Kumar and P.
Sharma, “Study of Non-Homogeneity on Free Vibration
of Orthotropic Visco-Elastic Rectangular Plate of Para-
bolic Varying Thickness,” Advanced Studies of Theory
Physics, Vol. 4, No. 10, 2010, pp. 467-486.
[15] K. Bhasker and B. Kaushik, “Simple and Exact Series
Solutions for Flexure of Orthotropic Rectangular Plates
with any Combination of Clamped and Simply Supported
Edges,” Composite Structure, Vol. 63, No. 1, 2004, pp.
63-81.