ABSTRACT

It is shown that a compact spacelike hypersurface which is contained in the chronological future (or past) of an equator of de Sitter space is a totally umbilical round sphere if the kth mean curvature function H_k is a linear combination of H_k+1,∙∙∙, H_n. This is a new angle to characterize round spheres.

1. Introduction

Let 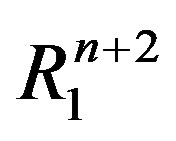be the (n + 2)-dimensional Lorentz-Minkowski space, namely, the real vector space 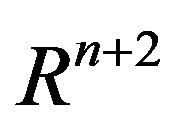endowed with the Lorentzian inner product given by

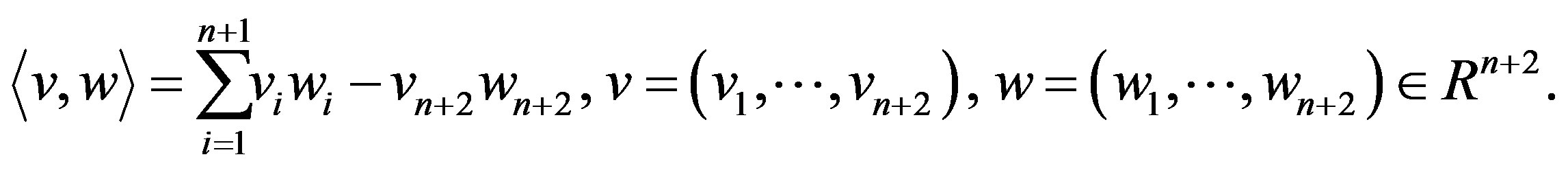
Then the n-dimensional de Sitter space is defined by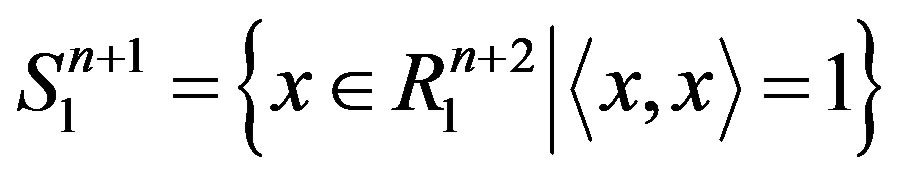. It is well known that, for 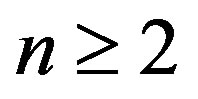the de Sitter space 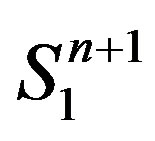is the standard simply connected Lorentzian space form of positive constant sectional curvature. A smooth immersio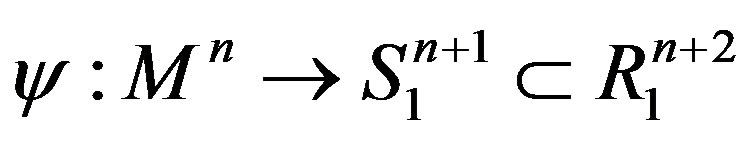n of an 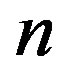-dimensional connected manifold 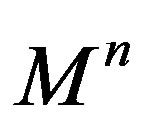is said to be a spacelike hypersurface if the induced metric via 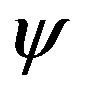is a Riemannian metric on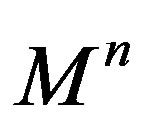, which, as usual, is also denoted by.

The interest for the study of spacelike hypersurfaces in de Sitter space is motivated by the fact that such hypersurfaces exhibit nice Bernstein-type properties. In 1977, Goddard [1] conjectured that the only complete spacelike hypersurfaces with constant mean curvature in 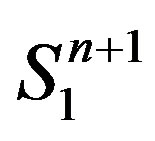should be the totally umbilical ones. This conjecture motivated the work of an important number of authors who considered the problem of characterizing the totally umbilical spacelike hypersurfaces of de Sitter space. In [2], Montiel showed that the only compact spacelike hypersurfaces in 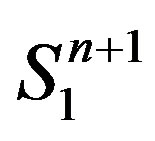with constant mean curvature 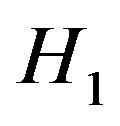were the totally umbilical round spheres. More recently, Cheng and Ishikawa [3] have shown that the totally umbilical round spheres are the only compact spacelike hypersurfaces in de Sitter space with constant scalar curvature.

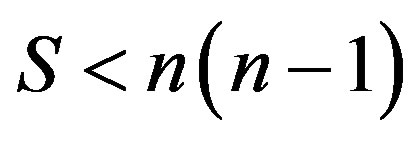The natural generalization of mean and scalar curvature for a spacelike hypersurface in de Sitter space are the kth mean curvature 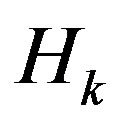for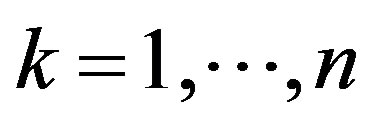. Actually, 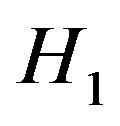is the mean curvature and 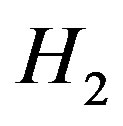is, up to a constant, the scalar curvature of the hypersurface. In [4], Aledo, jointly with Alias and Romero, developed some integral formulas for compact spacelike hypersurfaces in 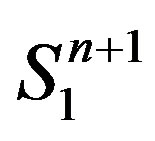and applied them in order to characterize the totally umbilical round spheres of.

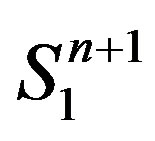Theorem 1([4], Theorem 7) Let 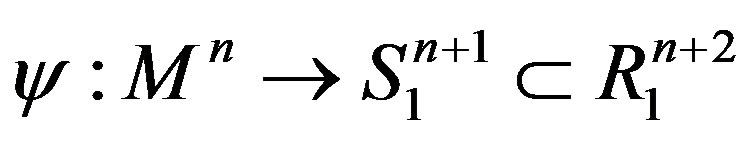be a compact spacelike hypersurface in de Sitter space which is contained in the chronological future (or past) of an equator o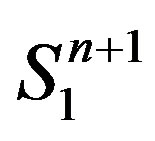f. If 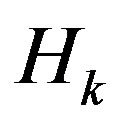is constant for some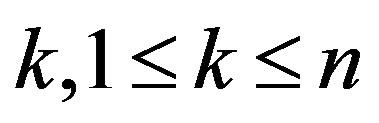, then 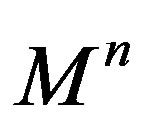is a totally umbilical round sphere.

Since 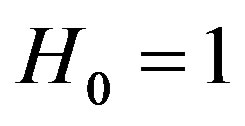by definition, the result above can be read as follows: if 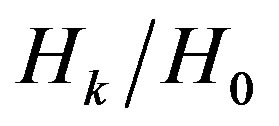is constant for some, then 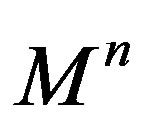is a totally umbilical round sphere. In [5], Alias extended Theorem 1 in the following way.

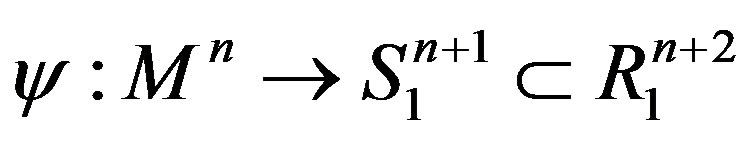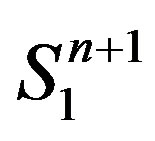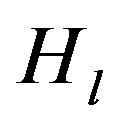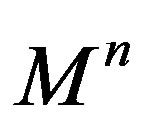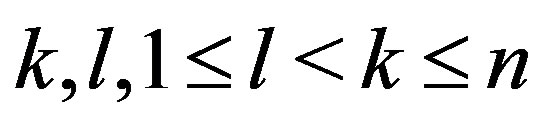Theorem 2 ([5]) Let be a compact spacelike hypersurface in de Sitter space which is contained in the chronological future (or past) of an equator of. If does not vanish on and the ratio is constant for some, then 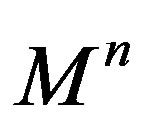is a totally umbilical round sphere.

In [6] the authors considered that 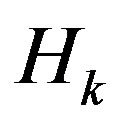is the linear combination of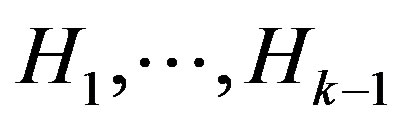, and proved:

Theorem 3 ([6]) Let 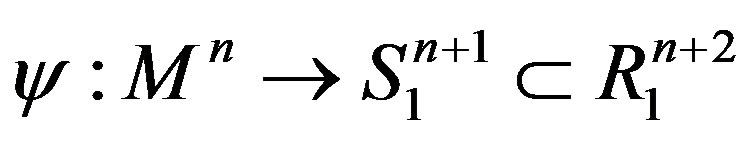be a compact spacelike hypersurface in de Sitter space which is contained in the chronological future (or past) of an equator of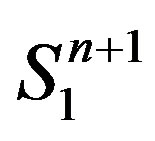. If there are nonnegative constants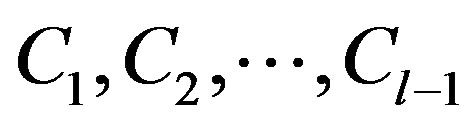, at least one 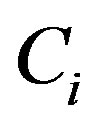is positive, such that holds on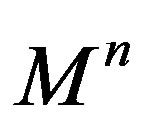, then is a totally umbilical round sphere.

In this paper, we will show another characterization of totally umbilical round sphere, which extends Theorems 1 and 2 above.

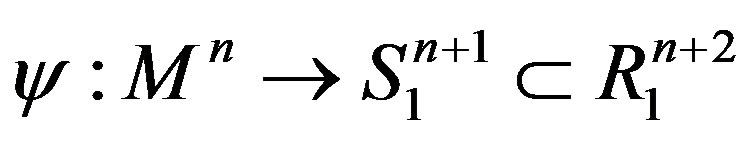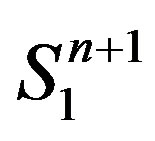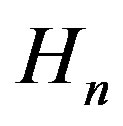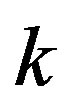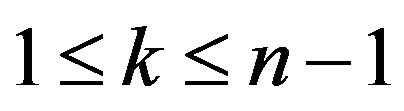Theorem 4 Let be a compact spacelike hypersurface in de Sitter space which is contained in the chronological future (or past) of an equator of. If does not vanish onŸ  for some fixed, , there exist constants 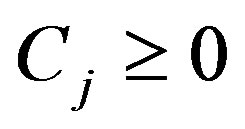such that

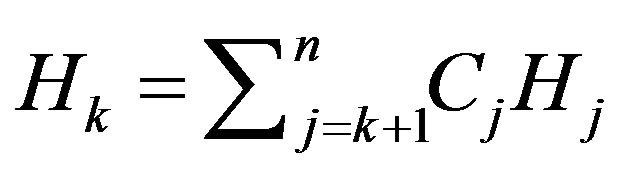on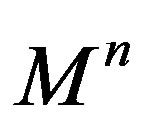, then 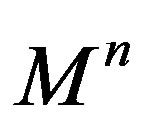is a totally umbilical round sphere.

Ÿ  there are 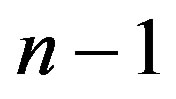constants 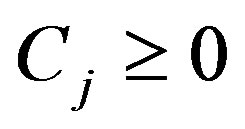such that 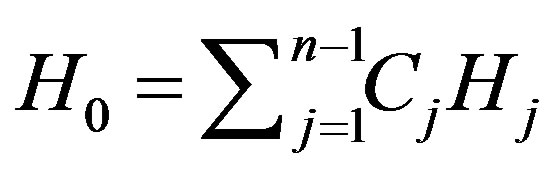on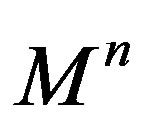, then 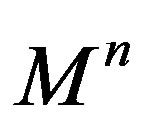is a totally umbilical round sphere.

Ÿ  Remark.

Ÿ  Note in some special cases the condition 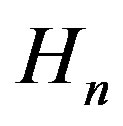does not vanish should can be dropped, for examples, only one coefficient 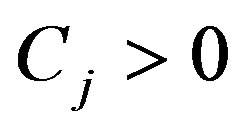case. However in general cases we can not drop it now.

Ÿ  The corresponding theorem characterizes ellipsoids also holds in affine differential geometry.

2. Preliminaries

Throughout this paper we will deal with compact spacelike hypersurfaces in de Sitter space. Recall that every compact spacelike hypersurfaces 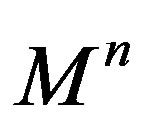in 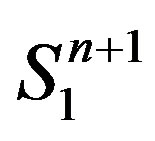is diffeomorphic to an n-sphere [4] and, in particular, it is orientable. Then, there exists a timelike unit normal field 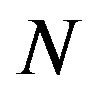globally defined on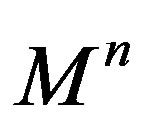. We will refer to 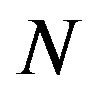as the Gauss map of the immersion and we will say that 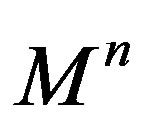is oriented by.

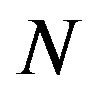We will denote by 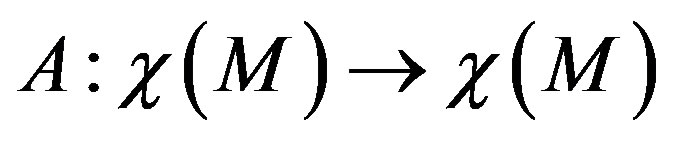the shape operator of 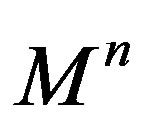in 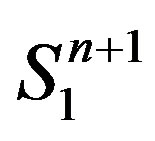with respect to, which is given by

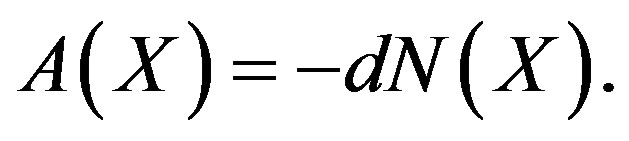
Associated to the shape operator of 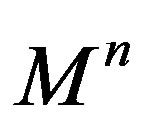there are 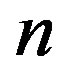algebraic invariants, which are the elementary symmetric functions 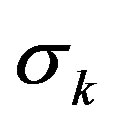of its principal curvatures 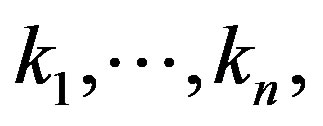given by

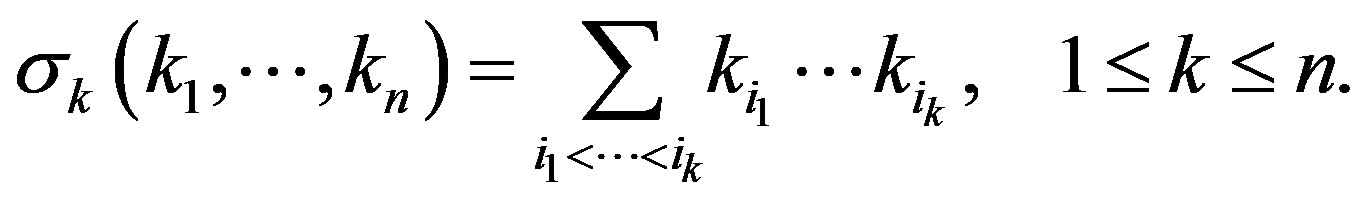
The kth mean curvature 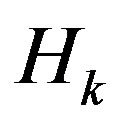of the spacelike hypersurfaces is then defined by

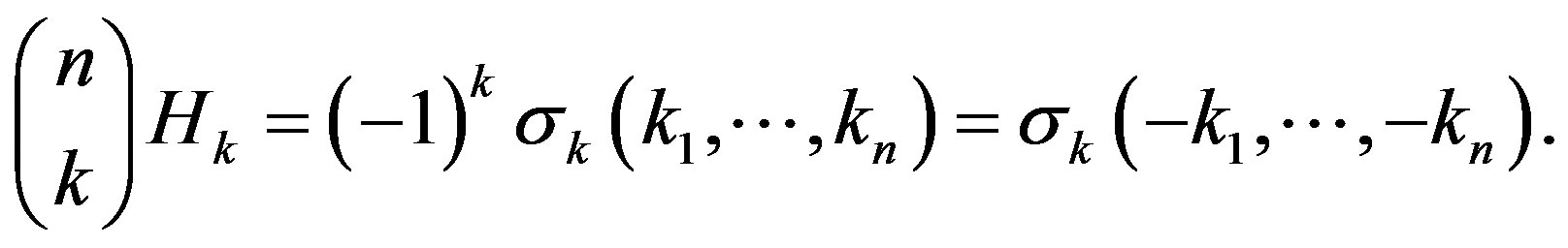
When 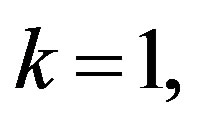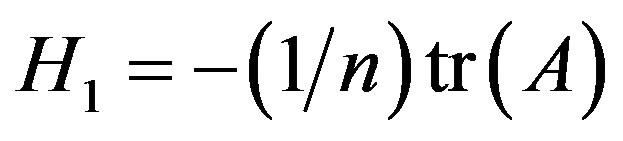is the mean curvature of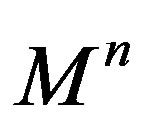. On the other hand, when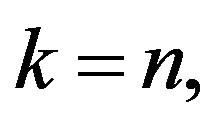 defines the Gauss-Kronecker curvature of the spacelike hypersurface, and for 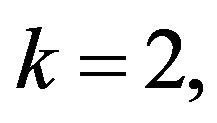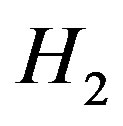is, up to a constant, the scalar curvature 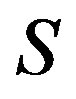of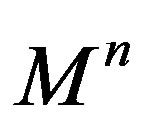, since 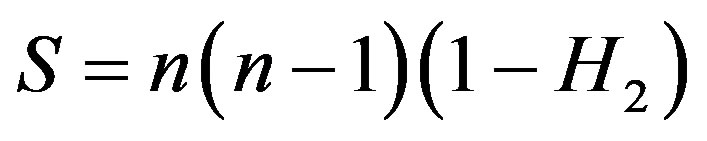(for details see [4]).

The proof of our theorem makes an essential use of the following integral formulas for compact spacelike hypersurfaces in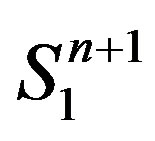, which is developed in [4].

Lemma 5 (Minkowski formulas) Let 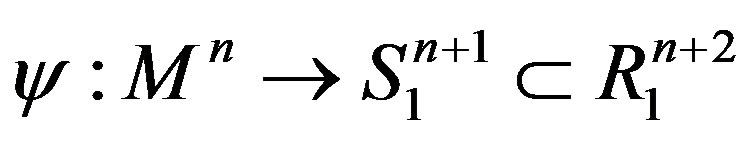be a compact spacelike hypersurface immersed into de Sitter space and let 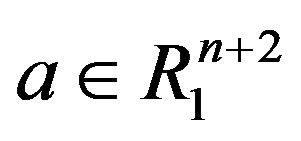a fixed arbitrary vector. For each 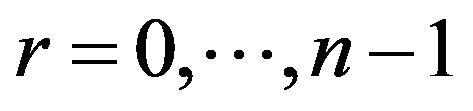the following formula holds:

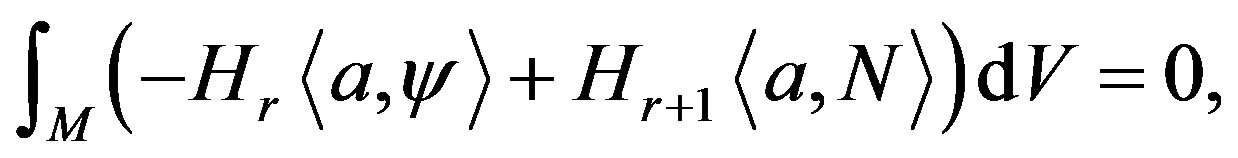
where 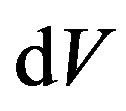is the n-dimensional volume element of 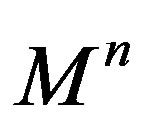with respect to the induced metric and the chosen orientation.

3. Proof of the Theorem 4

Let us assume, for instance, that the hypersurface 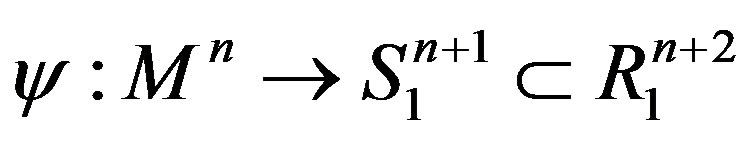is contained in the future of the equator determined by a unit timelike vector 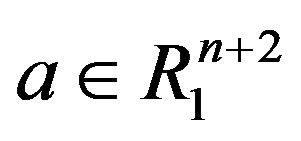(the case of the past is similar). That means that

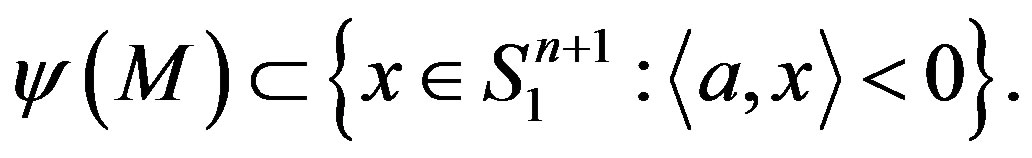
Let us orient 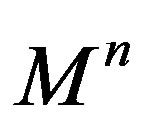by the Gauss map 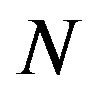which is in the same time-orientation as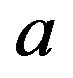, so that 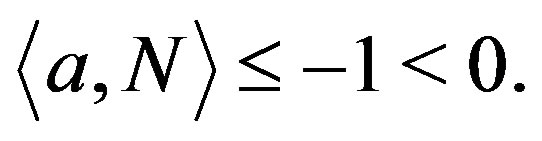Since the height function 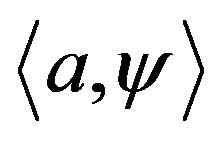is negative on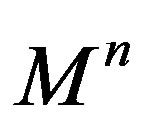, by compactness there exists a point 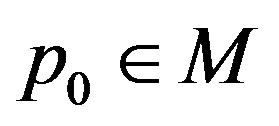where it attains its maximum

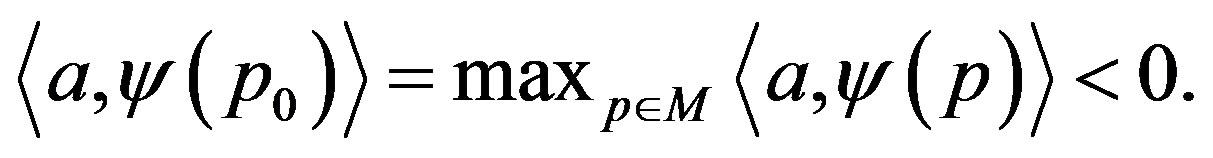
Therefore, its gradient vanishes at that point, 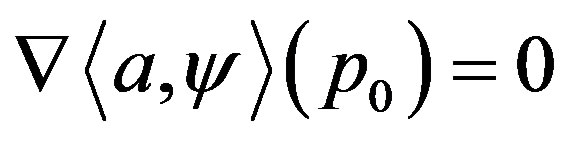, and its Hessian satisfies

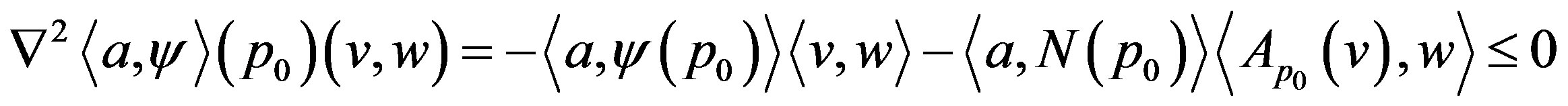
for all 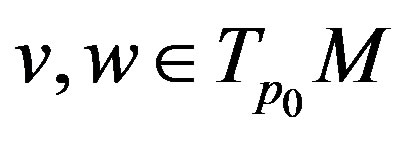(for the details see the proof of Theorem 7 in [4]). On the other hand, since

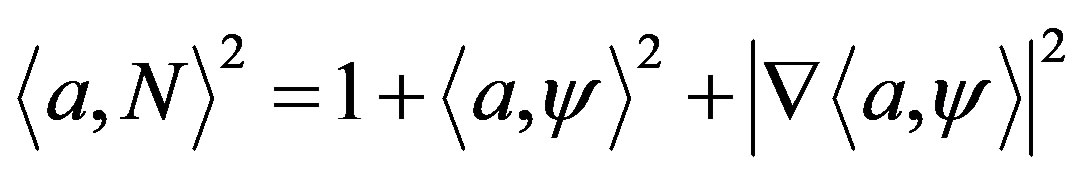
and

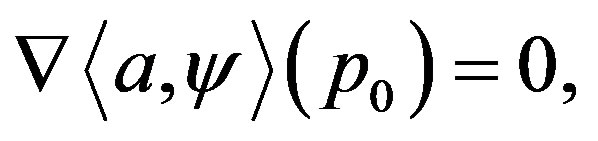
then

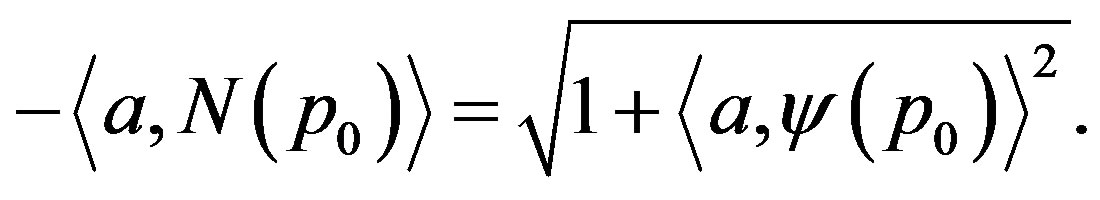
Therefore, choosing 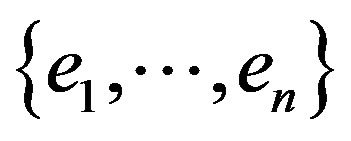a basis of principal directions at the point 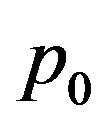we conclude that

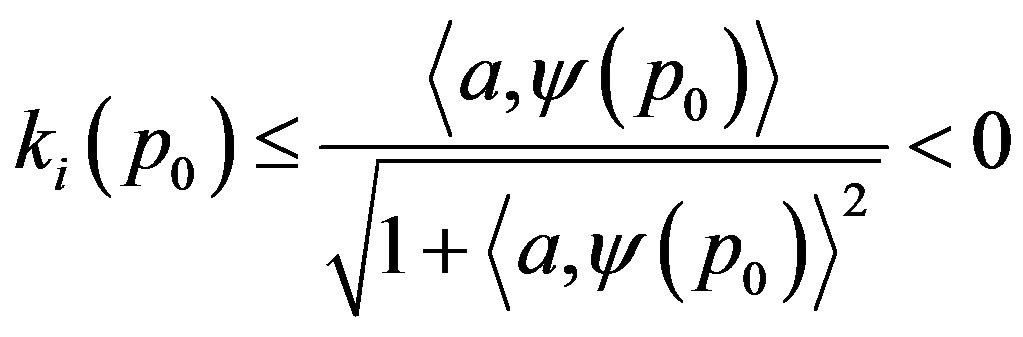(1)

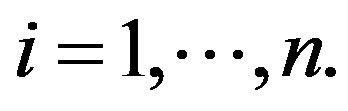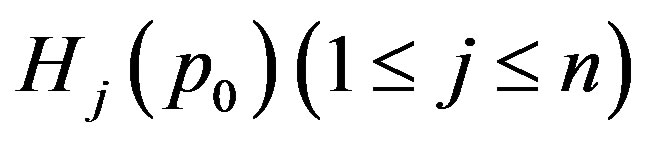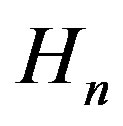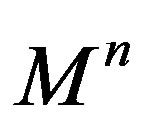for each In particular, are positive. The mean curvature functions is positive on (recall that does not vanish on by assumption). Therefore, from the proof of Lemma 1 in [7] and taking into account the sign convention in our definition of the higher order mean curvature, it follows that every 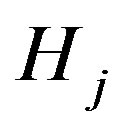is positive for 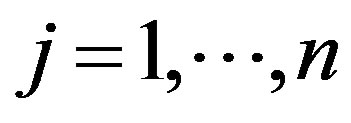and

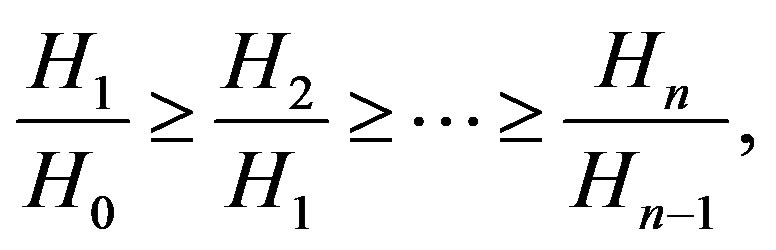(2)

with equality at any stage only at umbilical points.

Let us start proving the first statement of Theorem 4. Using

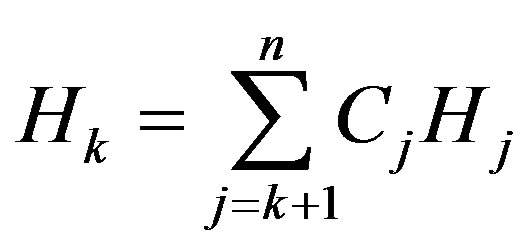
and the Minkowski formulae, we have

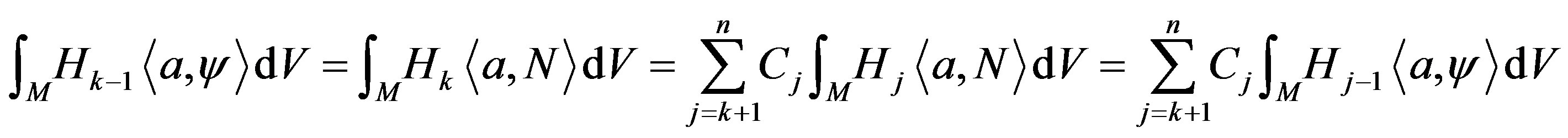
That is,

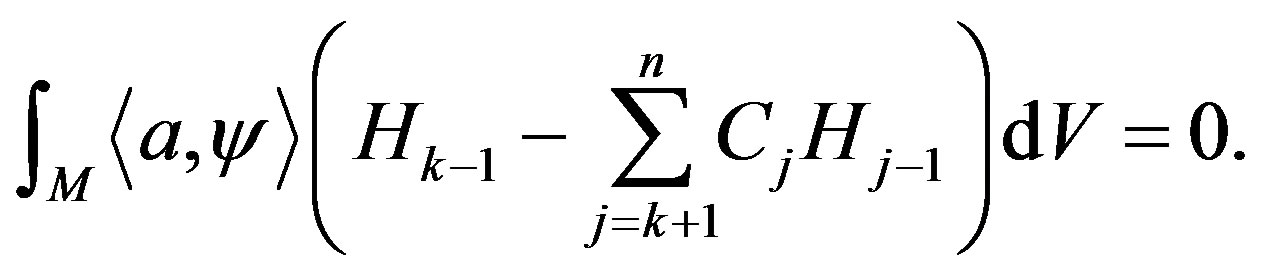
Now we claim that

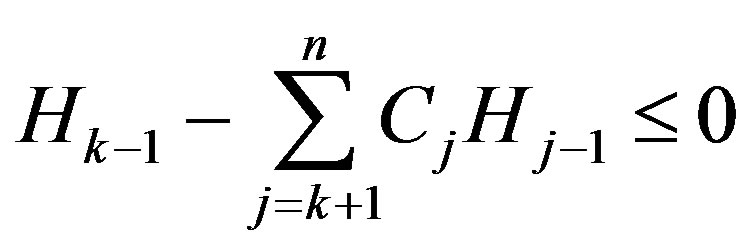(3)

on, with equality if and only if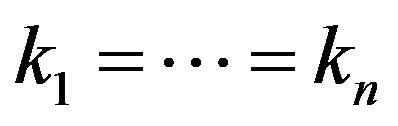. Assume that (3) is true. Then, since 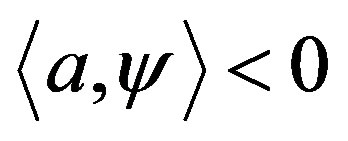on, we conclude that

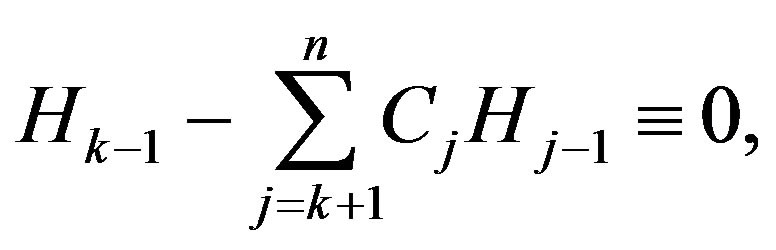
which implies that 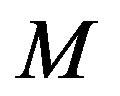is an totally umbilical round sphere.

It remains to prove (3). Using the assumption of theorem 4, that is 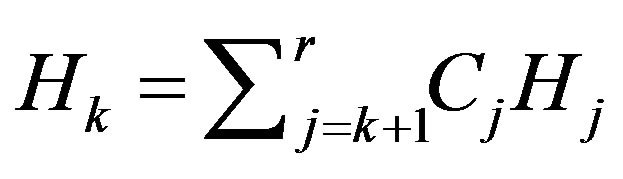and (2), we have

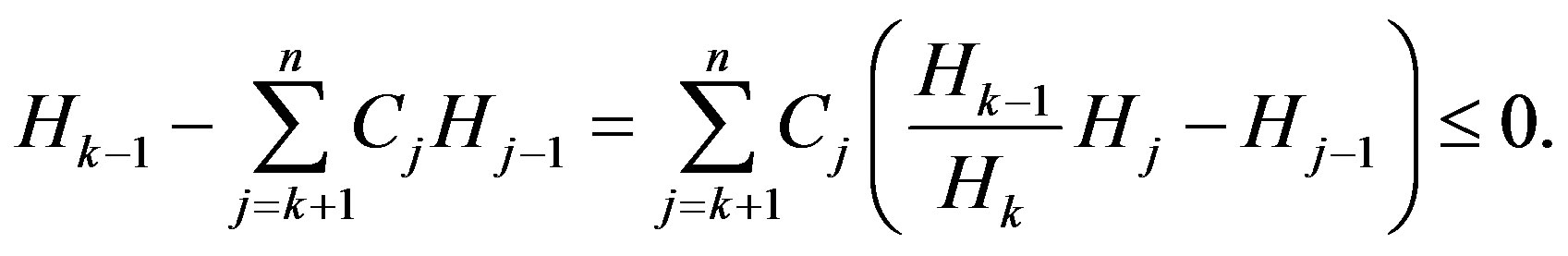(4)

Now we prove the second statement. Using

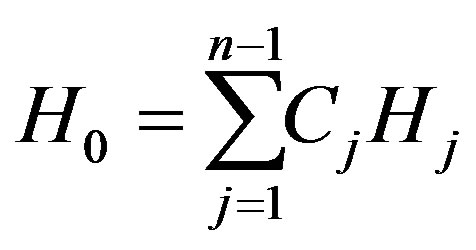
and the Minkowski formulae, we have

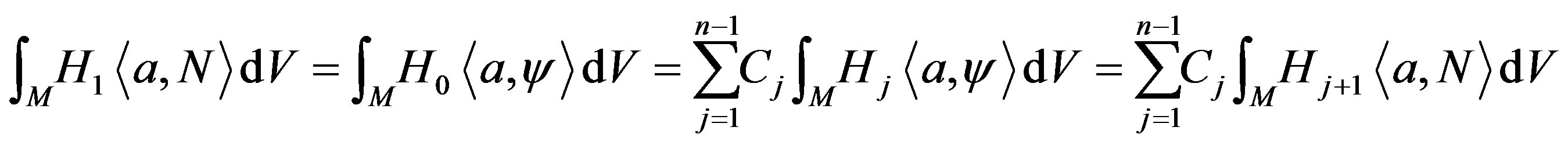
That is,

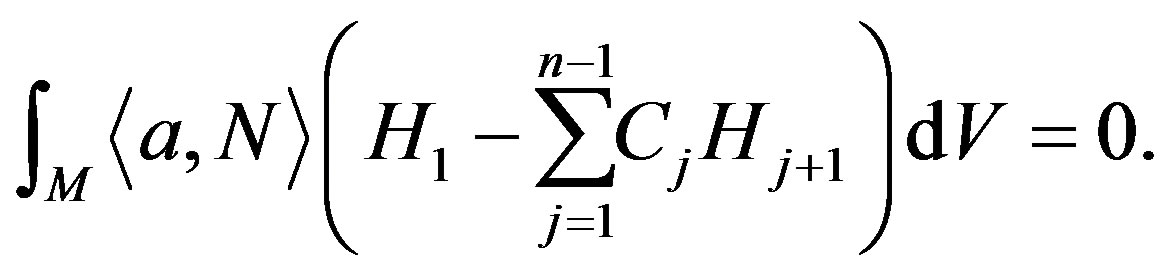
Now we claim that

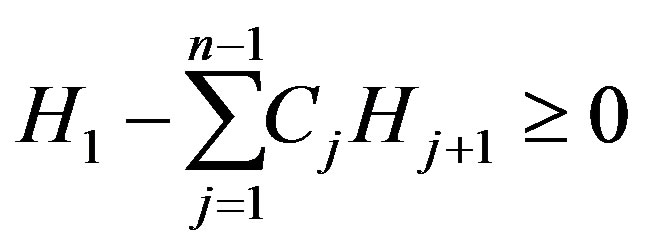(5)

on, with equality if and only if. Assume that (5) is true. Then, since on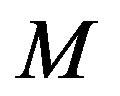, we conclude that

which implies that 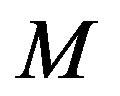is an totally umbilical round sphere. It remains to prove (5). As in the first proof, using the assumption of theorem 4, that is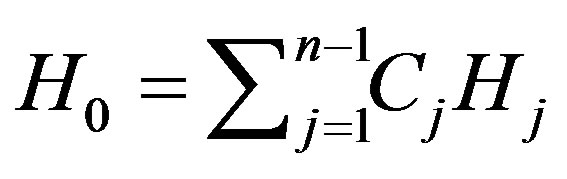, and (2) we known

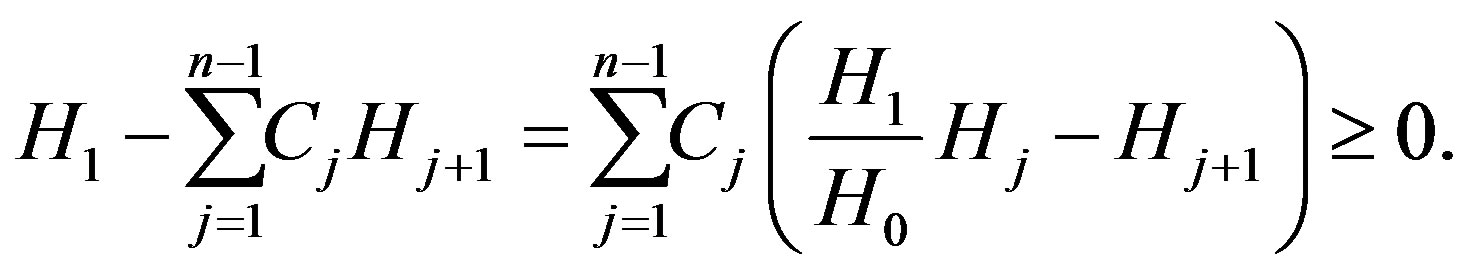
This completes the proof of the Theorem 4.

Funding

This work is supported by grant (No.U1304101 and 11171091) of NSFC and NSF of Henan Province (No.132300410141).

REFERENCES

[1]       A. J. Goddard, “Some Remarks on the Existence of Spacelike Hypersurfaces of Constant Mean Curvature,” Mathematical Proceedings of the Cambridge Philosophical Society, Vol. 82, 1977, pp. 489-495. http://dx.doi.org/10.1017/S0305004100054153

[2]       S. Montiel, “An Integral Inequality for Compact Spacelike Hypersurfaces in de Sitter Space and Applications to the Case of Constant Mean Curvature,” Indiana University Mathematics Journal, Vol. 37, No. 4, 1988, pp. 909-917. http://dx.doi.org/10.1017/S0305004100054153

[3]       Q.-M. Cheng and S. Ishikawa, “Spacelike Hypersurfaces with Constant Scalar Curvature,” Manuscripta Mathematica, Vol. 95, No. 4, 1998, pp. 499-505. http://dx.doi.org/10.1007/s002290050043

[4]       J. A. Aledo, L. J. Alias and A. Romero, “Integral Formulas for Compact Space-Like Hypersurfaces in de Sitter Space: Applications to the Case of Constant Higher Mean Curvature,” Journal of Geometry and Physics, Vol. 31, No. 2-3, 1999, pp. 195-208. http://dx.doi.org/10.1016/S0393-0440(99)00008-X

[5]       L. J. Alias and S.-E. Koh, “Remarks on Compact Spacelike Hypersurfaces in de Sitter Space with Constant Higher Order Mean Curvature,” Journal of Geometry and Physics, Vol. 39, No. 1, 2001, pp. 45-49. http://dx.doi.org/10.1016/S0393-0440(00)00073-5

[6]       S.-E. Koh and M. S. Yoo, “A Characterization of Totally Umbilical Hypersurfaces in de Sitter Space,” Journal of Geometry and Physics, Vol. 51, No.1, 2004, pp. 34-39. http://dx.doi.org/10.1016/j.geomphys.2003.09.006

[7]       S. Montiel and A. Ros, “Compact Hypersurfaces: The Alexandrov Theorem for Higher Order Mean Curvatures,” In: B. Lawson and K. Tenenblat, Eds., Differential Geometry, Longman, Essex, 1991, pp. 279-296.

[8]       K. Akutagawa, “On Spacelike Hypersurfaces with Constant Mean Curvature in the de Sitter Space,” Mathematische Zeitschrift, Vol. 196, No. 1, 1987, pp. 13-19. http://dx.doi.org/10.1007/BF01179263

[9]    L. J. Alias and A. G. Colares, “A Further Characterization of Ellipsoids,” Results in Mathematics, Vol. 48, No.1-2, 2005, pp. 1-8. http://dx.doi.org/10.1007/BF03322891

[10]    H. Li, “Global Rigidity Theorems of Hypersurfaces,” Arkiv for Matematik, Vol. 35, No. 2, 1997, pp. 327-351.

[11]    J. Ramanathan, “Complete Spacelike Hypersurfaces of Constant Mean Curvature in de Sitter Space,” Indiana University Mathematics Journal, Vol. 36, No. 2, 1987, pp. 349-359. http://dx.doi.org/10.1512/iumj.1987.36.36020

[12]    Y. Zheng, “On Space-Like Hypersurfaces in the de Sitter Space,” Annals of Global Analysis and Geometry, Vol.13, No. 4, 1995, pp. 317-321. http://dx.doi.org/10.1007/BF00773403

[13]    Y. Zheng, “Space-Like Hypersurfaces with Constant Scalar Curvature in the de Sitter Space,” Differential Geometry and Its Applications, Vol. 6, No. 1, 1996, pp. 51-54. http://dx.doi.org/10.1016/0926-2245(96)00006-X