Applied Mathematics
Vol.5 No.8(2014), Article ID:45710,8 pages DOI:10.4236/am.2014.58118
Generalization of Uniqueness Theorems for Entire and Meromorphic Functions
Harina P. Waghamore1, N. Shilpa2
1Department of Mathematics, Central College Campus, Bangalore University, Bangalore, India
2P. G. Department of Mathematics, M. E. S. College, Bangalore, India
Email: pree.tam@rediffmail.com, shilpajaikumar@gmail.com
Copyright © 2014 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/


Received 3 February 2014; revised 3 March 2014; accepted 10 March 2014
ABSTRACT
In this paper, we deal with the uniqueness problems on entire and meromorphic functions concerning differential polynomials that share fixed-points. Moreover, we generalise and improve some results of Weichuan Lin, Hongxun Yi, Meng Chao, C. Y. Fang, M. L. Fang and Junfeng xu.
Keywords
Nevanlinna Theory, Uniqueness, Entire Functions, Meromorphic Functions, Differential Polynomials, Fixed Points

1. Introduction
In this paper, the term “meromorphic” will always mean meromorphic in the complex plane C. Let a be a complex number and  be a meromorphic function such that
be a meromorphic function such that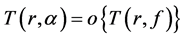 . We say f and g share the value
. We say f and g share the value  CM, if
CM, if  and
and ![]() assume the same zeros with the same multiplicities; if
assume the same zeros with the same multiplicities; if 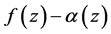 and
and  assume the same zeros with the same multiplicities, then we say
assume the same zeros with the same multiplicities, then we say  and
and 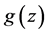 share
share  CM, especially we say that
CM, especially we say that 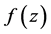 and
and 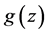 have the same fixed-points when
have the same fixed-points when . It is assumed that the reader is familiar with the notations of the Nevanlinna theory that can be found, for instance, in [1] . We denote by
. It is assumed that the reader is familiar with the notations of the Nevanlinna theory that can be found, for instance, in [1] . We denote by 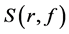 any function satisfying
any function satisfying
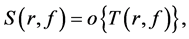
as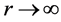 , possibly outside of finite measure.
, possibly outside of finite measure.
Set
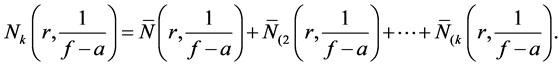
It is well known that if f and g share four distinct values CM, then f is a fractional transformation of g. In 1997, corresponding to one famous question of Hayman, C. C. Yang and X. H. Hua showed the similar conclusions hold for certain types of differential polynomials when they share only one value. They proved the following result.
Theorem A ([2] ). Let f and g be two non-constant meromorphic functions, ![]() be an integer and
be an integer and . If
. If 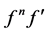 and
and 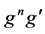 share the value a CM, then either
share the value a CM, then either 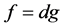 for some
for some 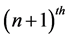 root of unity d or
root of unity d or ![]() and
and![]() , where
, where ![]() and
and 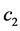 are constants and satisfy
are constants and satisfy 
In 2001, M. L. Fang and W. Hong obtained the following result.
Theorem B ([3] ). Let f and g be two transcendental entire functions, ![]() an integer. If
an integer. If ![]() and
and ![]() share the value 1 CM, then
share the value 1 CM, then .
.
Recently, W. C. Lin and H. X. Yi extended the above theorem with respect to fixed point. They proved the following results.
Theorem C ([4] ). Let  and
and ![]() be two transcendental meromorphic functions,
be two transcendental meromorphic functions, ![]() an integer. If
an integer. If ![]() and
and ![]() share z CM, then either
share z CM, then either 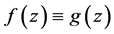 or
or
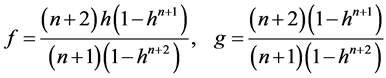
where h is a nonconstant meromorphic function.
Theorem D ([4] ). Let 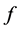 and
and ![]() be two transcendental meromorphic functions,
be two transcendental meromorphic functions, ![]() an integer. If
an integer. If 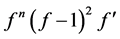 and
and  share z CM, then
share z CM, then .
.
We generalise the above results and prove the following Theorem.
Theorem 1.1 Let  and
and ![]() be two transcendental meromorphic functions,
be two transcendental meromorphic functions, ![]() an integer. If
an integer. If 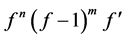 and
and 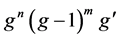 share
share 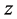 CM then
CM then 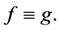
For![]() , we get Theorem C.
, we get Theorem C.
For![]() , we get Theorem D.
, we get Theorem D.
One may ask the following question, can the nature of the fixed point z be relaxed to IM in the above theorems?
In 2008, Meng Chao answered to the above question and proved the following theorems.
Theorem E ([5] ). Let  and
and ![]() be two transcendental meromorphic functions,
be two transcendental meromorphic functions, ![]() an integer. If
an integer. If ![]() and
and ![]() share z IM, then either
share z IM, then either 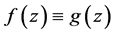 or
or
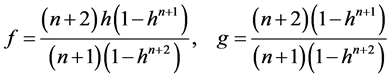
where h is a nonconstant meromorphic function.
Theorem F([5] ). Let  and
and ![]() be two transcendental meromorphic functions,
be two transcendental meromorphic functions, ![]() an integer. If
an integer. If  and
and  share z IM, then
share z IM, then .
.
We generalise the above results and prove the following Theorem.
Theorem 1.2 Let 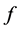 and
and ![]() be two transcendental meromorphic functions,
be two transcendental meromorphic functions, ![]() an integer. If
an integer. If  and
and  share
share 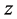 IM then
IM then 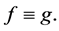
For![]() , we get
, we get ![]() which improves Theorem E.
which improves Theorem E.
For![]() , we get
, we get![]() , we get Theorem F.
, we get Theorem F.
In 2002, Fang and Fang [6] proved that there exists a differential polynomial d such that for any pair of nonconstant entire functions f and g we can get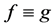 , if
, if 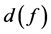 and
and 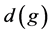 share one value CM.
share one value CM.
Theorem G ([6] ). Let  and
and ![]() be two nonconstant entire functions,
be two nonconstant entire functions, ![]() be a positive integer. If
be a positive integer. If ![]() and
and ![]() share 1 CM, then
share 1 CM, then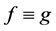 .
.
In 2004, Lin-Yi [7] and Qiu-Fang [8] proved that Theorem G remains valid for![]() .
.
Theorem H ([7] [8] ). Let  and
and ![]() be two nonconstant entire functions,
be two nonconstant entire functions, ![]() be a positive integer. If
be a positive integer. If  and
and ![]() share 1 CM, then
share 1 CM, then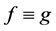 .
.
We generalise the above results and prove the following theorem.
Theorem 1.3 Let f and g be two transcendental entire functions, ![]() an integer. If
an integer. If  and
and  share z CM then
share z CM then 
For![]() ,
, ![]() we get Theorem H.
we get Theorem H.
For![]() ,
, ![]() , we get new result.
, we get new result.
Fang-Fang discussed Theorem H by replacing CM with IM and proved the following Theorem.
Theorem I ([6] ). Let f and g be two nonconstant entire functions, n be a positive integer. If ![]() and
and ![]() share 1 IM and
share 1 IM and![]() , then
, then .
.
We generalise the above results and prove the following Theorem.
Theorem 1.4 Let f and g be two transcendental entire functions, ![]() an integer. If
an integer. If  and
and 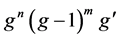 share z IM then
share z IM then 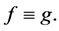
For![]() ,
, ![]() which improves Theorem I.
which improves Theorem I.
For![]() ,
, ![]() , we get new result.
, we get new result.
2. Some Lemmas
Lemma 2.1 ([9] ) Let f be a nonconstant meromorphic function, n be a positive integer. ![]() where ai is a meromorphic function satisfying
where ai is a meromorphic function satisfying 
 . Then
. Then

Lemma 2.2 ([10] ) Let f be a non-constant meromorphic function k be a positive integer, then
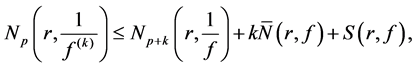
where  denotes the counting function of the zero’s of
denotes the counting function of the zero’s of 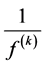 where a zero of multiplicity m is counted m times if
where a zero of multiplicity m is counted m times if  and p times if
and p times if![]() . Clearly
. Clearly .
.
Lemma 2.3 ([11] [12] ) Let F and G be two nonconstant meromorphic functions sharing the value 1 IM. Let
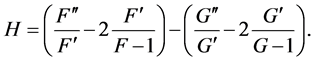
If![]() , then
, then
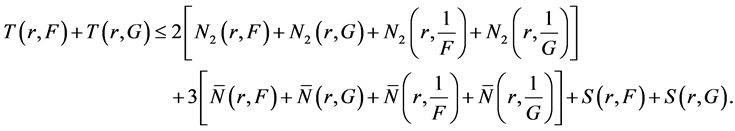
Lemma 2.4 ([5] ) Let f and g be two nonconstant meromorphic functions, ![]() ,
, ![]() positive integers,
positive integers,  denotes as in section 1 and
denotes as in section 1 and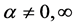 , and let
, and let

if F and G share  IM, then
IM, then 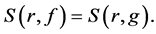
Lemma 2.5 ([13] ) Let H be defined as above. If ![]() and
and

where 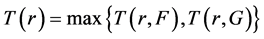 and I is a set with infinite linear measure, then
and I is a set with infinite linear measure, then ![]() or
or![]() .
.
Lemma 2.6 ([14] ) Let 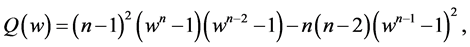 then
then
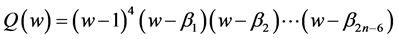
where 
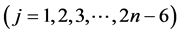 , which are distinct respectively.
, which are distinct respectively.
3. Proofs of the Theorems
In this section, we present the proofs of the main results.
Proof of Theorem 1.2.
Lemma 2.4 implies that .
.
Let
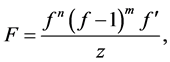 (1)
(1)
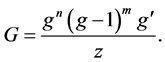 (2)
(2)
and
 (3)
(3)
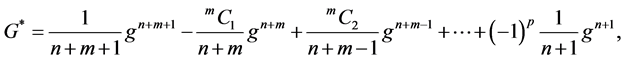 (4)
(4)
where 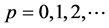
Thus we obtain that F and G share the value 1 IM. Moreover, by Lemma 2.1, we have
 (5)
(5)
 (6)
(6)
Noting that , we deduce
, we deduce
 (7)
(7)
and by the First Fundamental Theorem,
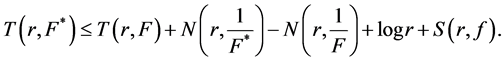 (8)
(8)
Note that,
 (9)
(9)
where 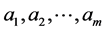 are distinct roots of the algebraic equation
are distinct roots of the algebraic equation
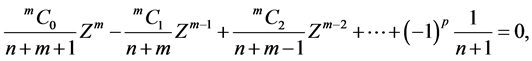
and
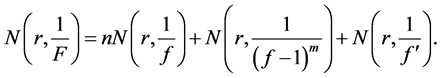 (10)
(10)
Since F and G share 1 IM, by Lemma 2.3, we have
 (11)
(11)
Obviously, we have
 (12)
(12)
 (13)
(13)
So, we have
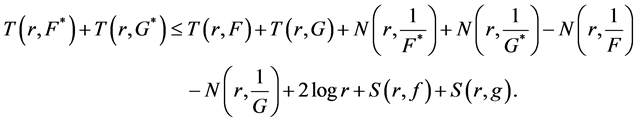 (14)
(14)
From (5) to (14), we have
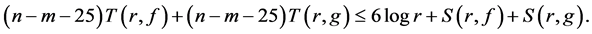 (15)
(15)
We obtain that ![]() which contradicts
which contradicts![]() .
.
Therefore![]() , that is
, that is
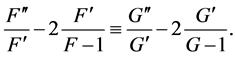 (16)
(16)
By integration, we have
 (17)
(17)
where 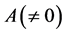 and B are constants. Thus
and B are constants. Thus
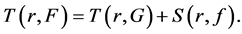 (18)
(18)
Since,
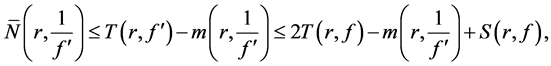 (19)
(19)
we note that,
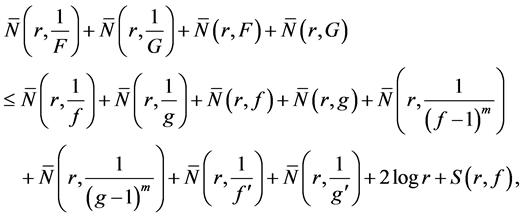 (20)
(20)
and
 (21)
(21)
Similarly, we have
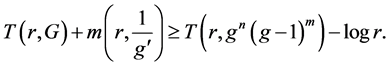 (22)
(22)
From (19) to (22) and applying Lemma 2.5, we get
![]() or
or![]() .
.
We discuss the following cases.
Case (i) Suppose that![]() .
.
As in the proof of Theorem 1, in [5] we arrive at a contradiction.
Case (ii)![]() , thus
, thus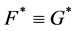 , that is,
, that is,
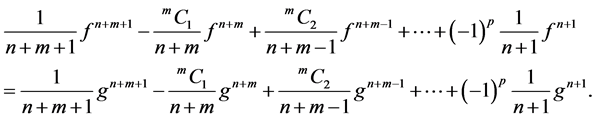
Set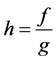 , we substitute
, we substitute 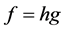 in the above, it follows that
in the above, it follows that
 (23)
(23)
If h is not a constant, using Lemma 2.6 and (23), we conclude that

By (23), we get
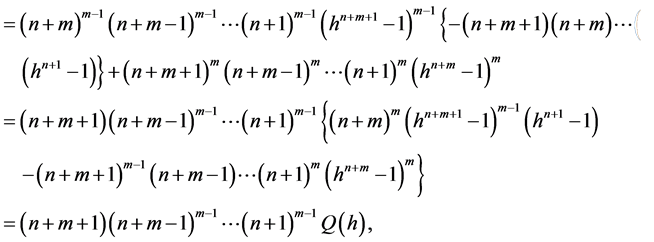
where

Using Lemma 2.6, we get

where  which are pairwise distinct.
which are pairwise distinct.
This implies that every zero of 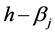
 has a multiplicity of at least n. By the Second Fundamental Theorem, we obtain that
has a multiplicity of at least n. By the Second Fundamental Theorem, we obtain that![]() , which is again a contradiction.
, which is again a contradiction.
Therefore h is a constant. We have from (23) that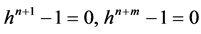 , which imply
, which imply , and hence
, and hence .
.
Proof of Theorem 1.1. Let F and G be given by (1) and (2). Suppose H is given as in Lemma 2.3, and![]() . Proceeding as in the proof of Theorem 1.2 we can obtain (3) to (10). Since F and G share 1 CM, by Lemma 2.3, we have
. Proceeding as in the proof of Theorem 1.2 we can obtain (3) to (10). Since F and G share 1 CM, by Lemma 2.3, we have

Hence from (3) to (10) and (12) to (14), we get

Hence![]() , which contradicts that
, which contradicts that![]() .
.
Proof of Theorem 1.4. Let F and G be given by (1) and (2). Suppose H is given as in Lemma 2.3, and![]() . Proceeding as in the proof of Theorem 1.2 we can obtain (3) to (10) and (11). Since F and G share 1 IM, by Lemma 2.3, obviously we have,
. Proceeding as in the proof of Theorem 1.2 we can obtain (3) to (10) and (11). Since F and G share 1 IM, by Lemma 2.3, obviously we have,


therefore (14) reduces to

Hence![]() , which contradicts that
, which contradicts that![]() . Proceeding in the same way as in Theorem 1.2 we get
. Proceeding in the same way as in Theorem 1.2 we get .
.
Proof of Theorem 1.3. Let F and G be given by (1) and (2). Suppose H is given as in Lemma 2.3, and![]() . Proceeding as in the proof of Theorem 1.2 we can obtain (3) to (10). Since F and G share 1 CM, by Lemma 2.3, we have
. Proceeding as in the proof of Theorem 1.2 we can obtain (3) to (10). Since F and G share 1 CM, by Lemma 2.3, we have
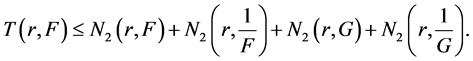
Hence from (3) to (10) and (12) to (14), we get
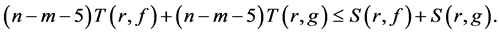
Hence![]() , which contradicts that
, which contradicts that![]() . Proceeding in the same way as in Theorem 1.2, we get
. Proceeding in the same way as in Theorem 1.2, we get .
.
Acknowledgements
The author would like thank Prof. S. S. Bhoosnurmath for his valuable suggestions for the improvement of the paper. This research was supported by the UGC under MRP(S).
References
- Hayman, W.K. (1964) Meromorphic Functions. Clarendon Press, Oxford.
- Yang, C.C. and Hua, X.H. (1997) Uniqueness and Value-Sharing of Meromorphic Functions. Annales Academiæ Scientiarum Fennicæ Mathematica, 22, 395-406.
- Fang, M.L. and Hong, W. (2001) A Unicity Theorem for Entire Functions Concerning Differential Polynomials, Indian. Journal of Pure and Applied Mathematics, 32, 1343-1348.
- Lin, W.C. and Yi, H.X. (2004) Uniqueness Theorem for Meromorphic Functions Concerning Fixed Points. Complex Variables, Theory and Application, 49, 793-806.
- Chao, M. (2008) Uniqueness Theorems for Differential Polynomials Concerning Fixed Points. Kyungpook Mathematical Journal, 48, 25-35. http://dx.doi.org/10.5666/KMJ.2008.48.1.025
- Fang, C.Y. and Fang, M.L. (2002) Uniqueness of Meromorphic Functions and Differential Polynomials. Computers & Mathematics with Applications, 44, 607-617. http://dx.doi.org/10.1016/S0898-1221(02)00175-X
- Lin, W.C. and Yi, H.X. (2004) Uniqueness Theorems for Meromorphic Functions. Indian Journal of Pure and Applied Mathematics, 35, 121-132.
- Qiu, H.L. and Fang, M.L. (2004) On the Uniqueness of Entire Functions. Bulletin of the Korean Mathematical Society, 41, 109-116. http://dx.doi.org/10.4134/BKMS.2004.41.1.109
- Yang, C.C. and Yi, H.X. (2003) Uniqueness Theory of Meromorphic Functions. Kluwer Academic Publishers, London.
- Zhang, Q.C. (2005) Meromorphic Functions That Share One Small Functions with Its Derivatives. Journal of Inequalities in Pure and Applied Mathematics, 6, Article ID: 116.
- Xu, J.F. and Yi, H.X. (2007) Uniqueness of Entire Functions and Differential Polynomials. Bulletin of the Korean Mathematical Society, 44, 623-629. http://dx.doi.org/10.4134/BKMS.2007.44.4.623
- Yi, H.X. (1999) Meromorphic Functions That Share One or Two Values II. Kodai Mathematical Journal, 22, 264-272. http://dx.doi.org/10.2996/kmj/1138044046
- Yi, H.X. (1995) Meromorphic functions That Share One or Two Values. Complex Variables, Theory and Application, 28, 1-11.
- Frank, G. and Reiders, M. (1998) A Unique Range Set for Meromorphic Functions with 11 Elements. Complex Variables, Theory and Application, 37, 185-193.

