Applied Mathematics
Vol. 3 No. 7 (2012) , Article ID: 19865 , 7 pages DOI:10.4236/am.2012.37115
Limit Cycle Bifurcations in a Class of Cubic System near a Nilpotent Center*
Department of Mathematics, Shanghai Maritime University, Shanghai, China
Email: jiaojiang08@yahoo.cn
Received April 25, 2012; revised June 4, 2012; accepted June 11, 2012
Keywords: Near-Hamiltonian System; Nilpotent Center; Hopf Bifurcation; Limit Cycle
ABSTRACT
In this paper we deal with a cubic near-Hamiltonian system whose unperturbed system is a simple cubic Hamiltonian system having a nilpotent center. We prove that the system can have 5 limit cycles by using bifurcation theory.
1. Introduction
In the International Congress of Mathematics held in Paris in 1900, Hilbert made a list of 23 problems. The second part of Hilbert’s 16th problem is still an open and difficult question: to find a upper bound of the number of limit cycles and their relative locations in polynomial vector fields of order n.
If the singular point of the system is a non-saddle, nor nilpotent, the related Hopf bifurcations are elementary, see [1-3] and their references. Hopf bifurcations from the elementary focus type of singularities have found broad and important applications in biology, chemistry and physics and engineering, see [4-7] for examples. Yet for the bifurcation of limit cycles from a non-elementary center in a more general planar vector field, its intrinsic dynamics is still far away from understanding due to the complexity and technical difficulties in dealing with such bifurcations.
Then it was natural to restrict the study of the nilpotent center by assuming the system is a perturbation of a Hamiltonian system. Consider the following system
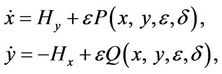 (1.1)
(1.1)
where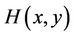 ,
,  and
and 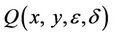 are
are 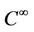 functions,
functions, 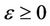 is small and
is small and 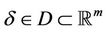 with D a compact set.
with D a compact set.
When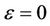 , system (1.1) becomes
, system (1.1) becomes
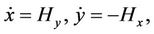 (1.2)
(1.2)
which is Hamiltonian system. Now suppose that the Hamiltonian system (1.2) has a nilpotent center at the origin, namely the function H satisfies the following conditions:
(H1) 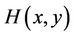 is a
is a 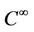 function, satisfying
function, satisfying
 ;
;
(H2) , the equation
, the equation  defines a closed curve Lh surrounding the origin and Lh approaches the origin as h goes to zero;
defines a closed curve Lh surrounding the origin and Lh approaches the origin as h goes to zero;
(H3)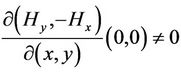 ,
, .
.
It follows that the expansion of H at the origin has the form
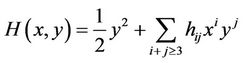
Assume that the equation  intersects the positive x-axis at
intersects the positive x-axis at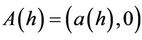 . Let
. Let  denote the first intersection point of the positive orbit of (1.1) starting at
denote the first intersection point of the positive orbit of (1.1) starting at 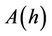 with the positive x-axis. Then, we have
with the positive x-axis. Then, we have
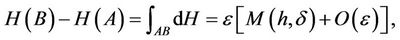 (1.3)
(1.3)
where
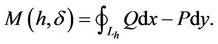 (1.4)
(1.4)
The Abelian integral M above is called the first order Melnikov function of system (1.1). From Han [8], we have a general theorem as follows.
Theorem 1.1. Suppose that the origin is nilpotent singular point 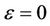 and that
and that 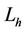 approaches the origin as h goes to zero. If there exist an integer
approaches the origin as h goes to zero. If there exist an integer 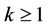 and
and  such that
such that

and
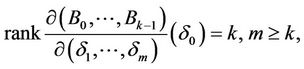
then we have 1) 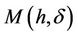 has at most k zeros near
has at most k zeros near 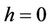 for
for  and all
and all 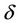 near
near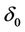 , and k zeros can appear for some
, and k zeros can appear for some 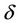 near
near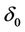 .
.
2) System (1.1) has at least k limit cycles near the origin for some 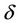 near
near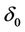 .
.
2. Main Results and Proof
Consider the following near-Hamiltonian system:
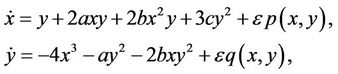 (2.1)
(2.1)
where 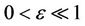 and p and q are cubic polynomials. We can write
and p and q are cubic polynomials. We can write
 (2.2)
(2.2)
Then unperturbed system 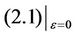 is a Hamiltonian system with Hamiltonian
is a Hamiltonian system with Hamiltonian
 (2.3)
(2.3)
system  has a nilpotent center at the origin. Let
has a nilpotent center at the origin. Let 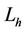 be the closed curve defined by
be the closed curve defined by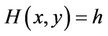 . Then it can be presented as
. Then it can be presented as
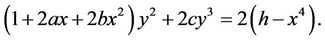 (2.4)
(2.4)
Assume that the positive solution of the above equation in y is
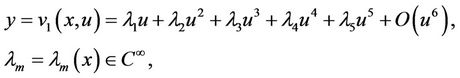 (2.5)
(2.5)
where 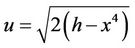 and
and . Then by (2.4) and (2.5) we obtain
. Then by (2.4) and (2.5) we obtain
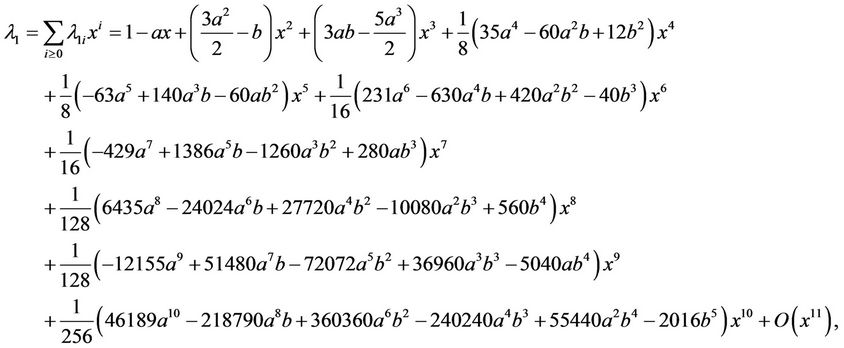
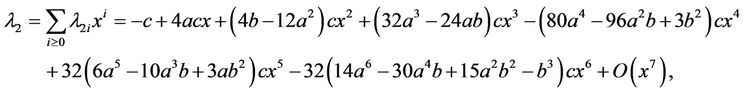



By [8] the negative solution of (2.4) in y satisfies . Thus, two solutions of (2.4) are
. Thus, two solutions of (2.4) are
 (2.6)
(2.6)
On the other hand, the intersection points of Lh and xaxis have the x-coordinates 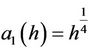 and
and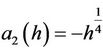 . Then by (2.2) we can write
. Then by (2.2) we can write
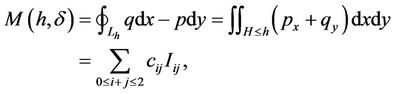 (2.7)
(2.7)
where
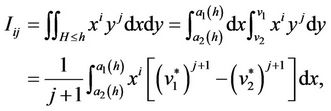 (2.8)
(2.8)
Here,

Introduce
 (2.9)
(2.9)
Then, similar to the method of Han [8] we have
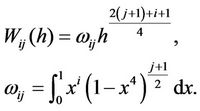 (2.10)
(2.10)
Therefore, in turn by (2.6)-(2.10) we have
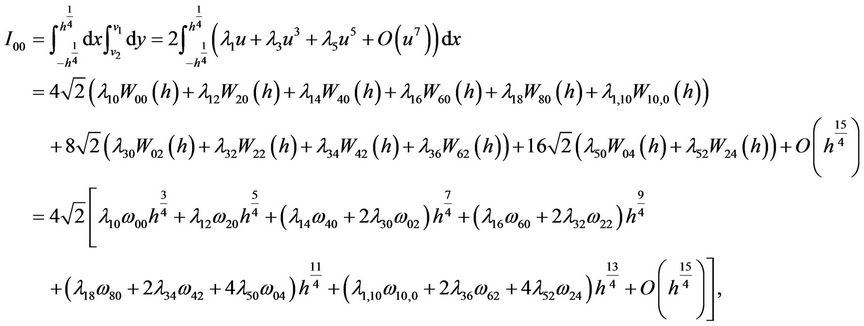
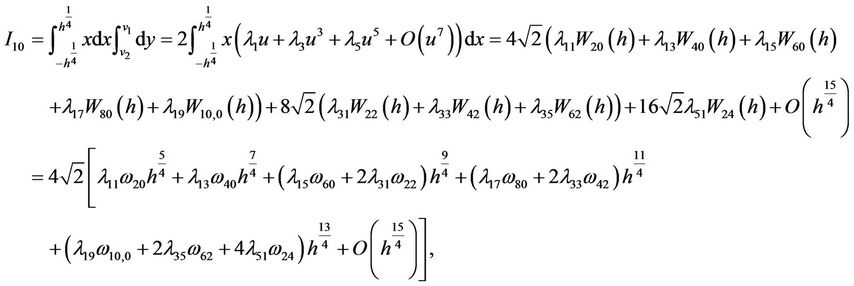
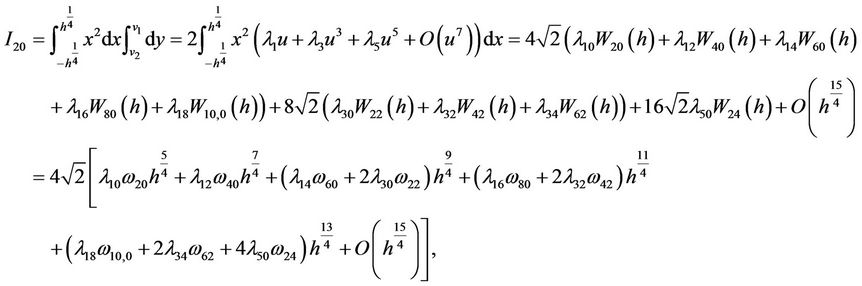
Noting that , then similarly we have
, then similarly we have
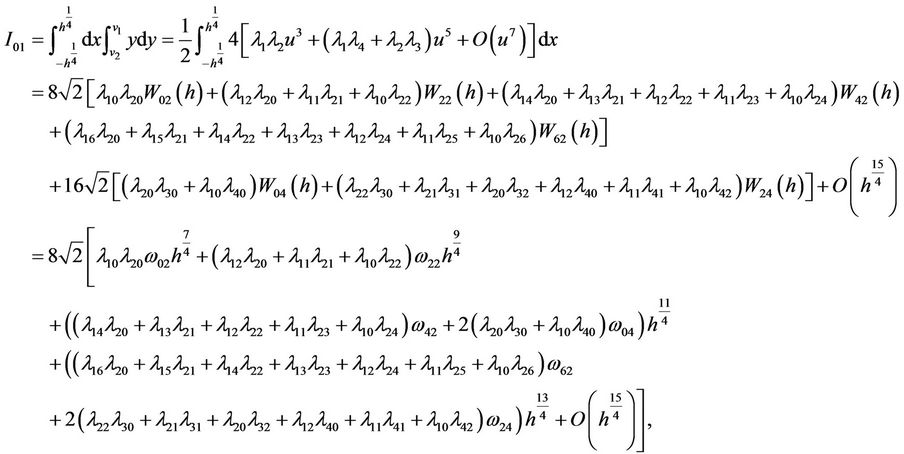

In the same way, using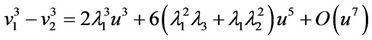 , we have
, we have

Hence, we have
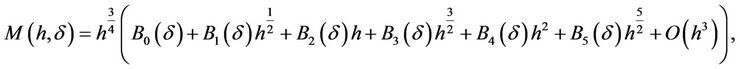
where  And
And
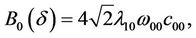

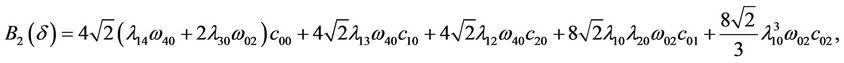

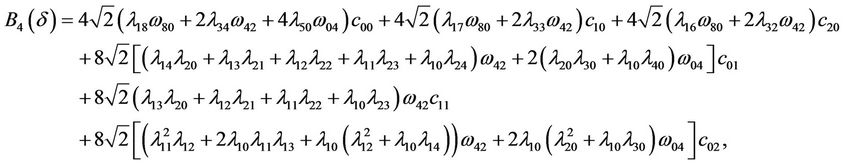

Now it is direct that


Here, if let ,
, 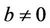 ,
, 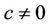 , then for some cubic system (2.1) we can obtain the above determinant is not zero, then the function M can have 5 simple zeros in h > 0 near h = 0 for some
, then for some cubic system (2.1) we can obtain the above determinant is not zero, then the function M can have 5 simple zeros in h > 0 near h = 0 for some 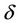 near
near . For example, let
. For example, let
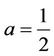 ,
, 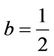 ,
,  , we obtain from the above formula
, we obtain from the above formula

Here,
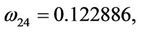
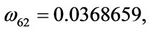
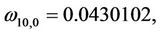




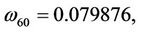


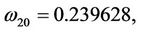
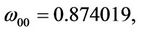
then we can obtain
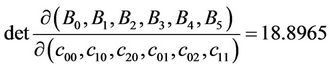 (2.11)
(2.11)
By Theorem 1.1 we have:
Theorem 2.1. The function 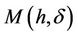 has at most 5 zeros in
has at most 5 zeros in 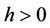 near
near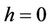 , and for
, and for 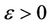 small the cubic system (2.1) can have 5 limit cycles near the origin.
small the cubic system (2.1) can have 5 limit cycles near the origin.
REFERENCES
- A. Andronov, E. Leontovich, I. Gordon and A. Maier, “Theory of Bifurcations of Dynamical Systems on a Plane,” Israel Program for Scientific Translations, Jerusalem, 1971.
- M. Han, “On Hopf Cyclicity of Planar Systems,” Journal of Mathematical Analysis and Applications, Vol. 245, No. 2, 2000, pp. 404-422. doi:10.1006/jmaa.2000.6758
- Y. A. Kuznetsov, “Elements of Applied Bifurcation Theory,” Springer-Verlag, New York, 1995.
- T. Carmon, R. Uzdin, C. Pigier, Z. Musslimani, M. Segev and A. Nepomnyashchy, “Rotating Propeller Solitons,” Physical Review Letters, Vol. 87, No. 14, 2001, p. 143901. doi:10.1103/PhysRevLett.87.143901
- J. Guckenheimer and P. Holmes, “Non-Linear Oscillations, Dynamical Systems and Bifurcation of Vector Fields,” Springer-Verlag, New York, 1983.
- B. D. Hassard, N. D. Kazarinoff and Y. H. Wan, “Theory and Applications of Hopf Bifurcation,” Cambridge University Press, Cambridge, 1981.
- S. Wiggins, “Global Bifurcations and Chaos: Analytical Methods,” Springer-Verlag, New York, 1988.
- M. Han J. Jiang and H. Zhu, “Limit Cycle Bifurcations in Near-Hamiltonian Systems by Perturbing a Nilpotent Center,” International Journal of Bifurcation and Chaos, Vol. 18, No. 10, 2008, pp. 3013-3027. doi:10.1142/S0218127408022226
NOTES
*The research was partially supported by National Natural Science Foundation of China (71101088).

