Applied Mathematics
Vol.07 No.15(2016), Article ID:71003,14 pages
10.4236/am.2016.715154
A Remark on Polynomial Mappings from 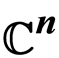 to
to 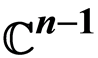 and an Application of the Software Maple in Research
and an Application of the Software Maple in Research
Thi Bich Thuy Nguyen
UNESP, Universidade Estadual Paulista, “Júlio de Mesquita Filho”, São José do Rio Preto, Brasil

Copyright © 2016 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: July 16, 2016; Accepted: September 26, 2016; Published: September 29, 2016
ABSTRACT
In [1] , we construct singular varieties 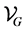 associated to a polynomial mapping
associated to a polynomial mapping 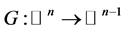 where
where 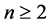 such that if G is a local submersion but is not a fibration, then the 2-dimensional homology and intersection homology (with total perversity) of the variety
such that if G is a local submersion but is not a fibration, then the 2-dimensional homology and intersection homology (with total perversity) of the variety 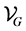 are not trivial. In [2] , the authors prove that if there exists a so-called very good projection with respect to the regular value
are not trivial. In [2] , the authors prove that if there exists a so-called very good projection with respect to the regular value 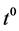 of a polynomial mapping
of a polynomial mapping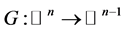 , then this value is an atypical value of G if and only if the Euler characteristic of the fibers is not constant. This paper provides relations of the results obtained in the articles [1] and [2] . Moreover, we provide some examples to illustrate these relations, using the software Maple to complete the calculations of the examples. We provide some discussions on these relations. This paper is an example for graduate students to apply a software that they study in the graduate program in advanced researches.
, then this value is an atypical value of G if and only if the Euler characteristic of the fibers is not constant. This paper provides relations of the results obtained in the articles [1] and [2] . Moreover, we provide some examples to illustrate these relations, using the software Maple to complete the calculations of the examples. We provide some discussions on these relations. This paper is an example for graduate students to apply a software that they study in the graduate program in advanced researches.
Keywords:
Singularities, Intersection Homology, Polynomial Mappings, Bifurcation

1. Preliminaries
1.1. Intersection Homology
We briefly recall the definition of intersection homology; for details, we refer to the fundamental work of M. Goresky and R. MacPherson [3] (see also [4] ).
Definition 1.1. Let X be an m-dimensional variety. A stratification of X is the data of a finite filtration
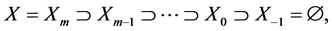
such that for every i, the set 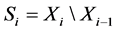 is either an empty set or a manifold of dimension i. A connected component of
is either an empty set or a manifold of dimension i. A connected component of 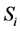 is called a stratum of X.
is called a stratum of X.
We denote by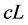 , the open cone on the space L, the cone on the empty set being a point. Observe that if L is a stratified set then
, the open cone on the space L, the cone on the empty set being a point. Observe that if L is a stratified set then 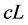 is stratified by the cones over the strata of L and an additional 0-dimensional stratum (the vertex of the cone).
is stratified by the cones over the strata of L and an additional 0-dimensional stratum (the vertex of the cone).
Definition 1.2. A stratification of X is said to be locally topologically trivial if for every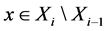 ,
, 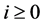 , there is an open neighborhood
, there is an open neighborhood  of x in X, a stratified set L and a homeomorphism
of x in X, a stratified set L and a homeomorphism
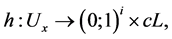
such that h maps the strata of 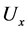 (induced stratification) onto the strata of
(induced stratification) onto the strata of ![]() (product stratification).
(product stratification).
The definition of perversities has originally been given by Goresky and MacPherson:
Definition 1.3. A perversity is an ![]() -uple of integers
-uple of integers ![]() such that
such that ![]() and
and![]() , for
, for![]() .
.
Traditionally we denote the zero perversity by![]() , the maximal perversity by
, the maximal perversity by![]() , and the middle perversities by
, and the middle perversities by
![]() (lower middle) and
(lower middle) and ![]() (upper middle). We say that the perversities
(upper middle). We say that the perversities ![]() and
and ![]() are complementary if
are complementary if![]() .
.
Let X be a variety such that X admits a locally topologically trivial stratification. We say that an i-dimensional subset ![]() is
is ![]() -allowable if
-allowable if
![]()
Define ![]() to be the
to be the ![]() -vector subspace of
-vector subspace of ![]() consisting in the chains
consisting in the chains ![]() such that
such that ![]() is
is ![]() -allowable and
-allowable and ![]() is
is ![]() -allowable.
-allowable.
Definition 1.4. The ![]() intersection homology group with perversity
intersection homology group with perversity![]() , denoted by
, denoted by![]() , is the
, is the ![]() homology group of the chain complex
homology group of the chain complex![]() .
.
The notation ![]() will refer to the intersection homology with compact supports, and the notation
will refer to the intersection homology with compact supports, and the notation ![]() will refer to the intersection homology with closed supports. In the compact case, they coincide and will be denoted by
will refer to the intersection homology with closed supports. In the compact case, they coincide and will be denoted by![]() . In general, when we write
. In general, when we write ![]() (resp.,
(resp.,![]() ), we mean the homology (resp., the intersection homology) with both compact supports and closed supports.
), we mean the homology (resp., the intersection homology) with both compact supports and closed supports.
Goresky and MacPherson proved that the intersection homology is independent on the choice of the stratification satisfying the locally topologically trivial conditions.
The Poincaré duality holds for the intersection homology of a (singular) variety:
Theorem 1.5. (Goresky, MacPherson [3] ) For any orientable compact stratified semi-algebraic m-dimensional variety X, the generalized Poincaré duality holds:
![]()
where ![]() and
and ![]() are complementary perversities.
are complementary perversities.
For the non-compact case, we have:
![]()
1.2. The Bifurcation Set, the Set of Asymptotic Critical Values and the Asymptotic Set
Let ![]() where
where ![]() be a polynomial mapping.
be a polynomial mapping.
i) The bifurcation set of G, denoted by ![]() is the smallest set in
is the smallest set in ![]() such that G is not
such that G is not ![]() -fibration on this set (see, for example, [5] ).
-fibration on this set (see, for example, [5] ).
ii) When![]() , we denote by
, we denote by ![]() the set of points at which the mapping G is not proper, i.e.
the set of points at which the mapping G is not proper, i.e.
![]()
and call it the asymptotic variety (see [6] ). The following holds: ![]() ( [6] ).
( [6] ).
2. Varieties ![]() Associated to a Polynomial Mapping
Associated to a Polynomial Mapping ![]()
In [1] , we construct singular varieties associated to a polynomial mapping ![]() as follows: let
as follows: let ![]() such that
such that![]() , where
, where ![]() is the set of critical values of G. Let
is the set of critical values of G. Let ![]() be a real function such that
be a real function such that
![]()
where![]() ,
, ![]() and
and![]() . Let us denote
. Let us denote ![]() and consider
and consider ![]() as a real mapping from
as a real mapping from ![]() to
to![]() . Let us define
. Let us define
![]()
where ![]() is the (real) Jacobian matrix of
is the (real) Jacobian matrix of ![]() at x. Notice that
at x. Notice that![]() , so we have
, so we have![]() .
.
Proposition 2.1. [1] For an open and dense set of polynomial mappings ![]() such that
such that![]() , the variety
, the variety ![]() is a smooth manifold of dimension
is a smooth manifold of dimension![]() .
.
Now, let us consider:
a) ![]() the restriction of G on
the restriction of G on![]() ,
,
b)![]() .
.
Since the dimension of ![]() is
is ![]() (Proposition 2.1), then locally, in a neighbourhood of any point
(Proposition 2.1), then locally, in a neighbourhood of any point ![]() in
in![]() , we get a mapping
, we get a mapping![]() . Then there exists a covering
. Then there exists a covering ![]() of
of ![]() by open semi-algebraic subsets (in
by open semi-algebraic subsets (in![]() ) such that on every element of this covering, the mapping F induces a diffeomorphism onto its image (see Lemma 2.1 of [7] ). We can find semi-algebraic closed subsets
) such that on every element of this covering, the mapping F induces a diffeomorphism onto its image (see Lemma 2.1 of [7] ). We can find semi-algebraic closed subsets ![]() (in
(in![]() ) which cover
) which cover ![]() as well. Thanks to Mostowski’s Separation Lemma (see Separation Lemma in [7] , p. 246), for each
as well. Thanks to Mostowski’s Separation Lemma (see Separation Lemma in [7] , p. 246), for each![]() , there exists a Nash function
, there exists a Nash function![]() , such that
, such that ![]() is positive on
is positive on ![]() and negative on
and negative on![]() . We can choose the Nash functions
. We can choose the Nash functions ![]() such that
such that ![]() tends to zero when
tends to zero when ![]() tends to infinity. Let the Nash functions
tends to infinity. Let the Nash functions ![]() and
and ![]() be such that
be such that ![]() tends to zero and
tends to zero and ![]() tends to infinity when
tends to infinity when ![]() tends to infinity. Define a variety
tends to infinity. Define a variety ![]() associated to
associated to ![]() as
as
![]()
that means ![]() is the closure of
is the closure of ![]() by
by![]() .
.
In order to understand better the construction of the variety![]() , see the example 4.13 in [1] .
, see the example 4.13 in [1] .
Proposition 2.2. [1] Let ![]() be a polynomial mapping such that
be a polynomial mapping such that ![]() and let
and let ![]() be a real function such that
be a real function such that
![]()
where![]() ,
, ![]() and
and ![]() for
for ![]() Then, there exists a real algebraic variety
Then, there exists a real algebraic variety ![]() in
in![]() , where
, where![]() , such that:
, such that:
1) The real dimension of ![]() is
is![]() ,
,
2) The singular set at infinity of the variety ![]() is contained in
is contained in ![]() where
where
![]()
3. The Bifurcation Set ![]() and the Homology, Intersection Homology of Varieties
and the Homology, Intersection Homology of Varieties ![]() Associated to a Polynomial Mapping
Associated to a Polynomial Mapping ![]()
We have the two following theorems dealing with the homology and intersection homology of the variety![]() .
.
Theorem 3.1. [1] Let ![]() be a polynomial mapping such that
be a polynomial mapping such that![]() . If
. If ![]() then
then
1) ![]()
2) ![]() where
where ![]() is the total perversity.
is the total perversity.
Theorem 3.2. [1] Let![]() , where
, where![]() , be a polynomial mapping such that
, be a polynomial mapping such that ![]() and
and![]() , where
, where ![]() is the leading form of
is the leading form of![]() , that is the homogenous part of highest degree of
, that is the homogenous part of highest degree of![]() , for
, for![]() . If
. If ![]() then
then
1) ![]()
2) ![]()
3) ![]() where
where ![]() is the total perversity.
is the total perversity.
Remark 3.3. The singular set at infinity of ![]() depends on the choice of the function
depends on the choice of the function![]() , since when
, since when ![]() changes, the set
changes, the set ![]() also changes. However, we have alway the property
also changes. However, we have alway the property ![]() (see [8] ).
(see [8] ).
Remark 3.4. The variety ![]() depends on the choice of the function
depends on the choice of the function ![]() and the functions
and the functions![]() , but the theorems 3.1 and 3.2 do not depend on the varieties
, but the theorems 3.1 and 3.2 do not depend on the varieties![]() . Form now, we denote by
. Form now, we denote by ![]() any variety
any variety ![]() associated to
associated to![]() . If we refer to
. If we refer to![]() , that means a variety
, that means a variety ![]() associated to
associated to ![]() for any
for any![]() .
.
4. The Bifurcation Set ![]() and the Euler Characteristic of the Fibers of a Polynomial Mapping
and the Euler Characteristic of the Fibers of a Polynomial Mapping ![]()
Let ![]() be a non-constant polynomial mapping and
be a non-constant polynomial mapping and ![]() be a regular value of G.
be a regular value of G.
Definition 4.1. [2] A linear function ![]() is said to be a very good projection with respect to the value
is said to be a very good projection with respect to the value ![]() if there exists a positive number
if there exists a positive number ![]() such that for all
such that for all![]() :
:
i) The restriction ![]() is proper,
is proper,
ii) The cardinal of ![]() does not depend on
does not depend on![]() , where
, where ![]() is a regular value of L.
is a regular value of L.
Theorem 4.2. [2] Let ![]() be a regular value of G. Assume that there exists a very good projection with respect to the value
be a regular value of G. Assume that there exists a very good projection with respect to the value![]() . Then,
. Then, ![]() is an atypical value of G if and only if the Euler characteristic of
is an atypical value of G if and only if the Euler characteristic of ![]() is bigger than that of the generic fiber.
is bigger than that of the generic fiber.
Theorem 4.3. [2] Assume that the zero set![]() , where
, where ![]() is the leading form of
is the leading form of![]() , has complex dimension one. Then any generic linear mapping L is a very good projection with respect to any regular value
, has complex dimension one. Then any generic linear mapping L is a very good projection with respect to any regular value ![]() of G.
of G.
5. Relations between [1] and [2]
Let ![]()
![]() be a polynomial mapping such that
be a polynomial mapping such that![]() . Then any
. Then any ![]() is a regular value of G. Let
is a regular value of G. Let ![]() be a real function such that
be a real function such that ![]() where
where![]() ,
, ![]() and
and ![]() for
for ![]() From theorems 3.1 and 4.2, we have the following corollary.
From theorems 3.1 and 4.2, we have the following corollary.
Corollary 5.1. Let ![]() be a polynomial mapping such that
be a polynomial mapping such that![]() . Assume that there exists a very good projection with respect to
. Assume that there exists a very good projection with respect to![]() . If the Euler characteristic of
. If the Euler characteristic of ![]() is bigger than that of the generic fiber, then
is bigger than that of the generic fiber, then
1) ![]() for any
for any![]() ,
,
2) ![]() for any
for any![]() , where
, where ![]() is the total perversity.
is the total perversity.
Proof. Let ![]() be a polynomial mapping such that
be a polynomial mapping such that![]() . Then every point
. Then every point ![]() is a regular point of G. Assume that there exists a very good projection with respect to
is a regular point of G. Assume that there exists a very good projection with respect to![]() . If the Euler characteristic of
. If the Euler characteristic of ![]() is bigger than that of the generic fiber, then by the theorem 4.2, the bifurcation set
is bigger than that of the generic fiber, then by the theorem 4.2, the bifurcation set ![]() is not empty. Then by the theorem 3.1, we have
is not empty. Then by the theorem 3.1, we have ![]() for any
for any ![]() and
and ![]() for any
for any![]() , where
, where ![]() is the total perversity. +
is the total perversity. +
From theorems 3.2 and 4.2, we have the following corollary.
Corollary 5.2. Let![]() , where
, where![]() , be a polynomial mapping such that
, be a polynomial mapping such that ![]() and
and![]() , where
, where ![]() is the leading form of
is the leading form of![]() . Assume that there exists a very good projection with respect to
. Assume that there exists a very good projection with respect to![]() . If the Euler characteristic of
. If the Euler characteristic of ![]() is bigger than that of the generic fiber, then
is bigger than that of the generic fiber, then
1) ![]() for any
for any![]() ,
,
2)![]() , for any
, for any![]() ,
,
3) ![]() for any
for any![]() , where
, where ![]() is the total perversity.
is the total perversity.
Proof. Let![]() , where
, where![]() , be a polynomial mapping such that
, be a polynomial mapping such that![]() . Then every point
. Then every point ![]() is a regular point of G. Assume that there exists a very good projection with respect to
is a regular point of G. Assume that there exists a very good projection with respect to![]() . By the theorem 4.2, the bifurcation set
. By the theorem 4.2, the bifurcation set ![]() is not empty. If
is not empty. If![]() , then by the theorem 3.2, we have
, then by the theorem 3.2, we have
1) ![]() for any
for any![]() ,
,
2)![]() , for any
, for any![]() ,
,
3) ![]() for any
for any![]() , where
, where ![]() is the total perversity. +
is the total perversity. +
We have also the following corollary.
Corollary 5.3. Let![]() , where
, where![]() , be a polynomial mapping such that
, be a polynomial mapping such that![]() . Assume that the zero set
. Assume that the zero set ![]() has complex dimension one, where
has complex dimension one, where ![]() is the leading form of
is the leading form of![]() . If the Euler characteristic of
. If the Euler characteristic of ![]() is bigger than that of the generic fiber, where
is bigger than that of the generic fiber, where![]() , then
, then
1) ![]() for any
for any![]() ,
,
2)![]() , for any
, for any![]() ,
,
3) ![]() for any
for any![]() , where
, where ![]() is the total perversity.
is the total perversity.
Proof. At first, since the zero set ![]() has complex dimension one, then by the theorem 4.3, any generic linear mapping L is a very good projection with respect to any regular value
has complex dimension one, then by the theorem 4.3, any generic linear mapping L is a very good projection with respect to any regular value ![]() of G. Moreover, the complex dimension of the set
of G. Moreover, the complex dimension of the set ![]() is the complex corank of
is the complex corank of ![]() . Then
. Then![]() . By the corollary 5.2, we get the proof of the corollary 5.3. +
. By the corollary 5.2, we get the proof of the corollary 5.3. +
Remark 5.4. We can construct the variety![]() , where L is a very good projection defined in 4.2 as the following: Let
, where L is a very good projection defined in 4.2 as the following: Let![]() , where
, where![]() , be a polynomial mapping such that
, be a polynomial mapping such that![]() . Assume that there exists a very good projection
. Assume that there exists a very good projection ![]() with respect to
with respect to![]() . Then L is a linear function. Assume that
. Then L is a linear function. Assume that![]() . Then the variety
. Then the variety ![]() is defined as the variety
is defined as the variety![]() , where
, where
![]()
with![]() ,
, ![]() are the modules of the complex numbers
are the modules of the complex numbers ![]() and
and![]() , respectively. With this variety
, respectively. With this variety![]() , all the results in the corollaries 5.1, 5.2 and 5.3 hold. Moreover, the varieties
, all the results in the corollaries 5.1, 5.2 and 5.3 hold. Moreover, the varieties ![]() make the corollaries 5.1, 5.2 and 5.3 simpler.
make the corollaries 5.1, 5.2 and 5.3 simpler.
Remark 5.5. In the construction of the variety ![]() [1] (see section 2), if we replace F by the restriction of
[1] (see section 2), if we replace F by the restriction of ![]() to
to![]() , that means
, that means
![]()
then we have the same results than in [1] . In fact, in this case, since the dimension of ![]() is
is![]() , then locally, in a neighbourhood of any point
, then locally, in a neighbourhood of any point ![]() in
in![]() , we get a mapping
, we get a mapping![]() . There exists also a covering
. There exists also a covering ![]() of
of ![]() by open semi-algebraic subsets (in
by open semi-algebraic subsets (in![]() ) such that on every element of this covering, the mapping F induces a diffeomorphism onto its image. We can find semi-algebraic closed subsets
) such that on every element of this covering, the mapping F induces a diffeomorphism onto its image. We can find semi-algebraic closed subsets ![]() (in
(in![]() ) which cover
) which cover ![]() as well. Thanks to Mostowski’s Separation Lemma, for each
as well. Thanks to Mostowski’s Separation Lemma, for each![]() , there exists a Nash function
, there exists a Nash function![]() , such that
, such that ![]() is positive on
is positive on ![]() and negative on
and negative on![]() . Let the Nash functions
. Let the Nash functions ![]() and
and
![]() be such that
be such that ![]() and
and ![]() tend to zero where
tend to zero where ![]() is a sequence in
is a sequence in ![]() tending to infinity. Define a variety
tending to infinity. Define a variety ![]() associated to
associated to ![]() as
as
![]()
We get the ![]() -dimensional singular variety
-dimensional singular variety ![]() in
in![]() , the singular set at infinity of which is
, the singular set at infinity of which is![]() .
.
With this construction of the set![]() , the corollaries 5.1, 5.2 and 5.3 also hold.
, the corollaries 5.1, 5.2 and 5.3 also hold.
6. Some Discussions
A natural question is to know if the converses of the corollaries 5.1 and 5.2 hold. That means, let ![]()
![]() be a polynomial mapping such that
be a polynomial mapping such that ![]() then
then
Question 6.1. If there exists a very good projection with respect to ![]() and if either
and if either ![]() or
or![]() , then is the Euler characteristic of
, then is the Euler characteristic of ![]() bigger than the one of the generic fiber?
bigger than the one of the generic fiber?
By the theorem 4.2, the above question is equivalent to the following question:
Question 6.2. If ![]() then are
then are ![]() and
and![]() ?
?
This question is equivalent to the converse of the theorems 3.1 and 3.2. Note that by the proposition 2.2, the singular set at infinity of the variety ![]() is contained in
is contained in ![]() Moreover, in the proofs of the theorems 3.1 and 3.2, we see that the characteristics of the homology and intersection homology of the variety
Moreover, in the proofs of the theorems 3.1 and 3.2, we see that the characteristics of the homology and intersection homology of the variety ![]() depend on the set
depend on the set![]() . In [1] , we provided an example to show that the answer to the question 6.2 is negative. In fact, let
. In [1] , we provided an example to show that the answer to the question 6.2 is negative. In fact, let
![]()
then ![]() and
and ![]() if we choose the function
if we choose the function ![]() then
then ![]() and
and![]() ; if we choose the function
; if we choose the function ![]() then
then ![]() and
and ![]() Then, we suggest the two following conjectures.
Then, we suggest the two following conjectures.
Conjecture 6.3. Does there exist a function ![]() such that if
such that if ![]() then
then![]() ?
?
Conjecture 6.4. Let ![]()
![]() be a polynomial mapping such that
be a polynomial mapping such that![]() . Assume that there exists a very good projection with respect to
. Assume that there exists a very good projection with respect to![]() . If the Euler characteristic of
. If the Euler characteristic of ![]() is constant, for any
is constant, for any![]() , then there exists a real positive function
, then there exists a real positive function ![]() such that
such that ![]() and
and![]() .
.
Remark 6.5. The construction of the variety ![]() in [1] (see section 2) can be applied for any polynomial mapping
in [1] (see section 2) can be applied for any polynomial mapping![]() , where
, where![]() , such that
, such that![]() . In fact, if G is generic then similarly to the proposition 2.1, the variety
. In fact, if G is generic then similarly to the proposition 2.1, the variety
![]()
has the real dimension 2 m. Hence, if we consider![]() , that means F is the restriction of G to
, that means F is the restriction of G to![]() , then locally we get a real mapping
, then locally we get a real mapping![]() . Moreover, in this case, we also have
. Moreover, in this case, we also have ![]() for any
for any ![]() (see [8] ), where
(see [8] ), where
![]()
So, we can use the same arguments in [1] , and we have the following results.
Proposition 6.6. Let ![]() be a polynomial mapping, where
be a polynomial mapping, where![]() , such that
, such that![]() . Let
. Let ![]() be a real function such that
be a real function such that
![]()
where![]() ,
, ![]() and
and ![]() for
for ![]() Then, there exists a real variety
Then, there exists a real variety ![]() in
in![]() , where
, where![]() , such that:
, such that:
1) The real dimension of ![]() is 2 m,
is 2 m,
2) The singular set at infinity of the variety ![]() is contained in
is contained in ![]()
Similarly to [1] , we have the two following theorems (see theorems 3.1 and 3.2).
Theorem 6.7. Let![]() , where
, where![]() , be a polynomial mapping such that
, be a polynomial mapping such that![]() . If
. If ![]() then
then
1) ![]()
2) ![]() where
where ![]() is the total perversity.
is the total perversity.
Theorem 6.8. Let![]() , where
, where![]() , be a polynomial mapping such that
, be a polynomial mapping such that![]() . Assume that
. Assume that![]() , where
, where ![]() is the leading form of
is the leading form of![]() . If
. If ![]() then
then
1) ![]() for any
for any![]() ,
,
2)![]() , for any
, for any![]() ,
,
3) ![]() for any
for any![]() , where
, where ![]() is the total perversity.
is the total perversity.
7. Examples
Example 7.1. We give here an example to illustrate the calculations of the set ![]() in the case of a polynomial mapping
in the case of a polynomial mapping ![]() where
where![]() ,
, ![]() and there exists a very good projection with respect to any point of
and there exists a very good projection with respect to any point of![]() . In general, the calculations of the set
. In general, the calculations of the set ![]() are enough complicate, but the software Maple may support us. That is what we do in this example.
are enough complicate, but the software Maple may support us. That is what we do in this example.
Let us consider the Broughton’s example [9] :
![]()
We have ![]() and
and![]() . In fact, since the system of equations
. In fact, since the system of equations ![]() has no solutions, then
has no solutions, then![]() . Moreover,
. Moreover,
![]()
and for any![]() , we have
, we have
![]()
So ![]() is not homeomorphic to
is not homeomorphic to ![]() for any
for any![]() . Hence
. Hence![]() . We determine now all the possible very good projections of G with respect to
. We determine now all the possible very good projections of G with respect to![]() . In fact, for any
. In fact, for any ![]() and for any
and for any![]() , we have
, we have
![]()
Assume that ![]() is a sequence in
is a sequence in ![]() tending to infinity. If
tending to infinity. If ![]() tends to infinity then
tends to infinity then ![]() tends to zero. If
tends to zero. If ![]() tends to infinity then
tends to infinity then ![]() tends to zero. If L is a very good projection with respect to
tends to zero. If L is a very good projection with respect to ![]() then, by definition, the restriction
then, by definition, the restriction ![]() is proper. Then
is proper. Then![]() , where
, where ![]() and
and![]() . We check now the cardinal
. We check now the cardinal ![]() of
of ![]() where
where ![]() is a regular value of L. Let us replace
is a regular value of L. Let us replace
![]() in the equation
in the equation![]() , we have the following equation
, we have the following equation
![]()
where![]() . This equation always has three (complex) solutions. Thus, the number
. This equation always has three (complex) solutions. Thus, the number ![]() does not depend on
does not depend on![]() . Hence, any linear function of the form
. Hence, any linear function of the form![]() , where
, where ![]() and
and![]() , is a very good projection of G with respect to
, is a very good projection of G with respect to![]() . It is easy to see that the set of very good projections of G with respect to
. It is easy to see that the set of very good projections of G with respect to ![]() is dense in the set of linear functions.
is dense in the set of linear functions.
We choose ![]() and we compute the variety
and we compute the variety ![]() associated to
associated to ![]() where
where![]() . Let us denote
. Let us denote
![]()
where![]() . Consider G as a real polynomial mapping, we have
. Consider G as a real polynomial mapping, we have
![]()
and
![]()
The set ![]() is the set of the solutions of the determinant of the minors
is the set of the solutions of the determinant of the minors ![]() of the matrix
of the matrix
![]()
Using Maple, we:
A) Calculate the determinants of the minors ![]() of the matrix
of the matrix![]() :
:
1) Calculate the determinant of the minor defined by the columns 1, 2 and 3:
![]()
2) Calculate the determinant of the minor defined by the columns 1, 2 and 4:
![]()
3) Calculate the determinant of the minor defined by the columns 1, 3 and 4:
![]()
4) Calculate the determinant of the minor defined by the columns 2, 3 and 4:
![]()
B) Solve now the system of equations of the above determinants:
![]()
We conclude that ![]() where
where
![]()
C) In order to calculate![]() , we have to calculate and draw
, we have to calculate and draw![]() , for
, for ![]() .
.
1) Calculate and draw![]() :
:
![]()
2) Calculate and draw![]() :
:
![]()
+ Calculate and draw![]() :
:
![]()
Since ![]() is the closure of
is the closure of ![]() then
then ![]() is connected and has a pure dimension, then
is connected and has a pure dimension, then ![]() is a cone:
is a cone:
![]()
Example 7.2. If we take the suspension of the Broughton’s example
![]()
then, similarly to the example 7.1, the variety ![]() is a cone as in the example 7.1 but it has dimension 4, in the space
is a cone as in the example 7.1 but it has dimension 4, in the space![]() . We can check easily that the intersection homology in dimension 2 of the variety
. We can check easily that the intersection homology in dimension 2 of the variety ![]() of this example is non-trivial. We get an example to illustrate the corollary 5.1.
of this example is non-trivial. We get an example to illustrate the corollary 5.1.
Example 7.3. If we take the Broughton example for ![]() such that
such that ![]() then similarly to the example 7.1, we get an example of varieties
then similarly to the example 7.1, we get an example of varieties ![]() for the case
for the case ![]() where
where![]() . This example illustrates the remark 6.5.
. This example illustrates the remark 6.5.
Cite this paper
Nguyen, T.B.T. (2016) A Remark on Polynomial Mappings from
to
and an Application of the Software Maple in Research. Applied Mathematics, 7, 1868-1881. http://dx.doi.org/10.4236/am.2016.715154
References
- 1. Nguyen, T.B.T. and Ruas, M.A.S. (2015) On a Singular Variety Associated to a Polynomial Mapping. ArXiv:1503.08079.
- 2. Ha, V.H. and Nguyen, T.T. (2011) On the Topology of Polynomial Mappings from Cn to Cn-1. International Journal of Mathematics, 22, 435-448.
http://dx.doi.org/10.1142/S0129167X11006842
- 3. Goresky, M. and MacPherson, R. (1980) Intersection Homology Theory. Topology, 19, 135-162.
http://dx.doi.org/10.1016/0040-9383(80)90003-8
- 4. Brasselet, J.-P. (2015) Introduction to Intersection Homology and Perverse Sheaves. Instituto de Matemática Pura e Aplicada, Rio de Janeiro.
- 5. Kurdyka, K., Orro, P. and Simon, S. (2000) Semialgebraic Sard Theorem for Generalized Critical Values. Journal of Differential Geometry, 56, 67-92.
- 6. Jelonek, Z. (1993) The Set of Point at Which Polynomial Map Is Not Proper. Annales Polonici Mathematici, 58, 259-266.
- 7. Mostowski, T. (1976) Some Properties of the Ring of Nash Functions. Annali della Scuola normale superiore di Pisa, 2, 245-266.
- 8. Dias, L.R.G., Ruas, M.A.S. and Tibar, M. (2012) Regularity at Infinity of Real Mappings and a Morse-Sard Theorem. Journal of Topology, 5, 323-340.
http://dx.doi.org/10.1112/jtopol/jts005
- 9. Broughton, A. (1981) On the Topology of Polynomial Hypersurfaces. Proc. Sympos. Pure Math., Vol. 40, 167-178.
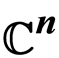 to
to 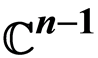 and an Application of the Software Maple in Research
and an Application of the Software Maple in Research



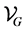 associated to a polynomial mapping
associated to a polynomial mapping 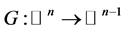 where
where 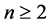 such that if G is a local submersion but is not a fibration, then the 2-dimensional homology and intersection homology (with total perversity) of the variety
such that if G is a local submersion but is not a fibration, then the 2-dimensional homology and intersection homology (with total perversity) of the variety 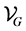 are not trivial. In [2] , the authors prove that if there exists a so-called very good projection with respect to the regular value
are not trivial. In [2] , the authors prove that if there exists a so-called very good projection with respect to the regular value 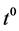 of a polynomial mapping
of a polynomial mapping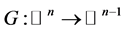 , then this value is an atypical value of G if and only if the Euler characteristic of the fibers is not constant. This paper provides relations of the results obtained in the articles [1] and [2] . Moreover, we provide some examples to illustrate these relations, using the software Maple to complete the calculations of the examples. We provide some discussions on these relations. This paper is an example for graduate students to apply a software that they study in the graduate program in advanced researches.
, then this value is an atypical value of G if and only if the Euler characteristic of the fibers is not constant. This paper provides relations of the results obtained in the articles [1] and [2] . Moreover, we provide some examples to illustrate these relations, using the software Maple to complete the calculations of the examples. We provide some discussions on these relations. This paper is an example for graduate students to apply a software that they study in the graduate program in advanced researches.
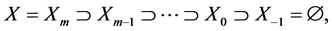
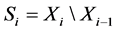 is either an empty set or a manifold of dimension i. A connected component of
is either an empty set or a manifold of dimension i. A connected component of 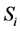 is called a stratum of X.
is called a stratum of X.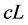 , the open cone on the space L, the cone on the empty set being a point. Observe that if L is a stratified set then
, the open cone on the space L, the cone on the empty set being a point. Observe that if L is a stratified set then 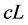 is stratified by the cones over the strata of L and an additional 0-dimensional stratum (the vertex of the cone).
is stratified by the cones over the strata of L and an additional 0-dimensional stratum (the vertex of the cone).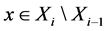 ,
, 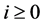 , there is an open neighborhood
, there is an open neighborhood  of x in X, a stratified set L and a homeomorphism
of x in X, a stratified set L and a homeomorphism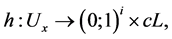
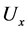 (induced stratification) onto the strata of
(induced stratification) onto the strata of 

































































































































































































































































































































































































































































































