ABSTRACT

By using Fubini theorem or Tonelli theorem, we find that the zeta function value at 2 is equal to a special integral. Furthermore, we find that this special integral is two times of another special integral. By using this fact we give an easy way to calculate the value of the alternating sum of without using the Fourier expansion. Also, we discuss the relationship between Genocchi numbers and Bernoulli numbers and get some results about Bernoulli polynomials.

Keywords:Basel Problem, Zeta Function, Bernoulli Numbers, Bernoulli Polynomials

1. Introduction

Basel problem asks for the precise value of the progression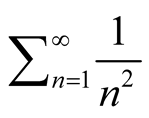. It was first posed by Pietro Mengoli in 1644 and solved by Leonhard Euler in 1735 [1] . The value is known as.

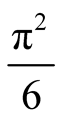There are more general results [2] about the progression,

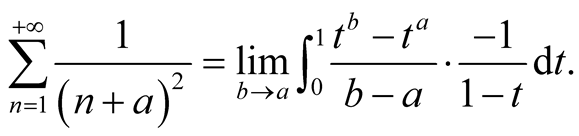
Let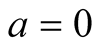, it becomes the.

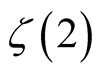Moreover, using Fourier expansion of,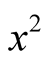

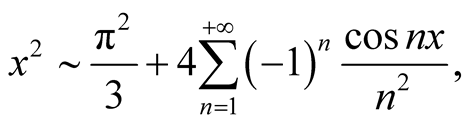
we will get

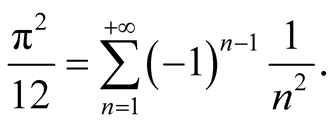(1.1)

In the end of Section 5, we give another proof of (1.1) by using the relationship of two special integrals which are introduced in Sections 3 and 4. Also, inspired by this, in Section 6, we discuss about Bernoulli numbers and Genocchi numbers. We obtain some properties of Bernoulli numbers and Bernoulli polynomials.

2. Basic Properties

The convergence of the infinite series 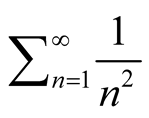is obvious. We can use various methods to prove it. Especially, when we consider Riemann-Zeta function,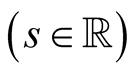 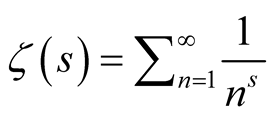, the progression diverges when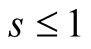and converges when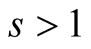. Also, we can use the estimate of the partial sum of the series.

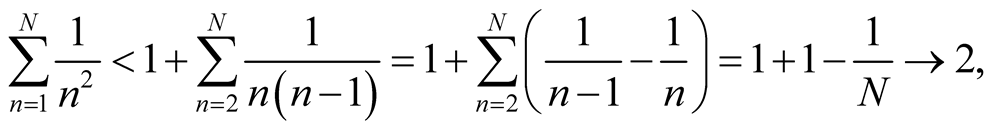
when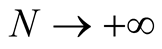. Or we can use the Cauchy principle. In fact, for,

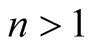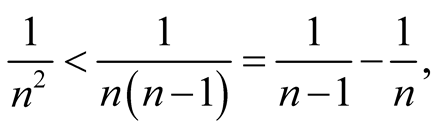
thus

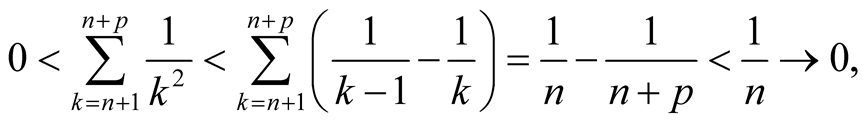
when. Then, the progression converges.

3. Calculation of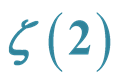

There are various proofs of the Basel problem and Robin Chapman wrote a survey [3] about these. Some are elementary and some will use advanced mathematics such as Fourier analysis, complex analysis or multivariable calculus. Here we review the method of Jiaqiang Mei [4] , which is rather elementary and easy to understand. There is also an elementary proof on the Wiki [1] .

Repeated use of the equation

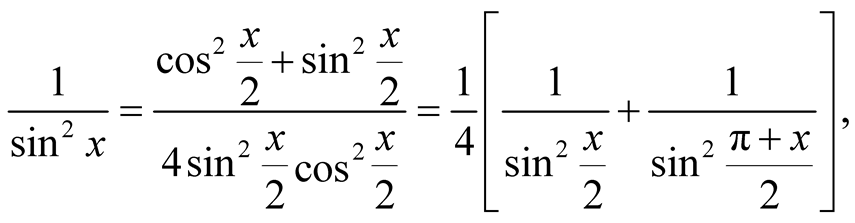
we get

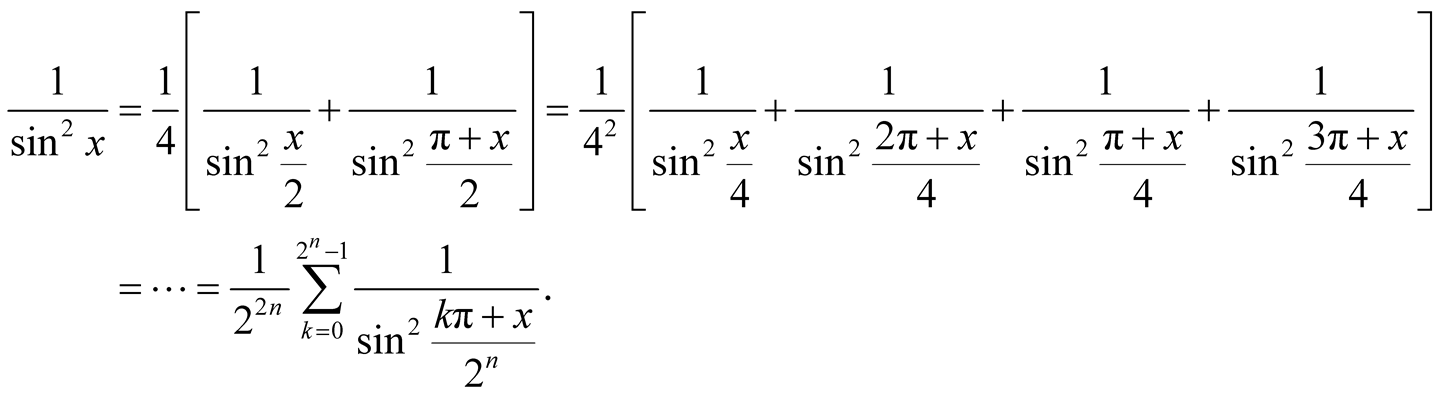(1.2)

Note that

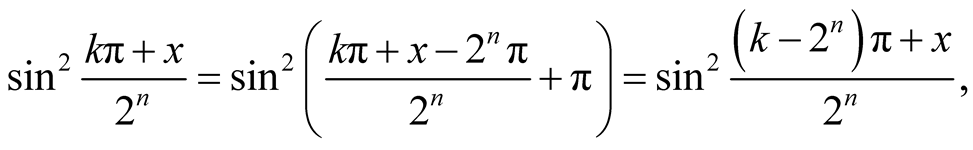
we may rewrite the Equation (1.2) as

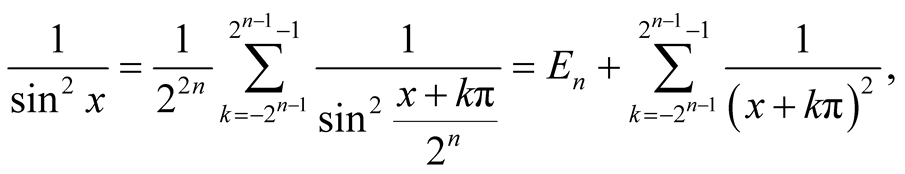
where

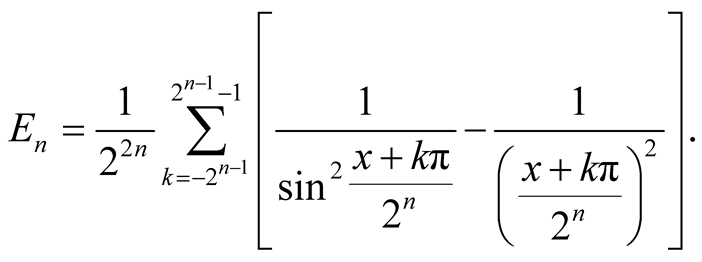
Using the inequality

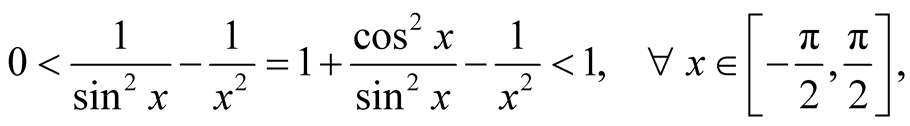
we get the estimation

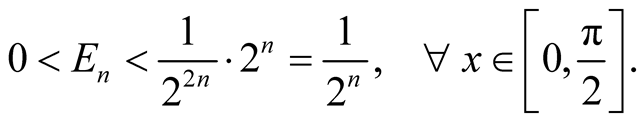
Let, we obtain the following equation

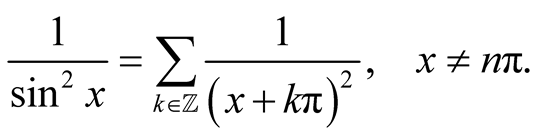
The above progression is uniformly convergent in any closed interval not containing 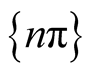and can be written as

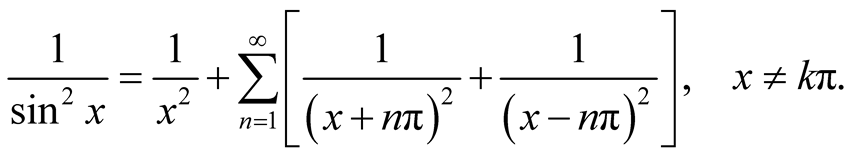
Especially, we have

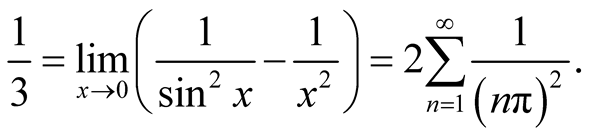
Therefore,

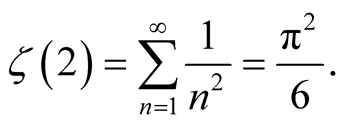
4. As a Special Case of Power Series

For the power series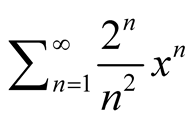, we calculate the domain of convergence. Since

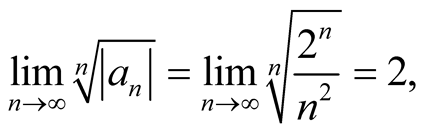
the radius of convergence equals. If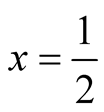, the power series becomes the progression 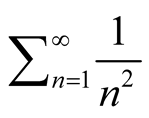which is convergent. If, then the power series becomes 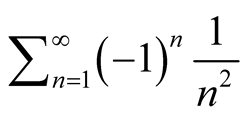which is also convergent. Therefore, the domain of convergence is.

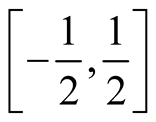Suppose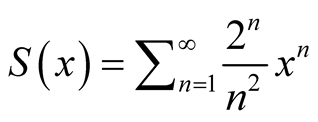. We can do the derivation item by item in the inteval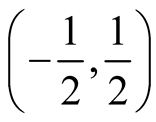. That is,

Multiply both sides by,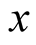

Derivate both sides again, we get

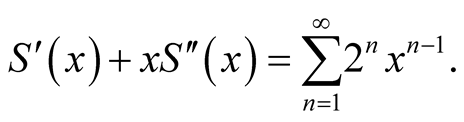
Thus,

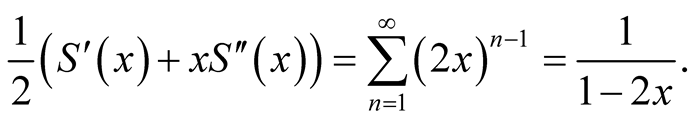
We obtain a second order ordinary differential equation

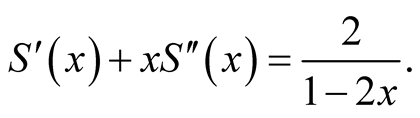(1.3)

If we set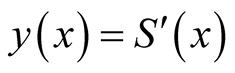, the Equation (1.3) is converted to a first order equation,

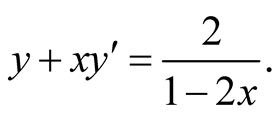
Multiplie both side by,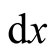

Let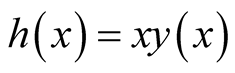, we have

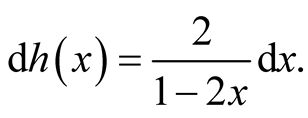
Then

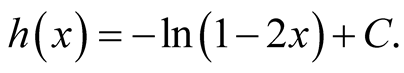
Using the initial conditions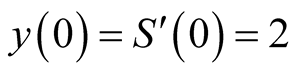, , 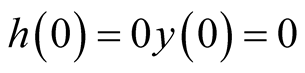we have

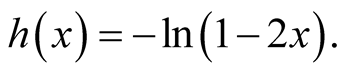
Then, if,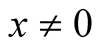

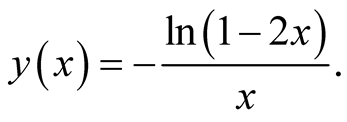
That is

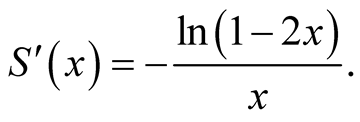
Note that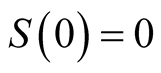. Then,

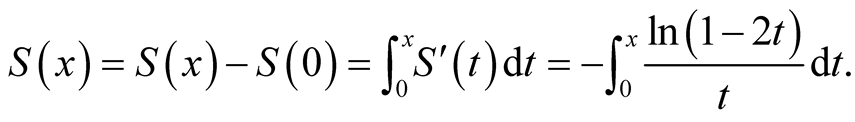
Particularly,

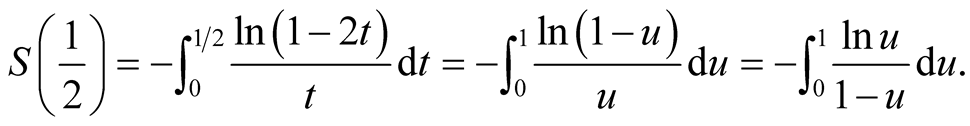
Therefore, for the improper integral, we know its value is equal to.

5. From the Special Integral to the Basel Problem

In this section, we will calculate the special integral arised in the last section, i.e.

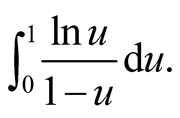
For,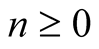

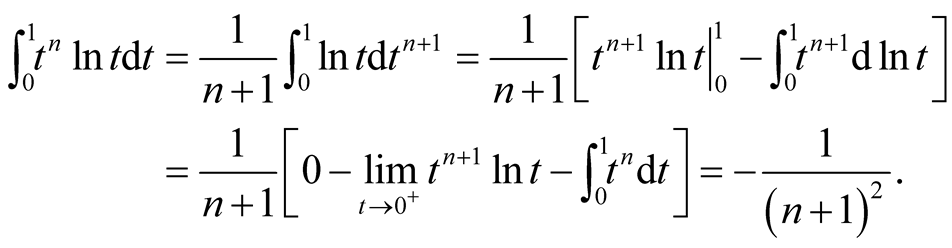
Thus

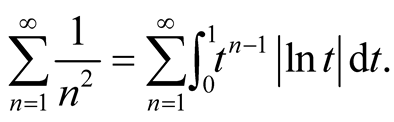
Let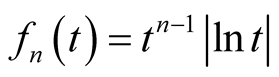, ,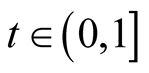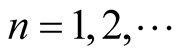. Obviously, 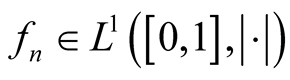, where 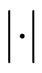is Lebesgue measure.

For simplicity, we denote 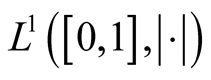by.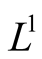

By Minkowski inequality [5] , we have

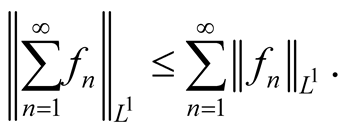(1.4)

Then,

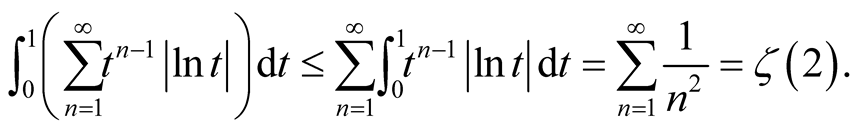
We will prove the equality holds in our case. First we have the following lemma.

Lemma 1. 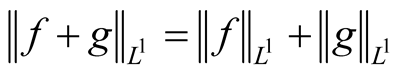if and only if there is a real valued function h that is nonnegative a.e. such that when both f and g are not 0 then 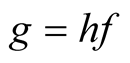a.e.

Proof. Please refer to [6] .

Lemma 1 can be generalized to infinite summation case.

Lemma 2. 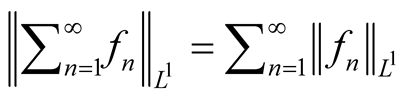if and only if there is a real valued function f and a series of real valued functions 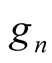which have the same signs such that 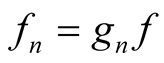a.e.

Proof. First, by induction, the lemma holds for finite sum. That is

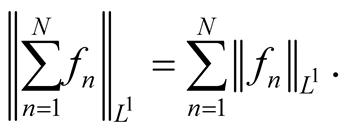
Let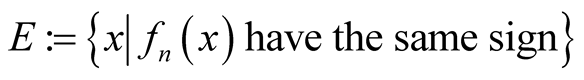. Then,

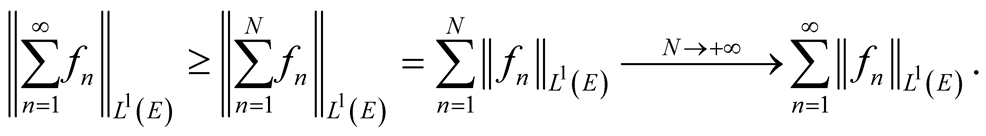
Since the measure of is zero, we have

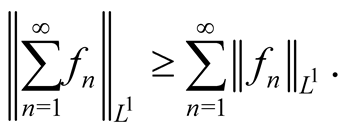
Combine Equation (1.4), we complete the proof.

On the other hand, we observe that, for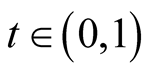

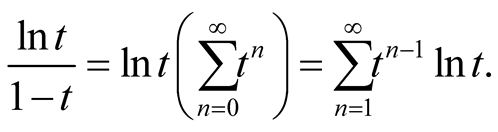
Then we get

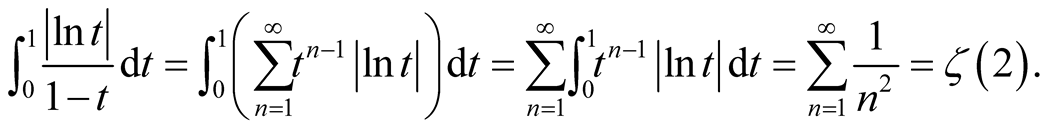
We give a remark about the second equality of the above equation. It can be infered by Fubini theorem or Tonelli’s theorem [7] .

Infact,

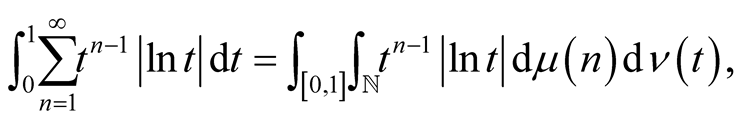
where 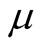is the counting measure on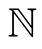, and 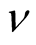is the Lebesgue measure on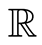. Obviously, they are both 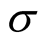-finite measures. And since 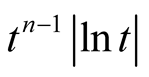is non-negative, by Tonelli’s theorem,

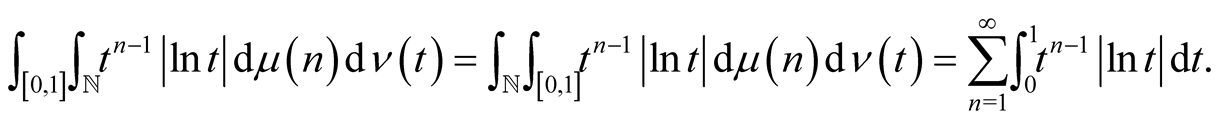
There are other ways to get this relationship from this special integral to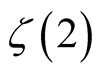. First, recall the lemma established by James P. Lesko and Wendy D. Smith [2] .

Lemma 3. For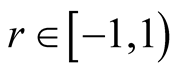, 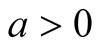and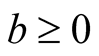, we have

(1.5)

Especially, when 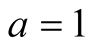and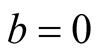, (1.5) yields

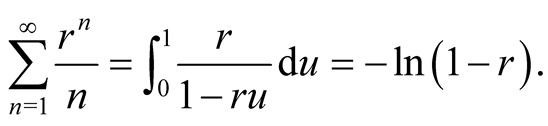
By this lemma,

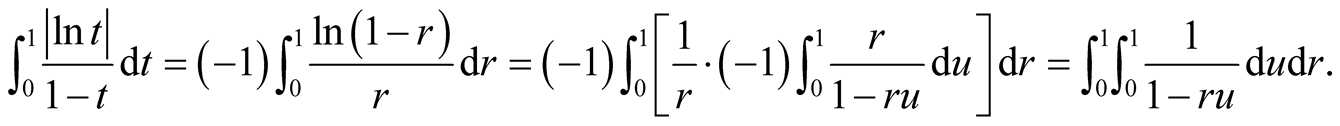
Then by monotone convergence theorem, it equals to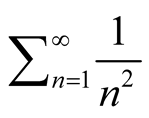. See [3] .

Or, we can do it in this way,

6. Relationship between Two Special Integrals

We will use a result from ([8] , Exer 20).

Lemma 4. Assume that the function 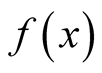is monotone on the interval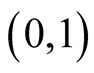. It need not be bounded at the points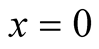,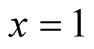; we assume however that the improper integral 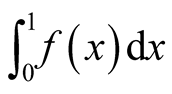exists. Under these conditions,

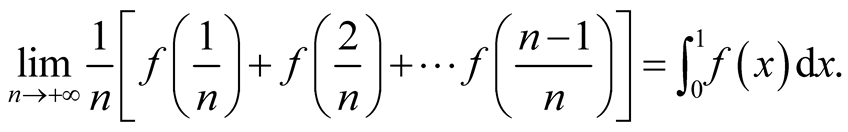
Then for our case, the integral 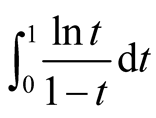exists and satisfies the conditions. In fact, let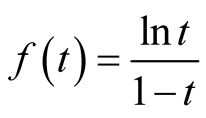, 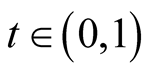then

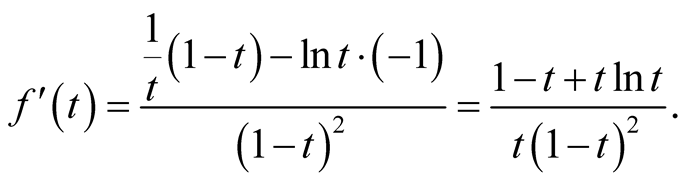
Let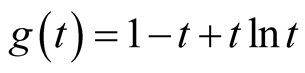, then

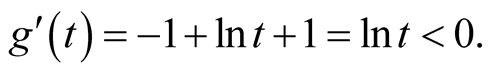
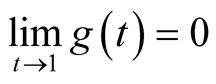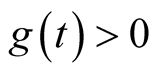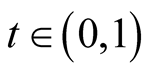Since, we have for. So 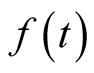is monotone on the interval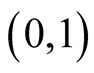. Then by Lemma 4, we have

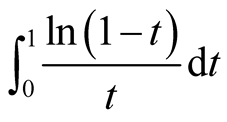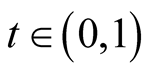If we consider the improper integral. Let,. Then

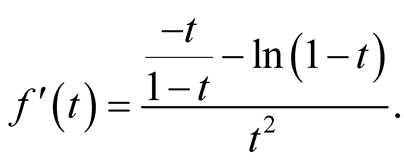
Let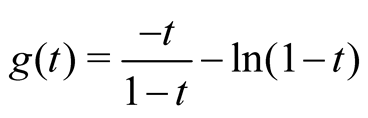, then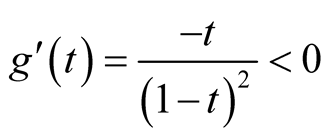. Since

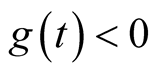. Thus,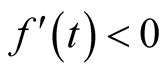. So 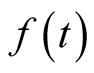is also monotone on the interval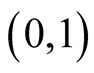. By Lemma 4, we have

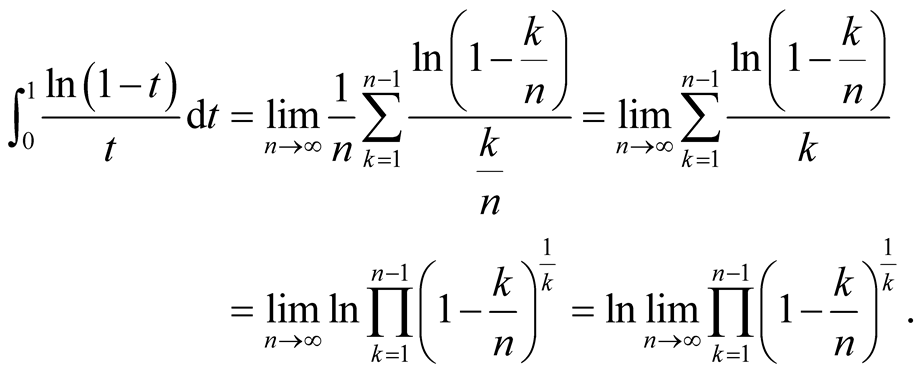
Next, we deduce the following equation and give another discription of (1.1),

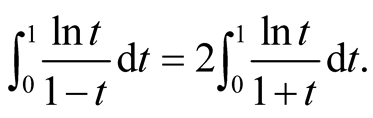(1.6)

Lemma 5. Let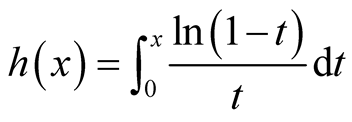, , 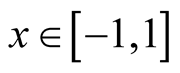then.

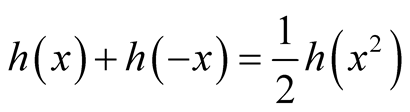Proof. Let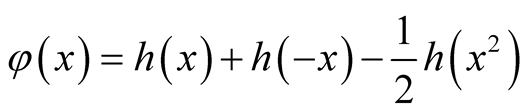, it is easy to note that its derivate 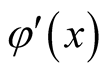equals to zero and

.

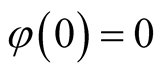By changing variables,

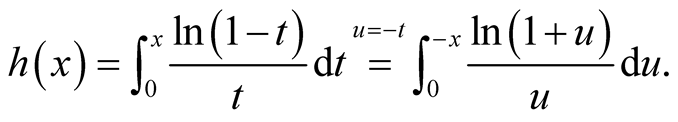
Then

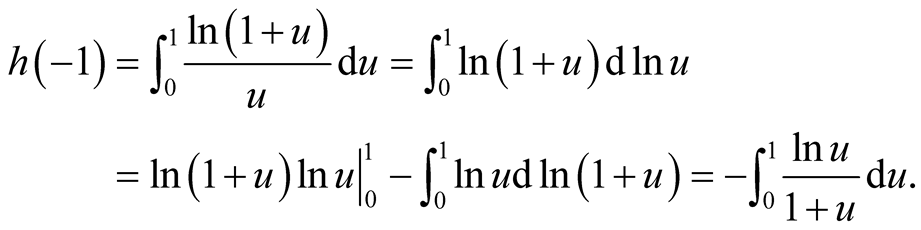
Here, note that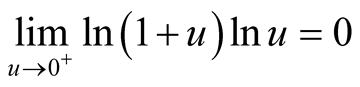. By Lemma 5,

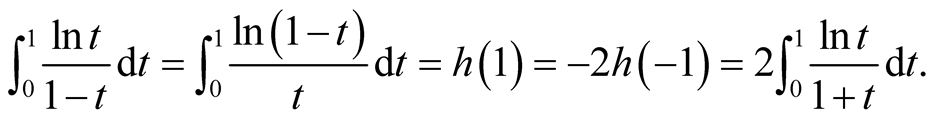
For the integral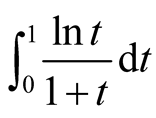, we have a relevant result.

Lemma 6. Let, , 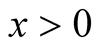then.

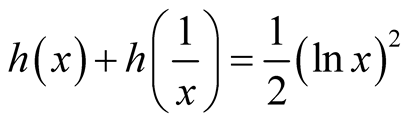Proof.

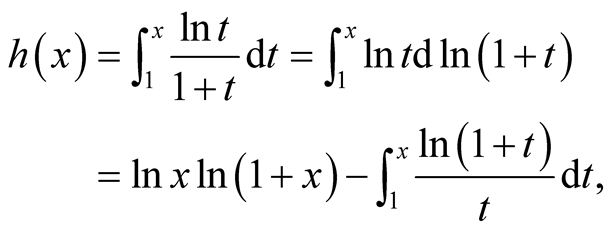
then,

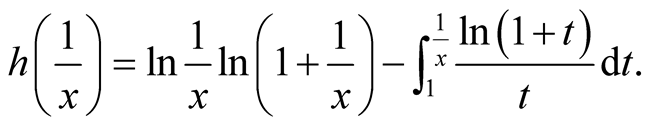
Hence,

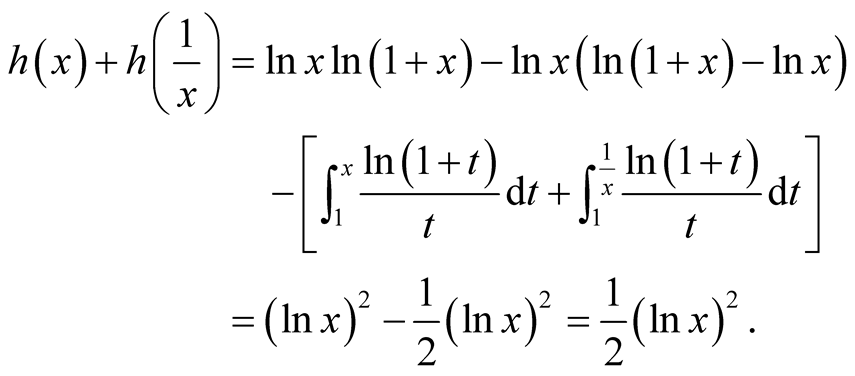
The second equality holds, because

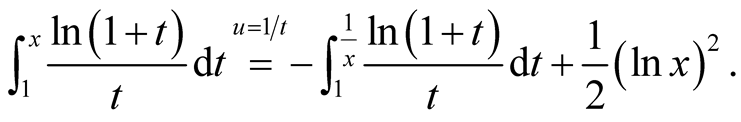
Observe that

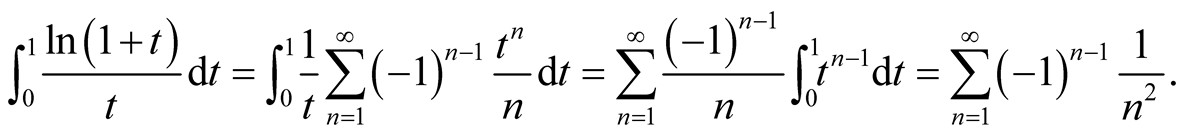
Thus

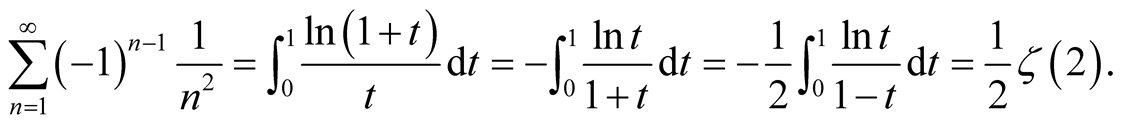
Applying the same argument in beginning of this section, we get

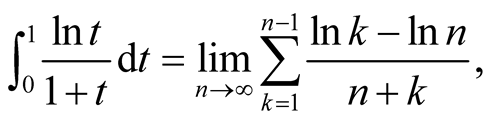
and

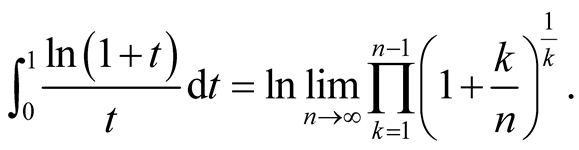
Remark. It must be very interesting if we could calculate the integral 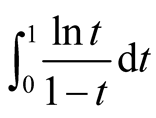not using the progression.

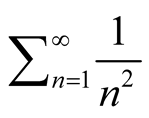Remark. Similarly, we can prove that.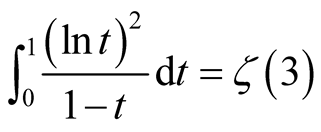

7. Bernoulli Numbers and Bernoulli Polynomials

Recall some facts of Bernoulli numbers, and for more information, please refer to [4] [9] -[12] .

The Bernoulli numbers 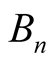are defined by the power series expansion

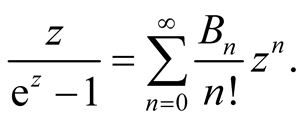
Then

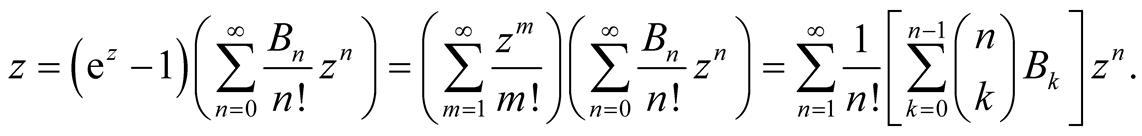
Thus we get a recursion formula for the Bernoulli numbers, namely

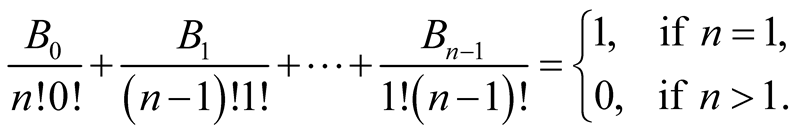
We get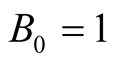. From the identity

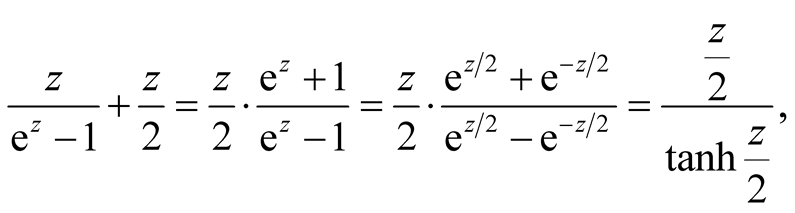
the function 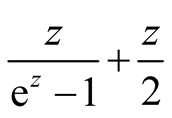is an even function. Hence it has only even terms in its power series expansion.

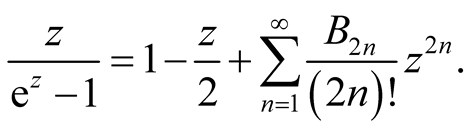
Sometimes, people prefer to use 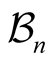to denote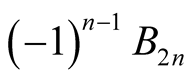, then

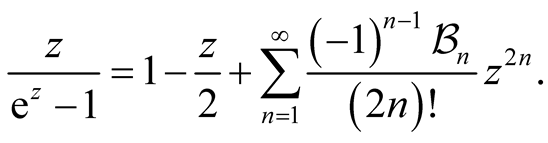
We have various ways to get the important equation.

Lemma 7.

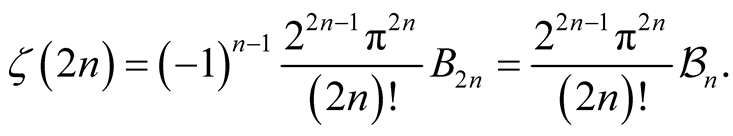
Proof. By replacing 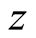by 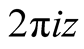in the identity

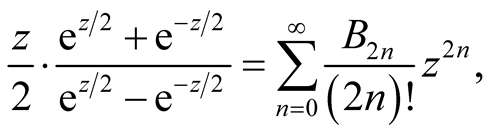
we get

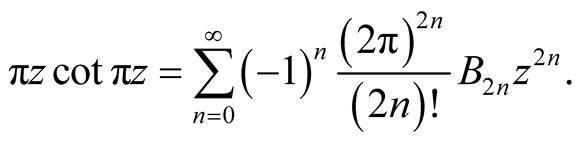
Then by taking the logarithmic derivative of the product expansion for the sine,

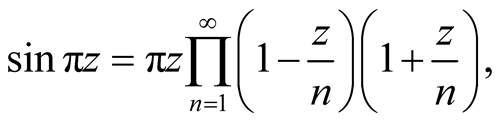
we get the expansion of.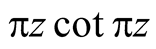

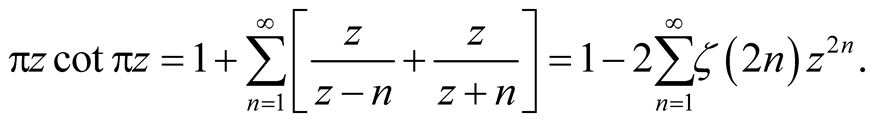
Comparing the coefficents of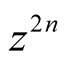, we get (7).

Another way is to take the logarithmic derivative of the identity [4]

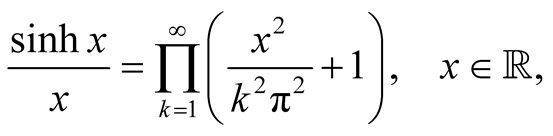
which yields

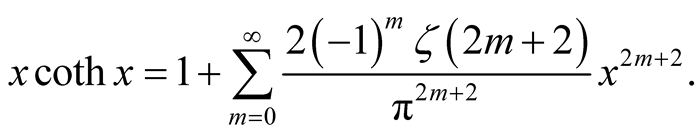
Then we get (7) by comparing with

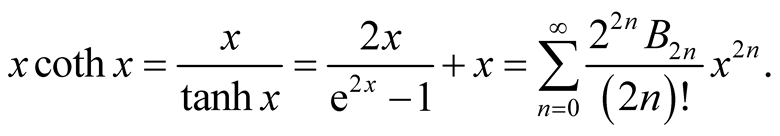
Proposition 1.

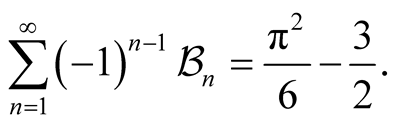
Proof.

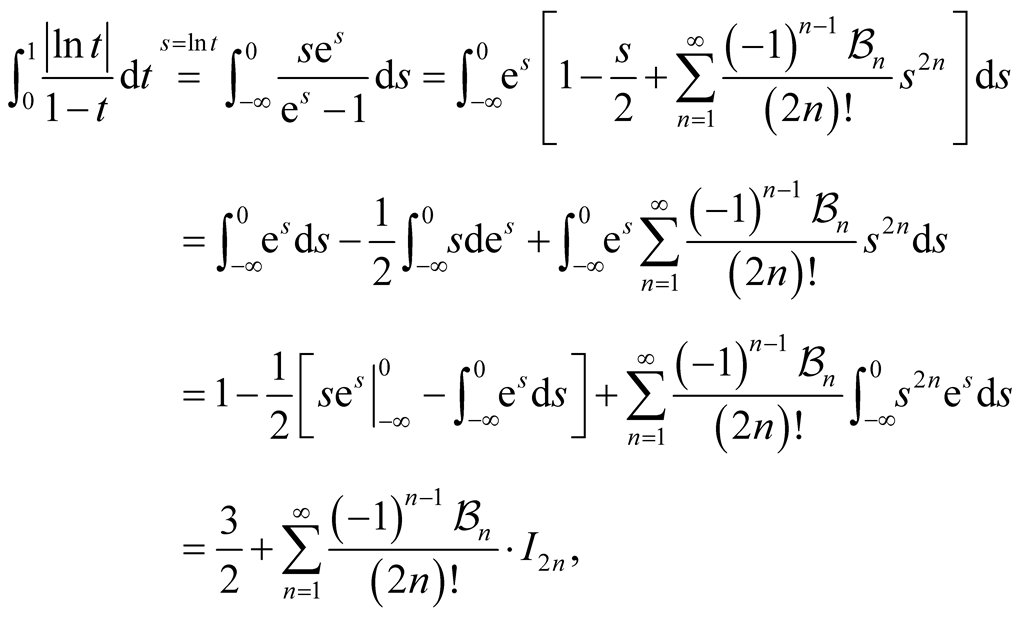
where

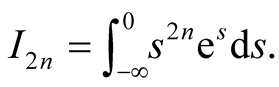
It is easy to prove that 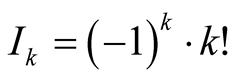by induction. Therefore,

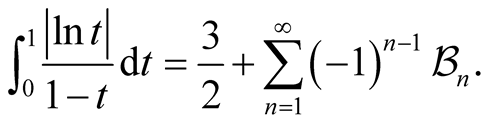
Let us consider the expansion

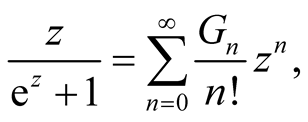
where are Genocchi numbers. Then

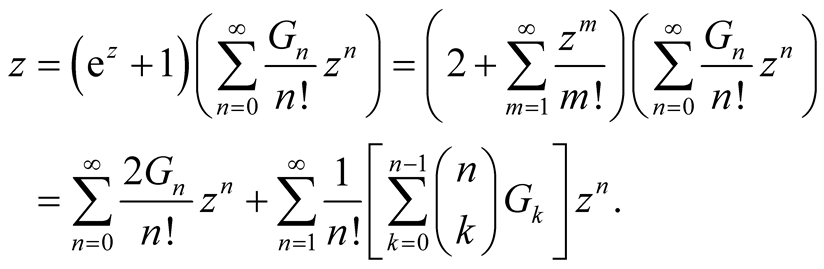
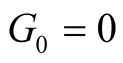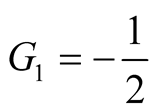Thus, and, which infers that. For,

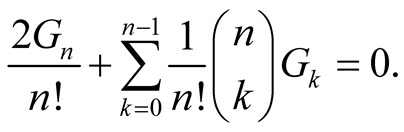
That is,

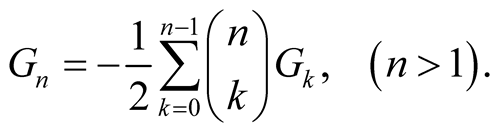(1.7)

Note that

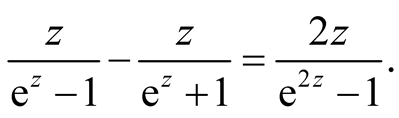
Taking expansion,

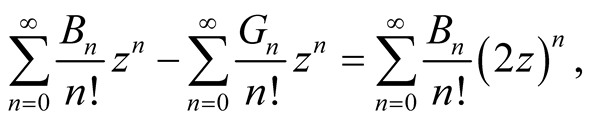
we have

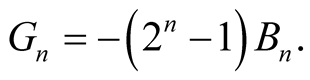(1.8)

This give a quick way to compute Bernoulli numbers since in (1.7) we have.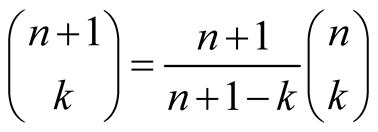

If let 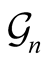denote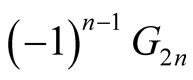, then

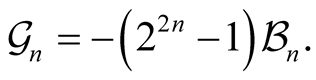
Proposition 2.

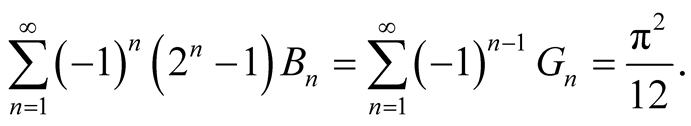
Proof. By changing variables,

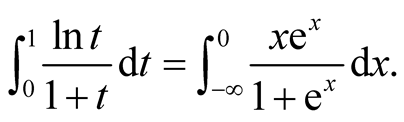
Note that

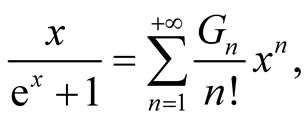
then

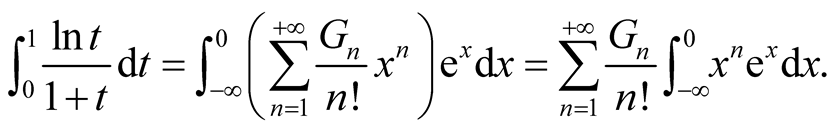
Set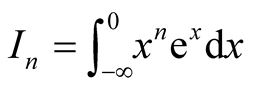, then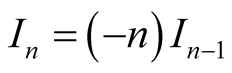. By induction, we prove that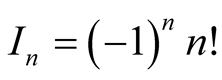. Therefore,

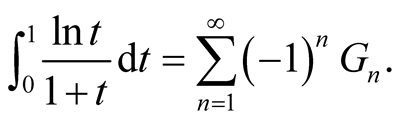
Together with (1.6), we have

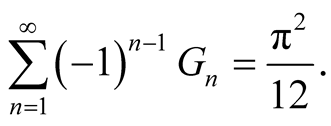
Remark. Since 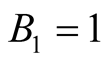and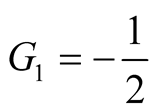, the proposition can also be written as

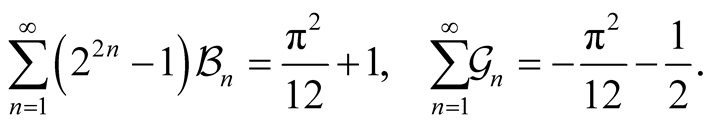
Bernoulli polynomials 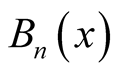are defined by the formula

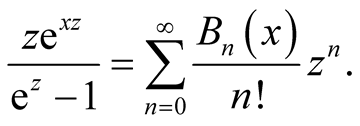
The functions 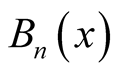are polynomials in 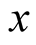and

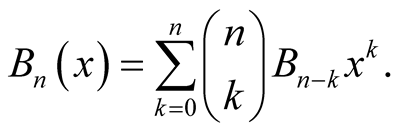
Similarly, we define 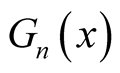by the formula

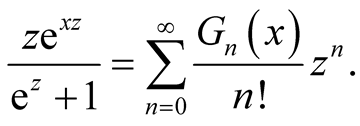(1.9)

The functions 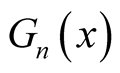are polynomials in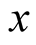. In fact,

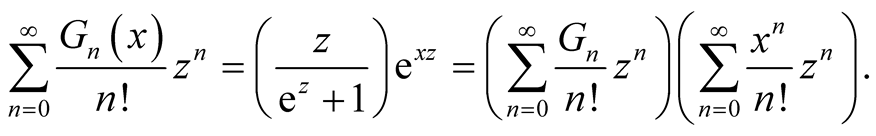
Comparing the coefficients of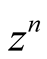, we have

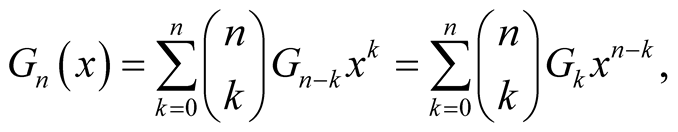(1.10)

and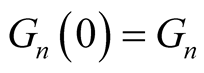. Using (1.7), for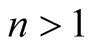, we have

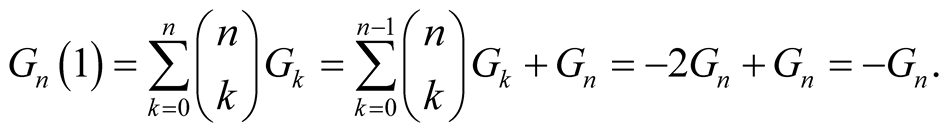
On the other hand, by definition,

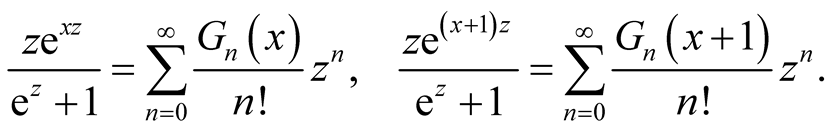
Do the addition,

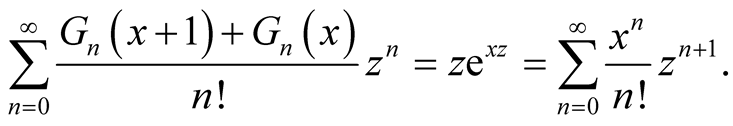
Comparing the coefficients of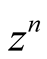, we have

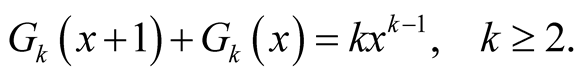
Let 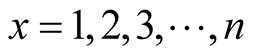and summation these equations, we get

From the equation, we infer that.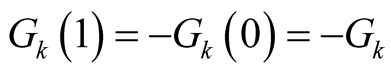

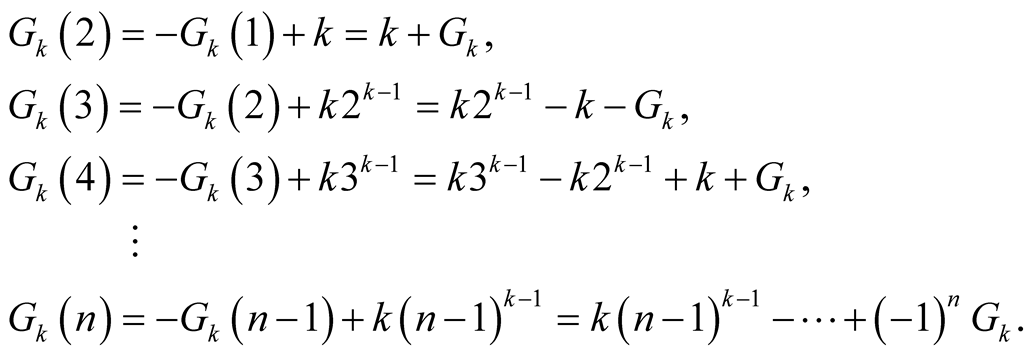
Therefore,

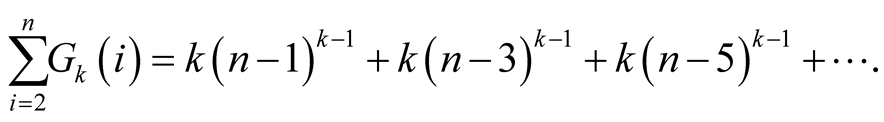
If,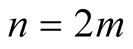

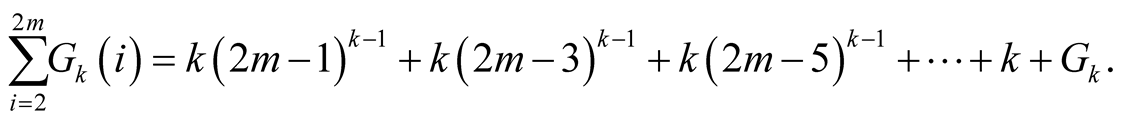
If,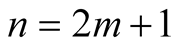

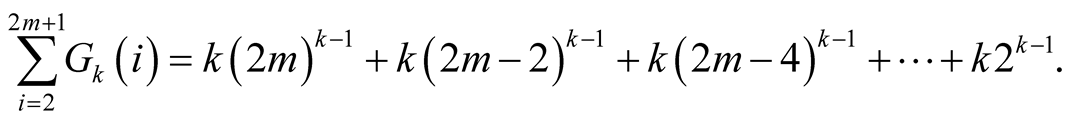
Whether is odd or even, we always have the following trivial identity.

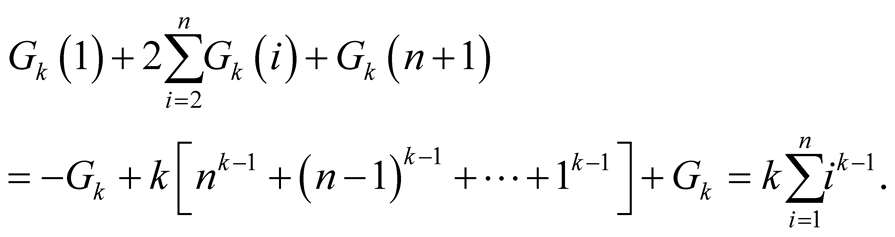
By differentiating on 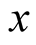at both sides of (1.9), we also have

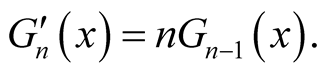
But being different from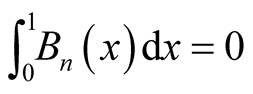, we have

Proposition 3. i).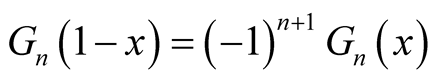

ii).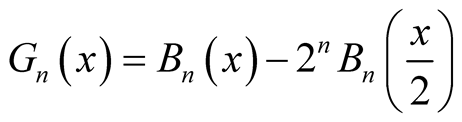

iii).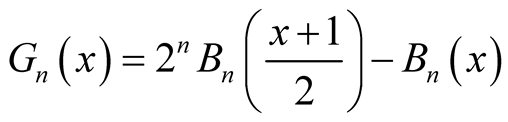

iv).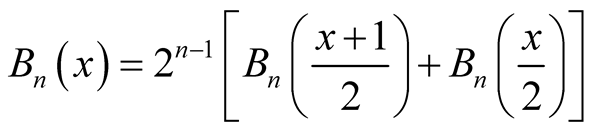

Proof. i)

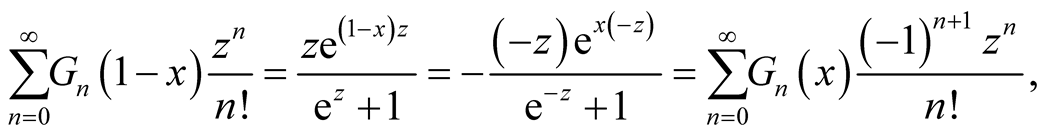
thus,

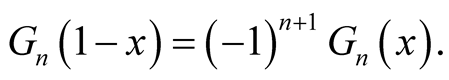
ii)

By comparing the coeffients of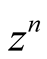, we get

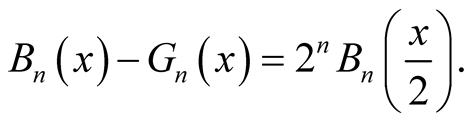
iii)

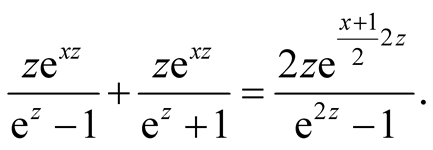
By comparing the coeffients of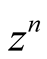, we get

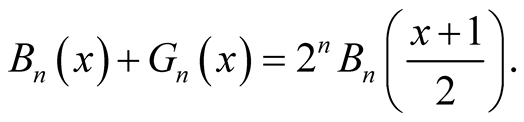
iv) by ii) and iii).

Remark. 1) Especially, we have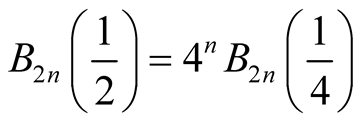, since.

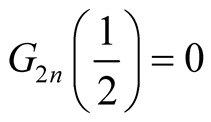2) Let 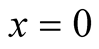or 1 in iv), we have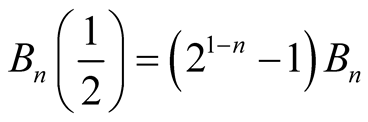. Thus,.

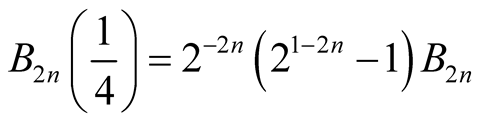3) Let 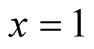in iii), we will get.

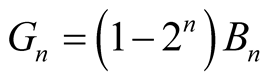Equation (1.8) can also be deduced in the following way. Using

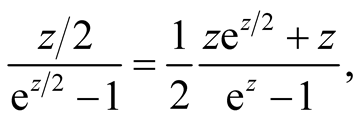
we obtain

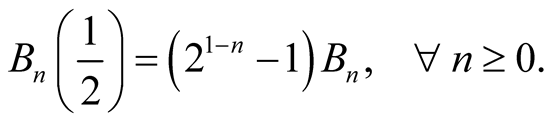(1.11)

Similarly,

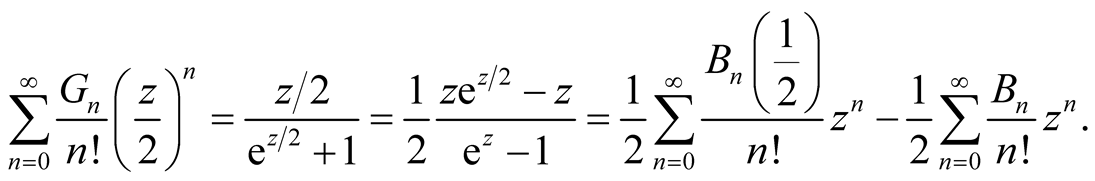
This infers that

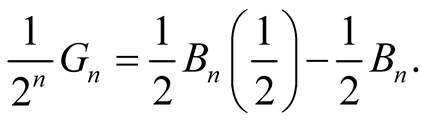
By substituting (1.11) in the above formula, we obtainde

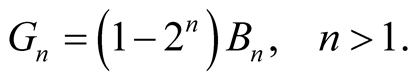
Acknowledgements

We express our gratitude to David Harvey who point out that the numbers 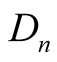in our manuscript (here is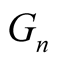) are essentially the Genocchi numbers, see [13] .

The work is partially supported by National Natural Science Foundation of China (NSFC), Tianyuan fund for Mathematics, No. 11126046, and the University Science Research Project of Jiangsu Province (13KJB110029).

References