International Journal of Astronomy and Astrophysics
Vol.06 No.01(2016), Article ID:65213,13 pages
10.4236/ijaa.2016.61010
A New Solution for the Friedmann Equations
Naser Mostaghel
Department of Civil Engineering, University of Toledo, Toledo, USA

Copyright © 2016 by author and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 30 November 2015; accepted 27 March 2016; published 30 March 2016
ABSTRACT
Assuming a flat universe expanding under a constant pressure and combining the first and the second Friedmann equations, a new equation, describing the evolution of the scale factor, is derived. The equation is a general kinematic equation. It includes all the ingredients composing the universe. An exact closed form solution for this equation is presented. The solution shows remarkable agreement with available observational data for redshifts from a low of z = 0.0152 to as high as z = 8.68. As such, this solution provides an alternative way of describing the expansion of space without involving the controversial dark energy.
Keywords:
Cosmological Constant, Distances and Redshifts, Expanding Universe, Friedmann Equations

1. Introduction
The evolution of the universe has already been investigated through the first Friedmann equation. As discussed by Carrol [1] , the first Friedmann equation is used because it only involves the first derivative of the scale factor. However, the resulting models such as ΛCDM-based models are in terms of limited numbers of parameters representing the ingredients of the universe. Two analytical solutions with restrictive assumptions are already available [2] [3] , which will be presented in Section three. There exists no general analytical solution for the Friedmann equations. Here, through combining the first and the second Friedmann equations, a general equation is formulated. Assuming a flat universe, an exact closed form solution for this general equation is obtained. This solution is remarkably consistent with the observational data over a wide range of measured redshifts from a low of z = 0.0152 to the highest recently measured value of z = 8.68.
Except for the flatness assumption, the analytical solution is completely general. It includes all the ingredients forming the universe. The ΛCDM-based models only consider specific combinations of limited numbers of ingredients. Also the value of the cosmological density parameter is analytically estimated to be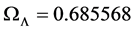 . This is essentially identical to the
. This is essentially identical to the  value estimated in 2014 by the Plank Collaboration [4] , based on combined data from “Plank + WP + high L + BAO”. It is also shown that the contribution of the cosmological constant is to cancel the pressure term in the Friedmann acceleration equation. As a consequence, the expansion equation turns out to be a kinematic equation in terms of the scale factor and its rates of change.
value estimated in 2014 by the Plank Collaboration [4] , based on combined data from “Plank + WP + high L + BAO”. It is also shown that the contribution of the cosmological constant is to cancel the pressure term in the Friedmann acceleration equation. As a consequence, the expansion equation turns out to be a kinematic equation in terms of the scale factor and its rates of change.
In the next section we develop the new general equation and derive an analytical estimate of the cosmological density parameter. The new analytical solution together with the two existing analytical solutions is presented in Section 3. Comparison of the new analytical solution with the analytical solution involving matter and lamda is presented in Section 4.1. Comparisons of the new analytical solution with the ΛCDM-based models are presented in Section 4.2. In Section 5, the efficacy of the new analytical solution is shown through comparisons with two ΛCDM-based solutions and through comparisons with three sets of observational data.
2. The New General Equation
The first Friedmann equation, including the curvature, k, and the cosmological constant, Λ, is
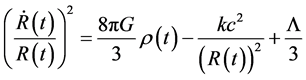 (1)
(1)
Alternatively, including the cosmological term in the total density, the above equation can be represented by
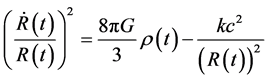 (2)
(2)
where now the density, 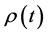 , is defined by
, is defined by
 (3)
(3)
where 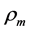 represents the mass density;
represents the mass density;  represents the energy density;
represents the energy density;  represents the radiation energy density and
represents the radiation energy density and  is the intrinsic vacuum energy density, which is defined by
is the intrinsic vacuum energy density, which is defined by
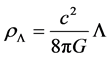 (4)
(4)
The curvature can be represented by
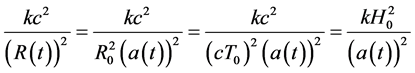 (5)
(5)
where 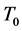 represents the present time and
represents the present time and 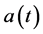 is the scale factor. The Friedmann acceleration equation is given by
is the scale factor. The Friedmann acceleration equation is given by
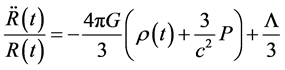 (6)
(6)
Substitutions for 

For the present time, 


To evaluate Λ from Equation (8), we need to evaluate 


To evaluate the present time values of 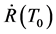


where 
iverse, 


Because at all points the expansion is taking place in all directions, to evaluate the rate of increase of space between any two galaxies, 


Correcting the velocity in the above relation for the effect of time dilation yields

where c represents the speed of light. The value of 

Therefore the corrected expansion velocity at the present time is given by

Also the present time value of the expansion acceleration, according to Equations (13) and (14), is given by

Substitutions for 



Solving the above relation for Λ yields

Therefore the cosmological density parameter can be represented by

Now substitution for Λ from Equation (18) back into Equation (7) yields the Friedmann acceleration equation as

In the next section, assuming a flat universe expanding under the constant pressure



where

and

Assuming a flat universe, i.e., 

This value of the energy density parameter is essentially identical to the 
3. The New Analytical Solution for the Friedmann Equations
Already there exist two analytical solutions for the Friedmann equations. One is for the case of a flat universe containing only matter, 


where





Assuming the pressure to be constant implies that in the above equation the term


pressure being equal to the negative of the vacuum density as discussed by Carroll [6] . Thus the Friedmann acceleration equation is further simplified to the following form:

It is clear that the above equation satisfies the present time boundary conditions as given in Equations (9), (15) and (16). To non-dimensionalize the above equation, let 


where now dot denotes differentiation with respect to



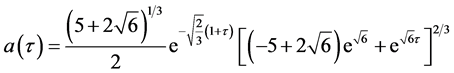
The beauty of the above analytical solution is the fact that it does not involve the fractional components forming the mix of the universe. Using the Mathematica code [7] , a plot of the scale factor as given by the above equation together with its first and second derivatives is presented in Figure 1.
As seen from the above figure, at the present time, 



Considering Equation (18), it is clear that in Equation (20), the pressure 
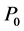
In the following subsections the analytical solution given by Equation (29) is compared with the analytical solution for a universe containing only matter and lambda as given by Equation (25). It is also compared with other models based on ΛCDM parameterizations.
4. Comparison of Analytical Solutions
In order to compare the analytical solution given by Equation (29) with the one given by Equation (25), we need to first decide on the value of 


Figure 1. Evolution of the scale factor.
Figure 2. Evolution of the scale factor with time.
The time 


4.1. Comparisons of the New Solution with ΛCDM-Based Models
The ΛCDM-based models characterize the universe with a limited number of energy density parameters as fractions of constituent ingredients. The values of these fractions are estimated through finding the optimum fit to the observationally measured data. The results are presented in terms of distance modulus versus redshift. Here, a comparison of the variation of scale factors versus redshift will be carried out first. The relation between the scale factor and the redshift, z, is defined by

Thus

To express the scale factor given by Equation (29) in terms of redshift, substitution for the scale factor, a, from Equation (29) back into Equation (31) yields the relation between the red shift, z, and the time 

Using the above equation, the variation of time versus the redshift is presented in Figure 3.
In Figure 3 the present time, 

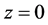


are tabulated for values of 

Figure 3. Variation of time with the redshift.
versus the tabulated values of the redshift, z, in Figure 4. Through this process, 

For ΛCDM-based models, considering Equation (30), and rewriting Friedmann Equation (2) in terms of fractions of constituent densities, for a universe containing mass, energy, radiation and curvature, one obtains the 
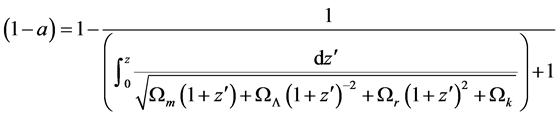
Using the above equation, the values of 
In the next subsection the analytical curve and the curves based on ΛCDM parameterization are compared with the observational data.
Figure 4. Comparisons of scale factors.
Table 1. Cosmological parameters used for ΛCDM based models.
4.2. Comparison of the New Solution with Observational Data
In this part the curve of the analytical scale factor given by Equation (29), transformed through Equation (32), is compared with the curves based on ΛCDM, as given by Equation (33), for the two different sets of ingredients presented in Table 1. To check how well these curves represent the reality, the following three sets of observational data will be used:
1) A set of 557 SNe data with redshifts from a low of 

2) A set of 394 extragalactic distances to 349 galaxies at cosmological redshifts significantly higher than the Union2 Compilation with redshifts from a low of 

3) A set of data for a quasar and the three most distant recently confirmed galaxies, as presented in Table 2.
To compare with the aforementioned observational data, the scale factors have to be presented in terms of distance modulus and redshift. The SNe and the Union2 data are already available in terms of distance modulus and redshift. The data for the galaxies and for the quasar are listed in Table 2, and, for the new analytical solution, the data for the scale factors in terms of distance modulus and redshift are represented through the following relation

where 


tions including the instrument corrections and the K-correction. These are well known corrections and they are
considered in various ways [17] - [20] . We evaluate the factor 
with the first set of the observational data. Then we check the validity of its value through comparisons with the
second and third sets of observational data as well as with the ΛCDM-based curves. To evaluate
the analytical curve, we only need to have the value for the Hubble constant. The recent estimated values of the
Hubble constant based on observational data are: (the Seven-Year Wilkinson Microwave Anisotropy Probe [21] , 2011),





Because this value is remarkably consistent with the observationally estimated values, we will use this value and substitute it together with the values of

Table 2. Data for recently confirmed galaxies.
Figure 5. Hubble diagram, evaluation of the factor
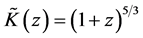
As can be seen from Figure 5, with this 


where



Now, to check the validity of the factor

on Equations (29) and (32), and the two ΛCDM curves based on Equation (33), are presented in Figures 6-9. As seen from these figures, in all cases, the analytical curve is remarkably consistent with the observational data as well as with the ΛCDM-based curves. The log-linear plots and the linear plots show how well the curves represent the observational data at the low and high values of the redshifts respectively.
The excellent match of the analytical curve and the ΛCDM curves with the second and the third set of the observational data validates Equation (36) representing the factor
luated value for the Hubble constant as given in Equation (35). It should be noted that, except for the flatness assumption, the analytical solution is completely general. It includes all the ingredients forming the universe. The ΛCDM-based solutions only consider specific combinations of limited numbers of ingredients.
5. Summary and Remarks
The value of the energy density parameter was analytically estimated to be
Figure 6. Hubble diagram, comparison of analytical and ΛCDM-1 curves with observational data.
Figure 7. Hubble diagram, comparison of analytical and ΛCDM-1 curves with observational data.
tency of the analytical solution with the observational data, as well as with the -based models, provide the necessary confidence in the fidelity of the analytical solution in the representation of reality.
The pressure is cancelled from the Friedmann acceleration equation through the contribution of the cosmological constant. As the result, Equation (28) may be interpreted as a kinematic equation. Its solution, Equation
Figure 8. Hubble diagram, comparison of analytical and ΛCDM-2 curves with observational data.
Figure 9. Hubble diagram, comparison of analytical and ΛCDM-2 curves with observational data.
(29) describes the evolution of the expansion of space. As such, this equation provides an alternative way of describing the expansion of space without involving the controversial dark energy.
Cite this paper
Naser Mostaghel, (2016) A New Solution for the Friedmann Equations. International Journal of Astronomy and Astrophysics,06,122-134. doi: 10.4236/ijaa.2016.61010
References
- 1. Carroll, S.M. (2013) Why Does Dark Energy Make the Universe Accelerate? Posted on 16 November.
http://www.preposterousuniverse.com/blog/2013/11/16/ - 2. Christiansen, J.L. and Siver, A. (2012) Computing Accurate Age and Distance Factors in Cosmology. arXiv: 1204. 0039v1 [astro-ph.CO], 30 March.
- 3. Ullrich, P. (2007) Exact and Perturbed Friedmann-Lemaitre Cosmologies. University of Waterloo, Ontario.
http://www-personal.umich.edu/~paullric/Ullrich-MastersThesis.pdf - 4. Ade, P.A.R., et al., Plank Collaboration (2014) Plank 2013 Results. XVI. Cosmological Parameters. arXiv: 1303. 5076v3 [astro-ph.CO], 20 March 2014.
- 5. Mostaghel, N. (2015) An Analytical Estimate of the Hubble Constant. American Journal of Astronomy and Astrophysics, 3, 44-49.
http://dx.doi.org/10.11648/j.ajaa.20150303.13 - 6. Carroll, S.M., Press, W.H. and Turner, E.L. (1992) The Cosmological Constant. Annual Review of Astronomy and Astrophysics, 30, 499-542.
http://www.nr.com/whp/CosmoConstAnnRev.pdf - 7. Wolfram Mathematica (2011) Version: 8.01.0.
- 8. Riess, A.G. (2012) Nobel Lecture: My Path to the Accelerating Universe. Reviews of Modern Physics, 84, 1165-1175.
http://dx.doi.org/10.1103/RevModPhys.84.1165 - 9. Schmidt, B.P. (2012) Nobel Lecture: Accelerating Expansion of the Universe through Observation of Distant Supernovae. Reviews of Modern Physics, 84, 1151-1163.
http://dx.doi.org/10.1103/RevModPhys.84.1151 - 10. Perlmutter, S. (2012) Nobel Lecture: Measuring the Acceleration of the Cosmic Expansion Using Supernovae. Review of Modern Physics, 84, 1127-1149.
http://dx.doi.org/10.1103/RevModPhys.84.1127 - 11. Amanullah, R., Lidman, C., Rubin, D., Aldering, G., Astier, P., Barbary, K., et al. (2010) Spectra and Hubble Space Telescope Light Curves of Six Types Ia Supernovae at 0.511< z < 1.12 and the Union2 Compilation. The Astrophysical Journal, 716, 712-738.
http://supernova.lbl.gov/Union/figur...n2_mu_vs_z.txt
http://dx.doi.org/10.1088/0004-637X/716/1/712 - 12. Mador, B.F. and Steer, I.P. (2008) NASA/IPAC Extragalactic Database Master List of Galaxy Distances. NED-4D.
http://ned.ipac.caltech.edu/level5/NED4D/ - 13. Zitrin, A., Labbe, I., Belli, S., Bouwens, R., Ellis, R.S., Roberts-Borsani, G., Stark, D.P., Oesch, P.A. and Smit, R. (2015) Lyman-Alpha Emission from a Luminous z = 8.68 Galaxy: Implications for Galaxies as Tracers of Cosmic Reionization. The Astrophysical Journal Letters, 810, L12.
https://en.wikipedia.org/wiki/List_of_the_most_distant_astronomical_objects>http://dx.doi.org/10.1088/2041-8205/810/1/L12
https://en.wikipedia.org/wiki/List_of_the_most_distant_astronomical_objects - 14. Oesch, P.A., Van Dokkum, P.G., Illingworth, G.D., Bouwens, R.J., Momcheva, I., Holden, B., Roberts-Borsani, G.W., Smit, R., Franx, M., Labbé, I., González, V. and Magee, D. (2015) A Spectroscopic Redshift Measurement for a Luminous Lyman Break Galaxy at z = 7.730 Using Keck/MOSFIRE. The Astrophysical Journal Letters, 804, L30.
http://dx.doi.org/10.1088/2041-8205/804/2/L30 - 15. Finkelstein, S.L., Papovich, C., Dickinson, M., Song, M., Tilvi, V., Koekemoer, A.M., et al. (2013) A Galaxy Rapidly Forming Stars 700 Million Years after the Big Bang at Redshift 7.51. Nature, 502, 524-527.
http://dx.doi.org/10.1038/nature12657 - 16. Matson, J. (2011) Brilliant, but Distant: Most Far-Flung Known Quasar Offers Glimpse into Early Universe. Scientific American, 29 June 2011.
http://eso.org/public/news/eso1122/ - 17. Peebles, P.J.E. (1993) Principles of Physical Cosmology. Princeton University Press, Princeton.
- 18. Peacock, J.A. (1999) Cosmological Physics. Cambridge University Press, Cambridge.
- 19. Hogg, D.W., Baldry, I.K., Blanton, M.R. and Eisenstein, D.J. (2002) The K Correction. arXiv:astro-ph/0210394v1.
- 20. Saunders, C., Aldering, G., Antilogus, P., Aragon, C., Bailey, S., Baltay, C., et al. (2014) Type IA Supernova Distance Modulus Bias and Dispersion from K-Correction Errors: A Direct Measurement Using Lightcurve Fits to Observed Spectral Time Series. The Astrophysical Journal, 800, 57.
http://dx.doi.org/10.1088/0004-637X/800/1/57 - 21. Larson, D., Dunkley, J., Hinshaw, G., Komatsu, E., Nolta, M.R., Bennett, C.L., et al. (2011) Seven-Year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Sky Maps, Systematic Errors, and Basic Results. Astrophysical Journal Supplement Series, 192, 16.
http://dx.doi.org/10.1088/0067-0049/192/2/16 - 22. Bennett, C.L., Larson, D., Weiland, J.L., Jarosik, N., Hinshaw, G., Odegard, N., et al. (2013) Nine-Year Wilkinson Microwave Anisotropy Probe (WMAP) Observations: Maps and Results. Astrophysical Journal Supplement Series, 208, 20.
http://dx.doi.org/10.1088/0067-0049/208/2/20 - 23. Reid, M.J., Braatz, J.A., Condon, J.J., Lo, K.Y., Kuo, C.Y., Impellizzeri, C.M.V. and Henkel, C. (2013) The Megamaser Cosmology Project IV. A Direct Measurement of the Hubble Constant from UGC 3789. Astrophysical Journal, 767, 154.
http://dx.doi.org/10.1088/0004-637x/767/2/154











