Received 12 July 2015; accepted 4 September 2015; published 7 September 2015

ABSTRACT

The convergence theory of ideals in generalized topological molecular lattices is studied. Some properties of this kind of convergence are investigated. Finally, the relations between convergence theories of both molecular nets and ideals in GTMLs are discussed together with the GT₂ separation axiom.

Keywords:

Generalized Topological Molecular Lattice, Continuous GOH, Convergence of Molecular Nets, Convergence of Ideals

1. Introduction

After Wang [1] introduced the theory of topological molecular lattices or TMLs for short, several authors established various kinds of convergence theory in TMLs by using a corresponding concept of remote neighborhoods (see e.g. [2] , [3] - [5] ). The theory of remote neighborhood has been established first by Wang [1] as a dual notion of Pu and Liu’s theory of the quasi-coincident neighborhoods in fuzzy topology [6] [7] .

In [8] , we introduced a generalization of Wang’s topological molecular lattice called generalized topological molecular lattice or briefly GTML and studied the convergence theory of molecular nets by using the concept of generalized remote neighborhoods in these spaces.

In this paper, we aim to study the convergence of ideals in GTMLs and investigate the relations among this notion and that of molecular nets. Moreover, we state the relations with other defined topological notions in GTMLs such as generalized order homomorphism or GOH for short.

The paper is organized as follows. In Section 2, we will review some useful concepts in the paper. In Section 3, we will study the convergence in GTMLs in terms of ideals and investigate some properties of such conver- gence. Furthermore, we show the relations between convergence of ideals and the continuity of GOHs. In Section 4, we will discuss the relations between convergence of molecular nets and convergence of ideals in TMLs. Finally, Section 5 presents our conclusions.

2. Preliminaries

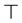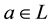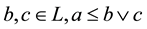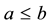This section is devoted to recall some useful concepts which is required in the sequel. Let L be a complete lattice with the smallest element and the largest element, an element is said to be a molecule (some time called co-prime or join-irreducible) if for then or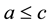. The set of all mole- cules in L is denoted by. The subset 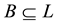is called a minimal family of a [1] , if the following two conditions are hold:

a).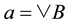

b) If 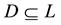and, then 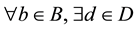such that.

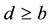The greatest minimal family of a is denoted by 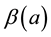while.

Throughout this paper, the entry 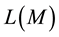denotes a molecular lattice, that is a lattice L and the set of its molecules M. For a non empty subset I of a complete lattice L, I is said to be an ideal [9] , if it satisfies the following conditions:

a) For and.

b) For all.

c).

Definition 2.1 [8] Let be a molecular lattice. A subfamily is said to be a generalized closed topology, or briefly, generalized co-topology, if

(T₁) is closed under arbitrary intersections;

(T₂).

The pair is called a generalized topological molecular lattice, or briefly, GTML.

Definition 2.2 [8] Let be a GTML, , and. Then F is said to be a genera- lized remote neighborhood of a. The set of all generalized remote neighborhoods of a will be denoted by.

Recall that according to the definition of ideals, the family is not necessary be an ideal in GTMLs while the family satisfies the ideal conditions.

For a GTML and, the intersection of all η-elements containing A is called the gene- ralized closure of A and denoted by. that is,

Definition 2.3 [8] Let be a GTML, , then a is said to be an adherence point of A, if for all, we have.

It is clear that a is an adherence point of A if and only if.

Definition 2.4 [1] Let and be complete lattices. A mapping is said to be a generalized order homomorphism or GOH for short if

a) if and only if.

b) f is join preserving, i.e;.

c) is join preserving, where,.

Definition 2.5 [8] Let and be GTMLs and be a GOH, then f is called:

1) continuous GOH, if for every, we have.

2) continuous at a molecule, if for every, we have.

For a directed set D and, the mapping is called a molecular net and denoted by. The molecular net s is said to be in A, if.

The molecular net S is said to be:

1) eventually in A if there exists such that, we have.

2) frequently in A if for all there exists such that.

Definition 2.6 [8] Let be a GTML, be a molecular net and, then:

1) a is called a limit point of S, if eventually true, and denoted by. The join of all limit points of S will be denoted by.

i.e,.

2) a is called a cluster point of S, if frequently true, and denoted by. The join of all cluster points of S will be denoted by.

i.e,.

Definition 2.7 [8] Let be a GTML, then is said to be a Gt₂, if, there exists and such that.

3. Convergence of Ideals in GTMLs

The aim of this section is to study the convergence in GTMLs in terms of ideals and investigate some properties of such convergence. Furthermore, we show the relations between convergence of ideals and the continuity of GOHs.

For the sake of convenience and no confusion, throughout this section and forwards, we restrict the attention of generalized remote neighborhoods of an element a in GTMLs into the set instead of.

Definition 3.1 Let be a GTML, be an ideal of L and, then

1) a is said to be a limit point of I if, denoted by. In this case, we say that i converges to a.

The join of all limit points of i will be denoted by.

2) a is said to be a cluster point of I if and, we have, denoted by. In this case, we say that i accumulates to a.

The join of all cluster points of i will be denoted by.

As a consequence, we obtain the following proposition:

Proposition 1 Let be a GTML, i and J be ideals of L with and. Then we have:

1).

2).

3).

4).

Proof.

1) Let, then. Thus, and hence.

Therefore, we have.

2) Let, then and, we have.

Since, then and hence and,.

Therefore, we have.

3) Let, then. Since, then we get. So,.

Therefore, we have.

4) Let, then and,. But, then and, we have. Therefore, we have. □

Theorem 2 Let be a GTML, I be an ideal of L and, then

1) if and only if.

2) if and only if.

Proof.

1) Let, by the definition of, it is clear that.

Conversely, let and, then and hence. So, there exists such that, then. Thus, we have but, hence. Therefore,.

2) Let, then similarly to 1), is clear.

Now, let and, than and hence. So, there exists such that, then. Thus, for all, also, we have. So, . Therefore,. □

Corollary 1 Let be a GTML, I be an ideal of L and, then

1) if and only if.

2) if and only if.

Theorem 3 Let be a GTML, , and, then if and only if there exists an ideal I in L such that and.

Proof. Since, then a is an adherence point of a, i.e;.

Put, then is an ideal and clearly that also, we have which implies.

Let, then, we have, i.e;. Since, then. So, by Definition 2.3, a is an adherence point of A and hence. □

Lemma 1 Let and be GTMLs, be a GOH, and I be an ideal in. Then the set

is an ideal in.

Proof. It is easily to check the conditions of ideals. □

Theorem 4 Let and be GTMLs, be a continuous GOH at and I be an ideal in. If, then.

Proof. Let f be a continuous GOH at and I be an ideal in with, then,

we have. Hence, we get that and for every. so,

which implies that.

Therefore,. □

Theorem 5 Let and be GTMLs, be a GOH, then f is continuous GOH if and only if for every ideal I of,.

Proof. Let I be an ideal of such that, hence. We need to show that. Since f is a continuous GOH, then f is continuous at and. Hence, by Theorem 4, we get, i.e;.

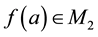
Since f is a GOH, then f preserves arbitrary joins and hence.

We want to prove that f is continuous at every, i.e;, we have

.

Assume that. Hence, there exists an ideal i such that and. Then

which implies that. Thus,.

So, , we have. By the definition of, there exists such that

equivalently that. Hence, but, so

. Contradiction.

Then, and hence.

Therefore, f is continuous GOH. □

4. Relations between Convergence of Molecular Nets and Convergence of Ideals in GTMLs

In [3] and [5] , the authors introduced a comparison between convergence of molecular nets and convergence of ideals in TMLs. In similar way, we discuss the relations between them in GTMs.

For a generalized topological molecular lattice, let I be an ideal in L, then the set

is a directed set with respect to the relation “”defined as

Set, then the set

is a molecular net in called the molecular net generated by the ideal I.

Now, let be a molecular net in L, then the set

is an ideal in l called the ideal generated by S.

Theorem 6 Let be a GTML, be an ideal in l and S be a molecular net in L, then we have

1), (resp.).

2).

3).

Proof. 1) Case I: Let, then eventually, i.e; there exists such that, we have. Hence, we get, so but which implies that.

Therefore, and.

Conversely, let, then. Since, then and such that, we have, but, hence. Thus,.

Case II: Let, and, then there exists with. Thus, , since, there exists such that and. Since , then but, so.

Thus, and,. Therefore,.

Conversely, we need to show that eventually. Let, then and .

Now, , we have, therefore, such that and. So, and,.

Therefore, frequently and.

2) Let, then. By the definition of, we have eventually which means that.

Conversely, let, then eventually. So, , i.e, which means.

3) Let, then there exists such that and with

, we have. But B ≥ A, hence, i.e; eventually.

Thus, and.

Now, let, then eventually, i.e; there exists such that , we have. Since and, then. Hence, and, then and.

Therefore,. □

According to Theorem 6, one can get directly the following result:

Corollary 2 Let be a GTML, I be an ideal in L and S be a molecular net in L, then the following statements hold:

1).

2).

3).

Theorem 7 Let be a GTML, I be an ideal in L and S be a molecular net in L, then we have

.

Proof. Let, then. So, we need to show that.

Now, frequently. Also, eventually and hence, fre- quently. So, and, we get.

Therefore, and hence,. □

In 1986, Yang [9] introduced the concepts of maximal ideals and universal nets.

Definition 4.1 [9] An ideal I in a complete lattice L is called a maximal ideal , if for each ideal J in L such that, we have.

Definition 4.2 [9] A molecular net S in a complete lattice L is called a universal net , if there exists a maximal ideal in L such that S is a subnet of.

Proposition 8 Let be a GTML and I be a maximal ideal in L, then

.

Proof. It is clear that. Now, we prove that.

Let, then. Put

Then J is an ideal in L and clearly that and.

Since I is a maximal ideal in L, we get, hence.

So, and. Therefore,. □

Theorem 9 Let be a GTML, then the following conditions are equivalent:

(i) For every ideal I, such that.

(ii) For every maximal ideal I, such that.

(iii) For every universal net S, such that.

Proof. Let I be a maximal ideal, by (i), such that. Since, I is a maximal, then by Proposition 8, we have.

Let I be an ideal, then there exists a maximal ideal J with and such that. Hence,.

So, and. Thus,.

Let S be a universal net and, then by the definition, there exists a maximal ideal I such that S is a subnet of. By (ii), we have and hence. Therefore,.

Let I be a maximal ideal, then is a universal net, by (iii), such that. Then, we get. □

Lastly, we conclude this section by studying the relation between the ideal convergence and the GT₂ separa- tion axiom in GTMLs.

Theorem 10 Let be a GTML, then it is GT₂, if and only if for every ideal I in L, contains no disjoint molecules.

Proof. Let be GT₂, I be an ideal in L. Assume that with. Then there exists and such that. Since and, we have that and. Hence, which implies that. Contradiction with the definition of I.

Therefore, contains no disjoint molecules.

Assume that is not GT₂, then with and, we have. Put

Then I is an ideal in L with and. Hence, limI contains two disjoint molecules which contradicts the assumption. Therefore, is GT₂. □

Corollary 3 Let be a GTML, then the following statements are equivalents:

a) is a GT₂.

b) For every molecular net S and every, we have.

c) For every ideal I in L and every, we have.

5. Conclusion

In this paper, we introduced a convergence theory of ideals in generalized topological molecular lattices by using the concept of generalized remote neighborhoods and studied some of its characterization and properties. Then, we investigated the relations between the ideal convergence and the continuity of GOH in GTMLs. Finally, we discussed the relations among the convergence theories of both ideals and molecular nets and also the GT₂ separation axiom.

Cite this paper

KamalEl-Saady,FatimaAl-Nabbat, (2015) Ideal Convergence in Generalized Topological Molecular Lattices. Advances in Pure Mathematics,05,653-659. doi: 10.4236/apm.2015.511059

References