Open Journal of Microphysics
Vol.09 No.01(2019), Article ID:90333,9 pages
10.4236/ojm.2019.91001
Pseudo-Hermitian Matrix Exactly Solvable Hamiltonian
Ancilla Nininahazwe
Institut de Pédagogie Appliquée, Université du Burundi, Bujumbura, Burundi
Copyright © 2019 by author(s) and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY 4.0).
http://creativecommons.org/licenses/by/4.0/



Received: January 9, 2019; Accepted: January 28, 2019; Published: January 31, 2019
ABSTRACT
The non PT-symmetric exactly solvable Hamiltonian describing a system of a fermion in the external magnetic field which couples to a harmonic oscillator through some pseudo-hermitian interaction is considered. We point out all properties of both of the original Mandal and the original Jaynes-Cummings Hamitonians. It is shown that these Hamiltonians are respectively pseudo-hermitian and hermitian [1] [2] . Like the direct approach to invariant vector spaces used in Refs. [3] [4] , we reveal the exact solvability of both the Mandal and Jaynes-Cummings Hamiltonians after expressing them in the position operator and the impulsion operator.
Keywords:
Pseudo-Hermiticity, Exact Solvability, Direct Method

1. Introduction
Several new theoretical aspects in quantum mechanics have been developed in last years. In the series of papers [5] [6] , it is shown that the traditional self adjointness requirement (i.e. the hermiticity property) of a Hamilton operator is not necessary condition to guarantee real eigenvalues and that the weaker condition PT-symmetry of the Hamiltonian is sufficient for the purpose. Following the theory developed in Refs. [5] [6] , let’s remind that a Hamiltonian is invariant under the action of the combined parity operator P and the time reversal operator T if the relation is proved (i.e. PT-symmetry is said to be broken). As a consequence, the spectrum associated the previous Hamiltonian is entirely real.
An alternative property called pseudo-hermiticity for a Hamiltonian to be associated to a real spectrum is shown in details in the Refs. [1] [2] .
Referring the ideas of [1] [2] , we recall here that a Hamiltonian is said to be pseudo-hermitian if it satisfies the relation , where denotes an invertible linear hermitian operator.
Another direction of quantum mechanics is the notions of quasi exact solvability and exact solvability [7] [8] [9] [10] .
In the last few years, a new class of operators has been discovered. This class is intermediate between exactly solvable operators and non solvable operators. Its name is the quasi-exactly solvable (QES) operators, for which a finite part of the spectrum can be computed algebraically.
This paper is organized as follows:
In Section 2, we briefly describe the general model which is expressed in terms of the creation and the annihilation operators. We show that the Hamiltonian describing the model is pseudo-hermitian if , or it is hermitian if .
In Section 3, we show in details the properties of the Mandal Hamiltonian namely the non-hermiticity, the non PT-symmetry, the pseudo-hermiticity and the exact solvability.
In Section 4, as in the previous section, it was pointed out that the original Jaynes-Cummings Hamiltonian is hermitian and exactly solvable.
2. The Model
In this section, we consider a Hamiltonian describing a system of a fermion in the external magnetic field,
which couples the harmonic oscillator interaction (i.e.
) and the pseudo-hermitian interaction if
, or the hermitian interaction if
(i.e.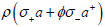 ) [1] [2] :
) [1] [2] :
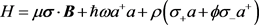 , (1)
, (1)
where
, denote Pauli matrices,
, are real parameters,
, a refer the creation and annihilation operators respectively satisfying the usual bosonic commutation relation
, and .
Recall that the matrices and can be expressed in the following matrix forms:
 (2)
(2)
For the sake simplicity, one can choose the external field in the z-direction (i.e. ) in order to reduce the Hamiltonian given by the Equation (1) and it becomes [1] [2] :
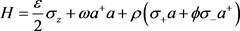 (3)
(3)
with and .
3. Properties of the Original Mandal Hamiltonian
3.1. The Non-Hermiticity
In this section, we reveal that the Hamiltonian described by the Equation (3) is non- hermitian if . It is called Mandal Hamiltonian (i.e. ) and it takes the following form:
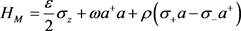 (4)
(4)
Taking account to the following identities:
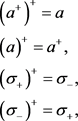 (5)
(5)
let’s show that the Mandal Hamiltonian given by the above Equation (4) is non hermitian:
,
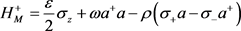 . (6)
. (6)
Comparing the expressions given by the Equations (4) and (6), we see that they are different (i.e. ), as a consequence, we are allowed to conclude that the Mandal Hamiltonian is non-hermitian.
3.2. The Non PT-Symmetry of HM
In this section, we prove that the Mandal Hamiltonian is non PT-symmetric [5] [6] . Recall that the parity operator is represented by the symbol P and the time-reversal operator is described by the symbol T.
The effect of the parity operator P implies the following changes [1] [2] :
 (7)
(7)
Notice also the changes of the following quantities under the effect of the time reversal operator T:
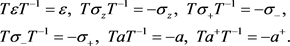 (8)
(8)
Taking account to the relations (7) and (8), one can easily deduce the changes of the Mandal Hamiltonian under the effect of combined operators P et T as follows
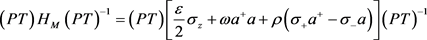 ,
,
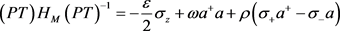 , (9)
, (9)
This above relation (9) can be written as follows
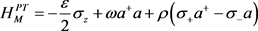 (10)
(10)
Comparing the relations (4) and (10), we see that they are different (i.e. ), it means that the Mandal Hamiltonian is not invariant under the combined action of the parity operator P and the time-reversal operator T. In other words, the Mandal Hamiltonian is not PT-symmetric.
3.3. Pseudo-Hermiticity of HM
In this section, we first prove that the non PT-symmetric Mandal Hamiltonian is pseudo-hermitian with respect to third Pauli matrix [1] [2] :
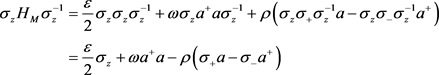 (11)
(11)
with and .
Comparing the Equations (6) and (11), it is seen that the following relation is satisfied:
(12)
Taking account to this above relation, we are allowed to conclude that the Mandal Hamiltonian is pseudo-hermitian with respect to .
Finally, we reveal a pseudo-hermiticity of with respect to the parity operator P:
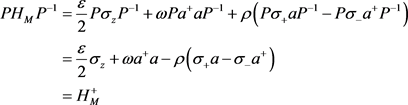 (13)
(13)
Here we have used the relations (7) in order to obtain this above equation (13). As a consequence, one can conclude that the Mandal Hamiltonian is pseudo-hermitian with respect to the parity operator P.
Note that even if is non hermitian and non PT-symmetric, its eigenvalues are entirely real due to the pseudo-hermiticity property [1] .
3.4. Differential Form and Exact Solvability of HM
In this step, our purpose is to change the Mandal Hamiltonian given by the Equation (4) in appropriate differential operator (i.e. is expressed in the position operator x and in the impulsion operator ). Thus, referring to the ideas of exactly and quasi-exactly solvable operators studied in the Refs. [7] [8] [9] [10] , we reveal that preserves a family of vector spaces of polynomials in the variable x.
With this aim, we use the usual representation of the creation and annihilation operators of the harmonic oscillator respectively and a [1] [2] :
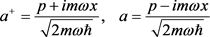 (14)
(14)
where
is the oscillation frequency, m denotes the mass, x refers to the position operator and the impulsion operator is ,
.
,
.
Using appropriate units, we can assume and the operators and a take the following forms:
 (15)
(15)
Replacing the operators and a by their expressions given by this above Equation (15) in the Equation (4), the Mandal Hamiltonian takes the following form:
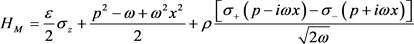 . (16)
. (16)
In order to reveal the exact solvability of the above operator , we first perform the standard gauge transformation [2] :

After some algebraic manipulations, the new Hamiltonian (known as gauge Hamiltonian) is obtained

Replacing the Pauli matrices 



Note that one can easily check if this above gauge Hamiltonian
preserves the vector spaces of polynomials 
Thus, the vector spaces preserved by the operator have the following form

where and denote respectively the polynomials of degree n − 1 and n.
As the gauge Mandal Hamiltonian , it is obvious that the original Mandal Hamiltonian is exactly solvable. Due to this property of exact solvability, the whole spectrum of can be computed exactly (i.e. by the algebraic methods) [1] [2] [3] .
4. Properties of the Jaynes-Cummings Hamiltonian
4.1. The Hermiticity
In this section, considering , the Hamiltonian given by the Equation (3) leads to the standard Jaynes-Cummings Hamiltonian of the following form
(21)
Our aim is now to prove that the above Hamiltonian is hermitian.
Indeed, in order to reveal the hermiticity of the Jaynes-Cummings Hamiltonian given by the above relation (21), the following relation must be satisfied.
Consider now the following relation
, (22)
Taking account to the identities of the relation (5), this above equation leads the following expression:
. (23)
Comparing the Equations (21) and (23), one can write that
. (24)
Referring to this equation (24), it is obvious that the standard Jaynes-Cummings Hamiltonian is hermitian. As a consequence, its eigenvalues are real due to the property of hermiticity.
4.2. Differential Form and Exact Solvability of HJC
Along the same lines as in the above section 3.4, our purpose is to change the Jaynes-Cummings Hamiltonian given by the Equation (21) in appropriate differential operator (i.e. is expressed in the position operator x and in the impulsion operator ).
With this purpose, we use the usual expressions of the creation and annihilation operators of the harmonic oscillator respectively and a given by the Equation (15).
Substituting (15) in the Equation (21), the Jaynes-Cummings Hamiltonian is written now as follows
(25)
Operating on the above operator the standard gauge transformation as
(26)
after some algebraic manipulations, the new Hamiltonian (known as gauge Hamiltonian) is obtained
(27)
Replacing the Pauli matrices and respectively by their matrix form given by the relation (2), the final form of the gauge Hamiltonian is
,
. (28)
Note that one can easily check if this above gauge Hamiltonian preserves the finite dimensional vector spaces of polynomials namely with . As the integer n is arbitrary, the gauge Jaynes-Cummings Hamiltonian is exactly solvable.
As a consequence, its all eigenvalues can be computed algebraically. Indeed, the vector spaces preserved by the operator have the following form
(29)
where and denote respectively the polynomials of degree n − 1 and n.
As the gauge Jaynes-Cummings Hamiltonian , it is obvious that the standard Jaynes-Cummings Hamiltonian is exactly solvable. In other words, all eigenvalues associated to the Hamiltonian can be calculated algebraically (i.e. by the algebraic methods) [1-3].
5. Conclusion
In this paper, we have put out all properties of the original Mandal Hamiltonian. We have shown that the Mandal Hamiltonian is non-hermitian and non-invariant under the combined action of the parity operator P and the time-reversal operator T. Even if the previous properties are not satisfied, it has been proved that the Mandal Hamiltonian is pseudo-hermitian with respect to P and with respect to also. With the direct method, we have revealed that preserves the finite dimensional vector spaces of polynomials namely . Indeed, the Mandal Hamiltonian is said to be exactly solvable [1] [2] [3] [4] . Along the same lines used in Section 3, we have pointed out that the standard Jaynes-Cummings Hamiltonian is hermitian and exactly solvable in Section 4.
Acknowledgements
I thank Pr. Yves Brihaye of useful discussions.
Conflicts of Interest
The author declares no conflicts of interest regarding the publication of this paper.
Cite this paper
Nininahazwe, A. (2019) Pseudo-Hermitian Matrix Exactly Solvable Hamiltonian. Open Journal of Microphysics, 9, 1-9. https://doi.org/10.4236/ojm.2019.91001
References
- 1. Mandal, B.P. (2005) Pseudo-Hermitian Interaction between an Oscillator and a Spin Particle in the External Magnetic Field. Modern Physics Letters A, 20, 655-662. https://doi.org/10.1142/S0217732305016488
- 2. Brihaye, Y. and Nininahazwe, A. (2006) Extended Jaynes-Cummings Models and Quasi-Exact Solvability. Journal of Physics A: Mathematical and General, 39, 1-14. https://doi.org/10.1088/0305-4470/39/31/011
- 3. Rühl, W. and Turbiner, A. (1995) Exact Solvability of the Calogero and the Sutherland Models. Modern Physics Letters A, 10, 2213. https://doi.org/10.1142/S0217732395002374
- 4. Gomez-Ullate, D., Kamran, N. and Milson, R. (2005) Quasi-Exact Solvability and the Direct Approach to Invariant Subspaces. Journal of Physics, A38, 2005-2019.https://doi.org/10.1088/0305-4470/38/9/011
- 5. Bender, C. and Boettcher, S. (1998) Real Spectra in Non-Hermitian Hamiltonians having PT-Symmetry. Physical Reviews Letters, 80, 5243.https://doi.org/10.1103/PhysRevLett.80.5243
- 6. Brihaye, Y. and Nininahazwe, A. (2004) On PT-Symmetric Extensions of the Calogero and the Sutherland Models. International Journal of Morden Physics, A19, 4391-4400. https://doi.org/10.1142/S0217751X04019858
- 7. Turbiner, A.V. (1988) Quasi-Exactly Solvable Problems and sl(2) Algebra. Communications in Mathematical Physics, 118, 467-474.https://doi.org/10.1007/BF01466727
- 8. Ushveridze, A.G. (1995) Quasi-Exactly Solvable Models in Quantum Mechanics. Institute of Physics Publishing.
- 9. Brihaye, Y. and Kosinski, P. (1997) Quasi-Exactly Solvable Matrix Models in sl(n). Physics Letters B, 424, 43-47. https://doi.org/10.1016/S0370-2693(98)00167-1
- 10. Brihaye, Y. and Hartmann, B. (2001) Quasi-Exactly Solvable -Matrix Schrödinger Operators. Modern Physics Letters A, 16, 1895-1906.https://doi.org/10.1142/S0217732301005242

